
Extremely Nondegenerate Two-photon Processes in Semiconductors
David J. Hagan
1,2
, Himansu S. Pattanaik
1
, Peng Zhao
1
, Matthew Reichert
3
and Eric W. Van Stryland
1,2
1
CREOL, The College of Optics and Photonics, University of Central Florida, P.O. Box 162700,
Orlando, FL 32816-2700, U.S.A.
2
Department of Physics, University of Central Florida, Orland, FL 32816, U.S.A.
3
Department of Electrical Engineering, Princeton University, Princeton, NJ 08455 U.S.A.
Keywords: Nonlinear Optics, Semiconductors, Infrared Detectors, Semiconductor Lasers.
Abstract: Direct-gap semiconductors show enhanced two-photon absorption and nonlinear refraction for the extremely
non-degenerate case, i.e. for two light waves of very different wavelength, as compared to the degenerate
case. We have verified this through measurements of non-degenerate two-photon absorption and nonlinear
refraction in several direct-gap semiconductors. We have demonstrated application towards mid-infrared
detection and imaging, as well as 2-photon gain in the mid infrared. We also show how semiconductor
quantum wells may be employed to engineer even larger enhancements of these effects.
1 INTRODUCTION
Two-photon absorption (2PA) in direct-gap
semiconductors has been extensively studied both
experimentally and theoretically, resulting in the
development of well-established scaling rules that
predict the 2PA coefficient of direct-gap
semiconductors. (Van Stryland, et al., 1985) The 2PA
coefficient,
is defined by,
,
(1)
where is the optical irradiance and is the
propagation depth inside the nonlinear material.
is found to scale as
, where
is the band-gap
energy. Therefore, 2PA coefficients in narrow-gap
semiconductors may be several orders of magnitude
greater than in large-gap semiconductors. For
example, in InSb where
= 0.23 eV, it is found that
≈ 2 cm/MW in the wavelength range 8 to 12 μm,
while for ZnO, for which
3.2eV,
≈ 5 cm/GW
at a wavelength of 532 nm. This scaling law may be
applied with similarly accurate predictions for any
direct gap semiconductor.
Moving to the nondegenerate case, where two
photons at different frequencies,
and
, with
corresponding irradiances,
and
, are
simultaneously absorbed, the 2PA coefficient should
be redefined as,
2
;
(2)
where the order of the frequencies in the argument is
important. Here it indicates that here we are observing
the change in irradiance at
due to the presence of
light at
. Nondegenerate 2PA was predicted
(Sheik-Bahae, et al., 1991) to follow:
;
;
,
(3)
where,
;
1
2
1
1
(4)
where
is the Kane energy parameter,
is the
bandgap energy,
and
the respective refraction
indices and K is a parameter which is almost material-
independent, usually taken to be 3100 cm GW
–1
eV
5/2
where all energies are in eV (Sheik-Bahae, et al.,
1991). Note that this predicts 2PA to become very
large in the extremely nondegenerate case, (
≫
), In 2011, our group experimentally demonstrated
that these theoretical predictions work well for
several direct-gap semiconductors, We have observed
J. Hagan D., Pattanaik H., Zhao P., Reicher t M. and Van Stryland E.
Extremely Nondegenerate Two-photon Processes in Semiconductors.
DOI: 10.5220/0006104700650069
In Proceedings of the 5th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2017), pages 65-69
ISBN: 978-989-758-223-3
Copyright
c
2017 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved
65
nondegenerate 2PA coefficients as large as 1 cm/MW
in CdTe, which is similar to the degenerate 2PA
coefficient of InSb at 10.6 μm (Cirloganu, et al.,
2011
)
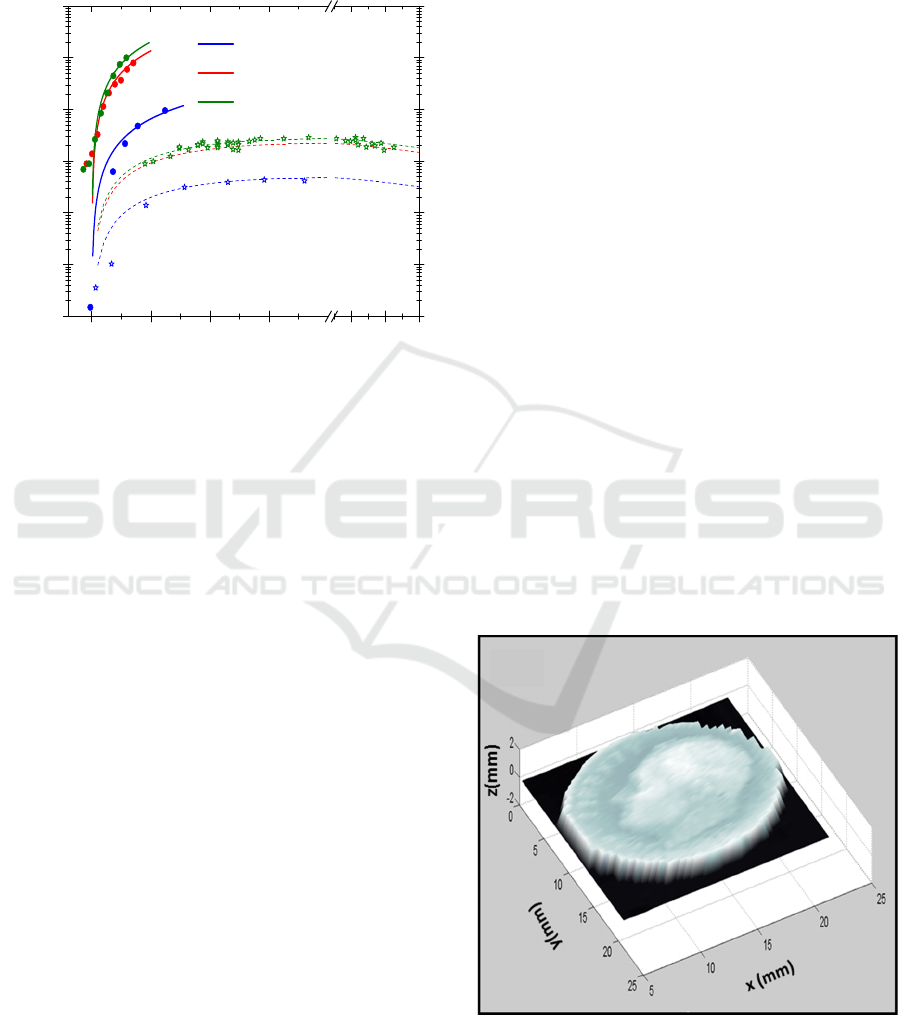
Figure 1 summarizes these early results.
0.50 0.55 0.60 0.65 0.8 0.9 1.0
0.01
0.1
1
10
100
1000
10000
Non-degenerate 2PA
h
pump
= 0.155*E
G
(ZnO)
h
pump
= 0.1*E
G
(GaAs)
h
pump
= 0.097*E
G
(CdTe)
2PA coefficient (cm/GW)
h
eff
/E
g
E
g
(ZnO) = 3.2eV
E
g
(GaAs) = 1.41eV
E
g
(CdTe) ~ 1.5eV
Degenerate 2PA
Figure 1: 2PA coefficient as a function of probe wavelength
normalized by the bandgap energy for ZnO, GaAs, and
CdTe. The solid lines are the prediction of the simple 2-
parabolic band model. The dashed lines are for the
degenerate 2PA along with associated data. (After
Cirloganu, et al., 2011).
This strong enhancement opens up a number of
possible applications for 2PA. As we shall describe
in this paper, we have demonstrated highly sensitive
gated infrared detection via 2PA using conventional
semiconductor photodiodes. (Fishman, et al. 2011,
Pattanaik, et al., 2016a) We have also investigated
and demonstrated that 2-photon gain is similarly
enhanced in the nondegenerate case, leading to the
tantalizing possibility of 2-photon semiconductor
laser devices. (Ironside, 1992). Additionally, we have
now shown that, as one might expect by causality, the
enhancement in 2PA for the nondegenerate case
translates directly to an enhancement of the bound-
electronic nonlinear refraction (NLR) (Sheik-Bahae,
et al., 1991). We describe our recent results that verify
this strong nondegenerate NLR and its large
anomalous dispersion above the 2PA edge. Finally,
we show theoretically that the nondegenerate 2PA
and consequently the 2-photon gain may be enhanced
by yet another order of magnitude by employing
quantum well geometries.
2 EXPERIMENTAL RESULTS
2.1 Infrared Detection using
Nondegenerate 2PA
In 2011, we first demonstrated infrared detection with
standard GaN and GaAs photodiodes using extremely
nondegenerate photon pairs with up to 14:1 energy
ratio (Fishman, et al. 2011). For detection in GaN,
we used a 390 nm strong “gating” pulse, of 100 fs
duration to sensitize a GaN pin photodiode to mid IR
radiation. Mid IR pulses at 5.6 μm were detected
when the gate and IR pulses were temporally
coincident on the detector. The minimum detected IR
pulse energy in uncooled GaN is as low as 20 pJ,
while under identical conditions for a standard cooled
MCT detector, the minimum detectable energy is 200
pJ. Although the quantum efficiency of such detectors
is not quite as high as for a traditional interband
detector, the noise is very low, due to the ultrafast
gating. We have now also demonstrated cw detection
using this method, although the sensitivity is not high
in this case.
Since the detection requires temporal overlap
between signal and gating pulses, it automatically
provides information about the time of arrival of the
signal pulse. We have recently applied this to 3-D
infrared imaging of remote objects, as shown in
Figure 2. (Pattanaik, et al., 2016a) It is worth noting
that this process does not use IR crystals or phase-
matching, as employed by upconversion detection
which is based on second-order nonlinearities.
Figure 2: 2PA-detected 3-D image of a US 10-cent coin.
(After Pattanaik, et al., 2016a.).
PHOTOPTICS 2017 - 5th International Conference on Photonics, Optics and Laser Technology
66

2.2 Application to 2-Photon Gain
Based on reciprocity between absorption and gain, we
also expect a similar effect for the reverse process of
2-photon gain (2PG). Taking the verified theory for
2PA to calculate the 2PG coefficient we find:
(Ironside, 1992)
;
;
;
;
(5)
where
is the 2-photon gain coefficient which is
positive in the case of a population inversion (
). The nondegenerate enhancement in this case may
be applied to the generation of tunable mid-IR
radiation. In Figure 3, we plot the gain as calculated
by Equation 5 for cooled bulk GaAs with a population
inversion. The gain coefficient is plotted versus the
sum of the two photon energies as the larger photon
energy is varied for several fixed values of the smaller
photon energy. The degenerate 2PG coefficient is
also shown for comparison.
Figure 3: 2PG coefficient versus photon energy sum for
bulk GaAs at 20 K with = 2×10
18
cm
-3
for several values
of the nondegeneracy, e.g. 0.10
has IR photons of 0.15
eV or = 8.2 m.
We present experimental data showing
extremely nondegenerate two-photon gain in bulk
GaAs via pump-probe experiments with an additional
optical excitation to generate a population inversion.
A commercial Ti:sapphire chirped-pulse amplifier
system producing 50 fs (FWHM) pulses at 800 nm
and 1 kHz repetition rate is used as an excitation to
generate population inversion in a 4 μm thick GaAs
sample. An optical parametric generator/amplifier
with a difference frequency generator (AgGaS
2
) is
tuned to produce pulses of wavelength 7.75 μm in the
mid-IR, and focused onto the GaAs to be used as a
pump. A white-light continuum is filtered via narrow
bandpass filters (10 nm FWHM) from 977 nm to 947
nm for use as a probe. The pump and probe photon
energies add to be slightly greater than the bandgap
energy of GaAs, where population inversion is
obtained. Changes in transmission of the probe are
monitored as the temporal delay between the pulses
is varied. In the absence of above-gap excitation, the
transmittance decreases as the pump and probe are
overlapped in time, which one would expect for 2PA.
This is indicated by the green squares in Figure 4.
When the above-gap excitation at 800 nm is turned on
so as to produce a population inversion, we expect
both 2PG and free-carrier absorption (FCA). After
using polarization discrimination to eliminate effects
of FCA, as described in (Reichert, et al., 2016), we
are able to observe nondegenerate two-photon gain,
as shown by the blue circles in Figure 4. Although
we observe 2PG, we note that the background losses
due to FCA still surpass the gain, and the observation
of net gain remains a challenge.
Figure 4: The transmittance change as a function of
temporal delay between a 7.75 m pump pulse and a 0.977
m probe without (green squares) and with (blue circles) an
above-gap excitation pulse that creates a population
inversion in GaAs. (After Reichert, et al., 2016).
2.3 Nondegenerate Nonlinear
Refraction
In addition to the extremely nondegenerate 2PA
enhancement, our theory predicts that the nonlinear
1.00 1.02 1.04 1.06 1.08 1.10
0.1
1
10
100
1000
1.00 1.02 1.04 1.06 1.08 1.10
0.1
1
10
100
1000
(cm/GW)
Degenerate
(cm/GW)
Extremely Nondegenerate Two-photon Processes in Semiconductors
67

refractive index
;
is also enhanced for very
different frequencies. Precise knowledge of the
magnitude, sign, and dispersion of
is needed for
design and prediction of Kerr-effect-based photonic
devices such as all-optical switching. Our earlier
theory relates the dispersion of
to nonlinear
absorption spectra via a Kramers-Kronig
transformation, where the major contributing NLA
mechanisms include 2PA, electronic Raman and the
optical (AC) Stark effect. (Sheik-Bahae, et al., 1991)
While the degenerate NLR coefficient
; has
been measured via the Z-scan method (Sheik-Bahae,
et al., 1990), the nondegenerate NLR, namely the
refractive index change at frequency
due to the
presence of a beam at frequency
, of coefficient
;
, is much less explored experimentally,
particularly for the extremely nondegenerate case
(i.e.,
≫
) and for spectral regions where
2PA is present.
Here we describe the results of experiments that
use a 2-beam method, nonlinear beam deflection, for
the measurement of nondegenerate NLR
(Ferdinandus, et al., 2013). Beam deflection utilizes
a strong excitation pulse at
to create an index
change at the sample that is sensed by the probe pulse
at
.
The index change
deflects the probe by a small
angle which is measured using a segmented detector
by taking the difference of the energy falling on the
left and right halves, Δ
. By
normalizing to the total energy , Δ/ is directly
proportional to
;
, and the transmission
change for the gives the NLA and hence the 2PA.
We use a Ti:sapphire laser system to pump an optical
parametric generator/amplifier to generate the
excitation pulses from the idler beam at a wavelength
of
= 2.3 m. A portion of the 800 nm laser output
is used to generate a white-light continuum (WLC) to
be used as the probe. The NLR is measured at several
wavelengths by filtering the WLC using narrow
bandpass filters of bandwidth in the range 10-25 nm
FWHM. The strong group-velocity mismatch
between the pump and probe pulses has to be
accounted for in determining the
. We measured
the dispersion of the nondegenerate NLR in the
direct-gap semiconductors ZnO, ZnSe and CdS. In
Figure 5, we show the measured nondegenerate
for
ZnSe. We see that the measured
follows the
theoretical prediction closely. However, we note that
the nondegenerate enhancement in NLR is not as
large as the enhancement of 2PA.
We are still looking for applications for the
enhanced NLR. The difficulty is that the regions
where it is enhanced are the same regions where 2PA
is enhanced. We have also performed experiments at
wavelengths at the zero crossing for
using
femtosecond pulses. These pulses are spectrally
broad, and we observe that different parts of the pulse
undergo different signs of NLR as is predicted by our
theory. This unusual behaviour may have some
unique applications. For example, combined with
anomalous dispersion, this effect could be applied to
the reduction of chirp in optical pulses.
Figure 5: Measured
;
dispersion (red circles) of
ZnSe, compared to theoretical calculations for
nondegenerate (solid lines) and degenerate (dashed lines)
; Shaded region represents errors from the bandwidth of
the excitation pulse; Degenerate
data is shown for
comparison (black squares).
2.4 Nondegenerate 2PA in
Semiconductor Quantum Wells
Due to their large density of states near the band edge,
it is expected that nondegenerate 2PA will be
enhanced even more in quantum well (QW) structures
than in the bulk. QWs show different linear and
nonlinear optical properties when the incident light
electric field vector polarized is changed from being
in the plane of the QW (TE) to perpendicular to it
(TM). We have developed a theory for ND-2PA in
QWs using second-order perturbation theory and
analytical expressions for the ND-2PA coefficient are
derived for the different polarization combinations.
(Pattaniak, et al., 2016b)
Our results indicate that TM-TM polarization
gives the greatest enhancement, and we show results
for this geometry. Figure 6 shows the 2PA coefficient
calculated for different well thicknesses (each with
infinite well depth) as a function of the normalized
total photon energy. The normalization is done is such
a way that the turn-on of linear absorption occurs at
PHOTOPTICS 2017 - 5th International Conference on Photonics, Optics and Laser Technology
68

the same energy, i.e., with respect to the one-photon
transition energies. This allows comparison of the
ND-2PA coefficient for the bulk and QW
semiconductors on the same scale and also makes
comparison to the respective degenerate 2PA
coefficient easier.
Figure 6: Nondegenerate 2PA coefficient in bulk GaAs and
GaAs QW’s of different widths for the TM-TM case. The
arrows indicate valence band to conduction band transition
energies.
Due to the large energy difference of photon pairs
in the nondegenerate case, for a bulk semiconductor
there is about a hundred-fold increase in
;
over the degenerate case. The plot in Figure 6 is
generated for a pump photon energy
0.12
,
corresponding to a wavelength of 7.5μm and by
varying the probe photon energy
. We have
restricted the probe photon energy,
, to be at least
30 meV below the linear absorption edge. This is
done to keep the linear Urbach–tail absorption low. In
a QW of width 10 nm, we obtain a maximum value
for the nondegenerate 2PA coefficient of
;
≈ 3000 cm⁄GW which is approximately 36 times
larger than predicted and measured in the bulk. These
conditions of large enhancement correspond to where
the mid-IR photon is near resonance with the inter
sub-band transition.
3 CONCLUSIONS
We have predicted and verified that the use of highly
nondegenerate photon energies in two-photon
processes in direct-gap semiconductors leads to
strongly enhanced nonlinear effects. This in turn has
led to the demonstration of sensitive mid-infrared
detection and imaging using large bandgap
semiconductor detectors. It has also led to the
observation of 2-photon gain in an optically pumped
semiconductor. As expected via causality, 2-photon
processes are accompanied by nonlinear refraction
which is also measured to be enhanced in the
extremely nondegenerate case. We have calculated
that the effects of 2-photon absorption and 2-photon
gain should be considerably larger in quantum wells
than in bulk.
ACKNOWLEDGEMENTS
We thank Greg Salamo at the University of Arkansas
for preparing the GaAs sample, and Arthur Smirl of
the University of Iowa and Jacob Khurgin of Johns
Hopkins University for stimulating discussions. This
work was supported by the National Science
Foundation Grants ECCS-1202471, ECCS-1229563,
and DMR-1609895.
REFERENCES
Cirloganu, C. M., Padilha, L.A., Fishman, D. A., Webster,
S.,. Hagan, D. J., and Van Stryland, E. W., 2011, Opt.
Express 19, 22951-22960.
Ferdinandus, M. R., Hu, H., Reichert,, M., Hagan, D. J., and
Van Stryland, E. W., 2013, Opt. Lett. 38, 3518-3521.
Fishman, D. A, Cirloganu, C. M . Webster, S., Padilha,
L.A., Monroe, M., Hagan, D. J., and Van Stryland, E.
W 2011, Nature Photonics 5, 561-565 (2011.
Ironside, C. N., 1992, IEEE J. Quantum Electron, 28, 842-
847.
Pattanaik, H.S., Reichert, M., Hagan, D.J., and Van
Stryland,E. W., 2016a, Opt. Express 24, 1196-1205.
Pattanaik, H.S., Reichert, M., Khurgin , J. B., Hagan, D.J.,
and Van Stryland,E. W., 2016b, IEEE J. Quantum
Electron., 52, 9000114-1--9000114-14.
Reichert, M., Smirl, A.L., Salamo, G., Hagan, D. J., and
Van Stryland, E. W., 2016, Phys. Rev. Lett. 117,
073602.
Sheik-Bahae, M., Said, A.A., Wei, T.-H., Hagan, D.J., and
Van Stryland, E.W., 1990, J. Quant. Electron, 26, 760-
769.
Sheik-Bahae, M., Hutchings, D. C.,. Hagan, D. J. and Van
Stryland, E. W., 1991, IEEE J. Quant. Electron. 27,
1296-1309.
Van Stryland, E. W., Woodall, M.A., Vanherzeele, H, and
Soileau, M. J., 1985, Opt. Lett. 10, 490.
Extremely Nondegenerate Two-photon Processes in Semiconductors
69
