A Pipeline and Metric for Validation of Personalized Human Body

Models

Sukhraj Singh and Subodh Kumar

Computer Science Department, Indian Institute of Technology Delhi, New Delhi, India

Keywords:

Shape Descriptors, Human Body Models, Anatomy, Mesh Validation.

Abstract:

Advanced and personalized Human Body Models (HBM) are increasingly important in human centered indu-

stry design such as passive vehicular safety analysis, using ﬁnite element and other methods. Often accurate

HBMs are painstakingly constructed for median human dimensions, and then modiﬁed and re-sized using per-

sonalization algorithms for various applications. Personalization algorithms rely on various anthropometric

measurements, and sometimes manual intervention, to deform the median HBM. The quality of a personalized

model is often deﬁned in terms of local properties such as aspect ratio of ﬁnite elements produced. In some

cases it is inferred by visual comparison with some ground truth model or by measuring the anthropometric

errors with respect to known values. We seek to deﬁne the quality of deformation in anatomically suitable

geometric terms, which can be automatically computed. To this end, we compare the deformed anatomical

surface meshes with that of the median mesh in a shape descriptor space. Shape comparison and matching is

a well studied area. The tools devised for the same are largely application dependent. We present pipeline and

a metric for validating anatomical surface meshes. It is a problem that has not been extensively studied, even

though general shape comparison and matching techniques abound. Our metric incorporates global and part

based shape signatures. The main contribution of our work is to explore techniques suitable for comparison of

anatomical meshes by non technical experts. We formulate a pipeline that needs minimal user intervention.

1 INTRODUCTION

With increasing use of Human Body Models (HBM)

in human centered industry design there is ongoing

effort to create more detailed advanced Finite Element

based Human Body Models (FE-HBM). FE-HBMs

are particularly important for injury prediction. In the

last decade there has been effort to create highly de-

tailed FE-HBMs for this purpose. Models like HU-

MOS (Robin, 2001), THUMS (Iwamoto et al., 2002)

and more recently GHBMC (Gayzik et al., 2011) are

some of the most popular HBMs in the domain of in-

jury biomechanics research.

Creation of a good quality FE-HBM from CT-scan

data is a tedious and time consuming task, which can

take many person-months. It usually requires manual

intervention and quality control can be challenging.

Due to these reasons FE-HBMs are generally avai-

lable in a standard occupant pose for a median di-

mension of a given population. However, it is really

important to predict the injury for subjects in non-

standard pose, and of non-median shape and size. To

solve this problem, deformation algorithms (Hwang

et al., 2016; Vezin and Verriest, 2005; CEESAR et al.,

2014) are used to automatically resize or personalize

a standard median template HBM within anatomical

constraints. Thus, these algorithms deform an HBM

known to be of a ‘good quality’ to a target HBM sa-

tisfying certain dimensional requirements. The tar-

get dimensions are in some cases derived from target

X-ray or CT-Scan images or from anthropometric in-

formation (Cheng et al., 1996) derived from statisti-

cal shape analysis of a population. Deformation can

be done on an individual anatomical volumetric mesh

of an organ or at the skin of FE-HBM, which con-

sequently deforms the internal anatomical meshes as

well. Again, it is critical that deformation leads to a

model of a ‘good quality’.

Our goal is to validate, or measure the quality of,

such a personalized FE-HBM. This could be done by

directly deﬁning the notion of ‘good’ in mathemati-

cal terms. The general way of computing the quality

of a personalized FE-HBM in bio-mechanics com-

munity is by using distortion measures like scaled

Jacobian, skewness or aspect ratio of the ﬁnite ele-

ments and comparing these values with the baseline

160

Singh S. and Kumar S.

A Pipeline and Metric for Validation of Personalized Human Body Models.

DOI: 10.5220/0006176201600171

In Proceedings of the 12th International Joint Conference on Computer Vision, Imaging and Computer Graphics Theory and Applications (VISIGRAPP 2017), pages 160-171

ISBN: 978-989-758-224-0

FE-HBM (Lalonde et al., 2013) and then manually

evaluating simulation results. It is also done by mea-

suring the errors in anthropometric measurements of

the personalized HBM or that of palpable landmarks

of internal organs with that of actual human (Poulard

et al., 2012) or by visual comparison with the baseline

HBM. An alternative or rather complementary way to

assess quality is by comparing the geometric proper-

ties of a surface mesh extracted from an anatomical

volumetric mesh with an accurate anatomically cor-

rect baseline surface mesh.

In computer graphics, 3D shapes are often repre-

sented as polygonal, or triangular, surface meshes.

Comparison and matching of two shapes is funda-

mental in shape analysis. With the advancement in

3D capture technologies there has been a continuous

expansion of shape databases. Consequently, signiﬁ-

cant advances in shape analysis have been made in the

area of shape retrieval and shape matching. A large

number of global shape signatures have been introdu-

ced so as to have efﬁcient and correct shape retrieval

(Iyer et al., 2005; Tangelder and Veltkamp, 2007).

The other important work in shape analysis is the

analysis of the property of a mesh after it has un-

dergone mesh processing algorithms like simpliﬁca-

tion, smoothing, watermarking, compression or de-

formation. These processed meshes are often com-

pared with the original mesh using absolute measures

like dihedral angles or other distortion measures. Me-

trics like Minkowski distance or Hausdorff distance

are also used to ﬁnd the difference between meshes

(Aspert et al., 2002; Cignoni et al., 1998). More re-

cently, metrics like MSDM2 by (Lavou, 2011) have

been introduced which are human perception oriented

distortion measures. However using such methods as-

sumes that the two meshes are in the same coordinate

space and fully aligned. There are other visualization

based comparison approaches where Gaussian curva-

ture or Mean curvature is used to color the mesh so as

to facilitate visual comparison of the mesh (Zhou and

Pang, 2001). This requires signiﬁcant user interven-

tion due to the visual inspection involved.

Further, many shape analysis methods depend on

the kind of shape domains they are applied to, e.g.,

man-made shapes, natural shapes, CAD models, etc.

Human anatomy is one such domain where these

shape analysis methods have not been applied exten-

sively. Although there has been research focused in

the image domain for anatomical organs, 3D surface

mesh analysis of human anatomical organs is not well

explored.

In particular, in the anatomical context, some de-

formations are acceptable, even desirable, while ot-

hers are not. For example, lengthening or fattening

of a Femur bone is likely due to the target dimen-

sion. On the other hand, a bulging lesser trochanter

implies a poor deformation. Thus purely geometric

distance between a median mesh and a personalized

mesh would not sufﬁce. Our research has lead us to

the set of local and global geometric features, each al-

ready found in the literature, which together provide

a usable metric to help guide the personalization al-

gorithm.

The main contribution of our work is a 3D anato-

mical surface mesh comparison pipeline and a quality

metric – two scalar values that provide a measure of

similarity between a template mesh and a deformed

candidate mesh. Again, the candidate mesh is not ex-

pected to ‘match’ the template mesh in the sense that

shape matching algorithms depend on. Rather, it is li-

kely to be non-linearly deformed. Our metric focuses

on the fact that ‘good’ meshes contain all anatomical

parts in the right conﬁguration and of the right relative

dimensions. In order to compute such a metric, we

• Incorporate various geometric feature based sig-

natures for anatomical meshes validation.

• Implement a part-based mesh validation pipeline,

which considers the local part based features, and

relational features between adjacent parts.

• Model the mesh validation problem as a graph

matching problem.

• Coin a resultant validation metric, which consi-

ders the local, relational and global geometric fe-

atures.

• Test the devised pipeline and metric for 3D anato-

mical surface meshes.

The remainder of the paper is organized as fol-

lows: in Section 2 we present the various proces-

sing blocks of the pipeline, Section 3 provides details

about the segmentation algorithm used to ﬁnd parts,

Section 4 provides detail about various shape signa-

tures used and also the formulation of graph. Further,

Section 5 provides details about the algorithm used

for ﬁnding correspondence with an optional segment

merge step detailed in Section 6 and Section 7 details

metric computation. Finally, in Section 8 we discuss

the results of the pipeline and Section 9 concludes

with limitations and future scope of work.

2 THE PIPELINE

In our work we have mainly used 3D surface mes-

hes of human skeletal system, primarily of the lower

limb region. On the basis of shape, anatomists bro-

adly classify the bones as a) Long bones b) Short bo-

nes c) Irregular bones and d) Flat bones (Gray, 2001).

A Pipeline and Metric for Validation of Personalized Human Body Models

161

A particular bone may further be classiﬁed into parts

based on its topography such as shaft, fovea, fossa,

neck, head, condyles, etc. These are essentially anato-

mical terms whose analogy would be parts like, cylin-

drical, depression, protruding, spherical, rough, etc.

However, a surface mesh, by construction, may not be

able to represent all such features of the bone, it de-

pends on the resolution of the mesh and the purpose

for which a mesh is constructed.

We envisage to incorporate geometric features that

cater to the above classiﬁcation of bony anatomy. Fe-

atures based on Eigen values help us gain an insight

into the overall shape of a bone, the shape diame-

ter function (Gal et al., 2007) caters to variability in

shape, and shape index values based signature (Koen-

derink and van Doorn, 1992) considers detailed sur-

face characteristics of a bone. The work of (Lavou

et al., 2013) also tries to ﬁnd an optimal metric by

linearly combining various metrics with weights pre-

dicted using machine learning techniques applied on

LIRIS Masking Database. They have used different

geometrical properties viz. dihedral angles, max and

min curvature. Also the database used for learning

did not have anatomical meshes and the resultant me-

tric is a global visual distortion metric. The details of

the signatures used in current research are explained

further in Section 4.

Further, the work of (Biederman, 1987) on recog-

nition by parts (RBC) suggests that humans tend to

recognize an object based on its parts, and this has

been exploited in various part based shape retrieval

algorithms (Theologou et al., 2014). The work by (Ji-

ang et al., 2013) also follows a part based matching

approach, using a signature based on eigenfunctions

of Leplace-Beltrami operator for CAD models. In

our present work, we also take this notion into con-

sideration and develop a metric that focuses on part

based signatures and relationships between parts as

well. The steps involved in metric computation are

explained next.

Let us consider two polygonal surface meshes P

and P

, where P

is the template mesh known to be

anatomically correct and P

is the candidate mesh to

be validated. Figure 1 shows the main processing

blocks of pipeline and metric computation, the same

are described with some details as follows:

• Mesh Segmentation of the given two meshes,P

and P

• Attributed Relational Graph(ARG) creation from

the segmented meshes.

• Computation of cost matrix between the two

ARGs

• Computation of the segment to segment matching,

i.e., permutation matrix, using Hungarian node as-

signment algorithm.

• An Optional segment merging step, in case there

are unmatched segments.

• Finally, computation of global, part-based and re-

sultant mesh validation metric.

Figure 1: Pipeline Block Diagram.

The following sections provide the detail of the

above mentioned pipeline blocks.

3 MESH SEGMENTATION

In order to compute a part-based metric, the ﬁrst step

is to compute these parts. We have chosen standard

segmentation to try to decompose a mesh into parts

as most anatomically salient parts indeed are geome-

trically also distinct from its neighbors. Although

segmentation is not our end goal and it is often suf-

ﬁcient for the two meshes P

and P

to undergo si-

milar segmentation, we have found the Shape Dia-

meter Function (SDF) based segmentation algorithm

(Shapira et al., 2008) does well to align segments with

anatomical parts. The Princeton benchmark on 3D

mesh segmentation (Chen et al., 2009) ranks SDF ba-

sed segmentation algorithm as third in its evaluation

metric. Further, the SDF based segmentation takes

about 10x less time than the top two. The algorithm

is largely automatic: it does not need user intervention

to decide segment count. In fact, the default parame-

ters values mentioned by the authors generally work.

SDF is a volume based shape function and is in-

variant to rigid transformation and largely oblivious

to pose changes. It is deﬁned as a scalar ﬁeld on the

mesh surface and it gives a measure of the diameter

of the object’s volume in the neighborhood of each

point on the surface. It involves computation of a lo-

cal diameter at each vertex of a mesh. The histogram

GRAPP 2017 - International Conference on Computer Graphics Theory and Applications

162

of the SDF values is processed using Gaussian Mix-

ture Models and Expectation Maximization algorithm

to create consistent mesh partitions.

We use the implementation provided by the

CGAL library package (Yaz and Loriot, 2016) with

default values of 25 rays, cone angle of 120 degrees,

5 clusters and 0.26 as smoothing factor. We further

build upon the facet-id map provided as output of the

algorithm, to create separate polygon segment mes-

hes. These segment meshes are later used for creating

shape signatures in order to get the part-based compa-

rison metric.

We use the following notation. S

and S

are the

segmented polygonal meshes such that

[

i=1

, S

is the i

segment of mesh P

[

j=1

b j

, S

b j

is the j

segment of mesh P

Where, n and m are number of segments of P

and

, respectively.

4 ATTRIBUTED RELATIONAL

GRAPH CREATION

The segmented meshes S

and S

are used to cre-

ate two adjacency graphs. We create a node corre-

sponding to a segment and an edge for every pair of

adjacent segments of the segmented mesh. We as-

sociate properties a.k.a. attributes to the nodes and

the edges based on these segments, such a graph is

known as Attributed Relational Graph (ARG). The

Boost Graph Library (Siek et al., 2001) has been ex-

tensively used for the implementation of ARG. Let

= (V

, E

) be the ARG for the segmented mesh

and G

= (V

, E

) be the ARG for the segmented

mesh S

The node attributes are the signatures based on ge-

ometric shape descriptors of a mesh segment and the

edge attributes are the relational properties between

two adjacent mesh segments. The following subsecti-

ons provide details of the various geometric proper-

ties and thus signatures used to create these attributes.

We will use the terms node and segment interchange-

ably henceforth.

4.1 Node Attributes

Geometrical properties of segments that are assigned

to the nodes are termed as node attributes. The fol-

lowing subsection provide details of the various node

attributes used in our implementation.

4.1.1 SDF Signature

We create SDF signature of a segment, which is a log

normalized histogram of SDF values as described in

(Shapira et al., 2008).

nsd f =

log



sd f ( f )−min(sd f )

max(sd f )−min(sd f )

∗ η + 1



log(η + 1)

Where sd f : F → R is the SDF value for each facet

f and η is a normalizing parameter, which is set to 4.

The SDF signature is pose-oblivious and captures the

volumetric variation of a 3D shape. We use 64 bins to

create the histogram which is same as the original bin

count used by the authors in (Gal et al., 2007). Let us

denote, V

sd f

and V

sd f

b j

, as the SDF signature for the

and the j

node of G

and G

, respectively.

4.1.2 Shape Index Signature

We create a Shape Index (SI) (Koenderink and van

Doorn, 1992) signature of a segment. which is a his-

togram of the SI values. If K

and K

are the principal

curvatures for a patch around a vertex of mesh, the SI

value for that patch is deﬁned as:

si =

arctan

+ K

− K

, where K

6 K

The principal curvatures K

and K

are determi-

ned using the second order differential properties of

a local representation of the manifold ﬁtted using a

jet, a truncated Taylor expansion (Cazals and Pouget,

2005). We use the CGAL library package by the same

authors (Pouget and Cazals, 2016) for estimating lo-

cal differential properties of a patch around a mesh

vertex via jet ﬁtting.

The formula for determining SI values is changed

slightly from the original expression so that it is po-

sitive always. For all patches except the planar patch,

for which it is indeterminate, si ∈ [0, 1]. si is a scalar

that provides information about the shape of a patch,

e.g., whether it is a cup, cap, ridge, rut or saddle. Li-

kewise sdf signature, we use 64 bin histogram here as

well to create SI signature for a mesh segment. Let,

and V

b j

, be the SI signature for the i

and the j

node of G

and G

, respectively.

4.1.3 Area Ratio

We compute the ratio of the area of a segment to the

area of the complete mesh. If Ar

is the surface area

of a mesh segment and Ar

the surface area of the

A Pipeline and Metric for Validation of Personalized Human Body Models

163

complete mesh, Area ratio accounts for the relative

size of each part.

α =

is deﬁned as the area ratio of that segment. The same

is associated with the corresponding node of ARG.

Let, V

and V

b j

, be the area ratio for the i

and the

node of G

and G

, respectively.

4.1.4 Eigen Value Based Shape Descriptor

If λ

, λ

and λ

are the three eigenvalues of a segment

s.t. λ

> λ

then the eigenvalues based shape

descriptor can be deﬁned as in (Sidi et al., 2011):

− λ

∑

i=1

2(λ

− λ

)

∑

i=1

3λ

∑

i=1

We compute the eigenvalues based shape descrip-

tor as a vector having components e = < e

, e

> of

each segment and assign it as an attribute to the cor-

responding node of the ARG. We use CGAL library

Principal Component Analysis package (Alliez et al.,

2016) to compute the eigenvalues of a mesh segment.

Let, V

and V

b j

, be the shape descriptor for i

and j

node of G

and G

, respectively.

4.1.5 Node Degree

The node degree is the number of edges connected to

a node. Although this attribute is assigned to a node,

in strict sense it is a relational attribute. Let, V

deg

and

deg

b j

be the node degree for the i

and the j

node of

and G

, respectively.

4.2 Edge Attribute

We compute a tuple that represents the angles bet-

ween the two largest eigenvectors (the ones with the

largest eigenvalues), respectively, of a segment with

its adjacent segment. If u

is the largest and u

the

next largest eigenvector of a segment S

and v

and

are similarly the two largest eigenvectors of an ad-

jacent segment S

, the tuple of eigenvector angles (in

radians) is deﬁned as.

= [u

− v

, u

− v

]

This tuple is a relational feature that describes the

relative orientation of an adjacent segment to the gi-

ven segment. It is computed for all the edges incident

on an ARG node. Let, V

and V

b j

, be the eigenvector

angle difference for the i

and the j

node of G

and

, respectively.

5 SEGMENT MATCHING

In this section we describe how to ﬁnd for each seg-

ment of the candidate mesh, its corresponding seg-

ment in the template mesh. Finding corresponding

segments between segmented meshes S

and S

is a

two step process. First, a cost matrix C

is compu-

ted, where n and m are the segment counts in S

and

, respectively. Each element of the cost matrix is

some form of distance between the two nodes in their

signature space. Second, this cost matrix is used to

obtain a node assignment using Harold Kuhn’s well-

known Hungarian Method for solving Optimal Assig-

nment Problems. The permutation matrix thus com-

puted is a boolean matrix with 1

s for the matched

nodes and 0

s for others. The following subsections

provide further details.

5.1 Cost Matrix Computation

Referring to the notations proposed in previous

section, the cost matrix for segmented mesh S

and

can be computed as follows:

loop: For all vertices a

of G

loop: For all vertices b

of G

sd f

i j

= EM D(V

sd f

b j

)

i j

= EM D(V

b j

)

i j

= L

b j

)

i j

= L

b j

)

deg

i j

= L

deg

b j

)

i j

= L

− V

b j

)

i j

= L

sd f

i j

, c

i j

, c

i j

, c

i j

, c

deg

i j

, c

i j

)

endloop

Where, EMD is the earth movers distance (Rub-

ner et al., 1998). We use EMD to compute distance

between the signatures and absolute difference in case

of scalars. Each of the distances are normalized over

its range during the computation of the cost matrix

Table 1 gives the description of the notations used in

the pseudocode above.

5.2 Permutation Matrix Computation

We use libhungarian library (Gerkey, 2004) to solve

the node assignment problem. The cost matrix C

described in section 5.1 is used for this computation.

We multiply Each element of the cost matrix with in-

teger 10, to just scale it, since the input to a libhun-

garian is supposed to be only integral values. The

Hungarian Algorithm produces a sparse permutation

GRAPP 2017 - International Conference on Computer Graphics Theory and Applications

164

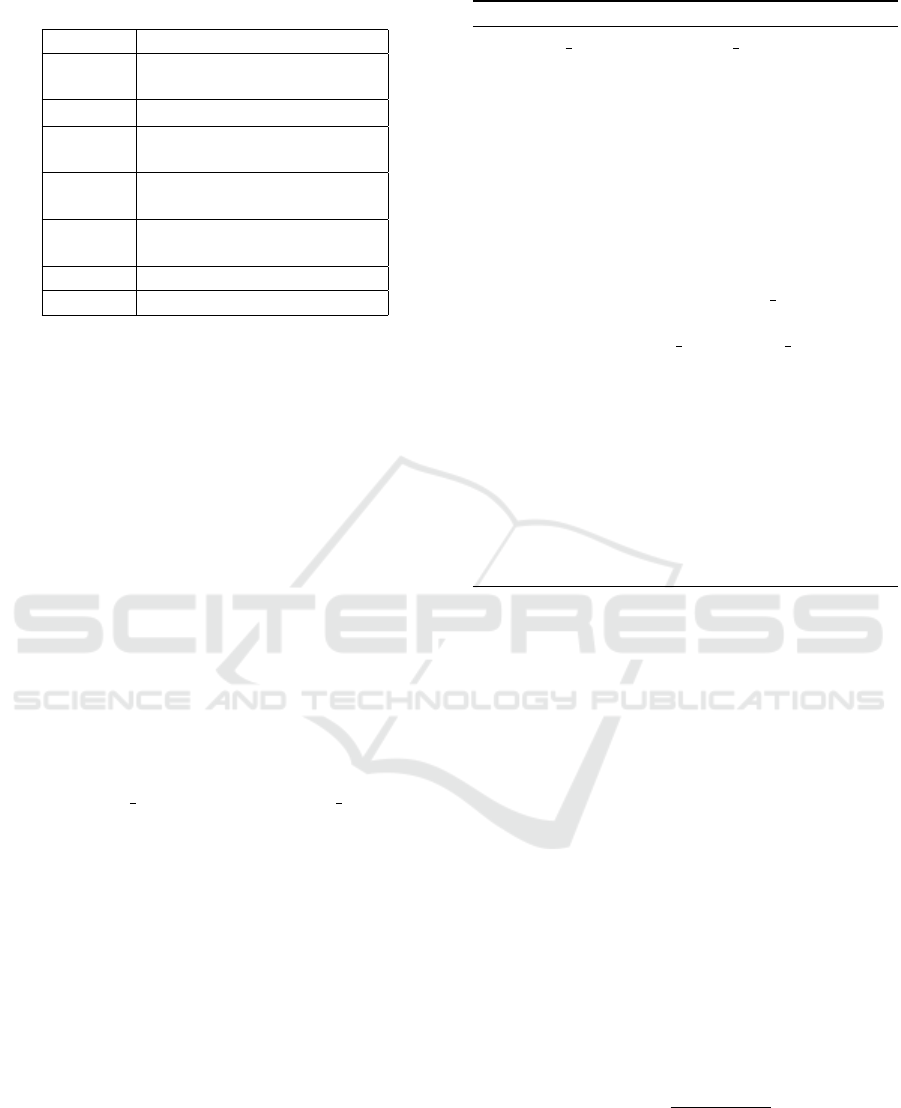
Table 1: Notations used for the various cost/distance.

Notation

Description

sd f

i j

EMD between SDF

signatures

i j

EMD between SI signatures

i j

Absolute difference

between area ratio

i j

Euclidean distance between

shape descriptors

deg

i j

Absolute difference

between degrees

L1 norm

L2 norm

n × m sized matrix P

, where P

i j

= 1 if i and j are

matching nodes.

6 SEGMENT MERGING

After completing the node assignment explained in

Section 5, some nodes of graph G

or G

may re-

main un-assigned. This happens when the number of

nodes in the two graphs are unequal. This could be

due to imperfect segmentation or some defect in the

candidate mesh. We consider the initial matching as

tentative in this case and iterate in an attempt to im-

prove the segmentation. In particular, we study the

impact on matching if we merge two adjacent seg-

ments. An improvement in matching is strongly sug-

gestive of imperfect segmentation and we ﬁnalize the

merger. The algorithm is listed in Algorithm 1. It may

happen that dist merge is greater than dist original in

all tentative merge cases, which results in ARGs ha-

ving unequal nodes even after the merge step. In that

case we use distance of the unmatched segment sig-

natures from zero valued signatures and consider that

as an added penalty in the metric computation.

is the resultant graph after the merge step.

We reapply the segment matching algorithm listed in

Section 5 between G

and the other graph. This again

results in a cost matrix and a permutation matrix. We

now use these two matrices to compute the part-based

metric as explained in following section.

7 METRIC COMPUTATION

The result of the segment matching step (see Sections

5 and 6) is the cost matrix C

and the permutation

matrix P

. The cost matrix C

is a collation of the

distances of the segments in signature space. These

Algorithm 1: Segment Merging algorithm.

1: if num vertices(G

) > num vertices(G

) then

2: MERGESEGMENT(G

);

3: else

4: MERGESEGMENT(G

);

5: end if

6: function MERGESEGMENT ( G

)

7: for all Unmatched vertices V

in G

8: for all Neighbours V

nbr

of V

9: if V

nbr

is matched then

10: Merge V

nbr

and V

to get V

merge

11: Compute unary attributes of V

merge

12: Find distance dist merge between

merge

and corresponding vertex of V

nbr

in G

13: if dist merge < dist original then

14: Keep the merge

15: else

16: Discard the merge

17: end if

18: end if

19: end for

20: end for

21: end function

 Return the resultant graph after merging

return G

distances are EMD, Euclidean, Manhattan or Abso-

lute difference. An element C

i j

of matrix C

is the

distance between segments i and j, respectively, of the

two meshes. As explained in Section 4, these node at-

tributes account for the segment shape and the edge

attributes account for the relation between segments.

The resultant metric is thus able to capture both the

part based shape and inter part relational aspect of the

meshes under comparison. Section 7.1 describes the

computation of complete part based metric. Section

7.2 describes the computation of the global metric.

7.1 Part-Based Metric

We use the computed cost matrix C

(refer Section

5.1) and permutation matrix P

(refer Section 5.2)

to compute the part based metric. We iterate through

and check for matched nodes, and the correspon-

ding cost is picked from C

. The L

norm of all these

costs is the computed part based metric. That is:

∑

i=1

∑

j=1

i j

A Pipeline and Metric for Validation of Personalized Human Body Models

165

7.2 Global Metric

In order to compute the global metric, we ﬁrst com-

pute the signature corresponding to the complete

mesh. We compute the SDF signature for the mes-

hes P

and P

and compute EMD between the two

signatures. If H

and H

are the histograms of SDF

values of the meshes P

and P

sd f

= EM D(H

, H

)

We also compute the eigenvalues based shape des-

criptor of the meshes as described in Section 4.1.4 for

mesh segments. If e

and e

are shape descriptors of

meshes P

and P

then,

− e

Lastly, we compute the SI values based global

shape signature of the meshes P

and P

(see Section

4.1.2). Thus if SI

and SI

are the histograms of SI

values of the meshes P

and P

= EM D(SI

, SI

)

We tested with both Euclidean distance and EMD

for histograms, and observed that EMD gives more re-

liable results in practice than does the Euclidean dis-

tance. This is due to the fact that EMD imparts a more

global sense to the distance between the two histo-

grams, whereas the Euclidean distance can be unduly

inﬂuenced by individual bins. For eigenvalues based

shape descriptor, which is not a histogram, we use the

Euclidean distance.

The resulting global metric is deﬁned as the L

norm of m

sd f

, m

and m

sd f

+ m

7.3 Overall Metric

The ﬁnal metric is reported as the pair of the part ba-

sed metric and the global metric, i.e.,

= (M

, M

)

Since both M

and M

are based on metrics like

EMD, Euclidean or Manhattan distances, the resul-

tant metric does follow non-negativity and symmetry

properties, but may not follow triangular inequality.

8 EXPERIMENTAL RESULTS

In section 8.1 we show an example validation for fe-

mur mesh having 16516 vertices and 33028 facets,

where the template femur mesh has been deformed

at the Lesser Trochanter region as shown in Figure

2. We shall discuss two examples: Example 1, where

the number of segments in the Template and the Test

meshes are the same, and Example 2, where the num-

ber of segments of the Template and Test meshes are

different. In the latter case, the mesh that has more

segments will undergo automatic merging based on

the algorithm as described in Section 6 The experi-

ment was carried out on a system having Processor:

Intel i7 CPU@2.40GHz and RAM: 8 GiB.

8.1 Example 1

Figure 5 shows the segmentation results of the tem-

plate mesh and the deformed test mesh at the Lesser

Trochanter region. It can be observed that for the gi-

ven parameters of the segmentation algorithm, it is

able to segment the femur into anatomically relevant

regions like a) Head b) Shaft c) Condylar region and

d) Greater trochanter. The results of the segmenta-

tion can change with the supplied parameters. In the

current example the test mesh has the same number

of segments as does the template mesh. The ARGs

are constructed from the segmented meshes, Figure 3

and Figure 4 show the obtained cost and permutation

matrices, respectively. Matching colors in Figure 5

represent the matched segments obtained in this step.

The cost and permutation matrices are used to

compute the part-based metric and the global metric.

The resultant metric is the pair of part-based and glo-

bal metric as explained in section 7.

= 13.5712 (1)

= 0.1307 (2)

= (13.5712, 0.1307) (3)

where, M

, M

and M

are the part-based, global

and resultant metrics, respectively. where, M

, M

and M

are the part-based, global and resultant me-

trics, respectively. The complete process took approx-

imately 43 seconds.

8.2 Example 2

Figure 10 shows the segmentation results of the the

template mesh and test mesh deformed at the same

location, but by a larger amount, as that in the mesh

in Example 1. The segmentation algorithm, for the

same parameters produces seven segments in this

case. Thus the test mesh undergoes a merger step. Fi-

gure 6 and Figure 7 show the obtained cost matrix and

permutation matrix respectively. The matched seg-

ments in Figure 10 are shown in the same color. No-

tice that there are two unmatched segments, colored

GRAPP 2017 - International Conference on Computer Graphics Theory and Applications

166

Figure 2: Femur Deformed at Lesser Trochanter: Template Mesh - Test Mesh 1 - Test Mesh 2.







10 39 23 25 41

40 2 29 27 25

25 32 6 20 34

33 29 18 1 26

44 26 27 22 5







Figure 3: 5 × 5 cost matrix femur example 1.







1 0 0 0 0

0 1 0 0 0

0 0 1 0 0

0 0 0 1 0

0 0 0 0 1







Figure 4: 5 × 5 permutation matrix computed femur exam-

ple 1.

pink and white. The complete process took approxi-

mately 42 seconds.

Figure 8 and Figure 9 show the obtained cost ma-

trix and permutation matrix after merging. Figure 11

shows the matched segments ﬁnally obtained in this

step. Notice that the two unmatched segments (from

Figure 10) are merged with their neighbouring seg-

ments in this step.

The cost and permutation matrices are used to

compute the part-based metric and the global metric.

These are then joined to produce the resultant metric

as explained in section 7.

= 19.2116 (4)

= 0.1347 (5)

= (19.2116, 0.1347) (6)

Clearly, in this case output global as well as part

based metric has greater value as compared to the ex-

ample 1 results, thus indicating a greater amount of

deformation. The merge step with computation of

metric took approximately 27 seconds.

Figure 5: Corresponding segments of Template and Test

Mesh 1.







24 7 39 31 21 29 33

33 33 5 34 29 23 21

24 21 30 27 7 22 23

29 24 24 33 19 5 17

37 36 21 32 32 19 12

0 0 0 0 0 0 0







Figure 6: 7 × 7 cost matrix Femur case 2.

8.3 Survey Results

We conducted a survey to check the efﬁcacy of the

pipeline and the metric. The survey comprised ima-

ges of ﬁve different sets of meshes. Each set had

four meshes: one original template mesh and three

deformed versions of it. Side-on snapshots of each

mesh from four mutually perpendicular viewing an-







0 1 0 0 0 0 0

0 0 1 0 0 0 0

0 0 0 0 1 0 0

0 0 0 0 0 1 0

0 0 0 0 0 0 1

1 0 0 0 0 0 0

0 0 0 1 0 0 0







Figure 7: 7 × 7 permutation matrix Femur example 2.

A Pipeline and Metric for Validation of Personalized Human Body Models

167







7 45 20 27 36

37 11 31 24 20

24 40 8 21 27

28 32 20 5 21

40 28 32 20 8







Figure 8: 5 × 5 cost matrix femur example 2 after merge.







1 0 0 0 0

0 1 0 0 0

0 0 1 0 0

0 0 0 1 0

0 0 0 0 1







Figure 9: 5 × 5 permutation matrix femur example 2 after

merge.

gles were shown for evaluation. The three deformed

meshes in each set all had deformations at the same

location but with varying magnitudes. One of the sets

include deformed mesh output from a GEBOD based

(CEESAR et al., 2014; Cheng et al., 1996) FE-Mesh

personalization algorithm. A group of ten experts,

which comprised bio-mechanic researchers and medi-

cal practitioners, were asked to compare each defor-

med mesh (on the basis of the four snapshots) to the

template mesh and assign a rank from 1 to 3 indica-

ting the degree of deformation in their judgement. Fi-

gure 12 shows the results of the ranking marked by the

surveyors. There was signiﬁcant agreement among

them.

We also computed the part-based and global me-

trics of the same set of deformed test meshes (with re-

spect to their respective templates). In Figure 13 and

Figure 14, we show the computed global and part-

based metrics, respectively.

We evaluate the metric against the survey. From

the survey, the modal rank of each deformed mesh

was taken as its anatomically standard rank. The ran-

kings from the metric were obtained directly: a hig-

her value of the metric implied a lower rank. Fi-

gure 15 shows the results of the comparison. Furt-

her, the surveyors were also asked to judge the ana-

tomical correctness of each test mesh by comparing

it to the corresponding template meshes. Speciﬁ-

cally, the question was ”Are the two bones anatomi-

cally the same?”, to which they had to answer as a)

Strongly agree b) Agree c) Disagree or d) Strongly

disagree. We computed a cumulative-score for a par-

ticular answer as

∑

i=1

weight

∗ mode

, where weights

were assigned from 1 − 4 with 1 for ‘strongly agree’

and so on. It was observed that the correlation bet-

ween cumulative-score and part-based metric is signi-

ﬁcant with correlation coefﬁcient = 0.724. The corre-

lation coefﬁcient between rankings and global-metric

is 0.900 and between rankings and part-based metric

Figure 10: Corresponding segments of Template and Test

Mesh 2 with two unmatched segments in color pink and

white.

Figure 11: Corresponding segments of Template and Test

Mesh 2 – Merged.

is 0.500.

Out of the ﬁve sets, the part-based metric dis-

agrees with the standard ranking of the ﬁrst mesh and

the ﬁfth mesh. For the ﬁrst mesh, which is quite sym-

metric with similar shapes for opposite ends of mes-

hes under comparison, matching failed, in part due

to the segmentation. The sphere-like lateral condy-

lar region was segmented for the template mesh but it

matched the femoral head segment of the test mesh.

These had similar geometric and relational features,

and ended up in an inverse segment match. One way

to improve this is to use different parameters for the

segmentation algorithm and break symmetry. Since

we wanted the same parameter for all meshes, we did

not explore this further.

The ﬁfth set had a torsion deformation at the fe-

mur condylar region. It was observed that the se-

cond mesh in this set had an equal number of seg-

ments to that of the template mesh. But the ﬁrst mesh

had an unequal number of segments. Even though

the segment merging algorithm accounts for this dis-

crepancy, this case results in signiﬁcant variations in

GRAPP 2017 - International Conference on Computer Graphics Theory and Applications

168

Figure 12: Ranking of Bone Deformation based on a Survey.

Figure 13: Deformed Mesh Global Metric.

Figure 14: Deformed Mesh Part-based Metric.

Figure 15: Comparison of Rankings based on a Survey, Global Metric and Part-Based Metric.

A Pipeline and Metric for Validation of Personalized Human Body Models

169

the segment boundaries, leading to deviations in the

computed metric. Overall, three cases out of ﬁve had

rankings identical to the survey’s for the part-based

metric and the other two cases had partly similar ran-

king, but in these case, the global metric had ranking

almost identical to the survey’s.

In particular, one might conclude that if both part-

based and global metrics indicate that a test-mesh has

a high rank, it is indeed of good anatomical quality.

9 CONCLUSION AND FUTURE

WORK

In this paper, we present a pipeline for the valida-

tion of personalized/deformed anatomical meshes by

comparing these with known correct meshes. The pi-

peline considers part-based, relational and global ge-

ometrical features and gives a resultant validation me-

tric. The complete pipeline requires little user inter-

vention and computes the validation metric in an au-

tomatic way. The efﬁcacy of the validation metric

depends on three important factors, a) segmentation

algorithm b) the choice of geometric features or sig-

natures and c) the distance metric. We observed that

SDF based segmentation algorithm gives satisfactory

mesh partitions in case of anatomical organs. Howe-

ver, the segmentation output varies with the change in

parameters provided to the algorithm. Further inves-

tigation is needed to determine if a better segmenta-

tion into actual anatomical parts can improve the ma-

tch metric further. In further work, one may provide

some control to segment the template as a one time

effort. The candidate mesh can then be automatically

co-segmented with the template. In future, one can

test for additional signatures, anatomical meshes of

upper body region and improve on limitations of the

metric.

ACKNOWLEDGEMENTS

We thank our colleagues and institutions involved in

PIPER project (CEESAR et al., 2014) for providing

us access to the Anatomical Surface Mesh Data Set

and GHBMC model. Also, all the volunteers who

helped in ﬁlling the on-line survey for subjective tes-

ting. Further, the comments from anonymous revie-

wers have been very helpful.

REFERENCES

Alliez, P., Pion, S., and Gupta, A. (2016). Principal compo-

nent analysis. In CGAL User and Reference Manual.

CGAL Editorial Board, 4.9 edition.

Aspert, N., Santa-Cruz, D., and Ebrahimi, T. (2002). Mesh:

measuring errors between surfaces using the hausdorff

distance. In Multimedia and Expo, 2002. ICME ’02.

Proceedings. 2002 IEEE International Conference on,

volume 1, pages 705–708 vol.1.

Biederman, I. (1987). Recognition-by-components: A the-

ory of human image understanding. Psychological Re-

view, 94:115–147.

Cazals, F. and Pouget, M. (2005). Estimating differential

quantities using polynomial ﬁtting of osculating jets.

Computer Aided Geometric Design, 22(2):121 – 146.

CEESAR, INRIA, U. et al. (2014). Position and Persona-

lize Advanced Human Body Models for Injury Pre-

diction. http://www.piper-project.eu/. [Online; acces-

sed 18-October-2016].

Chen, X., Golovinskiy, A., and Funkhouser, T. (2009). A

benchmark for 3d mesh segmentation. In ACM SIG-

GRAPH 2009 Papers, SIGGRAPH ’09, pages 73:1–

73:12, New York, NY, USA. ACM.

Cheng, H., Obergefell, L., and Rizer, A. (1996). The de-

velopment of the gebod program. In Biomedical En-

gineering Conference, 1996., Proceedings of the 1996

Fifteenth Southern, pages 251–254.

Cignoni, P., Rocchini, C., and Scopigno, R. (1998). Me-

tro: Measuring error on simpliﬁed surfaces. Computer

Graphics Forum, 17(2):167–174.

Gal, R., Shamir, A., and Cohen-Or, D. (2007). Pose-

oblivious shape signature. IEEE Transactions on Vi-

sualization and Computer Graphics, 13(2):261–271.

Gayzik, F. S., Moreno, D. P., Vavalle, N. A., Rhyne, A. C.,

and Stitzel, J. D. (2011). Development of the global

human body models consortium mid-sized male full

body model. Injury Biomechanics Research, pages

39–12.

Gerkey, B. P. (2004). C Implementation of the Hun-

garian Method. http://robotics.stanford.edu/ ger-

key/tools/hungarian.html. [Online; accessed 14-July-

2016].

Gray, H. (2001). Anatomy of the human body. Phi-

ladelphia: Lea & Febiger, 1918; Bartleby.com,

2000. http://www.bartleby.com/107/17.html. [Online;

accessed 18-October-2016].

Hwang, E., Hallman, J., Klein, K., Rupp, J., Reed, M., and

Hu, J. (2016). Rapid development of diverse human

body models for crash simulations through mesh mor-

phing. Technical report, SAE Technical Paper.

Iwamoto, M., Kisanuki, Y., Watanabe, I., Furusu, K., Miki,

K., and Hasegawa, J. (2002). Development of a ﬁ-

nite element model of the total human model for sa-

fety (thums) and application to injury reconstruction.

In Proceedings of the International Research Council

on the Biomechanics of Injury conference, volume 30,

pages 12–p. International Research Council on Bio-

mechanics of Injury.

Iyer, N., Jayanti, S., Lou, K., Kalyanaraman, Y., and Ra-

mani, K. (2005). Three-dimensional shape searching:

GRAPP 2017 - International Conference on Computer Graphics Theory and Applications

170

state-of-the-art review and future trends. Computer-

Aided Design, 37(5):509 – 530. Geometric Modeling

and Processing 2004.

Jiang, L., Zhang, X., and Zhang, G. (2013). Partial

shape matching of 3d models based on the laplace-

beltrami operator eigenfunction. Journal of Multime-

dia, 8(6):655–661.

Koenderink, J. J. and van Doorn, A. J. (1992). Surface shape

and curvature scales. Image and Vision Computing,

10(8):557 – 564.

Lalonde, N. M., Petit, Y., Aubin, C.-E., Wagnac, E.,

and Arnoux, P.-J. (2013). Method to geometrically

personalize a detailed ﬁnite-element model of the

spine. IEEE Transactions on Biomedical Engineer-

ing, 60(7):2014–2021.

Lavou, G. (2011). A multiscale metric for 3d mesh vi-

sual quality assessment. Computer Graphics Forum,

30(5):1427–1437.

Lavou, G., Cheng, I., and Basu, A. (2013). Perceptual qua-

lity metrics for 3d meshes: Towards an optimal multi-

attribute computational model. In 2013 IEEE Interna-

tional Conference on Systems, Man, and Cybernetics,

pages 3271–3276.

Pouget, M. and Cazals, F. (2016). Estimation of local diffe-

rential properties of point-sampled surfaces. In CGAL

User and Reference Manual. CGAL Editorial Board,

4.9 edition.

Poulard, D., Bermond, F., Dumas, R., Bruyere, K., and

Compigne, S. (2012). Geometrical personalisation

of human fe model using palpable markers on volun-

teers. Computer methods in biomechanics and biome-

dical engineering, 15(sup1):298–300.

Robin, S. (2001). Humos: human model for safety–a joint

effort towards the development of reﬁned human-like

car occupant models. In 17th international technical

conference on the enhanced safety vehicle, page 297.

Rubner, Y., Tomasi, C., and Guibas, L. J. (1998). A metric

for distributions with applications to image databases.

In Computer Vision, 1998. Sixth International Confe-

rence on, pages 59–66.

Shapira, L., Shamir, A., and Cohen-Or, D. (2008). Con-

sistent mesh partitioning and skeletonisation using

the shape diameter function. The Visual Computer,

24(4):249–259.

Sidi, O., van Kaick, O., Kleiman, Y., Zhang, H., and Cohen-

Or, D. (2011). Unsupervised co-segmentation of a

set of shapes via descriptor-space spectral clustering.

ACM Trans. on Graphics (Proc. SIGGRAPH Asia),

30(6):126:1–126:10.

Siek, J. G., Lee, L.-Q., and Lumsdaine, A. (2001). Boost

Graph Library: User Guide and Reference Manual,

The. Pearson Education.

Tangelder, J. W. H. and Veltkamp, R. C. (2007). A survey of

content based 3d shape retrieval methods. Multimedia

Tools and Applications, 39(3):441.

Theologou, P., Pratikakis, I., and Theoharis, T. (2014). A

review on 3d object retrieval methodologies using a

part-based representation. Computer-Aided Design

and Applications, 11(6):670–684.

Vezin, P. and Verriest, J. P. (2005). Development of a set of

numerical human models for safety. In 19th Internati-

onal Technical Conference on the Enhanced Safety of

Vehicles, Washington DC, pages 6–9.

Yaz, I. O. and Loriot, S. (2016). Triangulated surface mesh

segmentation. In CGAL User and Reference Manual.

CGAL Editorial Board, 4.9 edition.

Zhou, L. and Pang, A. (2001). Metrics and visualization

tools for surface mesh comparison. In Visual Data

Exploration and Analysis VIII, 99 (May 3, 2001), vo-

lume 4302, pages 99–110.

A Pipeline and Metric for Validation of Personalized Human Body Models

171