Privacy-Preserving Veriﬁability

A Case for an Electronic Exam Protocol

Rosario Giustolisi

, Vincenzo Iovino

and Gabriele Lenzini

IT University of Copenhagen, Copenhagen, Denmark

University of Luxembourg, Interdisciplinary Centre for Security, Reliability and Trust (SnT), Luxembourg, Luxembourg

Keywords:

Privacy-Preserving Veriﬁability, Electronic Exam Protocols, Analysis of Security and Privacy.

Abstract:

We introduce the notion of privacy-preserving veriﬁability for security protocols. It holds when a protocol

admits a veriﬁability test that does not reveal, to the veriﬁer that runs it, more pieces of information about

the protocol’s execution than those required to run the test. Our deﬁnition of privacy-preserving veriﬁability

is general and applies to cryptographic protocols as well as to human security protocols. In this paper we

exemplify it in the domain of e-exams. We prove that the notion is meaningful by studying an existing exam

protocol that is veriﬁable but whose veriﬁability tests are not privacy-preserving. We prove that the notion

is applicable: we review the protocol using functional encryption so that it admits a veriﬁability test that

preserves privacy according to our deﬁnition. We analyse, in ProVerif, that the veriﬁability holds despite

malicious parties and that the new protocol maintains all the security properties of the original protocol, so

proving that our privacy-preserving veriﬁability can be achieved starting from existing security.

1 INTRODUCTION

“Being veriﬁable” is an appreciated quality for a pro-

tocol. It says that during its execution the protocol

safely stores pieces of information that can be used

later as evidence to determine that certain properties

have been preserved by the protocol’s run. A voting

system that offers its voters to “verify that their votes

have been cast as intended”, for instance, generates

data that allow a voter to test that his/her ballot has

been registered and that it contains, with no mistake,

the expression of his/her vote (Adida and Neff, 2006).

This paper is about veriﬁability, but it also dis-

cusses a new requirement for it. The requirement

states that a curious veriﬁer, in addition to the veri-

ﬁability test’s result, should learn no more about the

system’s execution than the pieces of information that

he needs to know to run the test.

The need-to-know principle is not new (e.g., see

(Department of Defence, 1987)). Not new is also

the particular interpretation of the principle that link

to techniques like zero knowledge proofs (De Santis

et al., 1988) where a veriﬁer is convinced of a given

statement’s truth without learning no more than that.

However, in this paper we originally present and dis-

cuss the principle as a requirement for veriﬁability.

Our notion of privacy-preserving veriﬁability

needs to be formally deﬁned, and we do so in §3. We

contextualize the deﬁnition to the particular domain

of electronic exams, where veriﬁability and privacy

are sensitive properties (the ﬁrst should work despite

untrusted authorities, the second is not everlasting but

conditional). Besides, in §4, we prove that our notion

of privacy-preserving veriﬁability is meaningful. We

show for a particular protocol called Remark! (Gius-

tolisi et al., 2014) that several of its current universal

veriﬁability are not privacy-preserving according to

our deﬁnition.

In §5, we give evidence that achieving privacy-

preserving veriﬁability is possible. In particular,

again taking Remark! as use case, we demonstrate

that by modifying the protocol’s design it becomes

possible to have, for one of the protocol’s veriﬁability

property, a test that is privacy-preserving. The modi-

ﬁcation we operate on the protocol is guided straight-

forwardly by the predicate that the veriﬁability test

is expected to check. The other veriﬁability prop-

erties of Remark! rely on predicates which are, in

their structure, similar to that we prove our case (i.e.,

they are all predicate about integrity and authentica-

tion); thus, we can easily, we claim, achieve privacy-

preserving veriﬁability for them too.

In §6, we analyse formally the new protocol. We

verify with Proverif (Blanchet, 2014) that the new de-

Giustolisi, R., Iovino, V. and Lenzini, G.

Privacy-Preserving Veriﬁability - A Case for an Electronic Exam Protocol.

DOI: 10.5220/0006429101390150

In Proceedings of the 14th International Joint Conference on e-Business and Telecommunications (ICETE 2017) - Volume 4: SECRYPT, pages 139-150

ISBN: 978-989-758-259-2

139

sign does not compromise any of the protocol’s en-

joyed security including all its other veriﬁability prop-

erties. This analysis is a signiﬁcant step since we

prove that privacy-preserving veriﬁability for a spe-

ciﬁc veriﬁability test can be achieved while preserv-

ing all the existing security properties of the protocol.

Before moving to the technical part of the paper it

is important to anticipate that private-preserving veri-

ﬁability can be potentially achieved using many of the

instruments that computer security research offers to-

day for minimize the disclosure of information. Non-

interactive Zero-knowledge proofs (De Santis et al.,

1988) and functional encryption schemes (Boneh

et al., 2011) are the two instruments that stand out for

this purpose. Here, we use the second instrument. We

do not have a formal proof but we discuss in §7 that

only Functional Encryption has features that make it

suitable to our purpose.

2 RELATED WORK

Privacy and veriﬁability, apparently two contrast-

ing properties, have been discussed in the literature

mainly to show that they can be both satisﬁed in the

same system.

Most of the works discussing privacy and veriﬁa-

bility in this sense are about voting systems. The liter-

ature on the subject is vast but, to make our point, we

comment the work of Cuvelier et al. (Cuvelier et al.,

2013). The authors propose a new primitive, called

Commitment Consistent Encryption. With it, they re-

design a voting scheme for an election that is veri-

ﬁable while ensuring everlasting ballot privacy. The

problem they solve for voting is clearly related to that

we raise here: avoid that from a public audit trail a

veriﬁer could learn more than it is allowed to learn.

To a certain extent, Cuvelier et al. propose a crypto-

graphic instance of the problem that we formalize in

this paper. But our notion of privacy-preserving ver-

iﬁability is more general and not necessarily crypto-

graphic: it applies to cryptographic protocols as well

as to non-crypto protocols. It is, in other words, a

functional requirement for the veriﬁability tests that a

system offers to auditors. In this sense, our work re-

lates more to the plentiful research that discusses re-

quirements and deﬁnitions of privacy. Again, the lit-

erature on this subject is huge, but the work that most

closely represents the notion of privacy-preservation

that we advance here is that of M¨odersheim et al.

(M¨odersheim et al., 2013). The work presents and

discusses α−β privacy where α is what an adversary

knows, and β are the cryptographic messages that the

adversary sees. Then α − β privacy means that the

intruder can derive from β only what he can derive

from α already. We have not tried it, but we think

that our notion of privacy-preservation can be for-

mulated in term of α − β privacy. It that were true,

since M¨odersheim et al. prove that their deﬁnition

subsumes static equivalence of frames and is in some

part decidable with existing formal method tools, we

would gain further ways to prove our notion formally.

This paper also relates with the research on veriﬁ-

ability. This is a property that has been studied preva-

lently, but not exclusively, in secure voting, where

different models and requirements have been pro-

posed (K¨usters et al., 2010; Kremer et al., 2010).

For instance, individual veriﬁability is introduced as

the ability that voters have to prove that their votes

have been “cast as intended”, “recorded as cast”, and

“counted as recorded” (Benaloh and Tuinstra, 1994;

Hirt and Sako, 2000), while universal veriﬁability as

the ability to verify the correctness of the tally using

only public information (Cohen and Fischer, 1985;

Benaloh and Tuinstra, 1994; Benaloh, 1996). Tools

have been proposed to analyse the security of veriﬁa-

bility: Kremer et al. (Kremer et al., 2010) formalise

individual and universal veriﬁability in the applied pi-

calculus, and Smyth et al. (Smyth et al., 2010) use

ProVerif to check veriﬁability in three voting proto-

cols.

We also base our deﬁnition of veriﬁability on pre-

vious work. The formalization we propose is based

on the model proposed in (Dreier et al., 2015) This

choice is not a coincidence. That work is about veriﬁ-

able e-exams, the same domain that we take here as a

use case to demonstrate that our notion of privacy-

preserving veriﬁability is meaningful and effective.

Besides, that work proves that Remark!, an exam

protocol rich in security properties (Giustolisi et al.,

2014), is veriﬁable. Such a proof is fundamental

for this paper, since we need to consider a protocol

that is veriﬁable although we need it be not privacy-

preserving. Thus, as use case, we also chose Remark!.

3 MODELS & DEFINITIONS

We refer to the speciﬁc domain of exams. We con-

sider three fundamental roles: the candidate, who

takes the test; the exam authority, who helps run the

exam; the examiner, who grades the tests.

An exam’s workﬂow is rigidly structured in dis-

tinct sequential phases: Preparation, where an exam’s

instance is created, the questions are selected, and

the candidates enrol; Examination, where the candi-

dates are identiﬁed and checked for being eligible, re-

ceive the test sheet(s), answer the questions, and quit

SECRYPT 2017 - 14th International Conference on Security and Cryptography

140

or submit their answers for marking; Marking, where

the exam-tests are assessed and marked; Notiﬁcation,

where grades are notiﬁed and registered.

An Exam’s Abstract Model. An exam and its exe-

cution can be modelled concisely using sets. The deﬁ-

nitions below, slightly simpliﬁed, comes from (Dreier

et al., 2015)

Deﬁnition 1. An exam is a tuple (ID, Q, A, M) of ﬁnite

sets: ID are the candidates; Q are all the possible

questions; A the possible answers; M all the possible

marks.

Deﬁnition 2. Given an exam (ID, Q, A, M), an exam’s

execution, E , is a tuple of two sets

• ID

′

⊆ ID are the registered candidates;

• Q

′

⊆ Q are the ofﬁcial questions of the exam;

and of four relations over the exam

• Assigne dQuestions ⊆ (ID × Q), links a ques-

tion to a candidate, supposedly the question the

candidate receives by the authority;

• Submitt edTests ⊆ (ID× (Q× A)), links an an-

swered test to a candidate, supposedly the test

submitted for marking by the candidate;

• MarkedT ests ⊆ (ID×(Q×A)×M) links a mark

to a exam-tests of a candidate, supposedly the

grade given to the test;

• Notifie dMarks ⊆ (ID × M) links a mark to a

candidate, supposedly the mark notiﬁed to the

candidate;

These four relations model the state of the exam’s

execution at the end of each of the four phases. They

are, in fact, execution logs. There are no requirements

between the relations, and this is because the model is

meant to capture also executions which are erroneous

or corrupted. It may happen that a test assigned to no

one is submitted fraudulently (i.e., creation of test) or

that a test assigned to a candidate is duplicated and

answered in two possible ways, and both copies are

submitted (i.e., duplication of a test). It may happen

as well as that a submitted test is never marked be-

cause it got lost (i.e., loss of a legitimately submitted

and answered test).

Authentication and integrity properties are ex-

pressed as predicates over E . Let c, q, a and m range

over ID, Q, A and M respectively. An example of

property is Marking Integrity, which states that no

mark has changed from marking to notiﬁcation, and

it is written as follows:

NotifiedMarks ⊆

{(c, m) : (c, (q, a), m) ∈ MarkedT ests}

Another property, Testing Integrity expresses that all

the exams that have been marked are submitted for

marking. It is as follows:

{(q, a) : (c, (q, a), m) ∈ MarkedTests)} ⊆

{(q, a) : (c, (q, a)) ∈ SubmittedTests}

Properties can be easily combined. For example,

Marking and Test Integrity is obtained by conjunction

from the previous properties:

NotifiedMarks ⊆

{(c, m) : (c, (q, a), m) ∈ MarkedT ests

and (c, (q, a)) ∈ SubmittedTests}

(1)

Deﬁnition 2 serves well also to express properties

of veriﬁability of a generic security property p. An

exam protocol is p-veriﬁable if it has a test for p, say

test

. This is an algorithm that given any exam’s

execution E and some knowledge K (e.g., decryption

keys) returns true if and only if p holds on E . For-

mally, p-veriﬁability can be expressed as

∀E , test

(E, K) iff E |= p (2)

where E |= p means that p holds on E.

A Reﬁned Exam’s Abstract Model. Dreier et al.’s

model partially serves the goal of this paper: it is too

abstract to capture non-trivial notions of privacy like

the one we intend to advance (see Deﬁnition 4). We

need a formalism able to model encrypted logs, tests

which run over them, and knowledge gained from

running a test and about the exam’s run. It is in the

interplay between these elements where our notion of

privacy-preserving veriﬁability will emerge.

Precisely, besides the abstract model, E , which

represents an exam’s log always in clear-text, we con-

sider a concrete model, {E}, which models the actual

and possibly encrypted logs of an exam’s execution.

A veriﬁability test is executed on the concrete model

{E }.

Deﬁnition 3. Let E and {E} be an abstract and a

concrete exam’s execution respectively, and let p be a

security property over E . Then, p is veriﬁable if there

exists a test for p, test

, that given some knowledge

K satisﬁes the following condition:

∀E , test

({E }, K) iff E |= p (3)

Any proof aiming at convincing that p is veriﬁable

needs to clarify how the test that operates on {E } is

sound and complete with respect to the validity of p

on E .

The notion of privacy-preserving veriﬁability is

deﬁned in respect to what a veriﬁer, say v, can learn

Privacy-Preserving Veriﬁability - A Case for an Electronic Exam Protocol

141

about E by running test

({E }, K). We are, how-

ever, not interested to capture what v can learn in-

crementally by running several and cleverly selected

tests. Potentially, quite a deal can be deduced by in-

ference attacks, see (Naveed et al., 2015), but study-

ing the leaks due to such attacks is a goal outside the

scope of this paper.

Let [{E}]

be the portion of {E} to which a ver-

iﬁer needs to access to run a test for p. Since [{E }]

can be encrypted, v does not necessarily learn about

the exam’s execution E . It depends on what v can do

from knowing [{E}]

and K. If we assume v be a pas-

sive but curious adversary, and if we assume that the

learning process works at the symbolic level of mes-

sages, the deduction relation ⊢ over messages deﬁnes

the ability to learn. It is the minimal relation that sat-

isﬁes the following equations:

, k

−1

⊢ m

(m, m

′

) ⊢ m and m

′

In the relation above, we abstract from symmet-

ric/asymmetric encryption: k

−1

is the key to decrypt,

whatever the paradigm of reference.

Deﬁnition 4. Let E be any execution of an exam pro-

tocol and test

a test for veriﬁability for a property

p. The test is privacy-preserving if by running it v

does not learn more about the exam execution than

he knows from the information he is given to run the

test. Formally:

{m : ([{E }]

∪ K) ⊢ m} ∩ E = ([{E }]

∪ K) ∩ E

Deﬁnition 4 says that v should learn about E no

more than what he knows already from the portion of

the exam’s log and from additional knowledge, i.e.,

from the pieces of information that he needs to know

to run the test.

In Deﬁnition 4 we haveslightly overloaded the op-

erator ∩. Since E is a tuple of sets it must be consid-

ered applied over each element of the tuple that is:

(A, B)∩C = C∩(A, B) = (A∩C, B∩C). Similarly the

interpretation of ⊆ when applied to tuple of sets, must

be extended to tuple, that is: (A, B) ⊆ (C, D) iff A ⊆

C and B ⊆ D.

Deﬁnition 5 (Privacy-Preserving Veriﬁability). A

protocol is privacy-preserving veriﬁable with respect

to a property p, if p is veriﬁable and admits a veriﬁa-

bility test test

that is privacy-preserving.

4 MEANINGFULNESS

Claim 1. Our notion of privacy-preserving veriﬁabil-

ity is meaningful: it can be used to distinguish veriﬁ-

able protocols that are privacy-preserving from those

which are not.

To prove the claim, we refer to an e-exam proto-

col whose veriﬁability (with respect to several prop-

erties) has been established formally. The protocol is

called Remark! (Giustolisi et al., 2014). The proto-

col is structured in the four typical phases of an exam.

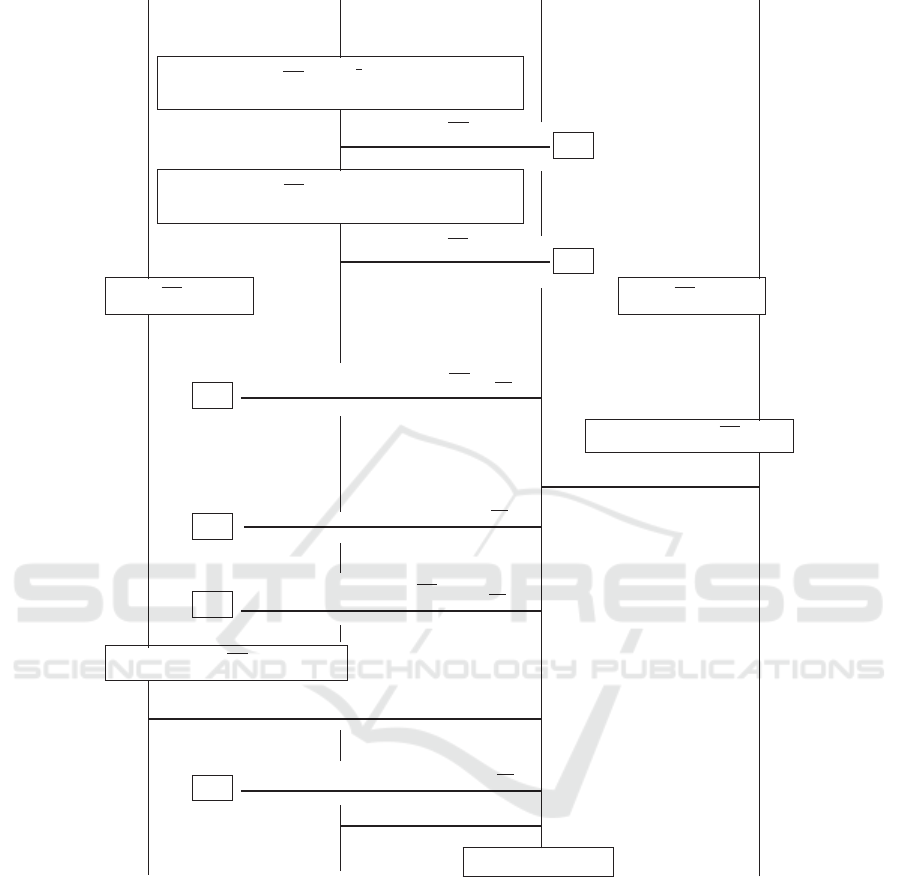
Its message ﬂow is recalled in Figure 1, where C is a

candidate and E is an examiner. Only one candidate

and one examiner are shown for conciseness, but the

protocol is supposed to work for all candidates and

all examiners and it is executed by them all. An exam

authority, A, helps the process.

Description. The Preparation is the phase where

pseudonyms are generated to ensure a double blind

anonymity in testing and marking. Here, n exponen-

tial mixnets, M in Figure, generate the pseudonyms

for the registered candidate C. The candi-

date already possesses a pair of public/private keys,

hPk

, Sk

i and uses the private key to recognize its

designated pseudonym among the pseudonyms gen-

erated for all the candidates and posted on a pub-

lic bulletin board (BB, in Figure). Similarly, the

mixnets generate pseudonyms for the examiner. A

zero-knowledge proof of the generation is present on

the bulletin board as proof of correctness of the gen-

eration process.

At Examination, the selected questions are signed

by the authority and encrypted with the pseudonym of

the candidates. The questions are posted on the bul-

letin board from where the candidates retrieve them.

Then they answer the questions. In Figure, ques is

the question and ans is an answer. The candidate C

prepares a tuple hques, ans,

i, which he signs with

its private key Sk

. The tuple is then encrypts with

the public key of the authority and send to it. The au-

thority decrypts and re-encrypts the message with the

candidate pseudonym and publishes the message on

the bulletin board.

At Marking the authority dispatches the tests to

the examiners. In Figure, A signs the answered test

and uses the pseudonyms of the examiner, i.e.,

as encryption keys. Hevene re-encrypts with it the

answered test and publishes it on the bulletin board.

From there, similarly to what the candidates did with

the questions, E retrieves the test, marks them (i.e.,

assigns a mark M), and prepares a tuple with the an-

swered tests signed by the authority and the marking,

which E signs further with its secret key Sk

. En-

crypted this with the public key of the authority, E

returns this message to the A who, in turn, decrypts

the marking and re-encrypts it with the pseudonym of

the candidate

At Notiﬁcation the candidates’ anonymity is re-

voked and the marks can ﬁnally be registered. The

SECRYPT 2017 - 14th International Conference on Security and Cryptography

142

phase starts when the authority publishes all the

marks together. On request the mixnets reveal (on an

authenticated encrypted channel like one using TLS)

the random values used to generate the pseudonyms

(in Figure,

, for C).

Security Properties. Remark! ensures several au-

thentication, anonymity, privacy and veriﬁability

properties. Here, we focus on veriﬁability, in partic-

ular on universal veriﬁability. Universal means that

anyone only by using public knowledge and without

having participated in the protocol execution can ver-

ify that a speciﬁc property holds over the execution.

The public data is generally that available on the bul-

letin board; the pieces of additional information are

explicitly provided for the test, e.g., by the authority.

Remark! has been proven to be veriﬁable for ﬁve

properties (Dreier et al., 2014):

Registration Correctness: all accepted tests are sub-

mitted by registered candidates.

Marking Correctness: all the marks attributed by

the examiners to the tests are computed correctly.

Test Integrity: all and only accepted tests are

marked without any modiﬁcation

Test Markedness: only the accepted tests are

marked without modiﬁcation

Mark Integrity: all and only the marks associated to

the tests are assigned to the corresponding candi-

dates with no modiﬁcations

The properties are veriﬁable only if the exam au-

thority handles to the veriﬁer some data after the

exam has ended. For instance to verify Registra-

tion Correctness the manager must reveal the signa-

tures inside the receipts {Sign

(H(T

)}

posted

on the bulletin board and the random values used to

encrypt the receipts. To verify Marking Correctness,

the manager must reveal the marked exam-tests inside

the evaluations {Sign

)}

, the random val-

ues used to encrypt the marked tests, and the table

correct

ans (not described in Figure 1) that deﬁnes

what is the mark given a question and an answer; to

verify Test Integrity and Test Markedness, the man-

ager must reveal the marked exam-tests inside the

evaluations, the random values used to encrypt the

marked tests, plus the data disclosed for Registration;

ﬁnally, to verify Mark Integrity, the manager must re-

veal the examiners’ signatures on the marked exam-

tests inside the evaluations, and the random values

used to encrypt the notiﬁcations {Sign

)}

before posting them on the bulletin board.

Discussion. Let us see where Remark!’s veriﬁabil-

ity fails to be privacy-preserving.

To verify Registration Correctness, Remark! pre-

scribes a test that takes the pseudonyms of the

candidates signed by the mixnet, i.e., message

Sign

(

, h

), and the receipts of submissions

generated by the exam authority i.e., messages

{Sign

(H(T

)}

. The test succeeds if for each

pseudonym there is a unique receipt of submission.

The veriﬁer does not know any other information

about the executionof the exam apart the pseudonyms

and the hash version of the tests. Hence, the registra-

tion test satisﬁes Deﬁnition 4.

To verify Marking Correctness, the protocol re-

quires a test that inputs Sign

(

, h

), the ta-

ble correct ans, and the mark notiﬁcations signed by

the examiner and published by the exam authority

{Sign

)}

. The test needs also the help of

the exam authority that decrypt these last messages,

and revealsthe M

= hSign

(

, T

), marki which

reveals the pseudonym of the examiner who marked

the answers, the questions, and the mark. This test

does not satisfy Deﬁnition 4: the veriﬁer learns the

questions, the answers, and the link between candi-

date and examiner pseudonyms.

To verify Test Integrity, the protocol needs a

test that takes message Sign

(

, h

), the re-

ceipts of submissions {Sign

(H(T

)}

, and the

mark notiﬁcations generated by the exam authority

{Sign

)}

. This test reveals to the veriﬁer

questions, answers, and the link between candidate

and examiner pseudonyms, which are leaked in the

mark notiﬁcations, so it does not satisfy Deﬁnition 4

To verify Test Markedness a test needs to input the

same data as in test integrity. The same considerations

outlined above applies for this test and the test does

not satisfy Deﬁnition 4.

To verify Mark Integrity, Remark! pre-

scribes a test that takes in the mark notiﬁcations

{Sign

)}

and allows the veriﬁer to check

if the marks that the exam authority assigned to the

candidates (in Figure, Register Pk

, mark) coincide

with the marks that the examiner assigned to the

candidates tests in M

= hSign

(

, T

), marki.

Once more, the knowledge of questions, answers, and

link between candidate and examiner pseudonyms is

leaked by M

, although those pieces of information

are not necessary to evaluate mark integrity. Also this

test does not respect Deﬁnition 4.

Overall, Remark! is privacy-preserving only for

Registration Correctness veriﬁability.

Privacy-Preserving Veriﬁability - A Case for an Electronic Exam Protocol

143

Examiner

Candidate

Mixnet

Exam Authority

q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q

Preparation

¯r

∏

i=1

= Pk

, h

= g

¯r

′

∏

i=1

′

= Pk

¯r

′

, h

= g

¯r

′

✲

1 : Sign

(

, h

)

✲

2 : Sign

(

, h

)

Check Pk

= h

Check Pk

= h

q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q

Testing

✛

3 : {Sign

(quest,

)}

✛

= hquest, ans, Pk

4 : {Sign

)}

✛

5 : {Sign

(H(T

)}

q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q

Marking

BB ✛

6 : {Sign

(

, T

)}

✲

= hSign

(

, T

), marki

7 : {Sign

)}

q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q

Notification

✛

8 : {Sign

)}

✲

9 : ¯r

(T LS)

, mark

Figure 1: The message ﬂow of Remark!

5 APPLICABILITY

Except for Registration Correctness, all the tests for

universal veriﬁability that Remark! offers are not pri-

vacy preserving. Here, we show how to make them

privacy-preserving. For reason of space we show the

technique only that the test about Mark and Test In-

tegrity veriﬁability. We claim, but we leave a proof

for future work, that we can apply the same idea of

solution to achieveprivacy-preservingveriﬁability for

all the other veriﬁability tests.

To achieve our goal we propose a version of Re-

mark! that relies on Functional Encryption (FE). This

is an encrypting paradigm that relies on the notion of

FE scheme (Boneh et al., 2011).

Deﬁnition 6. A FE scheme is a 4-tuple of functions

(Setup, KeyGen, Enc, Eval), where:

1. Setup(1

, 1

) returns a pair of keys (Pk, Msk).

The ﬁrst is a public key and the other a secret mas-

ter key. The keys depends on two parameters: λ,

a security parameter and n, a length. They are

SECRYPT 2017 - 14th International Conference on Security and Cryptography

144

polynomially related.

2. KeyGen(Msk, C) is a function that returns a to-

ken, Tok

Msk

. A token is a string that when evalu-

ated (see item 4, function Eval) on speciﬁc cipher-

texts encrypted with Pk returns the same output

as the Boolean Circuit C (a decider) would re-

turn if it were applied on the corresponding plain-

texts. We talk about Boolean Circuits (Jukna,

2012) because the “functionality” in Functional

Encryption is deﬁned on this model of computa-

tion. Here, C ∈ C

, wheren C

is the class of

Boolean Circuit on inputs long n bits.

3. Enc(Pk, m) encrypts the plaintext m ∈ {0, 1}

with the public key Pk.

4. Eval(Pk, Ct, Tok

Msk

) is the function that pro-

cesses the token as we anticipated in item 2. On

input Ct = Enc(Pk, m) the function returns C(m)

that is the same boolean output (accept/reject) as

that of circuit C applied on string m. The key Msk

embedded in the token is used retrieve the plain-

text m from Ct. For any other input than the spec-

iﬁed Ct, Eval is undeﬁned and returns ⊥.

A FE scheme should be of course instantiated

cryptographically, but whatever implementation we

chose it must satisfy two speciﬁc requirements: cor-

rectness and secure indistinguishability.

The requirement of correctness says that the func-

tions in Deﬁnition 6 behave as described: for all

(Pk, Msk) ← Setup(1

, 1

), and all C ∈ C

and m ∈

{0, 1}

, and for Tok

Msk

← KeyGen(Msk, C) and Ct ←

Enc(Pk, m) then Eval(Pk, Ct, Tok

Msk

) = C(m). Oth-

erwise it returns ⊥.

The requirement of secure indistinguishability

says that the scheme is “cryptographically secure”

against any active and strong enough adversary

(i.e., uniform probabilistic polynomial time adver-

saries). Such an adversary, despite having seen

tokens Tok

Msk

, cannot distinguish (with a non-

negligible advantage over pure guessing) two ci-

phertexts Enc(Pk, m

) and Enc(Pk, m

) for which

C(m

) = C(m

A stronger security requirement used in FE is

simulation-security, but due to impossibility results

for it (see (Boneh et al., 2011)), we limit our assump-

tion to indistinguishability security.

5.1 Revising the Protocol

We modify Remark! and let it have a test for Uni-

versal Marking and Test Integrity (UMTI) veriﬁabil-

ity that is privacy-preserving according to our Deﬁni-

tion 4. We use a FE scheme to encrypt the messages

that the veriﬁer needs to process when running the

test. From §3 we recall that the test should decide the

following predicate:

test

UMTI

({E }, K) iff (NotifiedMarks ⊆

{(c, m) : (c, (q, a), m) ∈ MarkedT ests

and (c, (q, a)) ∈ SubmittedTests})

(4)

The test should succeed if and only if the set of

all notiﬁed marks to candidates is included in the set

of exams that have been marked for those candidates

and the exams marked should be those that the have

submitted for marking.

As we discussed in §4, in Remark! this test allows

the veriﬁer see all messages Sign

) which he

needs to build the sets as in Equation (4). Thus, the

veriﬁer gets to know the questions and answers and

their link with the candidate identiﬁers.

By resorting to a FE scheme we intend to achieve

a version of the test that implements predicate (4) that

does not reveal a candidate’s questions and answers,

which is a sufﬁcient condition to satisfy Deﬁnition

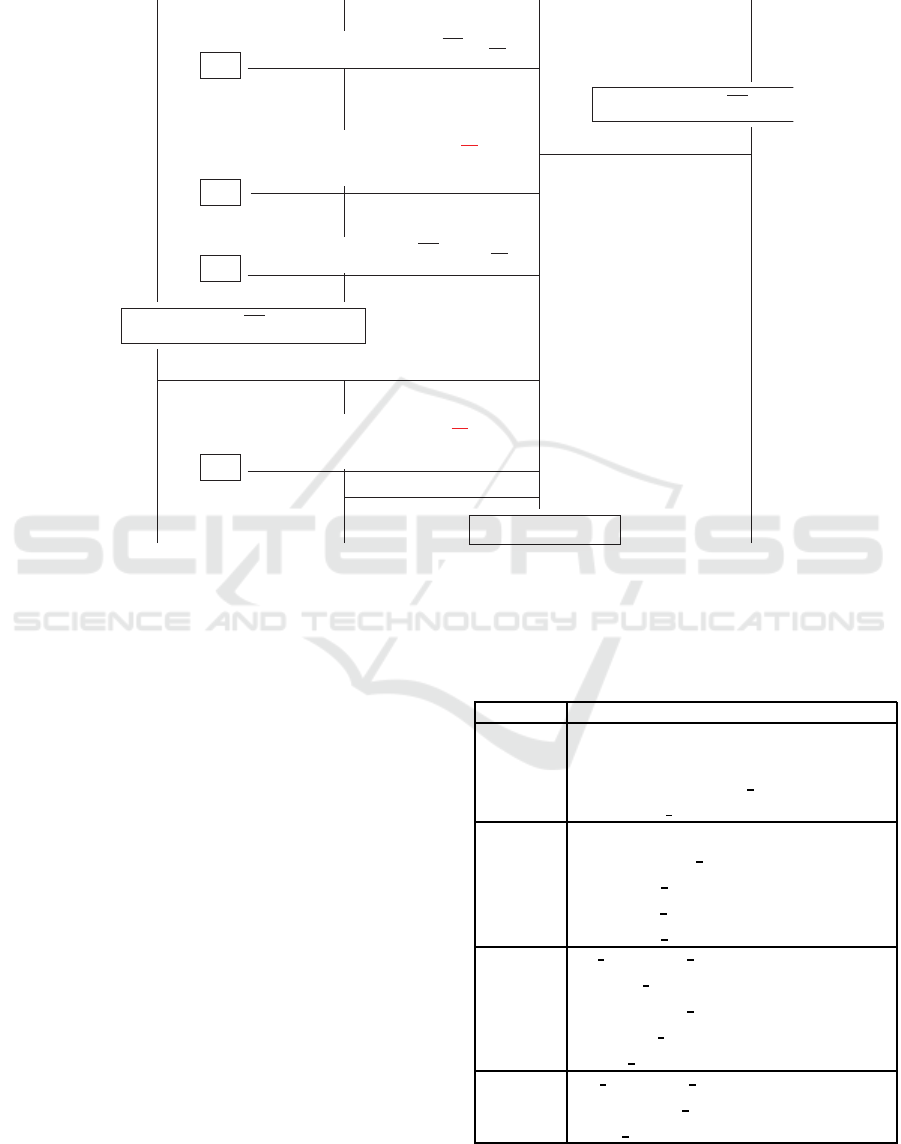
5. Figure 2 shows the new protocol, but reports only

the messages that have been modiﬁed. We assume

a trusted FE authority that generates the public Pk

from Msk. It can be any party, internal or external, but

must be trusted in this particular task. It is implicit in

the Figure 2, visible only when we use Pk

. Mes-

sages in step 5 and in step 8 are those which change.

In 5 the exam authority encrypts with Pk

the signa-

ture of a test T

that a candidate submitted for mark-

ing. It also post a digest of the same message, signed

with the candidate’s pseudonym. To avoid that the

message encrypted under Pk

and that encrypted un-

der P k

hide two different values (i.e., y and T

such

that H(T

) 6= y), we require a non-interactive zero

knowledge proof (NIZK) of consistency, π in Figure.

Similarly, in step 8 the authority encrypts with Pk

the marking and another NIZK π

′

is required.

5.2 Revising the Veriﬁability Test

Ciphertexts in 5 and in 8 will be used in combination

with the tokens that the FE authority prepares for the

veriﬁer. It needs tokens that implement two functions:

The ﬁrst, function f

, embeds the public-keys

and Pk

and the mark mark to be veriﬁed. It is sup-

posed to input Sign

), the message in 8. Af-

ter properly parsing the strings, checks whether the

mark, say mark

′

, contained in M

is equal to mark,

checks the signature and checks whether the

′

con-

tained in T

(in turn, contained in M

) equals

. It

also veriﬁes the validity of the zero-knowledge proof

π. If a check fails, the function returns 0. If all checks

succeed, it returns 1. Function f

is coded as follows:

Privacy-Preserving Veriﬁability - A Case for an Electronic Exam Protocol

145

Function f

[Pk

, mark, Pk

](σ)

1. If VERIFY(σ, Pk

) = 0 then Return 0

//veriﬁes a signature, returns 0 on fails

2. Parse σ as Sign

)

3. Parse M

as hSign

(

, T

), mark

′

(get mark

′

, T

)

4. Parse T

as hquest, ans,

′

(get Pk

′

)

5. If mark

′

6= mark Return 0

6. If

′

6= Pk

Return 0

7. Return 1

The second function, f

, embeds the public-key Pk

and the message Sign

). It expects to input

the second part of message 5, Sign

). It

checks the signatures and whether both messages

refer to the same exam test. The function returns 0 as

soon as one check fails, 1 otherwise. Function f

coded as follows:

Function f

[Pk

, Sign

)](σ)

1. If VERIFY(σ, Pk

) = 0 then Return 0

//veriﬁes a signature, returns 0 on fails

2. Parse σ as Sign

)

(get T

)

3. Parse M

as hSign

(

, T

′

), marki

(get T

′

)

4. If T

6= T

′

Return 0

5. Return 1

The veriﬁability test uses two sets of tokens of form

Tok

Msk

[x]

and Tok

Msk

[x]

where x ranges over the token

embedded parameters.

The test then calls the function Eval over those to-

kens to check whether the f

and f

holds on the mes-

sages found on the bulletin board that correspond the

the messages that the veriﬁer needs to implement the

test in Equation (4).

test

UMTI

({E }, K) ﬁrst veriﬁes whether for each

notiﬁed mark per candidate there exists at least one

message among those in step 8 that corresponds to

the mark for a test of that candidate; if one is found

the test continues and checks whether there is at least

one message among those in step 5 that corresponds

to that exam for that candidate submitted for marking.

The test requires to know the markings that have

been notiﬁed to the candidates and PK

the public

key of the authority, so K = {(Pk

, mark

) : C ∈

} ∪ {Pk

}. Formally the test is implemented by

the following algorithm:

function test

UMTI

({E}, K):

for (Pk

, mark

) ∈ K do

Tok

← GETTOK( f

[Pk

, mark

, Pk

])

for ({Sign

)}

, π) ∈ {E} do

← Eval(Tok

, {Sign

)}

);

if b

then ExitLoop;

if b

then

′

← VERIFY(π for R);

Tok

← GETTOK( f

[Sign

)]);

for ({Sign

)}

, π

′

)∈{E} do

← Eval(Tok

, {Sign

))}

);

if b

then ExitLoop

if b

then

′

← VERIFY(π

′

for R

′

);

return b

· b

′

· b

′

GETTOK is used to retrievea speciﬁc token. Here,

we use the function’s embedded parameters to indi-

cate which token is being requested. However, the

veriﬁer does not necessarily know the value of the pa-

rameter to perform such request, but he must be able

to indicate the token he needs e.g., by means of an

index. Relations R and R

′

are so deﬁned:

Relation R[Pk

, Pk

, H](x)

1. Parse x as

(Ct

= {Sign

(y)}

= {Sign

)}

)

(get y and T

)

2. If H(T

) 6= y Return 0

3. Return 1

Relation R

′

[Pk

, Pk

](x)

1. Parse x as

(Ct

= {Sign

(y)}

= {Sign

)}

)

(get y and T

)

2. If T

6= y Return 0

3. Return 1

Discussion. We argue that test

UMTI

({E }, K) is

privacy preserving. By assumption, the FE scheme

satisﬁes the indistinguishability security. Thus, a

Dolev-Yao attacker, given a ciphertext Ct encrypt-

ing a message m and a token for a function f, can

only derive f(m). This holds also for a curious ver-

iﬁer who observes the tokens for the two functions

and f

. The crucial consideration is that, by con-

struction, such two functions return a Boolean value

and nothing about the messages that are considered

in our model (cf. Deﬁnition 1) of an exam’s execu-

tion. Moreover, among the messages that the veriﬁer

gets in input, precisely in K, there is nothing that al-

lows him to decrypt other messages on the bulletin

board. Actually, all the decryption operations that the

veriﬁer needs are realized within the function Eval.

Therefore, it follows that test

UMTI

({E }, K) is pri-

vacy preserving according to Deﬁnition 4.

SECRYPT 2017 - 14th International Conference on Security and Cryptography

146

∼

Examiner

Candidate

Mixnet

Exam Authority

q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q

Testing

✛

3 : {Sign

(quest, Pk

)}

✛

= hquest, ans, Pk

4 : {Sign

)}

✛

5 : {Sign

(H(T

)}

{Sign

)}

, π

q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q

Marking

BB ✛

6 : {Sign

(Pk

, T

)}

✲

= hSign

(Pk

, T

), marki

7 : {Sign

)}

q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q q

Notification

✛

8 : {Sign

)}

{Sign

)}

, π

′

✲

9 : ¯r

(T L S)

, mark

Figure 2: A revision of Remark! using FE (only phases that have been changed).

6 FORMAL ANALYSIS

We prove formally that the veriﬁability test is sound

and complete and that the new protocol does satisfy

the same security properties as the original Remark!.

This exercise of formal analysis is necessary because

we intend to get insurance than we achieve privacy-

preserving veriﬁability incrementally,on top of all the

authentication, integrity and privacy properties hold-

ing on the protocol and not on their expenses.

We perform the analysis in Proverif (Blanchet,

2014). Our ProVerif model of the protocol is an ex-

tension of the original Remark! model as it was pre-

sented in (Dreier et al., 2014). The extension mainly

regards the following aspects:

• A ProVerif speciﬁcation that reﬂects the new pro-

tocol description.

• A new equational theory for the Functional En-

cryption primitives deﬁned in our protocol.

• A new UMTI veriﬁability test.

The equational theory is illustrated in Table 1 to

model the cryptographic primitives of the protocol.

Table 1: Equational theory in formal analysis of the privacy-

preserving veriﬁability version of Remark!

Name Equation

Signature

getmess(sig(m, k)) = m

checksig(sig(m, k), spk(k)) = m

checksig(sig(m, ps pr(k,

exp(rce))), ps

pub(pk(k), rce)) = m

ElGamal

decrypt(enc(m, pk(k), r), k) = m

decrypt(enc(m, ps

pub(pk(k),

rce), r), ps

pr(k, exp(rce))) = m

checkps(ps

pub(pk(k), rce),

pr(k, exp(rce))) = true

FE f1

eval f1(pk(k), ps pub(pk(k), rc),

pub(pk(k), re), mark,

enc(sig(sig(ps

pub(pk(k), re),

(q, a, ps

pub(pk(k), rc)), k),

mark, ps

pr(k, exp(re))), pk(k))) = true

FE f2

eval f2(pk(k), ps pub(pk(k), rc),

enc(sig((q, a, ps

pub(pk(k), rc)),

pr(k, exp(rce))), pk(k))) = true

Privacy-Preserving Veriﬁability - A Case for an Electronic Exam Protocol

147

The equations that model the digital signature are

rather standard in ProVerif.

ElGamal encryption is extended with equa-

tions that model pseudonyms as public keys: the

pseudonym, which also serves as test identiﬁer, can

be generated using the function ps

pub which takes

in a public key and a random exponent. In fact,

this function models the main feature of exponenti-

ation mixnet. Function ps

pr can be used by a prin-

cipal to decrypt or sign anonymous messages. The

function takes in the private key of the principal and

the new generator published by the mixnet. Func-

tion checkps allows a principal to check whether a

pseudonym is associated with the principal’s private

key. In practice, principals use this function to iden-

tify their pseudonyms published on the bulletin board.

A public channel models the bulletin board.

The the equations that deﬁnes eval

f1 and eval f2

model the two Functional Encryption primitives f

and f

respectively. They return true if and only if the

corresponding plaintext version f

and f

return true.

Note that our equational theory of functional encryp-

tion captures the property of veriﬁable FE. Whenever

an encrypted message has a form that is not consis-

tent with the constructor enc, the deterministic eval

fails, and no rule can be applied. We do not explicitly

model the generation of the token: Setup and KeyGen

are generated by a trustworthy authority. It follows

that the only two allowable functions are f

and f

Veriﬁability. From Deﬁnition 3, we recall that a

protocol is p-veriﬁable if it admits a sound and com-

plete algorithm that decides p. Soundness means that

the veriﬁability-test returns

true

only if the property

holds. Completeness means that if the property holds,

the veriﬁability-test always returns

true

We use ProVerif’s correspondence assertions to

prove soundness. The veriﬁcation strategy consists of

checking that the event

emitted by the veriﬁability-

test when it is supposed to return

true

is always pre-

ceded by the event emitted in the part of the code

where the property becomes satisﬁed. In case of Mark

and Test Integrity, this happens when the exam au-

thority assigns the mark to the candidate.

We resort to the unreachability of the event

which is emitted by the veriﬁability-test when it is

supposed to return

false

, to prove completeness. In

this case, the ProVerif model enforces only honest

principals and prevents the attacker to manipulate the

input data of the veriﬁability-test. In fact, a com-

plete veriﬁability-test must succeed if its input data

is correct. ProVerif conﬁrms that our protocol meets

UMTI veriﬁability and the veriﬁability-test is sound

and complete

We observe that ProVerif can only prove the case

in which only one entry on the bulletin board is con-

sidered. Mark Integrity veriﬁability requires that all

and only the marks associated to the tests are assigned

to the corresponding candidates. Thus, we need to

iterate over all candidates who submitted their tests,

but ProVerif cannot do this automatically as it does

not support loops. We resort on the manual induction

proofs provided by Dreier et al. (Dreier et al., 2014)

in which the base case is given by the automated re-

sult in ProVerif, and then generalise such result to the

general case with an arbitrary number of candidates,

tests, and marks. Those proofs applies to the original

Remark! as well as to this new version. In fact, the

assumption that the auditor can check the correspon-

dences of the assigned entries to the marked entries

on the bulletin board does not change: each entry that

appears on the bulletin board at step 6 of the protocol

can be matched to an entry that appears on the bulletin

board at step 8 of the protocol.

Authentication. The new protocol meets all the

original authentication properties of Remark!. Since

the new protocol differs from Remark! only in mes-

sages 5 and 8, we could reuse the same authentication

deﬁnitions advanced by Dreier et al. (Dreier et al.,

2014) and verify them in Proverif.

7 CONCLUSION

In this pioneering work, we put forth a new notion of

security that entails features of both veriﬁability and

privacy. To illustrate the beneﬁts of our framework,

we proved that our deﬁnition is meaningful enough to

empower applications in the concrete domain of elec-

tronic exams and in particular to an extension of an

exam protocol that satisﬁes several veriﬁability prop-

erties but which is not compliantly with our privacy-

preserving requirement.

To achieve our goals, we employ the crypto-

graphic tool of Functional Encryption (FE). Only with

FE we can verify obliviously a property that is global

over the execution of the protocol, a property that

is about the data handled and processed by several

agents in the protocol’s run. In making this choice

we considered to obtained the same effect with other

tools, the most promising be zero knowledge proofs,

but, we claim, using this tool would have required

an authority that looks at the execution and adds

The full ProVerif code is available on request.

SECRYPT 2017 - 14th International Conference on Security and Cryptography

148

the proofs and that interacts with the several proto-

col agent’s asking them for other Zero-Knowledge

proofs, so changing too much how an exam should

work. This argument requires however a proof and

we plan to inquire into this question as future work.

We have not benchmarked the new protocol. At

current stage, our result is only of theoretical rele-

vance due to the high computational cost of FE for

circuits that we assumed and for this reason we rec-

ognize that FE may look as be an overkilling to some-

one. However, there is another feature of electronic

exams that must be considered here. The limited di-

mension of the an exam’s audiences and the expected

time of an exam’s notiﬁcation makes feasible im-

plementations that rely on time-inefﬁcient encryption

schemes. Comparing with electronic voting, for in-

stance, where a whole country is involvedand where a

result is nowadays expected be announced within the

day, for an exam the expected audiences is deﬁnitely

far more contained while waiting weeks is a perfectly

acceptable time frame to get notiﬁed of the result.

We defer to further research about implementing our

privacy-preserving veriﬁability notion efﬁciently.

It should be stressed that although contextualized

in reference to exams, our research is not bound to

work in that domain only. The notion of privacy-

preserving veriﬁability is abstract and the solution

that we propose to ensure privacy-preservation is

demonstrated for a universal veriﬁability test of a

common integrity and authentiation property. There-

for it seems plausible to apply our results in other do-

mains, like voting or auction, where the veriﬁability

properties are also about integrity and authentication.

Proving this claim is future work.

We conclude by pointing to a future work for

us and an open problem to whom it may be inter-

ested: to study the relation between our notion and

that presented by M¨odersheim et al. of α-β pri-

vacy (M¨odersheim et al., 2013). Were this correlation

proved, we could gain a straightforward way to verify

formally privacy-preserving veriﬁability through the

fact α-β privacy subsumes static equivalence.

ACKOWLEDGEMENTS

R. Giustolisi’s research is supported in part by

DemTech grant 10-092309, Danish Council for

Strategic Research, Programme Commission on

Strategic Growth Technologies; G. Lenzini’s by the

SnT / pEp Security partnership project “Protocol of

Privacy Security Analisys”. V. Iovino is supported by

a FNR CORE grant (no. FNR11299247) of the Lux-

embourg National Research Fund.

REFERENCES

Adida, B. and Neff, C. A. (2006). Ballot Casting Assur-

ance. In Proc. of the USENIX/Accurate Electronic

Voting Technology, EVT’06, p. 7–7. USENIX Asso-

ciation.

Benaloh, J. (1996). Veriﬁable Secret-Ballot Elections. PhD

thesis, Yale University.

Benaloh, J. and Tuinstra, D. (1994). Receipt-free secret-

ballot elections. In 26th Sym. on Theory of Computing,

STOC’94, p. 544–553, New York, NY, USA. ACM.

Blanchet, B. (2014). Automatic Veriﬁcation of Secu-

rity Protocols in the Symbolic Model: the Veriﬁer

ProVerif. In Foundations of Security Analysis and De-

sign VII, FOSAD Tutorial Lectures, volume 8604 of

LNCS, p. 54–87. Springer.

Boneh, D., Sahai, A., and Waters, B. (2011). Functional en-

cryption: Deﬁnitions and challenges. In 8th Theory of

Cryptography Conf., TCC 11, volume 6597 of LNCS,

p. 253–273. Springer.

Cohen, J. and Fischer, M. (1985). A robust and veri-

ﬁable cryptographically secure election scheme. In

26th Ann. Sym. on Foundations of Computer Science,

FOCS’85, p. 372–382, Portland, Oregon, USA. IEEE

Computer Society.

Cuvelier, E., Pereira, O., and Peters, T. (2013). Election Ver-

iﬁability or Ballot Privacy: Do We Need to Choose?,

p. 481–498. Springer.

De Santis, A., Micali, S., and Persiano, G. (1988). Non-

interactive zero-knowledge proof systems. In Ad-

vances in Cryptology, CRYPTO87, volume 293 of

LNCS, p. 52–72. Springer.

Department of Defence (1987). DoD Personnel Security

Program. DOD 5200.2 R.

Dreier, J., Giustolisi, R., Kassem, A., Lafourcade, P., and

Lenzini, G. (2015). A Framework for Analyzing Ver-

iﬁability in Traditional and Electronic Exams. In 11th

Int. Conf. Information Security Practice and Experi-

ence, ISPEC 2015, volume 9065 of LNCS, p. 514–

529, 2015. Springer.

Dreier, J., Giustolisi, R., Kassem, A., Lafourcade, P.,

Lenzini, G., and Ryan, P. Y. A. (2014). Formal anal-

ysis of electronic exams. In 11th Int. Conf. on Se-

curity and Cryptography, SECRYPT 14, August, p.

101–112. SciTePress.

Giustolisi, R., Lenzini, G., and Ryan, P. Y. A. (2014). Re-

mark!: A Secure Protocol for Remote Exams, volume

8809 of LNCS, p. 38–48. Springer.

Hirt, M. and Sako, K. (2000). Efﬁcient receipt-free vot-

ing based on homomorphic encryption. In 19th Ann.

Conf. on the Theory and Applications of Crypto-

graphic Techniques: Advances in Cryptology (EU-

ROCRYPT’00), volume 1807 of LNCS, p. 539–556.

Springer.

Jukna, S. (2012). Boolean Function Complexity - Advances

and Frontiers, volume 27 of Algorithms and combina-

torics. Springer.

Kremer, S., Ryan, M., and Smyth, B. (2010). Elec-

tion veriﬁability in electronic voting protocols. In

15th Eur. Sym. on Research in Computer Security,

Privacy-Preserving Veriﬁability - A Case for an Electronic Exam Protocol

149

ESORICS’10, volume 6345 of LNCS, p. 389–404.

Springer.

K¨usters, R., Truderung, T., and Vogt, A. (2010). Account-

ability: deﬁnition and relationship to veriﬁability. In

17th Conf. on Computer and Communications Secu-

rity, CCS’10, p. 526–535. ACM.

M¨odersheim, S. A., Groß, T., and Vigan`o, L. (2013). Deﬁn-

ing Privacy Is Supposed to Be Easy, p. 619–635.

Springer.

Naveed, M., Kamara, S., and Wright, C. V. (2015). In-

ference Attacks on Property-Preserving Encrypted

Databases. In 22nd ACM SIGSAC Conf. on Computer

and Communications Security, CCS’15, p. 644–655,

New York, NY, USA. ACM.

Smyth, B., Ryan, M., Kremer, S., and Mounira, K. (2010).

Towards automatic analysis of election veriﬁability

properties. In Work. on Automated Reasoning for Se-

curity Protocol Analysis and Issues in the Theory of

Security, ARSPA-WITS’10, volume 6186 of LNCS,

p. 146–163. Springer.

SECRYPT 2017 - 14th International Conference on Security and Cryptography

150