Pixel Apodization for the Suppression of Higher Diffractive Orders in

Computer Holography

Joanna Starobrat and Michal Makowski

Faculty of Physics, Warsaw University of Technology, ul. Koszykowa 75, 00-662 Warsaw, Poland

Keywords:

Computer-generated Holography, Higher Diffraction Orders, LCoS Spatial Light Modulator, Pixel Apodiza-

tion.

Abstract:

Demand for a next generation of head-up displays have increased the demand for the applicable holographic

displays. From the available spatial light modulators (SLM), Liquid Crystal on Silicon (LCoS) SLMs are the

most popular for the simplicity of addressing and their relatively low costs. However, improving its efﬁciency

demands reduction of higher diffraction orders. In this work we propose a new solution, that is the pixel

apodization as the means to redirect the light intensity from the undesirable images to the main image. The

higher diffraction orders are the result of the rectangular shape of the pixels of the SLM, as it can be concluded

from the Fourier Transform (FT) analysis. Hence we exploit the known property that a Gaussian function is,

up to a constant multiple, its own FT. The presented simulations support the theoretical conclusions, as the

apodization allows for a signiﬁcant lowering of the intensity of the third and higher diffraction orders, in the

same time increasing the intensity directed to the main image.

1 INTRODUCTION

Holography as an imaging technique has been known

since the ﬁrst half of XIX century (Gabor, 1949), with

its theoretical background dating as early as 1920

(Wolfke, 1920). Being a method which allows re-

construction of not only amplitude, but also phase

of the saved image, it has soon found its application

in a wide range of ﬁelds, starting from simple three-

dimensional image reconstruction (Denisiuk, 1978),

going through beam-shaping (Meltaus et al., 2003),

i.e. in particle-trapping (Reicherter et al., 1999),

telecommunication (Parker et al., 1997) or medicine

(West, 1976), ending with a modern idea of a holo-

graphic memory of high security (Betin et al., 2013).

In the modern days the demand for a next gen-

eration of displays has been increasing. The head-

up displays are an especially pursued subject, which

is one of the main reason for further development of

applicable holographic displays. Among the advan-

tages offered by this technology, creating a real 3D

images, low intensity-to-heat conversion and uncom-

plicated set-up of few elements can be named (Slinger

et al., 2005).

Though many different types of spatial light mod-

ulators (SLM) are used in order to change the ampli-

tude and/or phase of the wavefront (Casasent, 1977),

the liquid crystal on silicon (LCoS) are considered

the most popular due to their diffraction efﬁciency,

simplicity of pixel addressing and relatively low costs

(Michakiewicz and Kujawiska, 2009). The LCoS

SLMs technology is still, however, facing challenges

that remain to be overcome. While the pixel size is

limited by the production machinery and the crosstalk

between single liquid crystal cells occurs (Yang et al.,

2013), another issue has been chosen as the main sub-

ject of our research, and that is the presence of im-

ages in higher diffractive orders (Makowski et al.,

2012; Yaras et al., 2010). The state of art technol-

ogy employs spatial ﬁltering as the method of re-

ducing the inﬂuence of the undesired images (Agour

et al., 2009). This approach allows to eliminate higher

diffraction orders, however, it largely decreases the

intensity of the ﬁnal wavefront while also present-

ing difﬁculties with successful ﬁltering of the highest

diffraction orders.

In this paper we conclude that the higher diffrac-

tion orders visible in a reconstruction of computer-

generated Fourier hologram are the result of the rect-

angular shape of the pixels. This judgement is based

on the analysis of both theory of the reconstruction of

the said type of a hologram, and known Fourier trans-

forms. Rectus function subjected to the FT results

in a sinc function (Kreyszig, 1988), characterised by

160

Starobrat, J. and Makowski, M.

Pixel Apodization for the Suppression of Higher Diffractive Orders in Computer Holography.

DOI: 10.5220/0006551901600165

In Proceedings of the 6th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2018), pages 160-165

ISBN: 978-989-758-286-8

multiple extrema in the spectrum. Thus, we propose

the effective change of the shape of pixels. As it is

known, a Gaussian function is, up to a constant multi-

ple, its own Fourier transform. We propose a change

of the pixel shape from rectus to a Gaussian function

in order to avoid the multiple maxima of the inten-

sity visible in the image reconstruction. Seeing as the

LCoS SLM production is limited by the LCD tech-

nology capabilities, changing not physical, but effec-

tive shape of the single SLM cells would be advis-

able. In our research we employ amplitude masks as

the means of altering the SLM effective structure.

2 PROPOSED METHOD

The high time-consumption and cost of employing

the suggested solution in an experiment led to a de-

cision of preparing and analysing simulations before

conducting the experiment itself. In the next sec-

tions the assumptions made for the theoretical model

of the hologram reconstruction are explained. In this

research, LightSword software was used, which en-

ables the simulation and numeric analysis of wave-

fronts propagation.

2.1 Simulated Set-up

A very simple set-up was chosen for the simulation

of image reconstruction from Fourier holograms, as

can be seen in Figure 1. He-Ne laser (λ = 632.8 nm)

serves as a light source, while a half-plate is used to

set proper polarisation in respect to the SLM. The lens

enables the reconstruction of the image in its focal

plane after wavefront reﬂects from the spatial light

modulator. Thanks to the beamsplitter, observing the

resulting intensity pattern to the side from the optical

axis of the set-up is possible.

Figure 1: A schematic diagram of a simulated experimental

set-up, where: L - He-Ne laser, λ/2 - half-wave plate, Ls -

lens, BS - beamsplitter, SLM - spatial light modulator, OP -

observation plane positioned in the focal distance from the

lens.

2.2 SLM Structure

In the simulations, an SLM of HoloEye Pluto type

was imitated as an amplitude distribution presented

in a numeric array. Said model is characterized by

the pixel size of 8 µm and the ﬁll factor estimated as

93%, which implies the presence of spacing between

single pixels. It is caused by fabrication limitations

and needs to be considered in the theoretical model.

Despite the resolution of 1920 x 1080 pixels of the

SLM, only a matrix of 128 by 128 pixels was con-

sidered to simplify the simulations. To observe the

effects of the apodization, oversampling was applied.

This means that a square of 16 by 16 sampling points

corresponded to a single SLM pixel and the ﬁnal array

size was 2048 by 2048. Such structure was approxi-

mated as a rectangular lattice consisting of squares

with 7 µm sides and 1 µm spacing between them. A vi-

sualised fragment of a created structure is presented in

the Figure 2.

Figure 2: Fragments of the simulated structures. Top: am-

plitude distribution of the SLM pixel structure. Bottom:

amplitude distribution of the Gaussian mask.

2.3 Masks Generation

As mentioned in the previous sections, a Gaussian

shape of the pixels was proposed as a solution to the

intensity leakage to the higher diffraction orders. The

change of the effective pixel shape implies that each

pixel needs to be apodized with a required function.

For that reason, a lattice of desired elements had to

be generated. In this case, the Gaussian functions of

Pixel Apodization for the Suppression of Higher Diffractive Orders in Computer Holography

161

a set radius were applied. A range of different radii

values were considered, spanning from w

= 4 µm,

through w

= 3.5 µm and w

= 3 µm, to w

= 2.5 µm.

Like in the case of the SLM structure, oversampling

was applied and the same size of an array was used

(2048 x 2048).

Bottom part of Figure 2 shows a fragment of the

amplitude mask structure of w

= 2.5 µm. Notably,

centres of each square pixel and each Gaussian func-

tion were aligned for correct apodization.

The postulated Gaussian shape of the pixels might

not be the optimal solution. Not only is it possi-

ble for another, more efﬁcient mask to exist, but the

difﬁculty of practical application is also an inﬂuen-

tial factor. For that reason a sinusoidal amplitude

mask was simulated and analysed alongside the Gaus-

sian masks. The period of the sinusoid function was

matched to the pixel period of the SLM structure.

Figure 3: Computer-generated Fourier hologram. Top: sim-

ulated image. Bottom: fragment of the phase distribution of

the hologram.

2.4 Fourier Hologram

Fourier hologram is a unique type of a hologram be-

cause of it saving the Fourier spectrum of the recorded

image. The image can be reconstructed, as in the

set-up shown on Figure 1, by a convergent spherical

wave. In a computer-generated hologram, a Fourier

transform of the simulated wavefront is calculated.

Then, it is presented in an array of values correspond-

ing to the phase change that is necessary to create

a desired distribution.

A simulation utilizing a simple image of a small

circle (Figure 3) was chosen to be presented in this pa-

per due to the simplicity of obtained results and their

viable analysis. A hologram of a size 128 by 128 pix-

els was generated, and only then it was rescaled to

match the matrices prepared in the previous sections,

that is to the size of 2048 x 2048. This allowed a uni-

form distribution of phase in the areas corresponding

to single SLM pixels, which were set to have a side of

16 pixels.

3 SIMULATIONS

In order to conduct the simulations, all elements

whose generation was described in the previous sec-

tion must be combined. The SLM structure was ﬁrst

merged with the Fourier hologram phase distribution,

and then mathematically multiplied by the amplitude

mask as to simulate the apodization. While all the

generated masks were applied, also a case of un-

apodized spatial light modulator was studied as the

reference for further results. It should be noted that

the zeroth diffraction order, present in the experimen-

tal hologram reconstruction, was not simulated, as the

crosstalk effect between the pixels was omitted in the

theoretical model.

The obtained wavefront amplitude distributions

were analysed by two different methods. First of them

was based on the visual presentation of the simula-

tion results. Even though the differences between the

obtained images were noticeable with bare eyes, the

cross-sections of the distributions were calculated for

improved assessment of the effectiveness of apodiza-

tion. Figure 4 presents the examples of the acquired

images and the cross-sections for the cases of no

apodization, as well as Gaussian masks of w

= 4 µm

and w

= 2.5 µm.

The visual judgement is known to be insufﬁcient

as the means of theory evaluation, therefore the nu-

merical results were also analysed and are demon-

strated in the Tables 1 and 2. The values were ob-

tained by the integration of the selected areas of

ﬁrst, second, as well as joint third and fourth or-

ders of diffraction. The results shown in the Table

1 present the percentage of the amplitude distribution

in each examined case separately, while in Table 2

normalized percentage distribution of amplitude can

be found. The values given in the tables do not total

up to 100%, as as the diffractive orders higher than

fourth were not included.

PHOTOPTICS 2018 - 6th International Conference on Photonics, Optics and Laser Technology

162

Figure 4: Amplitude of the image reconstructions from simulated holograms and the cross-sections of their distributions.

Left: image obtained with an un-apodized spatial light modulator. Center: image obtained with the use of a ﬁrst examined

Gaussian mask, w

= 4 µm. Right: image obtained with the use of a last examined Gaussian mask, w

= 2.5 µm.

4 DISCUSSION

The visual results are rather simple to analyse, as the

difference in hologram reconstructions is rather no-

ticeable. Both Gaussian masks, including the one of

the highest Gaussian diameter, redirects light into the

main image, which corresponds to the ﬁrst diffrac-

tion order. Said effect is more visible in the case of

the smaller Gaussian diameter, w

. On the presented

cross-sections the relative lowering of the amplitude

of the third and higher diffraction orders can easily be

observed.

The numeric values of the area integrals allow for

a more precise comparison. Even at a ﬁrst glance,

a trend among the Gaussian masks is rather visible,

with monotonous decrease of the amplitude of third

and fourth diffraction orders, and increase of the ﬁrst

diffraction order, that is, the amplitude of the main

image. The notable increase in the second order of

diffraction is, however, undesired. Yet, it could be re-

duced by the means of spatial ﬁltering - combining the

state of art technology with the method suggested in

this paper could result in the increase of the intensity

of the main image.

It is therefore crucial to analyse the normalized

percentage distribution of the amplitude in respective

diffraction orders, as presented in the Table 2. As

expected, the trend of decreasing amplitude integrals

in the area of the ﬁrst diffraction order is noticeable.

Despite redirecting more percentage of light into the

main image, the large part of the wavefront is blocked

due to applying the amplitude mask. The decrease

in the ﬁrst order is as big as 41.5 percentage points

(pp) between the un-apodized spatial light modulator

and the Gaussian mask of the smallest diameter, w

However, for the third and fourth diffraction order the

reduction is much more signiﬁcant, coming close to

95 percentage points. Again, in the second order of

diffraction an undesired rising tendency is observed,

with the amplitude increasing even despite the light

suppression by the amplitude mask.

It is worth noting that the numeric results for the

sinusoidal mask are rather similar to those obtained

with a Gaussian mask of w

= 2.5 µm. In the case of

amplitude percentage in the respective diffraction or-

ders, the value of the third and fourth orders is slightly

lower (by around 3 pp) and the main image is also

somewhat stronger (0.5 pp increase of the amplitude

value). The normalized amplitude show a decrease in

both of these areas (both by around 4-5 pp) in com-

parison to the Gaussian mask of w

. While the two

analysed data sets prove the rise of the amplitude in

the second diffraction order, the simplicity of applica-

tion of this mask, as explained in the next section, is

an advantage enough to consider the results satisfac-

tory.

Despite the seemingly undesired effect on the sec-

ond order of diffraction, which is as well the closest to

the main image and therefore can strongly inﬂuence

the quality of the image reconstruction from Fourier

hologram, the apodization simulation has proven the

possibility of changing the effective pixel shape. As

Pixel Apodization for the Suppression of Higher Diffractive Orders in Computer Holography

163

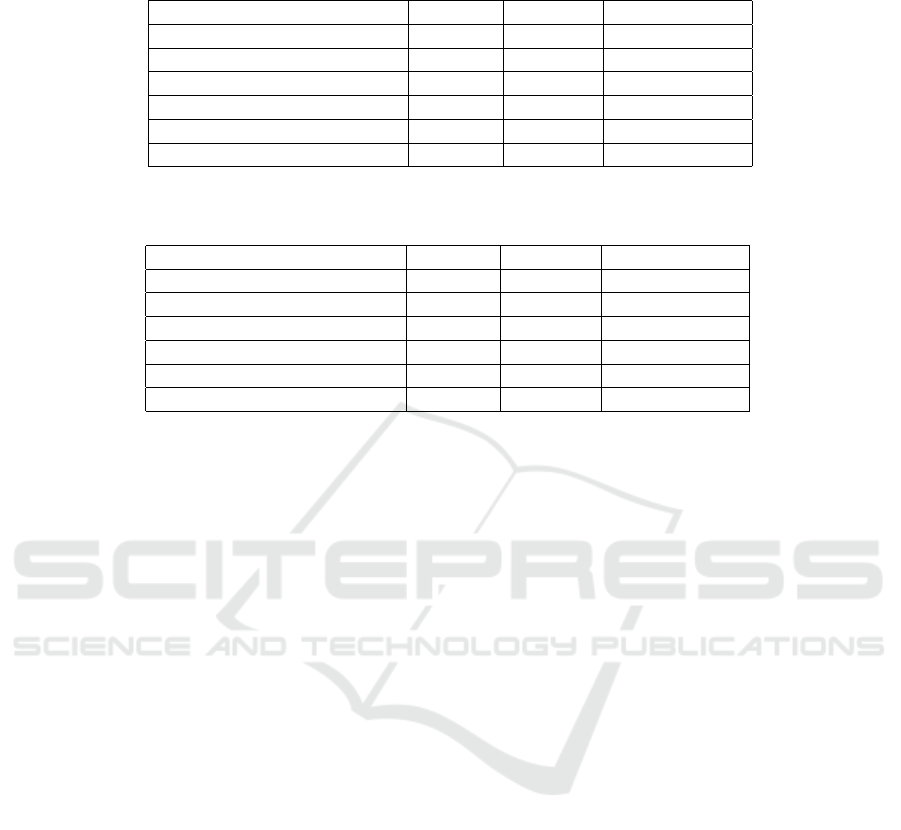
Table 1: Percentage of amplitude distribution in respective diffraction orders for different apodizing masks - result of the

simulations.

Mask shape 1st order 2nd order 3rd & 4th order

No apodization 17,74% 15,76% 27,31%

Gaussian mask (w

= 4 µm) 19,29% 22,08% 24,59%

Gaussian mask (w

= 3,5 µm) 20,36% 28,30% 21,79%

Gaussian mask (w

= 3 µm) 21,64% 39,26% 16,52%

Gaussian mask (w

= 2,5 µm) 22,48% 55,51% 8,58%

Sinusoidal mask 22,99% 69,97% 5,34%

Table 2: Normalized percentage of the amplitude distribution in respective diffraction orders for different apodizing masks -

result of the simulations.

Mask shape 1st order 2nd order 3rd & 4th order

No apodization 100% 100% 100%

Gaussian mask (w

= 4 µm) 82,92% 106,84% 68,64%

Gaussian mask (w

= 3,5 µm) 66,19% 103,60% 46,03%

Gaussian mask (w

= 3 µm) 51,60% 105,37% 25,59%

Gaussian mask (w

= 2,5 µm) 38,50% 107,03% 9,54%

Sinusoidal mask 33,38% 114,40% 5,04%

predicted, the amplitude masks allowed a change of

the distribution of the reconstructed images and while

the results obtained are not yet ideal for the proposed

purpose, the conducted research creates the possibil-

ity of optimising the masks parameters. Additionally,

displaying the image in between the intensity peaks

of main diffraction orders with the use of a carrier-

frequency would have the advantage of increasing re-

construction efﬁciency with the observed gain of in-

tensity in both ﬁrst and second order of diffraction.

5 EXPERIMENT

The simulations are the ﬁrst step of conﬁrming the

postulated hypothesis and because of the limita-

tions of theoretical models, experimental proof is

favourable. Especially the sinusoidal mask presents

the greatest opportunity of experimental application

due to its structure. In this paper we name a few of

the possible application approaches.

The simplest of them would be mask fabrication

and its positioning by the SLM surface in the set-up

analogous to the one presented in Figure 1. It should

be noted, however, that in such case the wavefront

propagates twice through the amplitude mask. The

shape of the mask should be then corrected. Using the

Talbot self-imaging length could eliminate said obsta-

cle, with the periodic structure reconstruction, up to

a constant multiple, at a distance. The mask itself can

be created precisely by the means of electrolitogra-

phy or, in a simplistic case, by recording a hologram

of an interference pattern of two plane waves, creat-

ing a sinusoidal structure in one of the dimensions.

In the latter instance, care should be taken to accu-

rately apply the correct angle between the waves. An-

other possible approach to the spatial light modulator

apodization is a direct interference of two waves on

the SLM plane.

6 SUMMARY

In this work, a solution to the intensity leakage in

the image reconstruction of the computer-generated

Fourier hologram was proposed. The higher diffrac-

tion orders are one of the reasons for the intensity de-

crease of the main image, thus we proposed the ef-

fective change of the pixel shape to reduce this effect.

The apodizing structures described in this paper, that

is Gaussian and sinusoidal masks, are not necessarily

optimal for this purpose, however, intensity redirec-

tion to lower diffraction orders was still observed. It

is a common practice to display the image off-axis

with the use of holographic diffraction gratings, thus

the ﬁnal projection can beneﬁt from the obtained in-

tensity raise of both ﬁrst and second orders.

Conducted simulations support the hypothesis that

it is possible and relatively uncomplicated to alter the

obtained amplitude distribution by the use of the am-

plitude mask, and control the change by modifying

parameters of the mask. In order to analyse both

masks of different shapes and experimental results for

the simulated data, further study of the subject is nec-

essary.

PHOTOPTICS 2018 - 6th International Conference on Photonics, Optics and Laser Technology

164

REFERENCES

Agour, M. et al. (2009). Suppression of higher diffraction

orders and intensity improvement of optically recon-

structed holograms from a spatial light modulator. In

Journal of Optics A: Pure and Applied Optics, 11(10),

105405, 120-128.

Betin, A. Y. et al. (2013). Holographic memory optical sys-

tem based on computer-generated fourier holograms.

In Applied Optics 52, 8142-8145.

Casasent, D. (1977). Spatial light modulators. In Proceed-

ings of the IEEE, 65(1), 143-157.

Denisiuk, I. N. (1978). Holography. In Optiko Mekhanich-

eskaia Promyshlennost, 45, 9-13.

Gabor, D. (1949). Microscopy by reconstructed wave-

fronts. In Proceedings of the Royal Society of London

A: Mathematical, Physical and Engineering Sciences,

Vol. 197, No. 1051, pp. 454-487. The Royal Society.

Kreyszig, E. (1988). Engineering mathematics. Wiley, 10th

edition.

Makowski, M. et al. (2012). Simple holographic projection

in color. In Optics express, 20(22), 25130-25136.

Meltaus, J. et al. (2003). Millimeter-wave beam shaping

using holograms. In IEEE Transactions on Microwave

Theory and Techniques, 51(4), 1274-1280.

Michakiewicz, A. and Kujawiska, M. (2009). Opto-

electronic reconstruction of digital holograms. In

Elektronika: konstrukcje, technologie, zastosowania,

50(1), 120-128.

Parker, M. C., Cohen, A. D., and Mears, R. J. (1997). Dy-

namic holographic spectral equalization for wdm. In

IEEE Photonics Technology Letters, 9(4), 529-531.

Reicherter, M. et al. (1999). Optical particle trapping with

computer-generated holograms written on a liquid-

crystal display. In Optics Letters, 24, 608-610.

Slinger, C., Cameron, C., and Stanley, M. (2005).

Computer-generated holography as a generic display

technology. In Computer, 38(8), 46-53.

West, P. A. (1976). Holography in medicine. In Journal of

Modern Optics, 23(10), 845-845.

Wolfke, M. (1920). Uber die moglichkeit der optis-

chen abbildung von molekulargittern. In Physikische

Zeitschrft, 21, 495-7.

Yang, H. et al. (2013). Origin of transient crosstalk and

its reduction in phase-only lcos wavelength selective

switches. In Journal of Lightwave Technology, 31(23),

3822-3829.

Yaras, F., Kang, H., and Onural, L. (2010). State of the art in

holographic displays: a survey. In Journal of display

technology 6(10), 443-454.

Pixel Apodization for the Suppression of Higher Diffractive Orders in Computer Holography

165