
Nonlinear Trapping and Interfering Modes
in a Quasi-One-Dimensional Microcavity Laser
Maciej Pieczarka
1,*
, Christian Schneider
2
, Sven Höfling
2,3
and Grzegorz Sęk
1
1
Laboratory for Optical Spectroscopy of Nanostructures, Department of Experimental Physics, Faculty of Fundamental
Problems of Technology, Wrocław University of Science and Technology, W. Wyspianskiego 27, 50-370 Wrocław, Poland
2
Technische Physik, Physikalisches Institut and Wilhelm Conrad Röntgen-Research Center for Complex Material Systems,
Universität Würzburg, Am Hubland, D-97074 Würzburg, Germany
3
SUPA, School of Physics and Astronomy, University of St. Andrews, St. Andrews, KY 16 9SS, U.K.
Keywords: Nonlinear Optics, Microcavities, VCSEL, Exciton-polaritons.
Abstract: Experimental studies of the emission from one-dimensional microcavity laser structure under nonresonant
optical excitation are presented. The one-dimensional laser was prepared by electron-beam lithography and
reactive ion etching from a planar microcavity sample. Below the lasing threshold, the system was in the
strong coupling regime, where the emission exhibits the common exciton-polariton far-field dispersion.
Above the threshold, the system switched to the weak coupling regime and the photon lasing was observed.
Interestingly, under higher pumping powers above the threshold, a strong blueshift of the lasing mode was
observed, with localisation of the far-field emission at finite wavevectors. The near-field images showed
interference fringes corresponding to the interference of propagating modes in k-space. This is interpreted in
terms of self-interfering modes confined between the pumping spot and the edges of the 1D microlaser.
1 INTRODUCTION
Light confinement in the sub-micrometre scale has
led to the realisation of novel nonlinear sources of
coherent light and paved the way for studies of
quantum electrodynamics in the semiconductor
material platform (Reithmaier et al. 2004). Since
reaching the strong coupling between the exciton and
photon (quasiparticles called exciton-polaritons) in
planar semiconductor cavities (Weisbuch et al. 1992),
there is a vast interest in nonlinear phenomena in
quantum bosonic fluids of light and matter realized in
a solid state system (Byrnes et al. 2014; Carusotto &
Ciuti 2013).
Due to the excellent development of etching
techniques in semiconductor technology, there is a
possibility to harness the photonic confinement into
any desired pattern. On the other hand, spatial
shaping of the nonresonant optical excitation can
create an effective potential for exciton-polaritons
(Schneider et al. 2017) or create nonlinear coupled
structures in photon lasers (Pal et al. 2017). In view
of the abovementioned approaches, one can combine
the etching techniques with strong nonresonant
excitation to provide custom photonic confinement
for the lasing modes.
In this paper, we present investigations of a one-
dimensional microcavity laser with nonresonant
optical excitation. Under strong pumping, the system
reaches the weak coupling regime and photon lasing
is observed at finite wavevectors in the far-field
spectrum. The emission is strongly blueshifted with
respect to the bare cavity mode resonance at low
pumping power densities. Power dependent
measurements in near and far field spectra give
insight into the observed phenomena, which are
interpreted in terms of nonlinear confinement of the
modes outside the pump region.
2 EXPERIMENTAL DETAILS
2.1 Optical Setup
The experiments were performed on the setup
depicted in Fig. 1. Excitation was provided with the
mode-locked femtosecond pulsed Ti: Sapphire laser
(parameters are indicated in the figure), tuned around
242
Pieczarka, M., Schneider, C., Höfling, S. and S˛ek, G.
Nonlinear Trapping and Interfering Modes in a Quasi-One-Dimensional Microcavity Laser.
DOI: 10.5220/0006637302420246
In Proceedings of the 6th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2018), pages 242-246
ISBN: 978-989-758-286-8
Copyright © 2018 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved
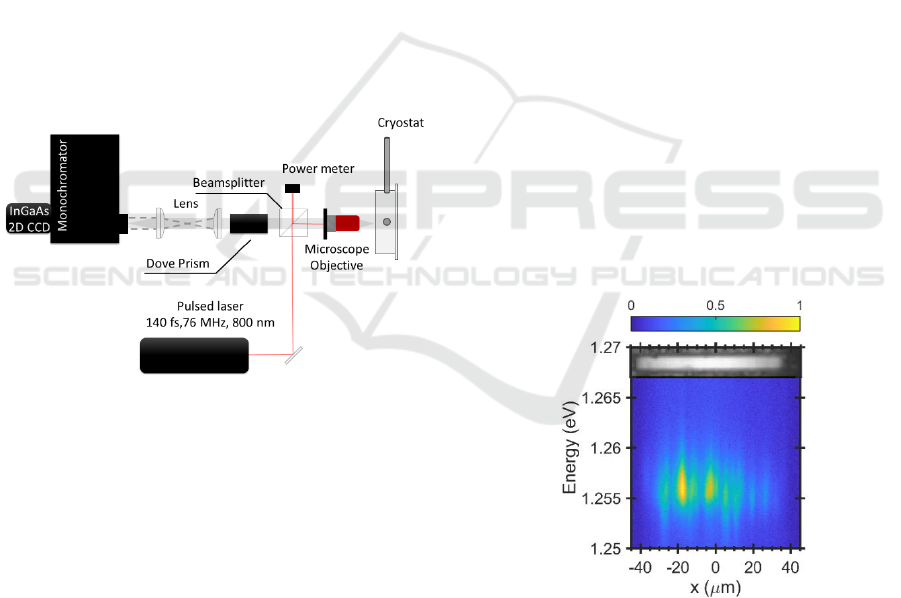
800 nm for efficient absorption in the GaAs material.
The laser beam was focused with a high numerical
aperture microscope objective (NA = 0.42) to a
diffraction limited Gaussian spot of diameter around
3 µm on the sample. The laser power is controlled
with the optical power meter placed after the beam
splitter. The investigated sample was kept in the
continuous-flow liquid-helium cryostat in order to
cool it down to the cryogenic temperature of 5 K.
Luminescence from the sample is collected with
the same objective and imaged with a set of
achromatic lenses onto the entrance slit of a
monochromator. A two-lens setup is used to
efficiently switch between near-field and far-field
images (one of the lenses is placed on a kinematic
mount). Cuts of the sample emission images are
analysed with the half-meter focal-length
monochromator coupled to a 2D InGaAs-based near-
infrared CCD camera. To conveniently adjust the
direction of the cuts done by the monochromator slit
a Dove prism on a rotational mount is placed in the
optical path, which enables rotation of the image with
respect to the detection system.
Figure 1: Scheme of the experimental setup.
2.2 Sample Details
We investigated a microcavity sample, which was
used in the previous work (Pieczarka et al. 2017). The
planar microcavity is grown in molecular beam
epitaxy technique (MBE) on a semi-insulation GaAs
substrate. It consists of two GaAs/AlGaAs distributed
Bragg reflectors (16 bottom and 12 top layer pairs
respectively), where the active region consists of two
stacks of four In
0.28
Ga
0.72
As 7 nm thick quantum wells
(QWs) placed at the antinodes of the confined
fundamental photon mode (in the growth direction).
The sample is characterized by the normal mode
splitting of the exciton and photon modes, with Rabi
splitting of 7.5 meV, where the theoretical exciton
resonance appears around 1.2605 eV. The cavity
mode is characterized by a quality factor exceeding
1000 (measured spectrally far from the exciton-
polariton resonance).
To obtain high-quality one-dimensional micro-
wires, a piece of the sample was etched in the post-
processing. Microwires of the length of tens to
hundreds of micrometres and widths of few micro-
metres were created via electron beam lithography
and etched deeply into the structure using electron-
cyclotron-resonance reactive-ion-etching. Due to the
optimized etching technique resulting in smooth and
steep sidewalls of extremely low roughness, no
detrimental influence on the cavity quality factor was
observed (Fischer et al. 2014). In this work, we focus
on a microwire of 75 µm length and width of 8 µm,
which is presented in the inset of Fig. 2.
The planar sample was characterized previously
with the high impact of local fluctuations of photonic
and excitonic disorder, which influences the exciton-
polariton fluid flow in the strong coupling regime
(Pieczarka et al. 2015). To check the level of disorder
in the investigated microwire, we excited the sample
with a defocused laser spot (with an additional lens in
the laser path) with low power density, very far from
the lasing threshold. Photoluminescence (PL) along
the wire was cut with the monochromator slit and
analysed spectrally. One can see in Fig. 2 that the PL
is fragmented, as the low-density polaritons are
confined in the local potential minima. However, the
amplitude of the local energy fluctuations is around
1 meV only, being much smaller from the disorder in
the planar sample observed previously.
Figure 2: Emission spectrum at wide spot excitation below
lasing threshold along the investigated microwire. Top view
white-light microscope image of the investigated
microlaser is in the top inset.
3 RESULTS
Excitation power dependent measurements of the
Nonlinear Trapping and Interfering Modes in a Quasi-One-Dimensional Microcavity Laser
243

polariton and photon luminescence from the
microwire were performed in the wide range of pump
powers. Results of the data analysis are presented in
Fig. 3. In the integrated intensity power curve in Fig.
3a, one can observe a distinct nonlinear threshold
(P
th
= 13 mW) with a simultaneous drop in the
linewidth and blueshift of the emission resonance,
Figs. 3b and 3c. This is an indication of the transition
to a coherent lasing state, as the spectrally narrow
lasing resonance is located around the bare cavity
mode, Fig. 3c.
Moreover, in the range of low pumping power
density below the threshold, the emission is located at
energies lower than the bare cavity mode, Fig. 3b and
the far-field dispersion corresponds to the lower
exciton-polariton branch (LPB), see Fig. 4a. The
emission is fragmented, which indicates the influence
of disorder, as presented in Fig. 2. At lasing threshold,
the emission is located around the bare cavity mode,
Fig. 4b, although the far-field dispersion is rather flat,
indicating a localised mode in real space, perhaps at a
local defect near the pump region. The observation
of the intensity dependence threshold, linewidth
narrowing and blueshift of the emission from LBP
to the cavity mode resonance are signatures of the
Figure 3: Power dependent analysis. (a) Integrated
intensity, (b) linewidth of the emission and (c) energy of the
lasing resonance.
Figure 4: Normalised far-field emission spectra below (a)
and slightly above the lasing threshold (b). LPB is depicted
(solid line) together with bare cavity photon dispersion
(dashed line) and QW exciton resonance (dotted line).
transition from strong to weak coupling regime and
occurrence of the photon lasing (Tempel et al. 2012).
The most important observation has been made at
pumping levels exceeding the threshold power. The
emission energy shows a continuous blueshift with
the pumping power up to P = 6P
th
, see Fig. 3c. This
kind of spectral behaviour is expected rather for a
polariton laser than for weak coupling photon lasing
(Bajoni et al. 2008). The far-field characteristics in
this power range show a flat and blueshifted
dispersion around the theoretical cavity mode, Fig.
5a. Further increase of the pumping level leads to the
pinning of the emission energy around the bare QW
exciton energy at 1.261 eV, Fig. 3. indicates the
maximum spectral gain at this energy, which
amplifies the lasing mode. Nevertheless, the far-field
dispersion showed a very distinct change, where
lasing at a well-defined wavevector is observed, Fig.
5b. This is a result of two counter-propagating
coherent wave packets along the microwire.
Figure 5: Normalised far-field emission spectra at higher
pumping powers. Flat and blueshifted dispersion (a) and
well-defined finite wavevector lasing (b).
PHOTOPTICS 2018 - 6th International Conference on Photonics, Optics and Laser Technology
244
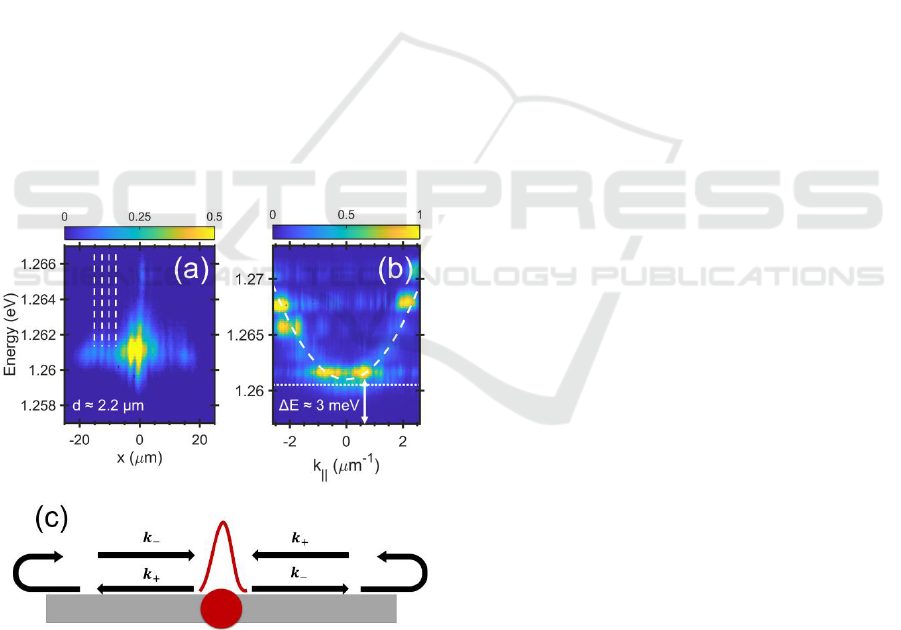
In order to verify this observation, we performed a
near-field imaging along the one-dimensional
microcavity at the highest pumping level. The result
is shown in Fig 6a. At the pump spot position
(x = 0 µm) the strongest emission is observed with
signatures of the occupation of higher energy
confined modes in the perpendicular direction, see
Fig. 6b. Moreover, the spatially extended emission is
detected, being spread around 20 µm in both
directions from the pump spot along the microwire at
the main lasing energy, Fig. 6a. This feature is
characterised by distinct interference fringes at both
sides of the near-field image. Interestingly, the fringe
spacing is equidistant and is equal to d = 2.2 µm. This
spacing corresponds almost exactly to the
interference of wave-packets travelling with the
emission wavevector observed in the far-field
luminescence k = π/d ≈ 1.43 µm
-1
. It is worth noting
that the equidistant spacing cannot be caused by the
irregular defect pattern, see Fig. 2.
The observed features can be interpreted in terms
of self-interference of coherent photon waves
travelling along the one-dimensional laser cavity.
Photons are emitted locally within the pump spot and
propagate in positive and negative directions in the
wire. Further, they are reflected from the cavity ends,
providing the counter propagating mode. This mode
Figure 6: Normalised near-field emission spectra at high
pumping power P = 23P
th
(a) (dashed lines indicate
equidistant interference maxima). The colour scale is
saturated at 0.5 to enhance the visibility of fringes. Far-field
spectrum in the perpendicular direction to the microwire at
the pumping spot (b). (c) Schematic picture of the
interpretation of the described phenomena. The red dot
indicates the pump spot, local blueshift as a red peak and
black arrows indicate travelling waves along the wire.
can be once again enhanced with the remnant gain in
the pump region. This mechanism provides
amplification of the propagating waves, similarly to
one-dimensional polariton condensates (Wertz et al.
2012). The scheme of the proposed interpretation is
presented in Fig. 6c.
Propagation outside of the pump spot is possible
by a local change in the refractive index due to the
excess carriers generated in the GaAs material (Henry
et al. 1981), which creates an effective potential
gradient. This change of the refractive index is
evidenced in the far field spectrum measured at the
pump spot in the direction perpendicular to the wire,
see fig. 6b. One can observe a series of confined
transversal modes following the strongly blueshifted
cavity dispersion curve. The local blueshift at k = 0 is
as large as 3 meV and is definitely not caused by any
strong coupling phenomena, like polariton-polariton
nonlinear interactions. The observed blueshift of the
emission occurs in the weak coupling regime, so it is
solely caused by the nonlinear refractive index
change in the microcavity. This effective potential
hill provides an additional spatial confinement of the
modes propagating in both directions between the
spot and the edges of the microwire.
4 CONCLUSIONS
To conclude, we investigated lasing properties of a
quasi-one-dimensional microcavity laser. We
observed continuous blueshift of the emission with
the increase of the pumping power below and above
the photon lasing threshold, although the system
entered the weak coupling regime. Well defined,
oblique angle lasing was observed, which is described
as self-interference of confined modes between the
blueshifted pump spot and the microwire edges.
Further investigation will be conducted to verify the
proposed interpretation, especially time-resolved
measurements of the near-field and far-field patterns,
which can give insight into the dynamics of the
observed propagating laser modes and its
amplification.
ACKNOWLEDGEMENTS
Authors would like to acknowledge useful
discussions with Elena Ostrovskaya. This work is
supported by National Science Centre, grant
PRELUDIUM 2016/23/N/ST3/01350.
Nonlinear Trapping and Interfering Modes in a Quasi-One-Dimensional Microcavity Laser
245

REFERENCES
Bajoni, D. et al., 2008. Polariton Laser Using Single
Micropillar GaAs − GaAlAs Semiconductor Cavities.
Physical Review Letters, 100(4), p.47401.
Byrnes, T., Kim, N. Y. & Yamamoto, Y., 2014. Exciton-
polariton condensates. Nature Physics, 10(11), pp.803–
813.
Carusotto, I. & Ciuti, C., 2013. Quantum fluids of light.
Reviews of Modern Physics, 85(1), pp.299–366.
Fischer, J. et al., 2014. Spatial Coherence Properties of
One-Dimensional Exciton-Polariton Condensates.
Physical Review Letters, 113(20), p.203902.
Henry, C. H., Logan, R. A. & Bertness, K. A., 1981.
Spectral dependence of the change in refractive index
due to carrier injection in GaAs lasers. Journal of
Applied Physics, 52(7), pp.4457–4461.
Pal, V. et al., 2017. Observing Dissipative Topological
Defects with Coupled Lasers. Physical Review Letters,
119(1), p.13902.
Pieczarka, M. et al., 2015. Ghost Branch
Photoluminescence from a Polariton Fluid under
Nonresonant Excitation. Physical Review Letters,
115(18), p.186401.
Pieczarka, M. et al., 2017. Relaxation Oscillations and
Ultrafast Emission Pulses in a Disordered Expanding
Polariton Condensate. Scientific Reports, 7(1), p.7094.
Reithmaier, J.P. et al., 2004. Strong coupling in a single
quantum dot-semiconductor microcavity system.
Nature, 432(7014), pp.197–200.
Schneider, C. et al., 2017. Exciton-polariton trapping and
potential landscape engineering. Reports on Progress in
Physics, 80(1), p.16503.
Tempel, J. S. et al., 2012. Characterization of two-threshold
behavior of the emission from a GaAs microcavity.
Physical Review B, 85(7), p.75318.
Weisbuch, C. et al., 1992. Observation of the coupled
exciton-photon mode splitting in a semiconductor
quantum microcavity. Physical Review Letters, 69(23),
pp.3314–3317.
Wertz, E. et al., 2012. Propagation and Amplification
Dynamics of 1D Polariton Condensates. Physical
Review Letters, 109(21), p.216404.
PHOTOPTICS 2018 - 6th International Conference on Photonics, Optics and Laser Technology
246
