Physical Layer Impairments in Cascaded Multi-degree CDC

ROADMs with NRZ and Nyquist Pulse Shaped Signals

Diogo G. Sequeira

, Luís G. Cancela

1,2

and João L. Rebola

1,2

Optical Communications and Photonics Group, Instituto de Telecomunicações, Lisbon, Portugal

Department of Information Science and Technology, Instituto Universitário de Lisboa (ISCTE-IUL), Lisbon, Portugal

Keywords: ASE Noise, Broadcast and Select, In-Band Crosstalk, Nyquist Pulse, Optical Filtering, ROADMs, Route

and Select.

Abstract: Nowadays, reconfigurable optical add/drop multiplexers (ROADMs) are mainly based on broadcast and

select (B&S) and route and select (R&S) architectures. Moreover, the most used components to implement

the colorless, directionless and contentionless (CDC) ROADM add/drop structures are the multicast

switches (MCSs) and the wavelength selective switches (WSSs). In-band crosstalk, amplified spontaneous

emission (ASE) noise accumulation and optical filtering are physical layer impairments (PLIs) that become

more enhanced in a CDC ROADM cascade. In this work, we investigate the impact of these PLIs in a

cascade of CDC ROADMs based on both B&S and R&S architectures, with MCSs and WSSs-based

add/drop structures and for nonreturn-to-zero (NRZ) rectangular and Nyquist pulse shaped signals. We

show that the optical filtering impairment is more limiting for a R&S architecture. We also show that the

ASE noise accumulation after 32 cascaded ROADMs leads to a 10 dB optical signal-to-noise ratio (OSNR)

penalty for all ROADM degrees investigated. We have also concluded that the in-band crosstalk leads to a 1

dB OSNR penalty, after 13 and 24 cascaded 16-degree CDC ROADMs based on B&S for, respectively,

NRZ rectangular and Nyquist pulse shapes. For a R&S architecture, the in-band crosstalk is not so harmful.

1 INTRODUCTION

The continuous and exponential increase of data

traffic in recent years has been putting the optical

network infrastructures in a constant pursuit of new

technologies that can transport huge amounts of bits

in a more cost effective and efficient way.

Technologies, such as coherent detection, advanced

digital signal processing, polarization division

multiplexing (PDM) and wavelength division

multiplexing (WDM) are now fundamental to

achieve these goals (Roberts et al., 2017).

Moreover, as the data traffic becomes more

heterogeneous in terms of bit rate and modulation

format, and the connections duration decreases, a

more dynamic, flexible and reconfigurable optical

transport network is required (Jinno, 2017). These

requirements can be provided by the optical network

nodes, currently known as reconfigurable optical

add/drop multiplexers (ROADMs) with colorless,

directionless and contentionless (CDC) add/drop

structures (Gringeri et al., 2010). The CDC ROADM

nodes can express, add and drop any WDM signal

without restrictions and contention of wavelengths

(Feuer et al., 2011).

The most used architectures to implement the

ROADM nodes are the broadcast and select (B&S)

and route and select (R&S) architectures (Simmons,

2014). The B&S is the cheapest implementation, but

has higher insertion losses and poorer isolation than

the R&S architecture. On the other hand, the R&S

architecture is the best choice in terms of isolation of

adjacent channels and has low insertion losses, but

since it is based on wavelength selective switches

(WSSs), the filtering effects are more relevant and

the cost is higher than the B&S architecture.

In a multi-degree CDC ROADM-based optical

network, the physical layer impairments (PLIs), such

as optical filtering, amplified spontaneous emission

(ASE) noise accumulation and in-band crosstalk,

limit the number of ROADM nodes that an optical

signal can pass along the network (Tibuleac and

Filer, 2010). These PLIs are cumulative along the

network and depend not only on the ROADM

architecture, e.g. B&S or R&S, but also on the

ROADM add/drop structures.

In the literature, some studies were performed to

Sequeira, D., Cancela, L. and Rebola, J.

Physical Layer Impairments in Cascaded Multi-degree CDC ROADMs with NRZ and Nyquist Pulse Shaped Signals.

DOI: 10.5220/0006861102230231

In Proceedings of the 15th International Joint Conference on e-Business and Telecommunications (ICETE 2018) - Volume 1: DCNET, ICE-B, OPTICS, SIGMAP and WINSYS, pages 223-231

ISBN: 978-989-758-319-3

223

address the impact of these PLIs on the network

performance. In (Filer and Tibuleac, 2012), the

optical filtering and in-band crosstalk impairments

due to a cascade of WSSs, have been considered, but

neglected the ROADM architectures types. In (Filer

and Tibuleac, 2014), the impact of the ROADM

architectures are considered, but the influence of the

ROADM add/drop structures has been neglected. In

(Pan and Tibuleac, 2016), the filtering and in-band

crosstalk impact were evaluated considering the

37.5 GHz flexible grid. In that study, the authors

considered a colorless add/drop structure. In (Morea

et al., 2015), the impact of filtering for both the

50 GHz fixed grid and 37.5 GHz flexible grid is

evaluated. In that study, the crosstalk impact is not

considered, as well as the contentionless ROADM

feature. In all these previous studies, the ASE noise

accumulation is not considered. Instead, the authors

considered that the ASE noise is totally loaded at the

system input (Pan and Tibuleac, 2016) or at the

system output (Morea et al., 2015).

In this work, we investigate the impact of the

optical filtering, ASE noise accumulation and

in-band crosstalk generated inside CDC ROADMs

on the network performance, through Monte-Carlo

(MC) simulation. PDM quadrature phase-shift

keying (PDM-QPSK) signals at 100-Gb/s, with

25 Gbaud symbol rate, for the 50 GHz fixed grid are

considered, although other scenarios could be

simulated (Fabrega et al., 2016). We investigate both

nonreturn-to-zero (NRZ) rectangular (Wang and

Lyubomirsky, 2010) and Nyquist pulse shaped

signals. These last signals considered a roll-off

factor (β) equal to 0.1, which is a typical value

(Morea et al., 2015). This study is performed by

properly modelling the ROADM nodes, considering

both B&S and R&S architectures and different

add/drop structures, based on multicast switches

(MCSs) (Way, 2012) and WSSs (Yang et al., 2017).

This work is organized as follows. Section 2

describes the simulation model of the multi-degree

CDC ROADM-based optical network. Details on the

ROADM add/drop structures are provided in

Subsection 2.1. In Subsection 2.2, the filtering

transfer functions used to model the ROADM

components are presented and characterized. The

optical filtering impact is studied in Section 3, for

both ROADM architectures, add/drop structures and

pulse shapes signals. Section 4 investigates the in-

band crosstalk level evolution in a CDC ROADM

cascade also for both ROADM architectures,

add/drop structures and signal shapes. In Section 5,

the impact of in-band crosstalk on the network

performance is evaluated. Finally, in Section 6, the

conclusions of this work are provided.

2 CDC ROADM-BASED OPTICAL

NETWORK MODEL

In this section, we present the simulation model of

an optical network based on multi-degree CDC

ROADMs, as well as, the in-band crosstalk terms

generated inside these ROADMs and the ASE noise

added to the primary signal along the network.

Subsection 2.1 describes the ROADM add/drop

structures modelling. Subsection 2.2 presents the

filtering transfer functions used to model the

ROADM components.

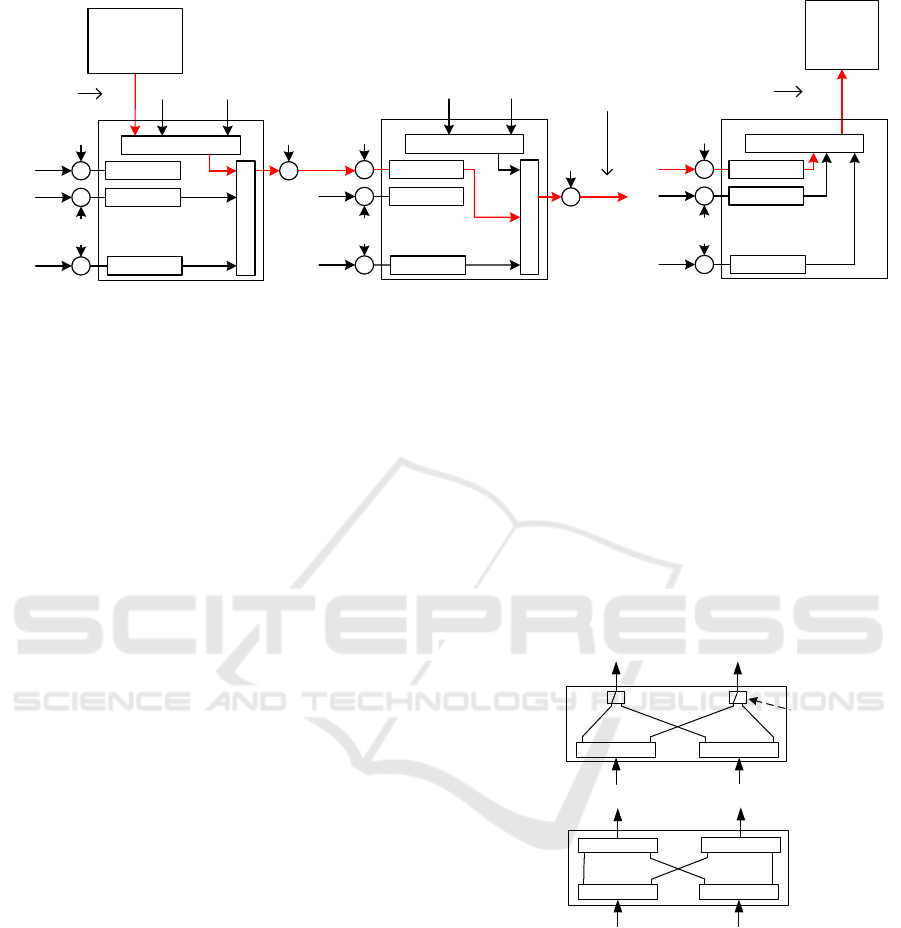
Figure 1 depicts the simulation model of an

optical network based on multi-degree CDC

ROADMs. The red line in this figure represents the

light-path of the primary signal (i.e., the signal that

is taken as a reference to study the impact of the

PLIs), 



, since it is added to the network, in the

first ROADM node, until it is dropped, in the

ROADM, 

,

. Throughout this work, we

consider a 100-Gb/s NRZ rectangular or Nyquist

pulse shaped signal and QPSK modulation for the

primary signal. In our MC simulator, we do not

consider the fiber transmission effects, so the fiber

impairments are neglected.

Regarding the in-band crosstalk signals

originated along the multi-degree CDC

ROADM-based optical network, we consider that all

interfering signals have the same modulation format

and bit rate as the primary signal, but with different

arbitrary transmitted symbols, characterized by a

phase difference and a time misalignment between

the primary signal and in-band interferers (Cancela

et al., 2016). These interfering signals arise from the

ROADM inputs and, also, from the ROADM add

structures, denominated, respectively, 

,

and



,

, with M indicating the ROADM node and R

the ROADM degree in which they are originated.

We consider that all ROADM degrees are sources of

interfering signals. In the ROADM inputs and

ROADM add structures, the interfering signals pass

through the respective components (e.g. WSS) and

then are added to the primary signal.

Concerning the ASE noise addition, we consider

that the ASE noise is added both at the ROADM

inputs and outputs. The optical amplifier (OA) at the

ROADM inputs is used to compensate the optical

path losses, whereas the OA at the ROADM outputs

is used to compensate the losses inside the ROADM

node (Zami, 2013).

OPTICS 2018 - International Conference on Optical Communication Systems

224

...

1,add2

1,add R

o,1

...

o,2

R-degree ROADM 2

R-degree ROADM

1,in1

1,in2

1,inR

ASE

noise

Add Structure

WSS

ASE

noise

...

2,add2

2,add R

2,in2

2,inR

ASE

noise

Add Structure

WSS

ASE

noise

M,inR

o,M-1

M,in2

ASE

noise

ASE

noise

o,M

ASE

noise

Drop Structure

ASE

noise

Add

signal

Express

signal

Comp. A

...

Drop

signal

...

Comp. A

Optical

Coherent

Receiver

Optical

Transmitter

Figure 1: Simulation model of an optical network based on M cascaded R-degree CDC ROADMs.

The node losses are considered independent of

the ROADM architectures. So, in the MC simulator,

we consider that all OAs have the same

characteristics: noise figure, gain and optical

bandwidth. Hence, they impose the same optical

signal-to-noise ratio (OSNR) at its outputs.

Throughout this work, the OSNRs presented

correspond to the OSNR at the output of each OA

and it is measured in the 0.1 nm reference bandwidth

(Essiambre et al., 2010). The ASE noise is

considered as an additive white Gaussian noise.

To drop the primary signal, in the last ROADM,

we use an ideal coherent detection receiver model

(Essiambre et al., 2010). In the decision circuit,

inside the optical coherent receiver, the bit error rate

(BER) is obtained by direct-error counting, for a

target BER of 10

−3

. The number of counted errors

considered is 1000 and either the primary signal and

the interfering signals are generated with 256

symbols. Our studies are done only for a single

polarization, a 50-Gb/s QPSK signal, which

corresponds to a 25 Gbaud symbol rate. We consider

that polarization transmission effects are ideal and

that ROADM components are polarization

independent. We also assume that the optical

receiver performs an ideal detection for both

polarizations (Seimetz and Weinert, 2006). Hence,

the results presented in this work, for a single

polarization, are valid for both polarizations.

As can be observed in Figure 1, at the ROADM

inputs, the signals pass through Component A,

which depends on the architecture used. In ROADM

nodes based on a B&S architecture, Component A is

an optical splitter, while with a R&S architecture,

this optical splitter is replaced by a WSS. In both

architectures, at the ROADM outputs, the signals go

through a WSS (Simmons, 2014). In Figure 1, to

simplify, we only show the output of one direction

of the ROADMs, to where the primary signal is sent.

2.1 ROADM Add/Drop Structures

In our ROADM model, we consider both MCSs and

WSSs-based add/drop structures. Figure 2 depicts

the model used to implement the drop structure

(Colbourne and Collings, 2011). Figure 2 (a)

considers a N×M MCS-based drop structure and

Figure 2 (b) considers a N×M WSS-based drop

structures.

N×1

Optical

Switches

N×M

MCS

Splitter 1×MSplitter 1×M

...

... ...

N×M

WSS

WSS 1×M WSS 1×M

...

WSS N×1

... ...

......

...

(a)

(

)

...

Figure 2: CDC ROADM N×M drop structures based on (a)

MCSs and (b) WSSs.

The corresponding model for the add structures

is obtained in a similar way, by just having in mind

the direction of the data flow. As can be observed

from Figure 2, the MCSs are based on 1×M splitters

and N×1 optical switches. As such, they are not

wavelength selective as the WSS structures. In terms

of in-band crosstalk generation, since inside a N×M

WSS, the interfering signals pass through the

isolation of two WSSs, the interferers are of second

order, instead of the first order interferers that appear

Physical Layer Impairments in Cascaded Multi-degree CDC ROADMs with NRZ and Nyquist Pulse Shaped Signals

225

on the N×M MCSs. On the other hand, the WSS

structures have higher costs and are more filtering

selective than the MCSs. In terms of modelling these

add/drop structures, the MCSs are modelled by one

filtering stage, while the WSSs are modelled by two

filtering stages.

2.2 ROADMs Filtering Model

We consider two types of transfer functions to

model the filtering inside the ROADM components,

a transfer function for modelling the WSS pass

through effect, represented by 









, and another

transfer function for modelling the WSS blocking

effect represented by 









. The transfer function











is modelled by a super Gaussian optical filter

with lowpass equivalent transfer function given by

(Pulikkaseril, 2011)

























⁄











(1)

where n is the super Gaussian filter order, which, in

this work, is set to n = 4, and B

is the 3 dB

bandwidth, which is set to 41 GHz, usually used for

the 50 GHz fixed grid (Filer and Tibuleac, 2012). On

the other hand, the lowpass equivalent transfer

function 









is given by











1



1



.









⁄











(2)

where a is the blocking amplitude in linear units,

10





. The 3 dB bandwidth of this stopband

filter, when setting B to 41 GHz, is equal to,

approximately, 48 GHz. Figure 3 shows the transfer

functions, 









(Figure 3 (a)) and 









, with

A = 40 dB (Figure 3 (b)).



(a) (b)

Figure 3: Transfer function of the (a) 4

order super

Gaussian optical passband filter, 









, and (b) optical

stopband filter, 









, with A = 40 dB.

3 OPTICAL FILTERING IMPACT

The impact of the optical filtering in the ROADM

cascade represented in Figure 1 is assessed in this

section. The primary signal, along its light-path,

passes through several filtering stages inside the

ROADMs before reaching its destination. These

cascaded filters lead to the narrowing of the

available optical bandwidth, and, consequently, to an

OSNR penalty due to the optical filtering (Hsueh,

2012). To evaluate the OSNR penalty only due to

optical filtering, i.e., the difference between the

required OSNR with and without the filtering

impairment, we only add ASE noise at the end of the

ROADM cascade, to the drop signal 

,

represented in Figure 1. To study the impact of the

optical filtering, we neglect the in-band crosstalk

interferers influence on the primary signal.

In this work, we consider a maximum of 32

ROADMs in cascade (Basch et al., 2006). Figure 4

depicts the OSNR penalty due to optical filtering as

a function of the number of ROADMs based on a

B&S (dashed lines) and a R&S (solid lines)

architectures, for both add/drop structures: WSSs

(blue lines) and MCSs (red lines) and considering

NRZ rectangular signals. From Figure 4, we can

conclude that, the add/drop structures do not have a

significant impact in terms of OSNR penalty due to

optical filtering. The difference between the OSNR

penalty obtained with MCSs and WSSs-based

add/drop structures is less than 0.15 dB. This

difference corresponds to the additional filtering that

the signal experiences when it is added and dropped

with WSSs-based add/drop structures.

Regarding the difference observed, in Figure 4,

between the curves for B&S and R&S architectures,

the OSNR penalty due to optical filtering, as

expected, is lower for a B&S architecture (Filer and

Tibuleac, 2014), since with this architecture, the

signal is not filtered at the ROADM inputs. For this

architecture, an OSNR penalty of 1 dB is not

reached after 32 cascaded ROADMs. For ROADM

nodes based on a R&S architecture, penalties of

~1.5 dB are observed after 32 cascaded ROADMs.

Considering a 1 dB OSNR penalty as the limit for

this penalty, the signal can cross 20 and 22 ROADM

nodes, respectively, with WSSs and MCSs-based

add/drop structures.

The same studies have been done for Nyquist

pulse shaped signals with β = 0.1. In this scenario,

the optical filtering impact is very low, causing

OSNR penalties lower than 0.1 dB after 32 cascaded

ROADMs. This is explained by noting that the

bandwidth of the Nyquist signals is, approximately,

equal to symbol rate, 25 GHz, and the 3 dB

bandwidth of the optical filters for the 50 GHz fixed

grid is much larger than the symbol rate, 41 GHz,

OPTICS 2018 - International Conference on Optical Communication Systems

226

originating a negligible OSNR penalty due to optical

filtering impact, as was also reported in (Morea et

al., 2015).

Figure 4: OSNR penalty due to optical filtering as a

function of the number of ROADMs, for a BER of 10

−3

B&S (dashed lines) and R&S (solid lines) architectures,

WSSs (blue lines) and MCSs (red lines) add/drop

structures and NRZ rectangular signals.

4 IN-BAND CROSSTALK LEVEL

IN A CDC ROADM CASCADE

In this section, the in-band crosstalk level evolution

along a cascade composed by 32 CDC ROADMs is

evaluated for A = 40 dB, several ROADM degrees,

considering both ROADM architectures, different

add/drop structures and rectangular and Nyquist

pulse shaped signals. The crosstalk level, at each

ROADM output, is defined by 

,



,



,

⁄

where 

,

is the average power of all interfering

signals and 

,

is the primary filtered signal

average power, at the output of the M

ROADM

(Cancela et al., 2016). The crosstalk level shown in

Figures 5 and 6 is obtained by averaging the power

of all crosstalk sample functions generated in the

MC simulator.

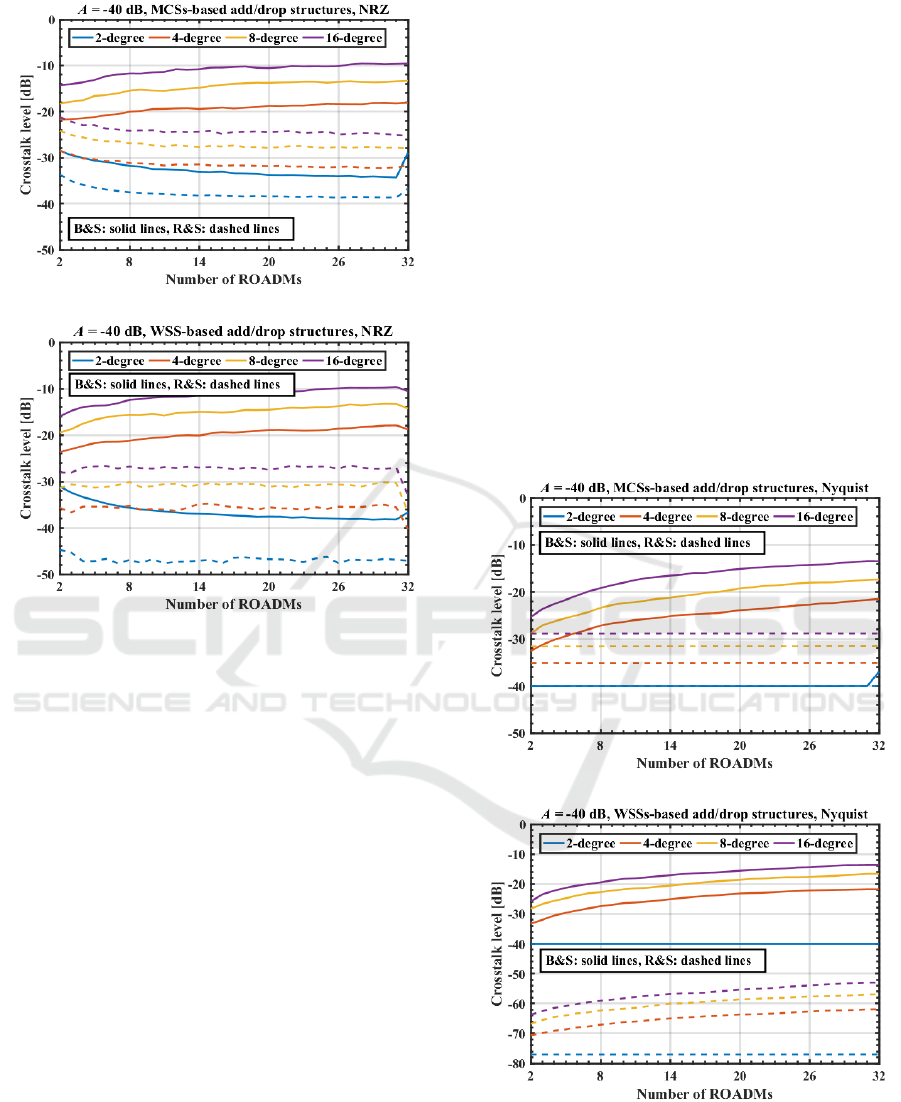
Figure 5 depicts the evolution of the crosstalk

level, in a cascade of 32 CDC ROADMs, as a

function of the number of ROADMs based on a

B&S (solid lines) and a R&S (dashed lines)

architecture, considering NRZ rectangular signals.

Figure 5 (a) considers MCSs and Figure 5 (b)

considers WSSs-based add/drop structures. Several

observations can be made from this figure.

First, as expected, the crosstalk level increases

with the increase of the ROADM degree.

Second, for a R&S architecture, the crosstalk

level along the ROADM cascade is lower than for a

B&S architecture, since, the interfering signals

experience more blocking filtering stages in a R&S

than in a B&S architecture.

Third observation: we can see in Figure 5 (a),

with MCSs-based add/drop structures, a decrease of

the crosstalk level along the network for the R&S

architecture (dashed lines). This can be explained by

noting that the interfering signals that came from the

first ROADM add structure are considered first

order crosstalk terms (i.e. they pass through one

stopband filter), whereas all the other interfering

signals that appear along the light-path are second

order terms (i.e. they pass through two stopband

filters). In this way, the first order in-band terms will

define the crosstalk level, which has a decrease

along the ROADM cascade due to the filtering

performed by the WSSs.

On the other hand, for a B&S architecture (solid

lines), the interfering signals are all first order terms,

so the total crosstalk level increases along the

ROADM cascade, except for 2-degree ROADMs. In

this case, the crosstalk level decreases along the

cascade until the last ROADM, where the crosstalk

level increases. This behaviour occurs because in the

add section of the first ROADM and in the ROADM

input of the last ROADM, first order terms are

originated. All the other ROADMs, where the signal

is expressed, do not contribute with first order terms,

consequently, the ROADM filtering decreases the

crosstalk level until the last ROADM. Note that, at

the end of the ROADM cascade, for 16-degree

ROADMs with MCSs-based add/drop structures, an

increase of 4 dB in the crosstalk level is observed.

Figure 5 (b) depicts the crosstalk level evolution

but with WSSs-based add/drop structures. Here, we

can observe a crosstalk level decreases in the last

ROADM. This decrease is more abrupt for the R&S

architecture, because the interfering signals pass

through three stopband filters in the last ROADM

node (one in the “route” WSS and two in the “drop”

WSS). This crosstalk level decrease is not observed

for 2-degree ROADMs based on a B&S architecture

(blue solid line), for the same reason mentioned in

the previous paragraph. For the R&S architecture,

with WSS-based add/drop structures, the crosstalk

level is practically constant along the ROADM

cascade, since all interfering terms generated are

second order. Consequently, the crosstalk level is,

mostly, defined in the first ROADM node.

Physical Layer Impairments in Cascaded Multi-degree CDC ROADMs with NRZ and Nyquist Pulse Shaped Signals

227

(a)

(b)

Figure 5: Crosstalk level as a function of the number of

ROADMs, A = 40 dB, for both architectures, B&S (solid

lines) and R&S (dashed lines), NRZ rectangular signals,

several ROADM degrees and (a) MCSs and

(b) WSSs-based add/drop structures.

Figure 6 shows the crosstalk level evolution

along the ROADM cascade, but considering Nyquist

pulse shaped signals. Figure 6 (a) refers to MCSs

and Figure 6 (b) to WSS-based add/drop structures.

In Figure 6 (a), for a R&S architecture (dashed

lines), a constant crosstalk level along the ROADM

cascade can be observed. This behaviour is justified

by the fact that the interfering terms from the first

ROADM add structure are first order terms, while

the other interfering terms coming from the other

ROADMs in the cascade, either from the ROADM

inputs or from ROADM add structure, are all second

order terms. Besides that, since the optical stopband

filter in the first ROADM is more effective with

Nyquist signals than with NRZ rectangular signals,

the crosstalk level remains constant along the

ROADM cascade.

For a B&S architecture (solid lines), the behavior

of the crosstalk level evolution along the optical

network is similar with the previously obtained for

NRZ rectangular signals. Nevertheless, the crosstalk

level variation between the first and the last

ROADM of the cascade, in this case, is higher than

with NRZ rectangular signals. For example, for

16-degree ROADM based on a B&S architecture

with MCSs-based add/drop structures, we have a

variation of ~4 dB and ~11 dB, respectively, for

NRZ rectangular and Nyquist pulse shaped signals.

The main reason is because the stopband filters used

in this work, for the 50 GHz fixed grid, provide a

better blocking of in-band crosstalk interfering

signals for the Nyquist pulse shaped signals, since

the Nyquist signals bandwidth with β = 0.1 is,

approximately, one half in comparison with the NRZ

rectangular signals bandwidth. For the same reason,

for Nyquist pulse shaped signals, we can observe

that after two cascaded ROADMs based on a B&S

architecture and with MCSs-based add/drop

structures, Figure 6 (a), the crosstalk level is lower

~10 dB than for NRZ rectangular pulse shaped

signals, Figure 5 (a).

(a)

(b)

Figure 6: Crosstalk level as a function of the number of

ROADMs, A = 40 dB, for both architectures, B&S (solid

lines) and R&S (dashed lines), Nyquist pulse shaped

signals, several ROADM degrees and (a) MCSs and

(b) WSSs-based add/drop structures.

OPTICS 2018 - International Conference on Optical Communication Systems

228

From Figure 6 (b), we can conclude that, with

Nyquist pulse shaped signals, WSSs-based add/drop

structures and a R&S architecture (dashed lines), the

crosstalk levels originated are very low, below

−50 dB. For a B&S architecture (solid lines), the

crosstalk levels obtained are very similar with those

obtained with MCSs-based add/drop structures in

Figure 6 (a).

5 IN-BAND CROSSTALK

IMPACT

After having studied the crosstalk level generated in

a CDC ROADM cascade, for both B&S and R&S

architectures, MCSs and WSSs-based add/drop

structures, NRZ rectangular and Nyquist pulse

shaped signals and several ROADM degrees, the

OSNR penalty due to in-band crosstalk is evaluated

in this section.

In the previous section, we have concluded that

with A = 40 dB and a R&S architecture, the

crosstalk levels generated along the ROADM

cascade are below −20 dB. Consequently, this

crosstalk level does not lead to a significant network

degradation. Thus, in this section, we only study the

OSNR penalty due to the in-band crosstalk for the

B&S architecture.

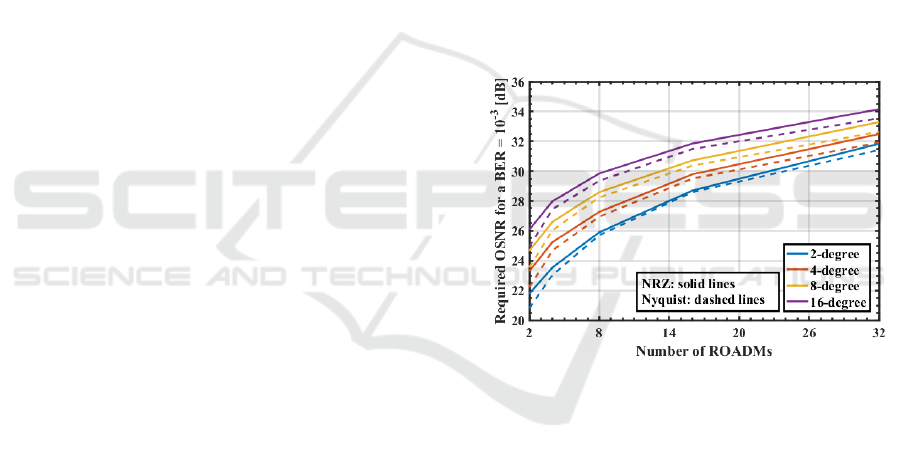
Figure 7 shows the required OSNR, at the output

of each OA, for a target BER of 10

−3

, as a function

of the number of ROADMs for NRZ rectangular

(solid lines) and Nyquist (dashed lines) pulse shaped

signals and a B&S architecture. The same studies

have been done for the R&S architecture and the

required OSNRs obtained are very similar, with

differences below 0.5 dB. Note that, in this work, we

consider that the required OSNR is the OSNR

imposed in each OA to reach a target BER of 10

−3

the end of the ROADM cascade. This required

OSNR is measured without the in-band crosstalk

impairment, but including the impact of the optical

filtering and ASE noise addition in all ROADM

inputs and outputs, as shown in Figure 1. In this

work, we consider that all ROADM nodes introduce

the same insertion losses regardless the ROADM

architecture and ROADM add/drop structures. For

future work, we will consider the insertion losses

depending on the ROADM architectures, and, also,

on the ROADM add/drop structures.

From Figure 7, we can conclude that, the

required OSNR variation with the number of

ROADMs and the ROADMs degree is very similar

for both signal shapes studied. For Nyquist pulse

shaped signals, there is an improvement of the

required OSNR that reaches 1 dB for 16-degree

ROADMs. For all ROADM degrees considered,

there is a degradation of about 10 dB of the required

OSNR from a cascade of 2 nodes to a cascade of 32

ROADMs nodes. For example, for 2-degree

ROADMs, the required OSNR after 2 nodes is

19 dB and after 32 nodes, it is approximately 29 dB,

for NRZ rectangular signals.

To calculate the OSNR penalty due to in-band

crosstalk shown in the Figure 8 and 9, we considered

the reference OSNR from the results plotted in

Figure 7.

Figure 8 shows the OSNR penalty due to in-band

crosstalk as a function of the number of ROADMs,

for a target BER of 10

−3

, A = −40 dB, considering

both add/drop structures, MCSs (dashed lines) and

WSSs (solid lines), for several ROADM degrees and

NRZ rectangular signals. From this figure, we can

conclude that, for an OSNR penalty of 1 dB, the

maximum number of cascaded ROADMs decreases

with the ROADM degree increase.

Figure 7: Required OSNR for a BER equal to 10

−3

as a

function of the number of ROADMs, for the NRZ

rectangular (solid lines) and Nyquist (solid lines) pulse

shaped signals, several ROADM degrees and a B&S

architecture.

For example, for 8-degree ROADMs, the optical

signal can pass through 20 and 28 nodes,

respectively, with MCSs and WSSs-based add/drop

structures. While for 16-degree ROADMs, where

more interfering signals arise in each node, the

signal can pass through 8 and 13 ROADMs,

respectively, with MCSs and WSSs-based add/drop

structures. So, by implementing the add/drop

structures with WSSs instead of MCSs, an

improvement of 8 and 5 ROADMs has been

obtained, respectively, with degree 8 and 16.

Figure 9 shows the OSNR penalty due to in-band

crosstalk as a function of the number of 16-degree

ROADMs, for NRZ rectangular (red lines) and

Physical Layer Impairments in Cascaded Multi-degree CDC ROADMs with NRZ and Nyquist Pulse Shaped Signals

229

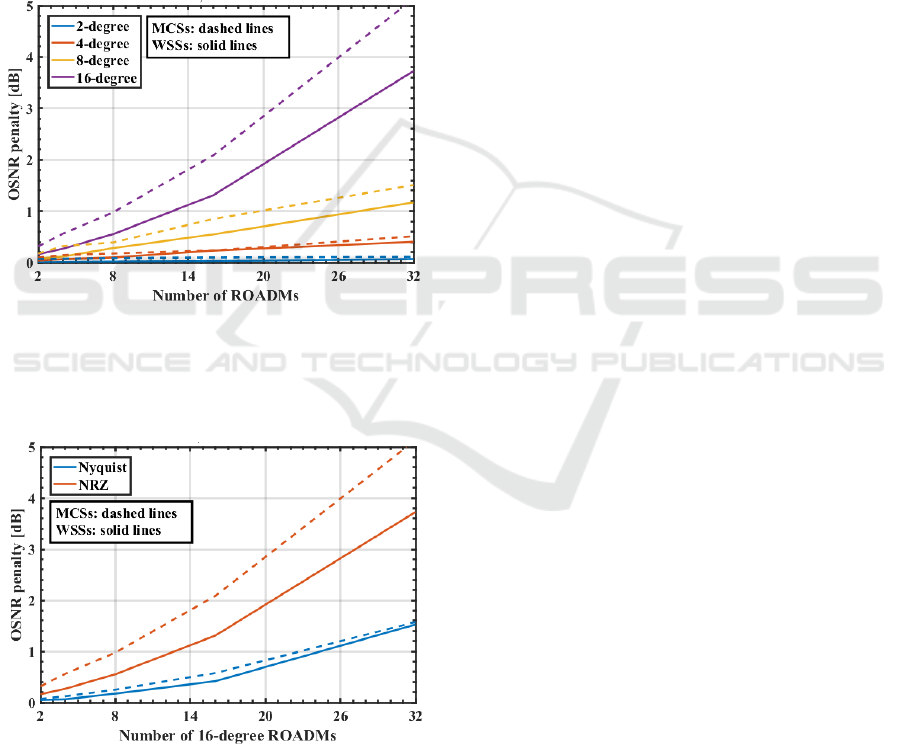
Nyquist (blue lines) pulse shaped signals. From this

figure, we can observe a significant improvement on

the ROADMs number that an optical signal can pass

with the Nyquist pulse shape. An OSNR penalty of

1 dB is reached after 13 and 24 cascaded 16-degree

ROADMs, for, respectively, NRZ rectangular and

Nyquist pulse shaped signals and with WSSs-based

add/drop structures. It means an improvement of 11

ROADMs. For MCSs-based add/drop structures, the

improvement is about 15 ROADMs. This

improvement is related with the crosstalk level at the

end of the ROADM cascade, which is higher for

NRZ rectangular signals than for Nyquist pulse

shaped signals, as shown in Figures 5 and 6.

Figure 8: OSNR penalty due to the in-band crosstalk as a

function of the number of ROADMs, for a BER of 10

−3

A = −40 dB, add/drop structures based on MCSs (dashed

lines) and on WSSs (solid lines) and NRZ rectangular

signals.

Figure 9: OSNR penalty due to in-band crosstalk as a

function of the number of 16-degree ROADMs, for a BER

of 10

−3

, A = −40 dB, add/drop structures based on MCSs

(dashed lines) and on WSSs (solid lines) and for NRZ

rectangular (red lines) and Nyquist (blue lines) pulse

shaped signals.

Comparing the impact of the ASE noise

accumulation with the in-band crosstalk impact in a

CDC ROADM cascade, we can conclude that, the

ASE noise accumulation has a greater impact than

the in-band crosstalk in terms of OSNR penalty. As

referred, at the end of a cascade with 32 ROADMs,

the ASE noise accumulation leads to an OSNR

penalty of, approximately, 10 dB. The in-band

crosstalk, in the worst case (i.e., with NRZ

rectangular signals, MCSs-based add/drop

structures, a B&S architecture and 16-degree

ROADMs) leads to an OSNR penalty slightly higher

than 5 dB.

6 CONCLUSIONS

In this work, we have investigated the impact of

PLIs, namely, optical filtering, in-band crosstalk and

ASE noise accumulation in a CDC ROADM cascade

for both B&S and R&S architectures and with MCSs

and WSSs-based add/drop structures. Our studies

have been performed considering 100-Gb/s QPSK

signals for the 50 GHz fixed grid with NRZ

rectangular and Nyquist pulse shapes.

Our results showed that the impact of the optical

filtering with NRZ rectangular signals and a R&S

architecture is more significant than with a B&S

architecture. For CDC ROADMs based on a R&S

architecture, the optical signal can pass through 20

and 22 ROADM nodes, respectively, with WSSs and

MCSs-based add/drop structures, until an OSNR

penalty of 1 dB is reached. The B&S architecture

does not lead to an OSNR penalty of 1 dB at the end

of 32 cascaded ROADMs. For Nyquist shaped

signals, we have observed that the impact of optical

filtering is negligible, for both ROADM

architectures.

In terms of the in-band crosstalk level generated

in a ROADM cascade, we have concluded that, for a

R&S architecture, the crosstalk level is below

–20 dB due to the enhanced signal blocking imposed

by the higher number of WSSs in the light-path. In

ROADMs based on a B&S architecture, the OSNR

penalty due to in-band crosstalk is higher with

MCSs-based add/drop structures. An OSNR penalty

of 1 dB is reached after a NRZ rectangular QPSK

signal passes through 20 and 8 CDC ROADM

nodes, respectively, with degree 8 and 16. An

improvement is reached using WSSs-based add/drop

structures. The OSNR penalty of 1 dB due to

in-band crosstalk is reached at the end of 28 and 13

cascaded ROADMs, respectively, with degree 8 and

16. For Nyquist pulse shaped signals, the OSNR

OPTICS 2018 - International Conference on Optical Communication Systems

230

penalty is lower than for NRZ rectangular signals,

for both add/drop structures. Our results showed an

improvement of 15 and 11 ROADMs in cascade

with Nyquist pulse shapes for 16-degree ROADMs,

and, respectively, MCS and WSSs-based add/drop

structures.

We, also, have seen that, the ASE noise

accumulation along the ROADM cascade leads to a

10 dB OSNR degradation after 32 cascaded

ROADMs and should be considered as a limitation

factor to the number of ROADMs that a signal can

cross in an optical network.

ACKNOWLEDGEMENTS

This work was supported by Fundação para a

Ciência e Tecnologia (FCT) of Portugal within the

project UID/EEA/50008/2013.

REFERENCES

Basch, E., et al. (2006). Architectural tradeoffs for

reconfigurable dense wavelength-division

multiplexing systems. IEEE J. Sel. Top. Quantum

Electron., 12(4), 615-626.

Cancela, L., et al. (2016). Analytical tools for evaluating

the impact of in-band crosstalk in DP-QPSK signals.

NOC, 6-11.

Colbourne, P. and Collings, B. (2011), ROADM

Switching Technologies. OFC, pp. OTuD1.

Essiambre, R., (2010). Capacity limits of optical fiber

networks. J. Lightw. Technol., 28(4), 662-701.

Fabrega, J., et al. (2016). On the filter narrowing issues in

elastic optical networks. J. Opt. Commun. Netw., 8(7),

A23-A33.

Feuer, M., et al. (2011). Intra-node contention in dynamic

photonic networks. J. Lightw. Technol., 29(4), 529-

535.

Filer, M. and Tibuleac, S. (2012). Generalized weighted

crosstalk for DWDM systems with cascaded

wavelength-selective switches. Opt. Exp., 20(16),

17620-17631.

Filer, M. and Tibuleac, S. (2014). N-degree ROADM

architecture comparison: Broadcast-and-select versus

route-and-select in 120 Gb/s DP-QPSK transmission

systems. OFC, pp. Th1I-2.

Gringeri, S., et al. (2010). Flexible architectures for optical

transport nodes and networks. IEEE Commun. Mag.,

48(7), 40-50.

Hsueh, Y., et al. (2012). Passband narrowing and crosstalk

impairments in ROADM-enabled 100G DWDM

networks. J. Lightw. Technol., 30(24), 3980-3986.

Jinno, M. (2017). Elastic optical networking: Roles and

benefits in beyond 100-Gb/s era. J. Lightw. Technol.,

35(5), 1116-1124.

Morea, A., et al. (2015). Throughput comparison between

50-GHz and 37.5-GHz grid transparent networks. J.

Opt. Commun. Netw., 7(2), A293-A300.

Pan, J. and Tibuleac, S. (2016). Filtering and crosstalk

penalties for PDM-8QAM/16QAM super-channels in

DWDM networks using broadcast-and-select and

route-and-select ROADMs. OFC, pp. W2A-49.

Pulikkaseril, C. (2011). Spectral modeling of channel band

shapes in wavelength selective switches. Opt. Exp.,

19(9), 8458-8470.

Roberts, K., et al. (2017). Beyond 100 Gb/s: capacity,

flexibility, and network optimization. J. Opt. Commun.

Netw., 9(4), C12-C24.

Seimetz, M. and Weinert, C. (2006). Options, feasibility,

and availability of 2×4 90º hybrids for coherent optical

systems. J. Lightw. Technol., 24(3), 1317-1322.

Simmons, J. (2014). Optical network design and planning.

Springer, 2

edition.

Tibuleac, S. and Filer, M. (2010). Transmission

impairments in DWDM networks with reconfigurable

optical add-drop multiplexers. J. Lightw. Technol.,

28(4), 557-598.

Wang, Y. and Lyubomirsky, I. (2010). Impact of DP-

QPSK pulse shape in nonlinear 100 G transmission. J.

Lightw. Technol, 28(18), 2750-2756.

Way, W. (2012). Optimum architecture for M×N multicast

switch-based colorless, directionless, contentionless,

and flexible-grid ROADM. OFC, pp. NW3F-5.

Woodward, S., et al., (2010). Intra-node contention in a

dynamic, colorless, non-directional ROADM. OFC.

Yang, H., et al. (2017). Low-cost CDC ROADM

architecture based on stacked wavelength selective

switches. J. Opt. Commun. Netw., 9(5), 375-384.

Zami, T. (2013). Current and Future Flexible Wavelength

Routing Cross‐Connects. Bell Labs Technical Journal,

18(3), 23-38.

Physical Layer Impairments in Cascaded Multi-degree CDC ROADMs with NRZ and Nyquist Pulse Shaped Signals

231