Thermal Cycling Behaviour of Dense Monolithic Alumina

Luís Guerra Rosa

, José Rodriguez

, José C. G. Pereira

and Jorge C. Fernandes

IDMEC, IST, Universidade de Lisboa, Av. Rovisco Pais, Lisboa, Portugal

Plataforma Solar de Almería, CIEMAT, Tabernas (Almería), Spain

DEQ, IST, Universidade de Lisboa, Av. Rovisco Pais, Lisboa, Portugal

Keywords: Alumina, Thermal Cycling, Concentrated Solar Radiation.

Abstract: The behaviour of circular discs, with 25 mm diameter and 2 mm thickness, made of commercial high-purity

dense monolithic Alumina (RAPAL

100) is evaluated under three different sequences of 100 heating-cooling

cycles, all of them with a maximum temperature of 1200ºC. None of the different sequences of 100 cycles

(even the most severe one with 100 cycles applied in 77 minutes, with maximum temperature of 1200°C and

minimum temperature of 400°C) has caused noticeable alterations in the studied properties (density; Young´s

modulus of elasticity; Coulomb´s modulus of elasticity; flexural strength) measured before and after the heat

treatment.

1 INTRODUCTION

The increasing demand of ceramic materials for

structural applications – namely in components for

wear resistance and high temperature use – stresses

the need for a deeper knowledge and correct

characterization of their mechanical behaviour. In

their nature, ceramics are brittle and critically

dependent of the presence of flaws (porosity, internal

and surface cracks, etc.) which are responsible, not

only for a significant decrease in strength, but also for

a high scatter in data. In brittle materials, strength

may be seen as a consequence of two factors: (1)

fracture toughness (K

, an intrinsic value of the

material), and (2) the distribution (in size and

orientation) of the population of flaws that are present

in the specimens or test-pieces. A flaw, geometrical

discontinuity or other heterogeneity, that causes an

effect of stress/strain concentration and it is

responsible for the rupture is named “critical flaw”.

For many applications of technical or advanced

ceramics, it is important to evaluate their behaviour

when they are exposed to high-temperature cycles

and rapid cooling at the surface. If the temperature to

which a test-piece is exposed varies rapidly i.e. if it is

subjected to “thermal shock”, a difference in

temperature between the surface and the bulk of the

test-piece will be generated, and this will create

mechanical stresses of high magnitude. These high-

magnitude stresses can cause the growth of pre-

existent flaws, thus leading to the degradation of

some of the mechanical properties. According to

(Evans et al., 1975) the stress 



caused by a thermal

shock T is given by:









  









(1)

where E is Young’s modulus, α is the linear thermal

expansion coefficient, ν is Poisson’s ratio, and 

is a function that introduces the influence of the

geometry of the test-piece that is subjected to thermal

shock. β is known as the Biot modulus, defined as:







(2)

where b is a specimen dimension, h is the heat transfer

coefficient and k is the thermal conductivity of the

specimen or test-piece. Consequently, we may notice

that the determination of thermal stresses caused by

thermal shock is not easy because the values of the

heat transfer coefficient h and the function  must

be known with significant precision. On the other

side, as suggested by (Hirata, 2015), the linear

thermal expansion coefficient α of a material has not

a constant value because it depends on temperature

and microstructure of the material.

In comparative terms, alumina (Al

) is to

technical ceramics what mild steel is to metals – it is

Rosa, L., Rodriguez, J., Pereira, J. and Fernandes, J.

Thermal Cycling Behaviour of Dense Monolithic Alumina.

DOI: 10.5220/0008187201730179

In The Second International Conference on Materials Chemistry and Environmental Protection (MEEP 2018), pages 173-179

ISBN: 978-989-758-360-5

173

relatively cheap, easy to process, and it has a wide

range of industrial applications. Pure alumina has a

melting point of 2072ºC, therefore alumina

components are used at operating temperatures as

high as 1200 – 1300°C. Despite there are some works

dedicated to the study of the alumina behaviour when

subjected to thermal shock (Saâdaoui and Fantozzi,

1998; Hahn and Lee, 1999; Lee et al., 2002;

Dimitrijevic et al., 2013; Belghalem et al., 2014; Li et

al., 2016) we do not know any experimental work

dealing with the behaviour of alumina when subjected

to high-temperatures cycles at the surface of the test-

pieces with rapid cooling/heating conditions.

Therefore, the primary goal of this work is to evaluate

the performance of test-pieces (circular discs with 25

mm diameter and 2 mm thickness) of a commercial

grade of high-purity dense monolithic Alumina

(RAPAL

100) when it is exposed to rapid cycles of

temperature variation.

The creation of rapid cycles of temperature

variation at the surface of a test-piece is not easy to

achieve using the traditional heating-systems,

whatever they are: fuel or gas combustion furnaces,

electric resistance furnaces, induction furnaces, or

even microwave furnaces. However, one way to

generate rapid variations of temperature is by direct

exposure of the test-piece to concentrated solar

radiation. Some examples of the use of concentrated

solar radiation for rapid heating and thermal cycling

are available in the literature (Douale et al., 1999;

Kováčik et al., 2014; Sallaberry et al., 2015).

2 MATERIAL AND TESTS

2.1 RAPAL

100 Alumina Discs

The alumina produced by Rauschert company with a

purity grade of 99.7% bears the trade names of

RAPAL

200 or RAPAL

100. This type of alumina

possesses a density close to the theoretical value,

because is practically free of porosity. It presents

excellent properties, namely: very high hardness

which gives the pieces a high wear resistance; high

mechanical strength; good temperature resistance up

to 1650ºC; good/moderate thermal conductivity; it is

an electrical isolator even at high temperatures; it is

corrosion resistant in diluted acids and lyes; it allows

highly polished surfaces, which show low-friction

coefficient.

As mentioned in the Introduction, in the present

work we have used circular discs, with 25 mm

diameter and 2 mm thickness, made of commercial

high-purity dense monolithic alumina RAPAL

100.

According to the manufacturer (the Rauschert

company), the discs were produced through uniaxial

pressing. The starting powder mean particle size (d

)

is 1 micron, with a specific area of 2.11 m

/g (BET).

The powder compacting pressure is 100 MPa, applied

at ambient temperature. After being compacted, the

discs are sintered at 1635ºC with a holding time of

150 minutes. The following properties of RAPAL

100 are mentioned by the manufacturer (Rauschert

data sheet, 2018): density: > 3.85 g/cm

; uniaxial

flexural strength: 300 MPa; Young’s modulus: 380

GPa; Mohs hardness: 9; Vickers hardness HV

0.1

1700‒2300; linear thermal expansion coefficient (in

the range 20‒1000°C): 910

-6

-1

; thermal

conductivity: 19‒30 W m

-1

; electrical resistivity

at 20°C: 10

.cm.

2.2 High Temperature Cycles

Tests with rapid heating-cooling cycles were carried

out thanks to the capabilities and characteristics of a

high-power solar furnace: the 60 kW power solar

furnace SF60 of the Plataforma Solar de Almería, in

Spain. A schematic of the solar furnace test system

for obtaining a concentrated solar beam horizontally

oriented towards the target is shown in Figure 1. In

the case of SF60, the solar direct radiation is reflected

by a 130 m

flat heliostat (placed outside the building)

onto a fixed parabolic concentrator (inside the

building) composed of 89 facets (curved mirrors)

which altogether make a total of 100 m

parabolic-

shape reflecting surface area (see Figure 2). The

parabolic concentrator reflects and concentrates the

sunlight on a focal area where the test setup is

installed. The solar flux can be controlled by

adjusting the opening of the shutter or attenuator; and

hence it is the opening percentage of the shutter that

controls the temperature in the test setup. In our

experiments, a 45º inclined mirror was placed before

the focal area so that the concentrated solar beam

becomes vertically oriented towards the target. The

45º inclined mirror is depicted in Figure 2.

The test setup placed at the focal area is depicted

in Figures 3 and 4 in order to explain the positioning

of the discs and the location of the thermocouples that

were used to measure the temperature at various

locations in the vicinity of the alumina discs. Figure

3 shows six discs of RAPAL

100 duly positioned on

a zirconia felt and ready to be irradiated by

concentrated sunlight. During this work, 36 identical

discs were exposed to the thermal cycles, in groups of

6 + 6 = 12 discs.

MEEP 2018 - The Second International Conference on Materials Chemistry and Environmental Protection

174

Figure 1: Schematics of SF60 test system at Plataforma

Solar de Almería (Martínez Plaza, 2013).

Figure 2: Parabolic concentrator, 45º inclined mirror and

the test table of SF60 solar furnace.

Figure 3: Discs of RAPAL

100 ready to be irradiated.

For measuring the temperature at various

locations in the vicinity of the discs, twelve type K

thermocouples were used. Figure 4 shows the

locations of the thermocouples’ joints, after removing

the discs, the zirconia felt, and the alumina

thermocouple protection sheaths.

Figure 4: Locations of the thermocouples’ joints.

For obtaining a more homogeneous distribution of

temperature at the surface of the discs, a device

(named “homogenizer”) composed of vertical mirrors

was placed close to the focal zone. The distribution of

temperature was also evaluated using an infra-red

camera and its software (see Figure 5); characteristics

of IR CAM model: Equus 327k SM PRO; detector:

Indium Antimonide (InSb) focal plane array (FPA);

resolution: 327 680 (640 512) pixels; spectral range:

1.5 μm to 5 μm (SM).

Figure 5: Image obtained by the IR CAM software.

To generate rapid heating-cooling cycles at the

surface of the irradiated discs, an automatic system

was developed composed by a plate with

reciprocating motion, i.e. with a repetitive back-and-

forth linear motion. This type of horizontal shutter is

placed close to the focal zone in order to interfere with

solar radiation flux before it irradiates the discs. The

cooling of the discs can be accelerated by blowing

them with compressed air.

Thermal Cycling Behaviour of Dense Monolithic Alumina

175

Table 1: Groups of discs and corresponding heating-cooling cycles.

No. of thermal

cycles

Temperature at central thermocouple

Total duration of 100

cycles

Maximum

Minimum

Group A

100

1200°C

900°C

≈ 35 minutes

Group B

100

1200°C

400°C

≈ 150 minutes

Group C

100

1200°C

400°C

≈ 77 minutes

Figure 6: Example of heating-cooling cycles.

All discs were exposed to 100 heating-cooling

cycles with the same maximum temperature, T

max

1200°C, measured by the thermocouple with the

reference number 1 (located at the centre). Table 1

summarizes the differences among the heat

treatments applied to the three groups of discs.

Beside these three groups (each one composed of

12 discs) that were exposed to the thermal cycling,

another group of 12 unexposed discs was used for

assessing the mechanical properties before the

thermal cycling.

An example of the heating-cooling cycles

obtained by exposure of the discs to concentrated

solar radiation is shown in Figure 6: the thermal

cycling of group C discs (T

max

≈ 1200°C; T

min

≈

400°C). In this case, 6 discs were exposed to 100

cycles between 10:36 and 11:56 (local time) and then

other 6 discs were exposed to 100 cycles between

12:13 and 13:23 (local time). In solar furnaces it is a

tradition to register the temperatures versus the local

time (hour of the day). We need the best insolation

conditions to attain high-temperature in the

experiments. In our experiments the solar radiation

reaching the heliostat was in range of 700 – 840 W/m

depending on the hour and weather conditions during

the day.

A close view of variation of temperature versus

time for two thermal cycles (in the period between

12:39:40 and 12:41:10 of Figure 6) is shown in Figure

7. The temperature indicated in both Figures 6 and 7

is the temperature measured by the thermocouple

located at the centre (i.e. the thermocouple with

reference no. 1 in Figure 4). Heating and cooling rates

must be determined from the experimental data. In the

MEEP 2018 - The Second International Conference on Materials Chemistry and Environmental Protection

176

case of Figure 7, each period of heating from 400ºC

till 1200ºC takes circa 17 seconds; and the cooling

period from 1200ºC back to 400ºC takes around 24

seconds.

Figure 7: Temperature versus time for cycles in the period

between 12:39:40 and 12:41:10 of Figure 6.

2.3 Determination of E and G

Young’s modulus (E) and Coulomb’s modulus (G)

were determined by excitation of vibration in the

discs and measurements of resonant frequencies,

according to ASTM E 1876 standard test method. The

equipment used was made by IMCE n.v. – Integrated

Material Control Engineering (Diepenbeek,

Belgium). The procedure consists in tapping the

sample (disc) with a small hammer and recording the

induced vibration signal with a microphone.

Afterwards, the acquired vibration signal in the time

domain is converted to the frequency domain by a fast

Fourier transformation. Dedicated software

determines the resonant frequency for each type of

vibration: flexural or torsional, and then, using the

classical theory of elasticity, calculates E and G

values once the mass and the geometry of the test-

piece are known.

2.4 Equibiaxial Flexural Strength

Figure 8 provides a schematics showing the ring-on-

ring test set-up used for determination of monotonic

equibiaxial flexural strength at ambient temperature,

according to ASTM C 1499 standard test method. A

self-aligning ring-on-ring jig that generates the

equibiaxial flexure is placed between compression

plates in a universal mechanical testing machine

(Instron 5566 equipped with a 10 kN load cell). The

test-piece (alumina disc) is compressed between two

concentric rings with different diameter. In our

experiments, the crosshead velocity of the testing

machine was 0.5 mm per minute. Figure 9 depicts a

group of alumina discs after being fractured by the

ring-on-ring test procedure.

Figure 8: Section view of basic fixturing and test specimen

for equibiaxial testing according to ASTM C 1499.

Figure 9: A group of alumina discs after ring-on-ring tests.

The flexural strength 



 in equibiaxial conditions

is given by (Fernandes and Rosa, 1991):













 



  









 













  















(3)

where F refers to the rupture load, h the specimen

thickness,



the Poisson's ratio of the specimen

material, 



the diameter of the lower ring (20.2mm),





the diameter of the upper ring (10.1 mm), and 

the diameter of the test-piece (alumina disc).

Thermal Cycling Behaviour of Dense Monolithic Alumina

177

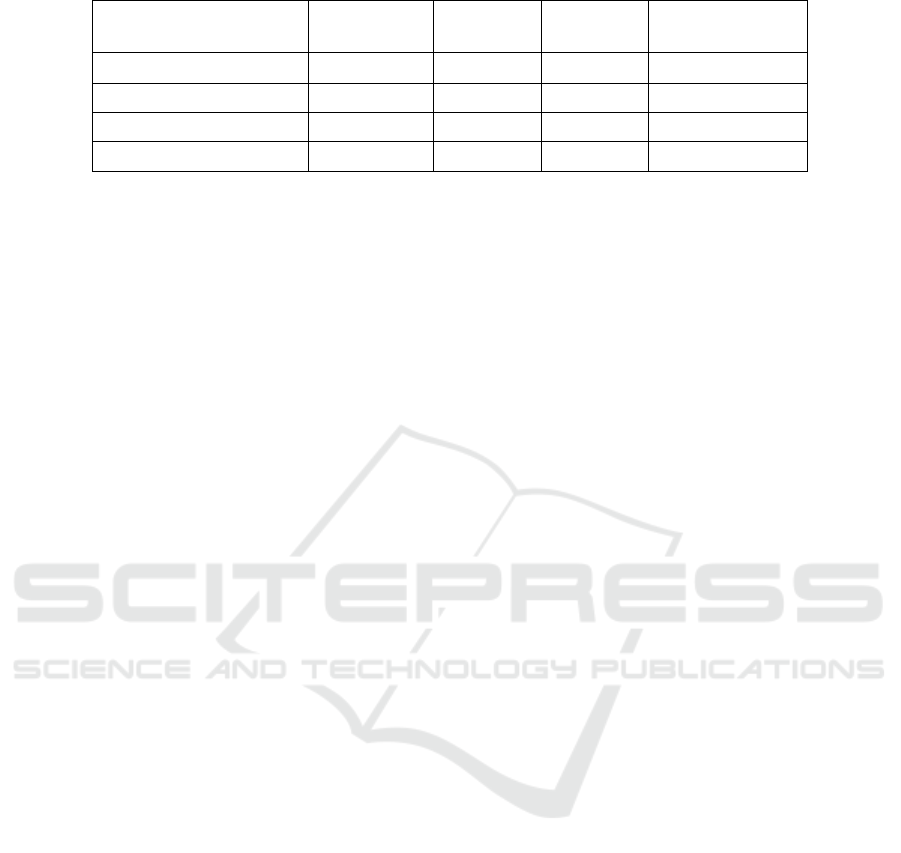
Table 2. Average values (± standard deviation) of properties before and after heating-cooling cycles.

Density

[g/cm

]

[GPa]

Flexural Strength

[MPa]

Before: reference group

3.91 ± 0.01

364 ± 7

158 ± 2

244 ± 46

After: Group A

3.93 ± 0.01

369 ± 8

159 ± 2

238 ± 37

After: Group B

3.92 ± 0.01

363 ± 7

157 ± 2

211 ± 34

After: Group C

3.92 ± 0.01

362 ± 6

158 ± 2

242 ± 31

3 RESULTS AND DISCUSSION

The results obtained from the experiments allow to

compare the values of the properties before and after

exposure to thermal cycling. This was the rationale

used to evaluate the possible degradation of the

alumina when exposed to rapid thermal cycles at

high-temperature. Table 2 summarizes the average (±

standard deviation) values obtained for the following

properties of RAPAL

100: density (determined by

Archimedes method); Young’s modulus of elasticity

(E); Coulomb’s modulus of elasticity (G); and

equibiaxial flexural strength.

In the whole literature, we did not find other

researchers’ results for comparison, because the

thermal cycling experiments described in this work

are very difficult to be performed with conventional

furnaces; and therefore the results obtained in the

present work are relevant. They have demonstrated

the good quality of RAPAL

100 and its adequacy for

high temperature applications. We have not observed

any rupture in the alumina discs during their exposure

to the thermal cycles. None of the different sequences

of 100 cycles (even the most severe one with 100

cycles applied in 77 minutes, with maximum

temperature of 1200°C and minimum temperature of

400°C) has caused noticeable alterations in the

studied properties (density; Young´s modulus of

elasticity; Coulomb´s modulus of elasticity; flexural

strength) when comparing the values before and after

applying the heating-cooling cycles. The only

perceptible difference it is practically negligible; it

consists in the decay of flexural strength after

exposure to 100 cycles in 150 minutes, with T

max

1200°C e T

min

= 400°C; initial value is 244 (±46) MPa

and after those 100 cycles is 211 (±34) MPa.

According to the work of (Hahn and Lee,1999)

the critical thermal stress which makes the cracks

grow catastrophically was found to be generated by

the critical cooling rate, and the critical cooling rate

of polycrystalline alumina ceramics was found to be

a certain value: 550ºC/second (Hahn and Lee, 1999).

In our experiments the highest cooling rate was

approximately 34ºC/s which is circa 16 times less

than 550ºC/s. This explains why we did not notice

any crack propagation in the test-pieces, even after

100 cycles; as well as we did not obtain any relevant

statistical changes in the mechanical properties.

The value of Young’s modulus mentioned in the

Rauschert data sheet (see section 2.1) is 380 GPa,

slightly higher than the value obtained in our work,

364 (±7) GPa, for the reference group of “as received”

samples i.e. before exposure to thermal cycling (see

Table 2). This small discrepancy can be explained by

differences in the testing methods. Also, Rauschert

data sheet mentions that uniaxial flexural strength of

RAPAL

100 is 300 MPa, without referring the data

scatter. In our work we measured the flexural strength

under equibiaxial conditions and obtained a lower

average value, 244 (±46) MPa, for the reference

group i.e. before the heating-cooling cycles. These

values are perfectly acceptable because the uniaxial

flexural strength is typically determined under a 3-

point bend test (where the sampled volume is

smaller), so the probability of finding a larger critical

flaw is higher in equibiaxial conditions, resulting in a

lower flexural strength.

4 CONCLUSIONS

This work demonstrates that rapid variations of

temperature at the surface of a material can be

attained by direct exposure to highly concentrated

solar radiation. For the first time, the thermal cycling

behaviour of dense monolithic alumina (RAPAL®

100) was studied, using rapid heating-cooling cycles

with a maximum temperature of 1200ºC. The results

prove the adequacy of this material for high

temperature applications subjected to rapidly

changing thermal conditions.

MEEP 2018 - The Second International Conference on Materials Chemistry and Environmental Protection

178

ACKNOWLEDGEMENTS

This research has been partially funded by the

European Commission in the frame of the SFERA-II

project (FP7, Grant. Agreement 312643) and project

INSHIP (Integrating National Research Agendas on

Solar Heat for Industrial Processes) www.inship.eu.

REFERENCES

Belghalem, H., Hamidouche, M., Gremillard, L.,

Bonnefont, G., Fantozzi, G., 2014. Thermal shock

resistance of two micro-structured alumina obtained by

natural sintering and SPS. Ceram. Int. 40, 619–627.

Dimitrijevic, M.M., Medjo, B., Heinemann, R.J., Rakin,

M., Volkov-Husovic, T., 2013. Experimental and

numerical analysis of thermal shock damages to

alumina based ceramic disk samples. Mater. Des. 50,

1011–1018.

Douale, P., Serror, S., Duval, R.M.P., Serra, J.J., Felder, E.,

1999. Thermal shocks on an electrolytic chromium

coating in a solar furnace. Journal de Physique IV 9,

429–434.

Evans, A.G., Linzer, M., Johnson, H., Hasselman, D.P.H.,

Kipp, M.E., 1975. Thermal fracture studies in ceramic

systems using an acoustic emission technique. J. Mater.

Sci. 10, 1608–1615.

Fernandes, J.J., Rosa, L.G., 1991. Ensaios biaxiais de

cerâmicos. Actas V Encontro Nacional da Sociedade

Portuguesa de Materiais, Lisboa, Vol.1, pp 375–384,

doi: 10.13140/RG.2.1.2589.6809.

Hahn, B.S., Lee, H.L., 1999. Effect of environmental

factors on thermal shock behaviour of polycrystalline

alumina ceramics. J. Mater. Sci. 34, 3623-3630.

Hirata, Y., 2015. Theoretical analyses of thermal shock and

thermal expansion coefficients of metals and ceramics.

Ceram. Int. 41, 1145–1153.

Kováčik, J., Emmer, S., Rodriguez, J., Cañadas I., 2014.

Solar furnace: thermal shock behaviour of TiB2 coating

on steel. Proceedings of METAL 2014, pp 863–868.

Tanger Ltd, Ostrava, Czech Republic.

Lee, J.H., Park, S.E., Lee, H.J., Lee, H.L., 2002. Thermal

shock behaviour of alumina ceramics by ball-on-3-ball

test. Mater. Lett. 56, 1022–1029.

Li, D.Y., Li, W.G., Wang, R.Z., Kou, H.B., 2016. Influence

of thermal shock damage on the flexure strength of

alumina ceramic at different temperatures. Mater. Lett.

173, 91–94.

Martínez Plaza, D., 2013. Desarollo de dispositivo para

producción de energía térmica a partir de un receptor

solar de tipo volumétrico y estudio de su aplicabilidad

a procesos de alta temperatura para fabricación

industrial de cerámicas, PhD thesis, Universidad de

Sevilla.

Rauschert data sheet, 2018. Available at:

https://rauschert.com/images/documents/products/tech

nical-ceramics/Rapal_Tabelle.pdf

Saâdaoui, M., Fantozzi, G., 1998. Crack growth resistance

under thermal shock loading of alumina. Mater. Sci.

Eng. A247, 142–151.

Sallaberry, F., García de Jalón, A., Zaversky, F., Vázquez,

A.J., López-Delgado A., Tamayo, A., Mazo, M.A.,

2015. Towards standard testing materials for high

temperature solar receivers. Energy Procedia 69, 532–

542.

Thermal Cycling Behaviour of Dense Monolithic Alumina

179