Misconceptions of English Students on Education Statistic

Mutia

State Islamic Institute of Curup, Rejang Lebong District, Bengkulu Province, Indonesia

Keywords: Misconceptions, Students, Education Statistic.

Abstract: This study aims to identify the misconceptions of English students in the Education Statistics course which

consists of descriptive statistics and inferential statistics. Research subjects are students of English semester

IV class B State Islamic Institute of Curup Academic Year 2017/2018 selected by purposive sampling. Data

collection techniques use written tests, interviews, and documentation. Test of data credibility in this research

is using technique triangulation. The results showed that students’ misconception in the use of parameters and

statistics so that further difficulty in determining the use of formulas for both single data and groups data and

misconceptions in the determination of hypotheses and statistical tests are used in accordance with the

research problems given to result in the wrong research conclusions. Result of the concept of descriptive

statistics are still weak and the students also have misconception in inferential statistics, so it is important to

understand the basic concepts in descriptive statistics in order to avoid a greater misconception in inferential

statistics because the two are highly interrelated. Students who are less rigorous in the process can also be a

source of misconception of students in solving statistical problems both descriptive and inferential.

1 INTRODUCTION

Mathematics, in its very nature, is full of abstract

representations. It is a hierarchical build-up of

concepts, skills and facts. The successful learning of

mathematics involves a systematic building up of

such a hierarchy of concepts (Ruberu, 1992) and ideas

need to be understood and woven together in order for

concepts to build on one another (Ashlock, 2002).

Mathematics is the study of quantity, structure, space,

and change. Mathematicians seek out patterns,

formulate new conjectures, and establish truth by

rigorous deduction from appropriately chosen axioms

and definitions (Schleicer & Lackmann, 2011).

Mathematics is taught from concrete, semi-concrete,

to abstract, and teaches concepts from simple to

complex concepts. During the past decades, research

on statistical literacy and statistics education has

established itself as an important and rapidly growing

research field (Callaert, 2002). Statistics is one of the

branches of mathematics studied from Elementary to

High School level which has many concepts, only at

the college level, the statistics studied are more

abstract and lead to research problems. Statistics in

college is a knowledge related to ways of collecting

data, processing, presenting, analyzing, and drawing

conclusions based on data and analysis performed.

The statistics section covers the methods and methods

of collecting, presenting, processing and analyzing

descriptive data called descriptive statistics and the

part which involves drawing conclusions called

inferential statistics (Setyo Winarni & Harmini,

2011). Both statistics are different types and different

studies. However, there is interrelation between

ability in inferential statistics and descriptive

statistics: if the ability of descriptive statistics can be

well controlled then the inferential statistics would be

able to do well. Sutrisno & Murtianto (2016) argue

that the mastery of descriptive statistics is desperately

needed inferential statistics courses used in

quantitative research.

Angle (2007) explained that many mathematical

concepts can be understood only after the learner has

acquired procedural skill in using the concept. More

than often in schools, teachings of mathematics are

more focused on rules, procedures and formulas used

to arrive at the correct answers rather than teaching

students’ basic concepts. Skemp (Orton, 2004)

suggests that mathematical concepts are structured

hierarchically, one concept being the basis for other

concepts. This means that the concepts in studying the

material are interrelated, to learn a new concept must

have to master the old concept first and in learning

mathematics always happen that way. While Irawati

Mutia, .

Misconceptions of English Students on Education Statistic.

DOI: 10.5220/0008522103690377

In Proceedings of the International Conference on Mathematics and Islam (ICMIs 2018), pages 369-377

ISBN: 978-989-758-407-7

369

et al. (2014) argued that the concept or material is an

extension or deepening of the material that has been

studied. It becomes very bad if the students are more

and more teachers have a misconception or

inappropriate to a certain mathematical concept or

called misconception.

Holmes, Midema, Nieuwkoop, & Haugen, (2013)

explained that misconceptions arise from the problem

of conceptual misunderstanding. Errors come from

calculations or minor accidents. On the other hand,

Thompson & Logue (2006) describe misconceptions

as ideas that provide a misconception about such

ideas, objects, or events built on one's experience.

Dzulfikar & Vitantri (2017) also explain that the

misconception of mathematics can also be a mistake

in the application of a rule or inappropriate

generalization. When someone systematically uses

the wrong rules or uses the right rules, but is used

outside his application. (Suparno, 2013) also suggests

that misconceptions are conceptual understandings

that are inconsistent with scientific understanding or

agreement of experts in the field. Essentially,

misconceptions are different from errors. Luneta &

Makonye (2010) defined an error is a mistake, slip,

blunder or inaccuracy and a deviation from accuracy.

While, Hansen (2011) also suggest that error is a

mistake made by someone due to carelessness,

misinterpretation of the problem, lack of experience

in solving problems related to a given topic or due to

the inability to check the answers obtained.

Misconceptions and errors must not be seen as

obstacles or 'dead ends' but must be regarded as an

opportunity to reflect and learn. Teachers should

recognize these misconceptions; prescribe

appropriate instructional strategies to be more

diagnostically oriented in order to avoid any

subsequent major conceptual problems. Diagnosis

should be continuous throughout instruction

(Roselizawati, Sarwadi & Shahrill, 2014).

Based on several notions of misconception above,

it can be concluded that misconception is a mistake in

understanding concepts or concepts that are not in

accordance with the concepts that have been put

forward by experts.

Misconceptions are caused by a variety of things.

Generally can be caused by students themselves,

teachers who teach, learning context, way of

teaching, and textbook (Suparno, 2013). The

identification of misconceptions is important in order

to locate misconceptions and their causes. Salirawati

(2011) explains that to simplify the process of

identification of misconception is categorized into

three criteria that is not understanding the concept,

misconception, and understand the concept. Irawati et

al. (2014) mentions that the location of

misconceptions experienced by learners is in re-state

the concept, classify objects according to certain

characteristics in accordance with the concept,

example on a concept, using and utilizing and

selecting a particular procedure or operation and

apply the concept or algorithm on solution to

problem. If these misconceptions occur continuously,

it will result in low student learning achievement.

Andini (2012) also points out that sustainable

misconceptions if not addressed properly and

addressed early may pose problems in subsequent

learning.

The problem of statistical misconception of

Tadris English students at IAIN Curup actually

originated from the difficulties experienced by the

students. Students basically also have gained the

concept of statistics when in school. However, it is

still often found difficulties when working on the

matter of statistics. This can be caused by several

things based on the observation results of the Tadris

English students IAIN Curup academic year

2017/2018 are: 1) the student forgot and there is no

understanding of the concept he has gained at the

school level both at the primary and middle level; 2)

majoring in current study is more about the science of

English than science especially mathematics. In the

Faculty of Tarbiyah English IAIN Curup is currently

only studying the branch of mathematics is statistics

and does not study other branches of mathematics

such as basic mathematics, so it is very influential in

understanding the symbols and mathematical

notation; 3) the level of motivation and academic

ability of different students, which cannot be equated

with one another. Though studying statistics is very

important because statistics will continue to be used

until the preparation of Student Final Project in the

form of thesis. Starting from the problem, then many

students who have difficulty in learning to cause

misconception both in descriptive and inferential

statistics. Research conducted by Firmansyah (2017)

also explains the same problem in studying statistics

that students have varied learning ability statistics and

students have a tendency of negative attitudes on the

course. In another study, Maizam (2009) also

explained that the topic in descriptive statistics is the

scale of measurement, summation and presentation of

data, relationships and correlations between two

variables. Most students consider statistics to be

nothing more than numbers and formulas with limited

use in their daily lives or their future professions. In

addition, some students believe that they understand

statistics if they are able to declare and put numbers

into the wrong formula because statistics are not

ICMIs 2018 - International Conference on Mathematics and Islam

370

about inserting numbers into formulas, but a process

for obtaining information (Chance, 1997 in Rumsey

2002) and performing calculations not the same as

understanding the statistics (Gal, 2000 in Rumsey,

2002). For example, the student's ability to calculate

standard deviations does not indicate a student's

ability to understand what standard deviations and

what is measured or how they are used. In addition,

students also feel that the usefulness of educational

statistics is limited only to answering in tests and

exams. The type of Asian students, these students are

also quite passive in the classroom. Statistical

misconceptions have been observed among students

on various topics including relationships and

correlations, hypothesis testing. On the other hand,

Zaidan, Ismail, Yosuf, & Kashefi (2012) also

explained that some students exhibit misconceptions

such as averages as a mean multiple, averages as the

sum of values of variables, and averages can only be

generated from a constant value and equal to that.

Based on these matters, the researchers are

interested to follow up in the form of research.

Researchers want to identify misconceptions of

English students in the Education Statistics course

which consists of descriptive statistics and inferential

statistics. In this study, descriptive statistics are

limited to conceptual misconceptions related to data

presentation, data central tendency, and data

dispersion. While inferential statistics are limited to

misconceptions of concepts related to hypothesis

testing.

2 METHOD

This research is a research with qualitative descriptive

approach with subject 3 (three) students of fourth

semester of Tadris English Faculty of Tarbiyah IAIN

Curup academic year 2017/2018. In this research,

researcher use purposive sampling that is technique

of taking sample of data source with certain

consideration. The main instrument in this study is the

researchers themselves (Sugiyono, 2011). The

instruments used are written tests and interviews. Test

the credibility of the data using triangulation

technique that is comparing the results of written tests

with interviews.

3 RESULTS

In the methodology, the researcher used purposive

sampling in sampling of data source that is 3 (three)

students who most misconception based on written

analysis. The three subjects were given a written test

of the concept of descriptive and inferential statistics.

These three subjects are presented in the following

table:

Table 1: Research Subject.

Initial

M/F

Code

In the Table 1, the initials containing AG, DU,

and FA are the three students selected for data

collection and interviewed further to locate

misconceptions and their causes. For M/F column

indicates student's gender while the code column

indicates the student's absence number. Each

misconception will be explained in this discussion.

The following issues are used in this research

instrument:

Figure 1: Question Item.

Here are the results of the work of the three research

subjects:

1. Subject with initials AG

Figure 2: Misconception by AG about data presentation and

central tendency.

Students are seen to be able to present data in the form

of tables namely Single Data Frequency Distribution

Table. It's just that in the use of notation is still a

mistake. In the table looks



 when it should be



 because the data is presented in the form of

frequency tables that have a frequency. Here students

seem to misunderstand the concept of



 and



.



is the sum of all known data, then



 is the

sum of the multiplication of each data with a lot of

Calculate the mean, median, mode, variance, and

standard deviation from the following data:

Calculate the mean, median, mode, variance, and

standard deviation from the following data:

Misconceptions of English Students on Education Statistic

371

data (frequency). This results in a false end result.

Likewise, with the use of the average formula (mean)

and notation that should be  and not Me. This shows

that students cannot distinguish between the average

notation  and median (Me).

In addition, in the median, the student also still

looks not yet able to write and the median formula for

even data is  















 









. If the data

given more number, then the student will more

difficult to calculate. These misconceptions fall into

the concept of presentation data size and central

tendency data. As for the concept of data dispersion

can be shown in the results of students as follows:

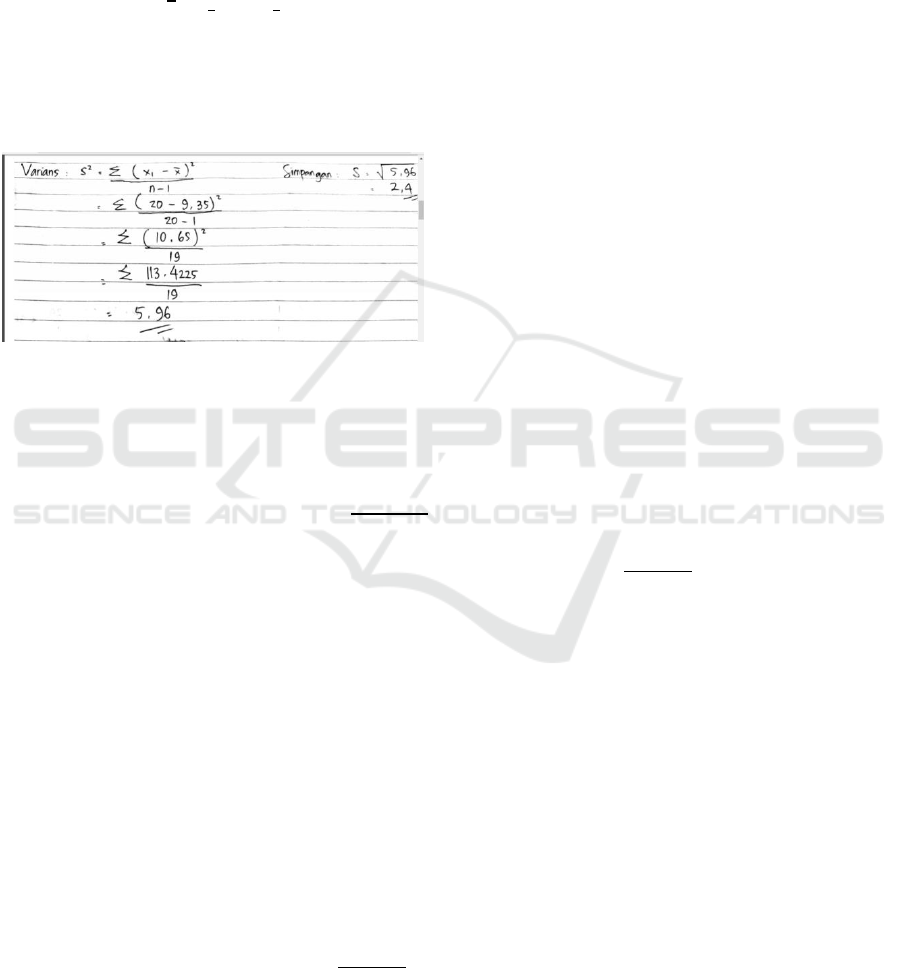
Figure 3: Misconception by AG about data dispersion.

In answer to the above variance, the student is quite

good considering the formula and write it down.

However, there is a slight disadvantage that in the

previous table students have created a frequency

distribution table, so students should write













to make it easier and simpler to complete the

calculation. For that, students should use auxiliary

tables only tables that have been made developed

again for the needs of calculation of standard

deviation in order to facilitate the calculation of the

difference in data with the average which then

squared and facilitate multiplication with the

frequency.

Then, the answer also shows that students are

wrong in entering data. The student enters the value

of n = 20 (n=



 into the value of x in the formula

when it should be x each is the data in the table, for

example x

=1, x

= 2, etc., which is then summed, by

therefore, it is necessary to multiply with frequency

to facilitate calculation. Students also do not

understand the sign of sigma in the formula, so at the

end of the settlement gives wrong results. This gives

researchers predictions to students that students have

not understood the concept of using the













If

this continues, it leads to continuous errors when

calculating statistical tests that require the calculation

of variance and standard deviation.

Based on the student's conversation with the

researcher about the above mistakes, the following

information is obtained:

Students realize not yet understand the use of

notation



 and



, whereas the intended by the

student is wanted to sum up the data by multiplying

the data known by the number of frequencies each

data. However, finally just add the data in the left

column (figure 1) i.e.



 =187, when it should be



  . Students do not realize that for data x

=2 it has a frequency of 5 and so on. As for the use

of the symbol of the average, it is due to forget.

Visible then students write back / justify the simbol 

before the formula.

For writing the median symbol, students explain

forgetting to write the symbol. As for the use of the

formula, the student realizes it does not remember and

does not understand the use of the median formula

that has been given during the lecture. As a result, do

not understand the concept, then be do not remember.

At the completion of the variance, the student

realizes that he does not understand the settlement

procedure. When entering the value of x, the student

does not understand the value of x which will be

included in the formula so that finally choose to enter

the value of n. During this time students are

accustomed to memorizing the formula given,

without understanding how to use it. Whereas in the

frequency distribution table, has written



, where

the data symbolized by x. In addition, the student is

also unaware that the formula should be used by using

frequency multiplication by the difference of data

then squared i.e..,













because the frequency is

already known in the table, so it is not necessary sum

up each difference in data with more than one

frequency.

2. Subject with initials DU

For explanation of picture 4. In student initials DU,

basically have been able to arrange settlement

procedure systematically and count well. However, it

is a mistake to write a notation for the number of N

sample sizes that should be n, even if the value

entered is the same. The difference is about where the

data comes from, whether population or sample. In

the use of notation, the average student is also still

inconsistent with the notation that has been agreed by

the expert that is . In addition, the students at DU are

also unable to present data with frequency

distribution tables in either single data or group data.

ICMIs 2018 - International Conference on Mathematics and Islam

372

Figure 4: Misconception by DU about central tendency.

Basically, students not only learn about the

centralization of data, but also about the location of

data. In the student's reply, the student understands

the location of the median in the location of quartile

data 2 or symbolized by Q

. Quartiles are a placement

measure that divides a data group into four equal parts

(Subana, Rahadi, & Sudrajat, 2000). Namely Q

, Q

and Q

. The location of Q

is the position in the

middle of the data that divides the data into two parts.

In the answer is seen students divided the data into

two equal parts. Thus, the median value is written as

the sum and division of the two data in the middle

position. In fact, in the central tendency, has written

the median formula for even and odd data so that

students can more easily solve it. Worrying if the data

provided in large numbers, students will have

difficulty completing it even if it gives the same

results. The formula for even n is   







and

for  odd 















 









. Researchers suspect

students find it difficult to use the formula that is to

understand the notations and enter the values.

Figure 5: Misconception by DU about data dispersion.

In the student answer above, it appears that the

student is not perfect in solving the problem that is

not writing the formula first. At the completion, it was

seen that students entered n only and not n-1. Even

though it should use n-1 because this is the sample

data where













. Not only that, the calculations

(60-11,35)

also experience errors so that the sigma

(addition) of the difference and the square becomes

wrong. As for writing the standard deviation formula

is good is the square root of the value of variance.

However, the final value is wrong because the value

of the variance is also incorrect. Therefore, the

calculation of the variance must be more precise so as

not to result in the standard deviation value. Here it

needs a good understanding of the concept to avoid

errors in the next.

On the subject of initials DU, obtained interview

results as follows:

a. Students understand the concept of calculating the

average of summing all the data then divided by a

lot of data and students also understand the

difference of parameters and statistic that is N and

n. However, the student is aware of his mistake in

writing the notation for the number of data sizes

that should use the n notation as it is the sample

data.

b. After being interviewed about presentation of data

in the table form. Students admitted better without

using tables. However, when given more data,

students are aware of the difficulty of counting.

Moreover, calculate median for even and odd

data. Students unable to remember the median

formula well and students also do not understand

the use of the formula. Students understand that

median location is when the data is divided into

two equal parts or called middle value.

c. Students realize less accurate in calculating and

less thoroughly using the formula that should be

n-1 but written into n. This is because students

rush, but in paper graffiti has written the formula

using n-1.

3. Subject with initials FA

Figure 6: Misconception by FA about central tendency.

In the above point about the central tendency, the

student has been able to calculate the mean (mean) of

a data, although it does not use the formula agreed by

the experts that  







. Likewise, with the median

Misconceptions of English Students on Education Statistic

373

value (Me), it appears that it does not use a mutually

agreed formula. Researchers suspect students still do

not understand in the use of these formulas. In

addition, in the use of notation / symbol for the

average, students also cannot write well. It can be

seen from the way students write that writing x, it

should be  For presentation data, students also can

not present data in the table so that the written

procedure is not systematic.

Figure 7: Misconception by FA about data dispersion.

When viewed from the student answers above, it can

be explained that the students are not understanding

the concept. Here also seen students do not use

textbooks but using the formula from google. In

writing the notation for the formula is not very clear







So the settlement procedure is not clear.

While the final result is correct. So, need further

confirmation to the subject of research about it

through interview.

Based on the mistakes made by the students, then

obtained confirmation between the researchers and

students initials FA as follows:

a. Students realize that they are weak in

remembering and using existing formulas which

should be used to calculate the average and

median.

b. On the variance error, the student realizes not

memorizing the variance formula and does not

understand the use of the formula. When it was

confirmed why the answer was correct and the

process was wrong, the student testified that the

answer was his friend's reply. Students are also

even difficult to read his own writing that is not

neat and unstructured.

In general, if simple concepts in descriptive

statistics are not well understood, it will affect the

completion of inferential statistical questions such as

the following :

a. In a student with the initials AG, there are

misconceptions such as error in using



 and



 not notation. This misconception is not

actually bad because it actually contains the same

meaning, only when the data is presented in the

table and the frequency is not counted, it will

produce a seriously error. Because it will result in

the mean calculation and then will result in the use

of the hypothesis test formula that is t test to see

the average, t =















with degrees of freedom n-

1, which in the use of the formula requires an

average value. If the incorrect value entered is

incorrect, the calculated  test value is also false

and then on hypothesis testing will lead to a false

conclusion that is rejected or accepted based on

the t

count

and t

table

values.

The following is the description of the student's

answer which caused misconception in the work on

the matter of



and hypothesis test (in figure 7).

After interviewed, the students gave information that

initially when working the students are still confused

to calculate the amount of data using that frequency



, so the results obtained are different from

what is written when calculate the average that is 811.

But then the students re-confirm the correct answer is



   because there is one data having

frequency 2 that is value 81. Thus, it needs to be

added back 811 and 81 so that it becomes 892, then

the average calculation then become true and on the

calculation of t test it also corrects.

Figure 8: Answer of student in solving hypothesis test

problem.

a. In students with initials DU, there are

misconceptions in the use of parameters and statistics

are N and n. The difference is about where the data

comes from, whether population or sample. Such an

error then continues while working on the inferential

ICMIs 2018 - International Conference on Mathematics and Islam

374

statistics problem, the student still uses the N notation

that shows the population data (as in figure 9).

Figure 9: Answer of student in solving hypothesis test

problem.

b. In students with the initials FA, there is a

misconception in remembering and using

formulas. This is due to material that has not been

well mastered, incomplete understanding of a

concept, and neat and structured writing

difficulties.

In this research, we will look for suitability of

written test analysis with interview result based on

technological triangulation such as:

a. Misconception in the use and development of help

tables is calculated as when calculating

multiplication between data x and its frequency f

and when calculating variance.

b. Students experience misconception on the use of



 and



 not notation. Basically, both

formulas have the same meaning, but it becomes

fatal when the frequency is not included in the

calculation. Not only that, on the use of the

formula













, the students also still have

difficulties.

c. Misconception determines the use of parameters

and statistics according to the origin of given data

such as n and N, then n and n-1, which then leads

to a miscalculation.

d. Misconception in entering x values, calculating

data differences, and so on.

e. Misconceptions in the use of average symbols,

medians, variance, and standard deviations such

as , Me, Var, s

, etc.

f. Misconceptions in remembering formulas and

using formulas so often have difficulty when

using formulas.

g. In inferential statistics, students still make many

mistakes in calculations looking for variance and

standard deviation, determine hypotheses, and test

hypotheses, and conclusions. In the hypothesis

determination, many students still do not

understand how to write more than () and less

than (). This is as a result of the students not

getting basic mathematics in previous lectures, so

it cannot distinguish mathematical notation and

cannot write what is meant in the matter of

hypotheses to be proved (e.g. wanting to prove the

average hypothesis of educational statistics value

is more than 70). While the misconception that

occurs when testing the hypothesis is a

misunderstanding in determining the statistical

test used and the use of these formulas involving

statistics in descriptive statistics. Thus, if the

concept of descriptive statistics is weak, then the

process of hypothesis testing and the conclusion

of students will experience a misunderstanding.

Misconceptions and learning Mathematics is a

common occurrence different group (Mulungye M. et

al., 2016). As an effort to overcome the problem of

misconception that occurs in mathematics is to do

remedial. They explained that teachers’ knowledge

on students’ errors was investigated together with

strategies for remedial teaching. Their studies also

showed that teachers need assistance not only in error

identification but also how the errors would be built

in the whole process of learning.

4 CONCLUSIONS

Statistics in college is a knowledge related to ways of

collecting data, processing, presenting, analyzing,

and drawing conclusions based on data and analysis

performed. The statistics section includes methods

and ways of collecting, presenting, processing and

analyzing descriptive data called descriptive statistics

and parts which include drawing conclusions called

inferential statistics. Both statistics are different types

and different studies. However, interrelated where

inferential statistics would be able to do well if

descriptive statistics can be well controlled.

Misconception is an error in understanding a concept

or concept that is not in accordance with the concepts

that have been put forward by experts.

Misconceptions are caused by a variety of things. In

general, it can be caused by students themselves,

teachers who teach, learning contexts, teaching

methods, and textbooks. The identification of

misconceptions is important in order to locate

misconceptions and their causes because sustained

misconceptions if not addressed properly and

resolved early may pose problems in subsequent

learning. Misconceptions in educational statistics

consist of misconceptions in the use and development

of help tables of calculations, misconceptions in the

use of



 and



 notations, misconceptions in

determining the use of parameters and statistics,

Misconceptions of English Students on Education Statistic

375

misconceptions in entering x values, calculating data

differences, misconceptions in using symbols

average, median, variance, and standard deviation,

misconceptions in remembering formulas and using

formulas so that they often experience difficulties

when using formulas, in calculating the search for

variance and standard deviation, determining

hypotheses, and testing hypotheses, and conclusions.

The misconception of educational statistics is

basically the mistakes that students have made when

solving statistical problems related to data

summation, data presentation, and so forth. However,

it cannot be entirely the student's mistake, because

misconception can be caused by several things such

as teacher / lecturer, student / student, learning

context, model / learning method, and textbook or

other learning resources provided by the teacher /

lecturer. In this study, misconceptions occur as a

result of students' desire to learn and try hard to solve

statistical problems are still very low, agree with the

statement expressed by (Maizam, 2009) that most

students consider statistics to be no more than

numbers and formulas with limited use in everyday

life. Furthermore, the lecturer's model / method also

needs to be reflected in order to improve the mindset

of the students in solving the statistical problems. The

use of various textbooks or learning resources also

needs to be agreed upon to make no difference in the

use of notations and formulas and provide a good

understanding of the basic concepts of notations and

formulas.

ACKNOWLEDGEMENT

I would like to thank Rector of State Islamic Institute

of Curup and Vices of Rector in the institute. Thanks

should also be bestowed upon Reviewer, who

conscientiously reviewed the abstract, introduction,

and finally this paper. And also would like to thank

my family for the constants support.

REFERENCES

Andini, D., 2012. Miskonsepsi Siswa dalam Mata Pelajaran

Matematika di Sekolah Dasar. Retrieved from

https://www.academia.edu/9746128/Miskonsepsi_Mat

ematika_Siswa_Sekolah_Dasar

Angle, D., 2007. What is conceptual understanding?

Retrieved from https://www.maa.org/external_archive/

devlin/devlin_09_07.html

Firmansyah, M. A., 2017. Analisis Hambatan Belajar

Mahasiswa pada Mata Kuliah Statistika. Jurnal

Penelitian dan Pembelajaran Matematka, 10(2), 115–

127. http://dx.doi.org/10.30870/jppm.v10i2.2036

Ashlock, R. B., 2006. Error Patterns in Computation: using

Error Patterns to Improve Instruction. New Jersey:

Pearson Merrill Prentice Hall.

Callaert, H., 2002. Understanding Statistical

Misconceptions. ICOTS6, 1-4.

Dzulfikar, A., & Vitantri, C. A., 2017. Miskonsepsi

Matematika pada Guru Sekolah Dasar. Suska Journal

of Mathematics Education, 3(1), 41–48.

Hansen, A., 2011. Children Errors in Mathematics:

Understanding Common Misconceptions in Primary

School. Exeter: Learning Matters.

Holmes, V-L, Miedema, C., Nieuwkoop, L., & Haugen, N.,

2013. Data-Driven Intervention: Correcting

Mathematics Students’ Misconception, not mistakes.

Journal of Mathematics Educator, 23(1), 24–44.

Irawati, R., Indiati, I., & Shodiqin, A., 2014. Miskonsepsi

Peserta Didik dalam menyelesaikan Soal pada Materi

Relasi dan Fungsi Kelas VIII Semester Gasal SMP

Negeri 4 Kudus. Prosiding Mathematics and Sciences

Forum, pp. 805-812. Retrieved from

http://prosiding.upgris.ac.id/index.php/masif2014/masi

f2014/paper/viewFile/511/451.

Luneta, K., & Makonye, P. J., 2010. Learners Errors and

Misconceptions in Elementary Analysis: A Case Study

of a Grade 12 in South Africa. Acta Didactica

Napocensia, 3(3), 35–46.

Maizam, A., 2009. Integrating Technology into Classroom

Instructions for Reduced Misconceptions in Statistics.

International Electronic Journal of Mathematics

Education, 4(2), 77–91.

Mulungye M., M., O‘Connor, M., & Ndethiu S., 2016.

Sources of Student Errors and Misconceptions in

Algebra and Effectiveness of Classroom Practice

Remediation in Machakos County- Kenya. Journal of

Education and Practice, Vol.7 (10), 31-33.

Orton, A., 2004. Learning Mathematics: Issues, Theory and

Classroom Practice (3 edition). London: New York

Continuum.

Roselizawati, Sarwadi, & Shahrill, M., 2014.

Understanding Students’ Mathematical Errors and

Misconceptions: The Case of Year 11 Repeating

Students. Mathematics Education Trends and

Research, Vol 2014, Article ID metr-00051,

https://doi.org/10.5899/2014/metr-00051.

Ruberu, J., 1992. How Mathematical Concepts are

Understood and Misunderstood. Science and

Mathematics Education, 1(2), 2–6.

Salirawati, D., 2011. Pengembangan Instrumen Pendeteksi

Miskonsepsi Kesetimbangan Kimia pada Peserta Didik

SMA. Jurnal Penelitian dan Evaluasi Pendidikan,

15(2), 232–249.

Schleicer, D., & Lackmann, M., 2011. An Invitation to

Mathematics: from Competition to Research. Retrieved

from www.springer.com/978-3-642- 19532-7.

Winarni, E. S., & Harmini, S., 2011. Matematika Untuk

PGSD. Bandung: PT Remaja Rosdakarya.

Subana, M., Rahadi, M., & Sudrajat, 2000. Statistik

Pendidikan. Bandung: Pustaka Setia.

ICMIs 2018 - International Conference on Mathematics and Islam

376

Sugiyono, 2011. Metode Penelitian Kuntitatif, Kualitatif,

dan R&D. Bandung: Alfabeta.

Suparno, P., 2013. Miskonsepsi dan Perubahan Konsep

dalam Pendidikan Fisika. Jakarta: Gramedia

Widiasarana Indonesia.

Sutrisno, & Murtianto, Y. H., 2016. Miskonsepsi

Mahasiswa pada Mata Kuliah Statistika Deskriptif

Materi Ukuran Tendensi Sentral, Ukuran Dispersi, dan

Ukuran Letak. Media Penelitian Pendidikan: Jurnal

Penelitian dalam Bidang Pendidikan dan Pengajaran,

10(1 JUNI). https://doi.org/10.26877/mpp.v10i1

JUNI.1256

Thompson, F., & Logue, S., 2006. An Exploration of

Common Student Misconception in Science.

International Education Journal, 7(4), 553–559.

Zaidan, A., Ismail, Z., Yosuf, Y. M., & Kashefi, H., 2012.

Misconceptions in Descriptive Statistics among

Postgraduates in Social Sciences. Procedia - Social and

Behavioral Sciences, 46, 3535 – 3540.

Misconceptions of English Students on Education Statistic

377