
Transmission-Reflection Wave on Curtainwall Pile Breakwater with
and without Bottom Protection
Subekti
1,3
, Nur Yuwono
2
and Suseno Darsono
3
1
Department of Civil Engineering, Universitas Sultan Ageng Tirtayasa, Banten, Indonesia
2
Department of Civil Engineering, Universitas Gadjah Mada, Yogyakarta, Indonesia
3
Department of Civil Engineering, Universitas Diponegoro, Semarang, Indonesia
Keywords: Transmission wave, Reflection wave, Curtainwall pile breakwater, Bottom protection
Abstract: The quay is ideally free from wave interference so ships can dock to rise and fall of passengers or loading and
unloading goods safely and comfortably. The harbours that open directly to the ocean without being protected
by breakwaters are quite vulnerable to unfriendly weather when very high wind speed occurs high sea waves
rise very high can lead to the process of relying on the ship on rise and fall of passengers or loading and
unloading goods cannot be done safely and comfortably. Generally the size of the gravitational breakwater
increases with the depth of the water, starting from the base of a large foundation and requiring an enormous
amount of construction material if it is built in deep water, so this breakwater type are expensive in deeper
water.The curtainwall pile breakwater is one of partially barrier breakwater types, that requires less concrete
per-unit run and capable of transmitting less wave energy. Research on curtaianwall-pile breakwater (CPB)
and the same types has been done by many researchers, but there is no research on CPB equipped with
protection base to protect the pile from wave scouring. The experimentally study has be done in wave flume
with physical model. The size of the wave flume are length 15 meters, depth 0.45 meters, and wide 0.30 meter
that equipped with wave maker, damper, and wave probe. The purpose of study investigate transmission wave
coefficient (K
) and reflection wave coefficient (K
) CPB effect of the bottom protection with low rubble-
mound. Based on the result of study is known that the curtainwall pile breakwater (CPB) with bottom
protection give transmission wave coefficient (K
) more less (decrease value: 0.04 – 0.22) than CPB without
bottom protection and give reflection wave wave coefficient (K
) larger (increase value 0.07) depend value
of h/d. The generally curtainwall pile breakwater (CPB) without bottom protection, if the value h/d and H/L
increase, transmission wave coefficient (K
) will decrease and reflection wave coefficient (K
) will increase.
The value K
= 0.91 – 0.42 and K
= 0.26 – 0.65 depend h/d and H/L.
1 INTRODUCTION
There are 2 types of breakwaters, namely full
protection breakwaters and partial protection
breakwaters (Ahmed, 2011). Full protection
breakwaters such as rubblemound, caisson, and
combination breakwaters, while partial protection
breakwaters such as submerged breakwaters,
detached breakwaters, pipe breakwaters, floating
breakwaters and peneumatic breakwaters. Gravity
breakwaters that use upright stacks of rocks or
caisson are often used to obtain a calm pond for ships
and protect port facilities from sea wave attacks. In
general, the size of gravity breakwaters increases with
the depth of water, starting from the bottom of large
foundations and requiring a very large amount of
construction material if built in deep waters (Suh et
al., 2006 and 2007). The construction of gravitational
breakwaters becomes very expensive with increasing
water depth (size and volume increases) and the width
of the pond also decreases with the size of the
structure. Gravity breakwater cannot be built on soft
soils, where hard soil structures are deep enough.
One solution to the problems is the curtainwall
pile breakwater (CPB) or pile support skirtwall
breakwater (PSSB). The basic concept of CPB is to
become a wave barrier around the surface area where
the movement of water particles is larger, while the
bottom is unobstructed (slot). Pile support structure
of breakwater allows through outflow so that water
circulation is very good and fish and organisms can
go in and out through the CPB so that this type of
Subekti, ., Yuwono, N. and Darsono, S.
Transmission-Reflection Wave on Curtainwall Pile Breakwater with and without Bottom Protection.
DOI: 10.5220/0009007201170124
In Proceedings of the 7th Engineering International Conference on Education, Concept and Application on Green Technology (EIC 2018), pages 117-124
ISBN: 978-989-758-411-4
Copyright
c
2020 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved
117

breakwater is environmentally friendly. In soft soil
with deep hard soil conditions, curtainwall pile
breakwater (CPB) can be an alternative allaternative
(Laju et al., 2011). There are many Research on the
wall-pile breakwater (CPB) and the same types have
been done by many : Koraim (2015), Ahmed and
Schlenkoff (2014), Zang and Li (2014), Najedkazem
and Gharabi ( 2012), Laju et al. (2011), Liu and Li
(2011), Rageh et al. (2009), Ji and Suh (2008), Suh et
al. (2006 & 2007), and Nelami and Rajendran (2002).
Najedkazem and Gharabi (2012) proposed a
coefficient of friction consisting of hydrodynamic
characteristics for estimation. Liu and Li (2011)
examined the hydrodynamic performance of the CPB
with double walls to obtain reflection, transmission
and wave force coefficients. The study of Ji and Suh
(2008) conducted a study with irregular waves on
many wall CPB in order to obtain reflection and
transmission wave coefficients. There is no reseach
CPB. There has been no previous studies on CPB
combined with bottom protection.
The purpose of this study investigate of the
reflection wave coefficient value (K
) and he
reflection wave coefficient value (K
) of CPB
combination botton protection is compared to CPB
without bottom protection with CPB function from
relative curtainwall depth (h/d) and wave steepness
(H/L).
Sea waves do not transfer mass, but transfer
energy. The rate of energy transport is called energy
flux (F). Waves propagate through barriers such as
curtainwall pile breakwaters (CPB), some of their
energy will be reflected by the barrier, some will be
forwarded to the rear of the structure through the
structural gap and some will be damped. Generally,
the concept of flux energy (F) which attack
curtainwall pile breakwater (CPB) is illustrated in
Figure 1 and a description of wave flux energy (F can
be shown in equation 1 and equation 2, according to
the concept of Paotonan and Yuwono (2011).
Figure 1: Definition sketch.
Where, E =
..
; n =
1
; k=
; E=
wave energy, H= wave heigt, C= wave propagation,
T= wave period and L= wave length.
=
+
+
then
=
– (
+
)
(2)
=
..
-
..
..
(3)
=1 –
(4)
= 1 – (
+
) or
= 1 -
(5)
Where,
,
,
,
,
dan
successively
are incident wave height, reflection wave height,
transmission wave height, energy dissipation
coefficient, reflection energy coefficient,
transmission energy coefficient and
,
,
equation can be seen in Equation 6-8.
=
(6)
=
(7)
= 1- (
+
)
(8)
The incident wave attack the barrier of partial
protection, the reflection wave height is smaller than
the height of the incident wave height. The reflected
wave partial protection, which is characterized by
upper envelope and lower envelope of the wave.
Sketch envelop wave concepts of Dean and
Dalrymple (1999) can be illustrated in Figure 2.
Figure 2: Envelope in a partial standing wave system.
The incident wave height (
) and the reflection
wave wave (
) in the reflection wave that atact the
partial protection breakwater, where the distance
between
and
is ¼ L (Dean and Dalrymple,
1) is defined Equation 9 and 10.
=
(9)
=
(10)
EIC 2018 - The 7th Engineering International Conference (EIC), Engineering International Conference on Education, Concept and
Application on Green Technology
118

Figure 3: Sketch of experimental setup.
(a)
(b)
Figure 4: CPB: a. CPB without bottom protection a. CPB with bottom protection.
According to the concept of Koraim (2015) to find
the value of
as far as L (for example waves
when being modeled at the peak, at the same time the
wave reaches the peak, as far as one wavelength) and
is obtained at the position ¼ L of the reflected
wave (as far as 1.25L from the physical model).
2 RESEARCH METHODOLOGY
This research is an experimental research using
physical models in a wave flume laboratory equipped
with wave generators and wave dampers. Wave
height recording uses a wave probe equipped with
WTM (wave tide meter) and a computer to get the
wave height. The wave flume sketch and the laying
of the wave probe are shown in Figure 3.
The wave flume used in the study was 30 cm
wide, 45 cm high, 15 m long and a water depth at the
study of 20 cm. WP-1 (wave probe-1) is used to
measure the incident wave height that be generated
from wave generator, WP-2 and WP-3 measure the
incident wave height and reflection wave height, and
WP-4 measures the transmission wave height. Wave
probe placement: WP-1 is located between the wave
generator and the model for measuring the incident
wave height, WP-2 and WP-3 are placed in front of
the model with distances as far as L and 1.25 L to
measure the reflection wave height (mixed wave
height at H_max and H_min) as in the Koraim (2015)
study, and WP-4 was placed in back beetwen model
and wave damper to measure transmission wave
height.
The depth of curtainwall (h/d) range 0-0.70 of
curtainwall pile breakwater (CPB) was combinated
with bottom protection (t/d = 0.20) and CPB without
bottom protection (t/d= 0). Value h/d CPB
respectively 0 (base curtainwall position on SWL),
0.10, 0.30, 0.50 and 0.70 below surface water level
(SWL). Waves generated at the wave flume are
regular waves with wave steepness (H/L) range
0.0097 - 0.0285. The wall material of the CPB model
is acrylic, piles of CPB model are round woods, while
the bottom of the pile is split with size: 5-10 mm as
shown in Figure 4.
Dimensional analysis of transmission height wave
(
) and transmission height wave (
) on CPB with
Transmission-Reflection Wave on Curtainwall Pile Breakwater with and without Bottom Protection
119

Figure 5: Effect of relative curtainwall depth on
(t/d=0).
bottom protection
= ƒ (h, t, d, T,
, g) and
= ƒ
(h, t, d, T,
, g) with dependent parameter is
or
, the independent parameters are h, t, d, T, dan
,
others parameter is g.
Based on result dimensioanal analysis, the
formula is shown in Equation 11 and 12 respectively.
=
= ƒ(H/L, h/d, t/d) or
=
= ƒ(
, h/d, t/d)
(11)
=
=ƒ(H/L h/d, t/d) or
=
= ƒ(
, h/d, t/d)
(12)
In this study it that CPB for function of bottom
protection height (t/d) is CPB with bottom protection,
just one bottom protection condition (t/d=0.20).
3 RESULTS AND ANALYSIS
In this study the parameters that be reseached are
reflection coefficient value (
) and transmission
coefficient value (
) of curtaianwall pile breakwater
(CPB) without bottom protection (t/d = 0) and
curtaianwall pile breakwater (CPB) with bottom
protection function of relative curtainwall depth (h/d)
and wavesteepness (H/L).
Transmission coefficient (
) value of
curtaianwall pile breakwater (CPB) without the
bottom protection (t/d= 0) function of relative
curtainwalldepth (h/d) and wave steepness (H/L)
shown on Table 1, while the graph of effect of relative
curtainwall depth (h/d)
shown in Figure 5.
Table 1: Transmission wave coefficient (K_t) function from
h/d and H/L (t/d=0).
H/L
h/d
0 0.10 0.30 0.50 0.70
0.0097 0.93 0.85 0.90 0.79 0.68
0.0170 0.88 0.86 0.80 0.73 0.62
0.0285 0.84 0.83 0.54 0.52 0.38
Equation line is resulted statistical analysis using
the SPSS program with multivariate non-linear data
on Table 1 shown on Figure 5.
The reflection wave coefficient (
) of
curtaianwall pile breakwater (CPB) without bottom
protection (t/d= 0) on function of the relative
curtainwalldepth (h/d) and wavesteepness (H/L) are
displayed on Table 2, while the graph of the
relationship to the function h/d and H/L is shown in
Figure 6.
Table 2: Reflection wave coefficient (
) fuction of h/d and
H/L (t/d=0).
H/L
h/d
0 0.10 0.30 0.50 0.70
0.0097 0.26 0.33 0.34 0.40 0.58
0.0170 0.32 0.32 0.35 0.40 0.60
0.0285 0.32 0.35 0.44 0.55 0.78
0.00
0.20
0.40
0.60
0.80
1.00
0.00 0.20 0.40 0.60 0.80 1.00
Kt
h/d
Transmission
H/L=0.00970
H/L=0.01697
H/L=0.02848
EIC 2018 - The 7th Engineering International Conference (EIC), Engineering International Conference on Education, Concept and
Application on Green Technology
120
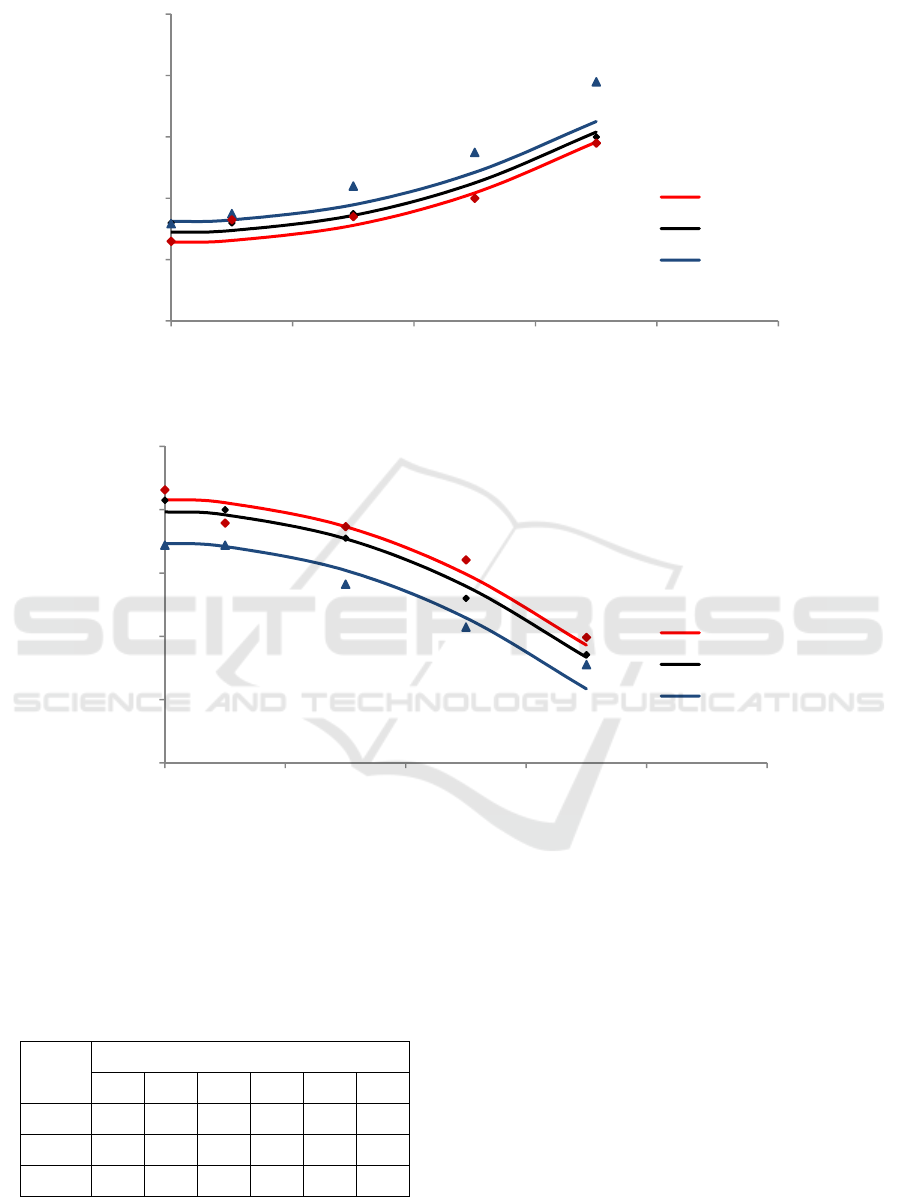
Figure 6: Effect of relative curtainwall depth on
(t/d=0).
Figure 7: Effect of relative curtainwall depth on
(t/d=0.20).
Equation line statistical analysis using the SPSS
program with multivariate non-linear data on Table 2
shown on Figure 6.
Table 3: Reflection wave coefficient (
) fuction of h/d and
H/L (t/d=0.20).
H/L
h/d
0 0.10 0.30 0.50 0.70 0.80
0.0097
0.86 0.76 0.75 0.64 0.40 0.19
0.0170
0.83 0.80 0.71 0.52 0.34 0.17
0.0285
0.69 0.69 0.56 0.43 0.31 0.09
The transmission coefficient (
) value of
curtaianwall pile breakwater (CPB) with the bottom
protection (t/d = 0.20) function of relative curtainwall
depth (h/d) and wavesteepness (H/L) shown on Table
3, while the graph of the
relationship to the
function h/d and H/L is shown in Figure 7.
Equation line statistical analysis using the SPSS
program with multivariate non-linear data on Table 3
shown on Figure7.
The reflection wave coefficient (
) of
curtaianwall pile breakwater (CPB) with the bottom
protection (t/d= 0.20) on function of the relative
curtainwall depth (h/d) and wavesteepness (H/L) are
displayed on Table 4, while the graph of the
0.00
0.20
0.40
0.60
0.80
1.00
0.00 0.20 0.40 0.60 0.80 1.00
Kr
h/d
Reflection
H/L=0.00970
H/L=0.01697
H/L=0.02848
0.00
0.20
0.40
0.60
0.80
1.00
0.00 0.20 0.40 0.60 0.80 1.00
Kt
h/d
Transmission
H/L=0.00970
H/L=0.01697
H/L=0.02848
Transmission-Reflection Wave on Curtainwall Pile Breakwater with and without Bottom Protection
121

Figure 8: Effect of relative curtainwall depth on
(t/d=0.20).
relationship to the function h/d and H/L shown in
Figure 8.
Table 4: Reflection wave coefficient (
) fuction of h/d and
H/L (t/d=0.20).
H/L
h/d
0 0.10 0.30 0.50 0.70 0.80
0.0097
0.24 0.35 0.37 0.44 0.57 0.73
0.0170
0.32 0.35 0.38 0.52 0.77 0.80
0.0285
0.26 0.38 0.46 0.60 0.76 0.83
Equation line statistical analysis using the SPSS
program with multivariate non-linear data on Table 4
shown on Figure 8.
Based on Figure 5 graph effect of relative
curtainwall depth (h/d) on transmission wave
coefficient (K
) of CPB without bottom protection
obtained that the transmission wave coefficient (K
)
decrease with increasing relative curtainwall depth
(h/d) and wave steepness (H/L). The transmission
wave coefficient (K
) value ranges from 0.91-0.42
depending on relative curtainwall depth (h/d) and and
wave steepness (H/L). The decreasing in transmission
wave coefficient (K
) ranges from 0-0.32 depending
on relative curtainwall depth (h/d). The transmission
wave coefficient (K
) value decrease with increasing
relative curtainwall depth (h/d) which curtainwall
deeper causes the slot height of CPB to be reduced by
increasing curtainwall causing the wave's ability to
pass CPB that become transmission wave decreases
and transmission wave coefficient (K
) value
decreases. If the wave steepness (HL) increases,
increasing elevation wave peak and wave height
while fixed slot height, thus incident wave pass
through CPB that become transmission wave
decrease and transmission wave coefficient (K
)
value decrease.
Based on Figure 6 graph effect of relative
curtainwall depth (h/d) on reflection wave coefficient
(K
) of CPB without bottom protection obtained that
reflection wave coefficient (K
) decrease with
increasing relative curtainwall depth (h/d) and wave
steepness (H/L). The reflection wave coefficient (K
)
value ranges 0.26-0.65 depending on the value
relative curtainwall depth (h/d) and wave steepness
(H/L). The increasing in the reflection wave
coefficient (K
) value ranges 0-0.33 depending on the
relative curtainwall depth (h/d). The increasing
relative curtainwall depth (h/d) so that curtainwall) of
CPB becomes the wave barrier increase will
increasing reflected wave. The wave steepness (H/L)
increases, increasing elevation wave peak and wave
height while fixed slot depth of CPB below the
curtainwall, thus the incident wave (H
) passes
through CPB will be reflected wave increase and
reflection wave coefficient (K
) increase.
Based on Figure 7 graph effect of relative
curtainwall depth (h/d) on transmission wave
coefficient (K
) of CPB with bottom protection
(t/d=0.20) obtained that the transmission wave
coefficient (K
) decrease with increasing relative
curtainwall depth (h/d) and wave steepness (H/L).
The transmission wave coefficient (K
) value ranges
from 0.83-0.23depending on relative curtainwall
depth (h/d) and and wave steepness (H/L). The
decreasing in transmission wave coefficient (K
)
0.00
0.20
0.40
0.60
0.80
1.00
0.00 0.20 0.40 0.60 0.80 1.00
Kr
h/d
Reflection
H/L=0.00970
H/L=0.01697
H/L=0.02848
EIC 2018 - The 7th Engineering International Conference (EIC), Engineering International Conference on Education, Concept and
Application on Green Technology
122

ranges 0-0.46 depending on relative curtainwall depth
(h/d). The transmission wave coefficient (K
) value
decrease with increasing relative curtainwall depth
(h/d) which curtainwall deeper causes the slot height
of CPB to be reduced by increasing curtainwall
causing the wave's ability to pass CPB that become
transmission wave decreases and transmission wave
coefficient (K
) value decreases. If the wave steepness
(HL) increases, increasing elevation wave peak and
wave height while fixed slot height, thus incident
wave pass through CPB that become transmission
wave decreas and transmission wave coefficient (K
)
value decrease.
Based on Figure 8 graph effect of relative
curtainwall depth (h/d) on reflection wave coefficient
(K
) of CPB with bottom protection (t/d=0.20)
obtained that reflection wave coefficient (K
)
decrease with increasing relative curtainwall depth
(h/d) and wave steepness (H/L). The reflection wave
coefficient (K
) value ranges 0.26 - 0.72 depending
on the value relative curtainwall depth (h/d) and wave
steepness (H/L). The increasing in the reflection wave
coefficient (K
) value ranges 0-0.39 depending on the
relative curtainwall depth (h/d). The increasing
relative curtainwall depth (h/d) so that curtainwall) of
CPB becomes the wave barrier increase will
increasing reflected wave. The wave steepness (H/L)
increases, increasing elevation wave peak and wave
height while fixed slot depth of CPB below the
curtainwall, thus the incident wave (H
) passes
through CPB will be reflected wave increase and
reflection wave coefficient (K
) increase
Transmission wave coefficient (
) of CPB with
bottom protection (this study t/d=0.20 ) when be
compared with transmission wave coefficient (
) of
CPB without bottom protection increase than
transmission wave coefficient (
) of CPB without
bottom protection. The increasing of the transmission
wave coefficient (
) value of CPB with the bottom
protection ranges 0 - 0.07 depending on the value of
relative curtainwall depth (h/d), shown in Figure 5
and Figure 7. Equation line statistical result analysis
using the SPSS program with multivariate non-linear
data on Table 3 shown on Figure 7, defined equation
13.
0.935
.
160.23
.
0.851
(13)
with
= 0.979 and
is determination
coefficient, where
is influenced on h/d dan H/L
parameters by 97.9 % and the equation line shown on
Figure 7
Reflection wave coefficient (
) of CPB with
bottom protection (this study t/d=0.20 ) when be
compared with reflection wave coefficient (
) of
CPB without bottom protection decrease than
reflection coefficient (
) of CPB without bottom
protection. The increasing of the transmission wave
coefficient (
) value of CPB with the bottom
protection ranges 0 - 0.07 depending on the value of
relative curtainwall depth (h/d), shown in Fig 5 and
Figure 7. Equation line statistical result analysis using
the SPSS program with multivariate non-linear data
on Table 4 shown on Figure 8, defined Equation 14.
0.699
.
.
0.036
(14)
4 CONCLUSION
The wave incident, transmission, and reflection
characteristics of CPB, curtainwall, and bottom
protector are experimentally studied under normal
regular waves. The influence of different wave and
structure parameters on CPB are studied e.g. the wave
length and height, curtainwall depth, and the bottom
protection height (t/d=0.20).
As a whole, the transmission coefficient (
)
decreases with relative curtainwall depth (h/d) and
wave steepness (H/L) increasing while reflection
coefficient (
) takes the opposite trend. The
transmission coefficient (
) of CPB without bottom
protection range 0.91-0.42 and reflection coefficient
(
) range 0.26-0.65 depends on relative curtainwall
depth (h/d) and wave steepness (H/L). The
transmission coefficient (
) of CPB with bottom
protection (t/d=0.20) range 0.83-0.23 and reflection
coefficient (
) range 0.26-0.72 depends on relative
curtainwall depth (h/d) and wave steepness (H/L).
Comparison between transmission coefficient (
)
and reflection coefficient (
) of CPB without bottom
protection and transmission coefficient (
) and
reflection coefficient (
) of CPB without bottom
protection of CPB without bottom protection is
obtained that transmission coefficient (
) CPB with
bottom protection (t/d=0.20) has a lower (range 0.04
– 0.22) and reflection coefficient (
) is higher (range
0 – 0.07) compared to CPB without bottom protection
depends on relative curtainwall depth (h/d).
It is recommended that the curtainwall pile
breakwater (CPB) can be combined with the bottom
protector based on the results of the study, the
curtainwall pile breakwater (CPB) that be combined
bottom protection can be applied to reduce the
Transmission-Reflection Wave on Curtainwall Pile Breakwater with and without Bottom Protection
123

transmission wave height. The results of this research
can be used to design the dimensions of the CPB to
obtain the expected transmission wave coefficient
(
) value must be required that wave steepness (H/L)
range 0.010 – 0.030, relative curtainwall depth (h/d)
range 0-0.70 and t/d = 0.20.
NOMENCLATURE
The following symbol have been adopted for use
in this paper :
C : propagation wave
/
d : water depth
H : wave height
: incident wave height
: reflection wave height
: transmission wave height
h/d : relative curtaiwall depth
H/L : wave steepness
: reflection wave coefficient
: transmission wave coefficient
L : wave length
T : wave period
t/d : relative the bottom protection height
t : the bottom protection height
ACKNOWLEDGEMENTS
The experimental work of this study was carried out
at Hydrology and Hydraulics Laboratory (Lab H-H)
PSIT of Universitas Gadjah Mada.
REFERENCES
Ahmed, H., 2011. Wave Interaction with Vertical Slotted
Walls as Permeable Breakwater, Ph.D Thesis, Hydro
Science (IGAW). Bergische Universitat Wupertal,
Germany.
Dean, G. D. & Dalrymple, R. A., 1999. Water Wave
Mechanics For Engineer and Scientists, Word
Scientific.
Ji, C. H. & Suh, K. D., 2008. “Reflection and Transmission
of Irregular Waves by Multiple-Row Curtainwall-Pile
Breakwater”, Proceedings of the Eighteenth (2008)
International Offshore and Polar Engineering
Conference Vancouver, BC, Canada. July 6-11, 2008
Koraim, A. S., 2015. “Mathematical Study for Analyzing
Caisson Breakwater Supported by Two Rows of Piles”,
Ocean Engineering, Vol. 104, pp. 89-106.
Koraim, A.S., Iskander, M.M., & Elsayed, W.R., 2014.
“Hydrodinamic Characteristics of Double Rows of Pile
Suspending Horizontal C Shaped Bar”, Ocean
Engineering, Vol. 84, pp. 81-96.
Laju, K., Sundar, V., & Sundaravadivelu, R., 2011.
“Hydrodynamic Characteristics of Pile Supported Skirt
Breakwater Models”, Applied Ocean Reseach, Vol. 33,
pp.12-22.
Liu, Y. & Li, Y.C., 2011. “Wave Interaction with a Wave
Absorbing Double Curtain-wall Breakwater”, Ocean
Engineering, 38, pp. 1237-1245.
Nejadkazem, O. & Gharabaghi, A. R. M., 2012. “Non-
propagating Waves and Behavior of Curtainwall-pile
Breakwaters”, Jurnal of Persian Gulf (Marine Science),
Vol. 3, pp.11-26.
Paotonan, C. & Yuwono, N., 2011. “Disipasi Energi
Gelombang yang Merambat Melalui Struktur Bawah
Air”, Dinamika Teknik Sipil, Vol. 11, No.2, pp. 107-
111.
Suh, K. D., Jung, H. Y., & Pyun, C. K., 2007. “Wave
Reflection and Transmission by Curtainwall–pile
Breakwaters Using Circular Piles”, Ocean Engineering,
Vol. 34, pp. 2100-2106.
Suh, K.D., Shin, S. & Cox, D. T., 2006. “Hydrodinamik
Charakteristics of Pile Supported vertical Wall
Breakwaters”, Journal of Waterway, Port, Coastal, and
Ocean Engineering, Vol. 132, No. 2, pp. 83-96.
EIC 2018 - The 7th Engineering International Conference (EIC), Engineering International Conference on Education, Concept and
Application on Green Technology
124
