Hull Resistance Analysis of Hydrofoil Mode-crocodile Ship Prototype

Wisnu Wardhana

1,a

, Meitha Soetardjo

, Ede M. Wardhana

, Sujantoko

Department of Ocean Engineering, Institut Teknologi Sepuluh Nopember, Indonesia

PT Tunas Maritim Global, Indonesia

Department of Marine Engineering, Institut Teknologi Sepuluh Nopember, Indonesia

Keywords: CFD Resistance Simulation, Crocodile Ship Prototype, RANSE Methods.

Abstract: This study presents resistance simulation of hydrofoil mode crocodile ship prototype in calm seawater

conditions using CFD in hydrofoil mode. The RANSE (Reynolds Averaged Navier-Stokes Equation) methods

are used for the viscosity solution of turbulent flow around the ship’s hull. Different turbulent models are used

for comparing the results in ship resistance calculations, in order to select the most appropriate methods. This

study on the creation of geometrical model considered exact pressure and velocity around hydrofoil mode

vessel submerged in calm seawater conditions, grid generation, setting mathematical model in Fluent and

evaluation of the simulations results. Comparison with experimental results also carried out.

1 INTRODUCTION

The prototype of Crocodile Ship has been designed

and fabricated to combine operational modes of

hydrofoil vessels, surface ships and submarines in its

mission. The vessel is designed with front and rear

wings of hydrofoil that can be controlled in all modes

of ship operation. The current paper investigates the

ship resistance in hydrofoil operation mode using

numerical simulation based on RANS Equations.

Assessment of ship resistance started to gain

importance with the advent of machine-propelled

ships in the early nineteenth century. The dependence

of ship resistance on velocity was necessary for

calculating the required power of the propulsion

system. Computational Fluid Dynamics (CFD) used

in this research, requires cooperation of several

disciplines such as mathematics, physics and

information technology for the development of this

method. CFD simulation gained larger scale

acceptance in the 90's and has sometimes replaced

experiments in many fields today. The CFD

simulations have commonly been integrated into

project of every new vessel, especially in the design

of seagoing ships. The approach for assessing ships

resistance by using experimental and CFD

simulations are also a part of this study. This study

aims at developing the process and selection of

appropriate methods for creating the geometrical

model, setting-up the mathematical model and

creating the preliminary CFD simulation. This will be

applied in crocodile ship prototype in calm seawater,

which represents seawater condition in archipelagic

countries such as Indonesia.

This paper presents a numerical study in different

speed conditions. This study has predicted resistances

characteristics of special hull using numerical

simulation and comparing the results with the

experimental results in the towing tank.

2 METHODOLOGY

This study is intended to conduct calculation as a

technique to predict resistance for ships. This method

starts with full scale dimension of ship model ran in

CFD analysis. The CAD ship model have previously

been conducted at various speed condition in calm

seawater: length (L) of 11m, breadth (B) of 3m, depth

(H) of 2m, maximum draft (T) of 1.8m and maximum

speed of carriage is at 4.0 m/s. Model resistance was

measured with dynamometer. The model was

attached to the measuring head of the resistance

dynamometer by a connector which can transmit and

measure only a horizontal tow force, even though the

model should be at the correct calculated

displacement. The resistance dynamometers were

attached at the LCB of the model as close to the shaft

line as possible. The electrical signal from

dynamometer are transmitted through overhead

136

Wardhana, W., Soetardjo, M., Wardhana, E. and Sujantoko, .

Hull Resistance Analysis of Hydrofoil Mode-crocodile Ship Prototype.

DOI: 10.5220/0010856100003261

In Proceedings of the 4th International Conference on Marine Technology (senta 2019) - Transforming Maritime Technology for Fair and Sustainable Development in the Era of Industrial

Revolution 4.0, pages 136-140

ISBN: 978-989-758-557-9; ISSN: 2795-4579

cables on trolley wires to the signal conditioning

equipment and ultimately to the computer. Data were

sampled for resistance and speed in the longitudinal

direction Fx. The forces are measured in mass (kg)

and converted to N through gravity (g) multiplication.

2.1 Experimental Setup

3D model of the crocodile hull was created according

to the dimensions (as shown in Tab.1) and shapes

defined seen in Figure 1 below which showed the

strout and nacelle positions under three modes of

operation. The vessel itself is currently under

construction in Institut Teknologi Sepuluh Nopember

(ITS) Surabaya as shown in Figure 2.

The calculation of the total resistance was

achieved by using the procedure as outlined in the

ITTC recommended procedures (ITTC, 2011). The

total resistance was calculated where the total hull

resistance is a function of hull form, ship speed, and

water properties, the coefficient of total hull

resistance is also a function of hull form, ship speed,

and water properties. The coefficient of total hull

resistance is found from the following equation.

𝑅



 0.5. 𝜌. 𝐶𝑡. 𝑆. 𝑉



(1)

The equation for the Froude number is

𝐹𝑟 

𝑉

𝑔𝐿

(2)

Where:

Ct : total resistance coefficient

RT : total hull resistance



: water density (kg/m

)

V : velocity (m/s)

S : wetted surface area of the

underwater hull (m

)

L : ship length (m)

g : gravity (m/s

)

(a) Submarine

(b) Surface

drofoils

Figure 1: 3D Model Crocodile hull condition.

Figure 2: Crocodile hull prototype under construction.

2.2 Numerical Setup

The simulation is made for various speed at 10, 20,

30, and 40 knots velocity of the crocodile prototype.

The Realizable k- epsilon, viscous models were also

used. For a detailed explanation of the discretization

of FVM, please refer to the book published by

Versteeg and Malalasekera (2007). The calculations

involved in this study are all for steady cases. Since

there are two types of flows solved with FVM

(inviscid and viscous), conditions of the viscous

solver is divided into two. For the viscous solver, the

realizable and standard k –ε turbulence model is used.

The inlet of the fluid domain is selected as velocity

inlet and the outlet as pressure outlet. The top and

bottom walls are in symmetrical boundary condition.

Simple algorithm was used for pressure- velocity

coupling, which is widely used and considered the

Table 1: Principal dimension crocodile ship

Principal

Dimension

Full Scale Model Scale

LOA

(

)

11 1.1

B (Breadth) (m) 3.0 0.3

T Draft

(

)

0.75 0.075

Full/Model

Scale ratio ()

1 1/10

Hull Resistance Analysis of Hydrofoil Mode-crocodile Ship Prototype

137

most suitable method. Pressure, momentum,

turbulent kinetic energy and dissipation rate are all

selected as second order.

This approach is called Reynolds Averaged

Navier Stokes Equation (RANSE):

𝜕𝜌

𝜕𝑡



𝜕

𝜕𝑥





𝜌𝑣





(3)

In this study, the flow is steady and

incompressible and the effects of free surface and

cavitation are ignored. Due to incompressible flow,

energy equation is automatically eliminated from the

conservation equations and only the continuity and

momentum equations are left. The viscosity term in

Equation (3) may also be eliminated depending on the

type of solution FVM will return.

3 RESULT AND DISCUSSION

Results from Numerical Simulation using K-

EPSILON Realizable Model Scale Results are shown

in table below that fr = 0.5, 0.99, 1.49 and 1.98

resistance obtained by results data of Force in x-axis

for each Froude Number are 28.4, 113.01, 253.41,

and 468.12 kN.

Numerical Simulation using K-EPSILON

Realizable Model Scale graph grows exponentially as

Froude Number increased.

(a) View 1 (b) View 2

Figure 3: 3D crocodile ship meshed model.

Table 2 : K-epsilon realizable model scale resul tnumerical

simulation.

Froude

Number

Velocity

model Vs

(

m/s

)

Velocity

model Vs

(

knots

)

Force in

x- axis

(

)

Model Scale

Resistance

(

)

0.55.14 10 28.4 32.5

0.9910.29 20 113.01 122.67

1.4915.43 30 253.41 257.83

1.9820.58 40 468.12 472.17

senta 2019 - The International Conference on Marine Technology (SENTA)

138

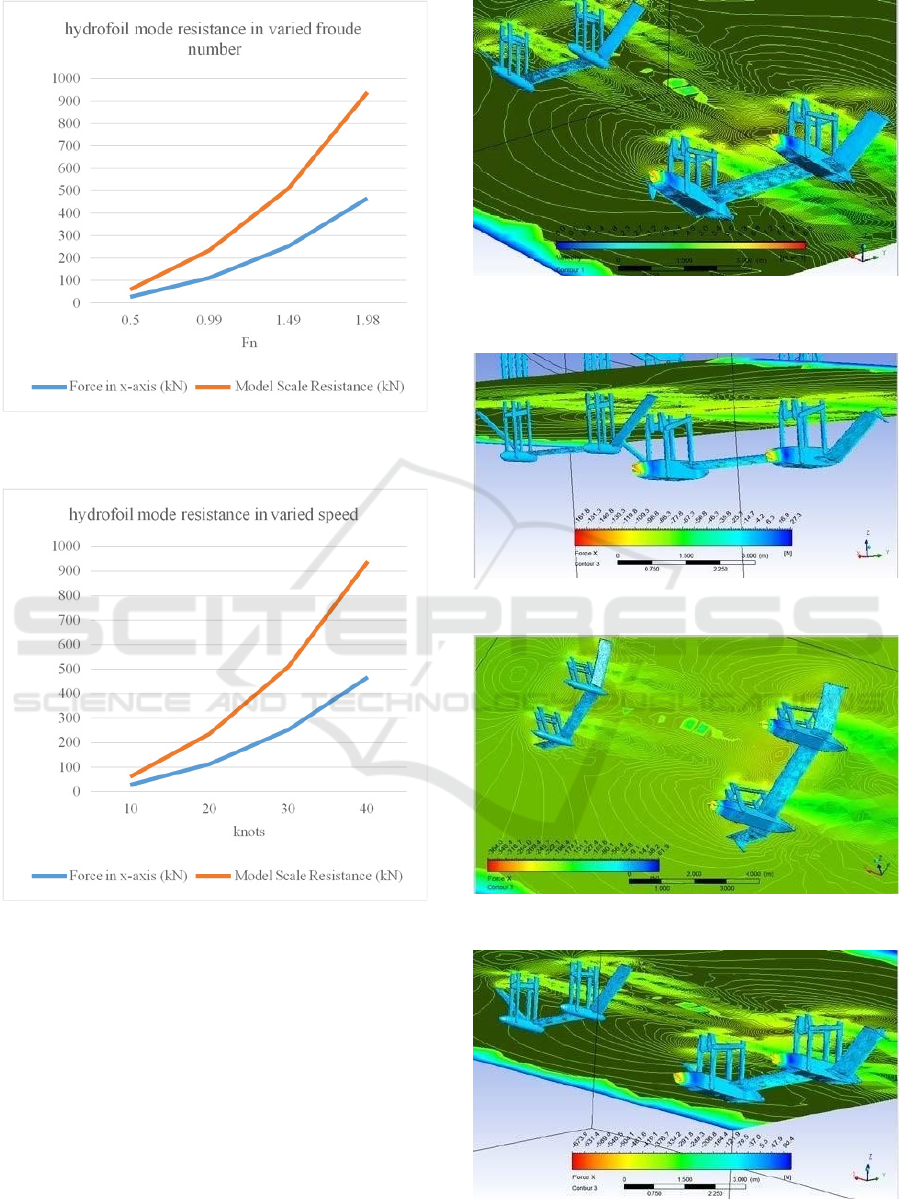
Figure 4: Resistance of each froude number in full scale

CFD experiment.

Figure 5: Resistance in each speed of full scale CFD

experiment.

Figure 6: Force in x-axis contour at 10 knots speed of

Hydrofoil Mode.

Figure 7: Force in x-axis 20 knots speed of Hydrofoil Mode.

Figure 8: Force in x-axis 30 knots speed of Hydrofoil Mode.

Figure 9: Force in x-axis 40 knots speed of Hydrofoil Mode.

Hull Resistance Analysis of Hydrofoil Mode-crocodile Ship Prototype

139

Figure 10: Towing tank test at 12knots speed.

4 CONCLUSION

This paper investigates the resistance and

hydrodynamic crocodile ship in hydrofoil condition.

A finite volume based RANS solver has been used to

evaluate the performance of these systems.

ACKNOWLEDGEMENTS

This work was financially supported by the Ministry

of Research, Technology and Higher Education of

Indonesia.

REFERENCES

Jones, B, 2008. ”Technological Aspects of Submarine”,

Journal of Underwater Tech, Canberra

Lewis,, E.V. 2002. ”Principle of Naval Architecture”,

London

Sarwito, S., 2015. ”Main System Development of

Crocodile- Hydrofoil Craft for Efficient and Effective

Sea Defense and Security”, PUPT Kemenristek-dikti

Walree, van F., 2007. ”Hydrofoils, Model Tests

andComputations”,

Hydrodynamics: Computations, Model Tests and Reality

Wardhana, W., 1998. ”Numerical and Experimental

Assessment of Hydrofoil Wing Interactions”, RUT IV

Wardhana, W., 2004. ”Numerical and Experimental of

Manned Hydrofoil Model”, RUT X

Wardhana, W., 2007. ”Numerical and Experimental

Assessment of Midget for Indonesian Purposes”, Riset

Insentif, Ristek, Jakarta

Wardhana, W., 2008. “Military Submarine Design for

Indonesia’s Defence and Security Purposes”, CCCL-

ITS International Joint Seminar, ITS Surabaya

Wardhana, W., 2013. ”Numerical and Experimental of

Crocodile- Hydrofoil Craft for Future Technology of

Sea Defense and Security”, RUSNAS, Kemendikbud-

Dikti

Wardhana, W., 2013. ”Design Philosophy of Crocodile-

Hydrofoil Craft”, SENTA ITS

Wardhana, W., 2015. ”Design Development of Crocodile-

Hydrofoil Craft for Future Technology of Sea Defense

and Security”, PUPT, Kemenristek-dikti

Versteeg, H., K. and Malalasekera, W., 2007. An

introduction to computational fluid dynamics-the finite

volume method. Glasgow

senta 2019 - The International Conference on Marine Technology (SENTA)

140