Modeling and Discretization of Hydraulic Actuated Telescopic Boom

System in Port-Hamiltonian Formulation

Lingchong Gao

, Wang Mei

, Michael Kleeberger

, Haijun Peng

and Johannes Fottner

Chair of Materials Handling, Material Flow, Logistics, Technical University of Munich,

Botlzmannstrasse15, 85748 Garching, Germany

Chair of Automatic Control, Technical University of Munich, Botlzmannstrasse15, 85748 Garching, Germany

Department of Engineering Mechanics, Dalian University of Technology,

Linggong Road No. 1, 116023 Dalian, P.R. China

Keywords:

Port-Hamiltonian System, Structure-preserving Discretization, Hydraulic Cylinder, Telescopic boom.

Abstract:

The hydraulic actuated telescopic boom system is the primary operation actuator of mobile cranes and aerial

platform vehicles. The purpose of this paper is to develope a uniﬁed mathematic model of such a boom

system which is a multi-domain system consisting of boom structure and hydraulic drive system. The model

is formulated within the port-Hamilton (PH) formalism using the deﬁnition of hydraulic system and elastic

boom structure as (Stokes-) Dirac structures. The Port-Hamiltonian systems can be easily interconnected thus

allowing the description of a complex system as a composition of subsystems. This property is especially

useful to model a multi-domain system with energy exchanges between subsystems. Considering the boom

structure as a Timoshenko beam, the lufﬁng operation of boom system is simpliﬁed in a plane coordinate

system. The Port-Hamiltonian model of the hydraulic system and the boom structure are described with

details separately, a structure-preserving discretization is applied to transfer the distributed-parameter boom

model into a lumped-parameter model. Then the interconnections between the subsystems are illustrated and

a complete simulation including hydraulic system is accomplished in MATLAB/Simulink.

1 INTRODUCTION

Mobile cranes and aerial platform vehicles are con-

struction machineries designed to lift heavy loads

for construction operations and to assist high al-

titude operations respectively. They are generally

equipped with long boom systems. The boom sys-

tem can be considered as a long boom manipu-

lator that consists of single or multiple long and

lightweight booms, using hydraulic actuators along

with an electro-hydraulic servo system to control the

movements. Because of its high energy density, the

hydraulic system is suitable to actuate such large scale

boom systems with heavy loads. Due to the long

boom system, mobile cranes are suitable for lifting

tasks with large radius and height, whereas aerial plat-

form vehicle can transport persons and equipment to

high operation positions for installation, maintenance

or ﬁre rescue missions. The longest boom system of

mobile cranes has reached the length of 250 meter and

the highest position that can be reached by aerial plat-

form vehicles is 114 meter.

In order to reduce the self-weight and to ensure the

mobility as well as transport-ability, the boom sys-

tems of mobile cranes and aerial platform vehicles

are designed as light as possible. The boom struc-

tures with limited stiffness are always performing a

strong ﬂexible behavior, even though the strength of

the boom system is ensured. Due to the dynamic

behavior of hydraulic actuators (hydraulic cylinders

or motors) which derives from the elastic hydraulic

oil and ﬂow characteristics of hydraulic components,

the output of the hydraulic system during start-up and

braking stages of the operations could ﬂuctuate signif-

icantly. The combination of the elastic drive system

and the ﬂexible boom structure leads to a hybrid sys-

tem with complex dynamic behavior. The ﬂexibility

of the boom structure can cause an intense vibration

response when the loads applied on the boom struc-

ture or the motion statuses of boom system change.

The structure vibration will increase the maximum

dynamic stress which could cause structure fatigue or

even structure failure. The oscillation at the boom’s

tip could also increase the difﬁculty of load locat-

Gao, L., Mei, W., Kleeberger, M., Peng, H. and Fottner, J.

Modeling and Discretization of Hydraulic Actuated Telescopic Boom System in Port-Hamiltonian Formulation.

DOI: 10.5220/0007832100690079

In Proceedings of the 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications (SIMULTECH 2019), pages 69-79

ISBN: 978-989-758-381-0

ing or endanger the safety of the personnel on plat-

forms. The vibration suppression for these large scale

boom systems is essential for improving the structure

fatigue life-cycle, operation efﬁciency and personnel

safety.

Some researchers focus on the dynamic analysis

of the boom structure, some others have investigated

the analysis of the whole boom system including hy-

draulic system and use hydraulic actuators to control

the structure vibration. Sun combined the ﬁnite el-

ement calculation method with mathematical formu-

lations of hydraulic drive system including essential

hydraulic components, to obtain a new model which

describes the dynamic interaction between the boom

structure and the drive system of mobile crane. (Sun

and Kleeberger, 2003). The method has been applied

for the exemplary calculation of slewing, lifting and

lufﬁng operations of lattice boom cranes (Sun et al.,

2005)(Sun and Liu, 2006). Both the load-bearing

structure and the drive system can be described in

details. The calculation of a telescopic boom crane

has also been studied. Similar long boom systems

are also used in ﬁre-rescue turntable ladders. The

boom system of a ﬁre-rescue turntable ladder is a

telescopic lattice boom actuated by a hydraulic cylin-

der. Sawodny described the long ﬁre-rescue turntable

ladder as a ﬂexible multi-body system (Zuyev and

Sawodny, 2005) and the dynamic behavior of the hy-

draulic drive system was included in the mathemat-

ical model equations (Sawodny et al., 2002). In the

work of Pertsch (Pertsch et al., 2009), a distributed-

parameter model for the ﬁre-rescue turntable ladder

was derived, based on the Euler-Bernoulli beam the-

ory. The model of the ladder structure was transferred

into low dimensional model space. In their recent

work (Pertsch and Sawodny, 2016), a model for the

coupled bending-torsional vibration associated with

the rotational motion of an articulated aerial ladder

has been derived and an active vibration damping con-

trol has been developed and validated in real opera-

tion.

The hydraulic actuated telescopic boom can be re-

garded as a multi-physical system consisting of the

mechanical structure, hydraulic drives and electrical

control system. The interconnections between the

subsystems are achieved by hydraulic actuators and

electro-hydraulic servo systems. With the increas-

ing demands for more accurate control precision dur-

ing the operations of these long boom manipulators,

the dynamic analysis for such multi-physical systems

has been extended from the dynamic respond study

of separated subsystems to a coupling analysis of dif-

ferent domain subsystems. The bond-graph method

(Gawthrop and Bevan, 2007) is a graphical approach

in which the component energy ports are connected

by bonds using power-conjugate variables, efforts

and ﬂows, to illustrate the energy transformation and

conversion between components and different phys-

ical systems. This port-based modeling method is

widely used to build a mathematical model of electro-

mechanical multi-domain systems (Guo et al., 2016)

(Cheng et al., 2016). The representation of a physi-

cal system as a bond graph can lead to a dynamical

system endowed with a geometric structure, which is

called a Port-Hamiltonian (PH) system. The geomet-

ric structure called Dirac structure is introduced as the

key mathematical concept to unify the description of

complex interactions in multi-physical systems. The

Hamiltonian function of the system energy which is

used to derive system state space equations, can also

be used as Lyapunov function for stability analysis.

Many researchers did a lot of work on the

system modeling and control design for ﬂexible

beam systems based on their representation as Port-

Hamiltonian system. Macchelli also reformulated the

Timoshenko model of beam within the framework

of the Port-Hamiltonian system (Macchelli and Mel-

chiorri, 2004). The transitional and rotational deﬂec-

tions and momenta were chosen as state variables to

build the (Stokes-) Dirac structure. He also used Port-

Hamiltonian approach to describe the multi-body sys-

tem (rigid body, ﬂexible links and kinematic pairs)

based on the power conserving interconnection (Mac-

chelli et al., 2009). In order to solve the inﬁnite di-

mensional model in Port-Hamiltonian formulation, a

structure-preserving discretization method is needed.

So that the discretized ﬁnite-dimensional model still

has the property of (Stokes-) Dirac structure (Moulla

et al., 2012)(Vu et al., 2013). Wang applied a ge-

ometric pseudo-spectral discretization to obtain the

ﬁnite-dimensional Port-Hamiltonian framework of

linear Timoshenko beam model, and solved the feed-

forward motion control problem based on this lumped

model (Wang et al., 2017). For the hydraulic sys-

tems, Kugi designed a nonlinear controller for a clas-

sical hydraulic piston actuator system based on the

Port-Hamiltonian model (Kugi and Kemmetm

uller,

2004). In his doctor thesis (Stadlmayr, 2009), Stadl-

mayr gave a Port-Hamiltonian representation of ﬂex-

ible manipulator consisting a long boom with a mass

at the tip and a hydraulic system using hydraulic

cylinder to actuate the manipulator (Stadlmayr and

Schlacher, 2004). Combined with feed-forward and

feedback control system, a MIMO-control was de-

signed and used to accomplish path tracking and vi-

bration suppression for the ﬂexible manipulator.

SIMULTECH 2019 - 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

2 SYSTEM DESCRIPTION

2.1 Port-Hamiltonian System

From the above research, it has shown that the Port-

Hamiltonian framework is a proper modeling method

for multi-domain systems because of its unique math-

ematical structure. To deﬁnite a Port-Hamiltonian

system, we start with the deﬁnition of a suitable space

of power variables which are strictly related to the ge-

ometry structure of the system. A Dirac structure de-

ﬁned on this space of power variables will be used to

describe the internal and external interconnection of

the system. We consider a linear space F (space of

generalized velocities or ﬂows) and its dual denotes

as E = F

∗

(space of generalized forces or efforts).

The space of the power variables is F ∗ E . Accord-

ing to the deﬁnition given by Duidam (Duindam et al.,

2009), there exists a canonically deﬁned symmetric

bilinear form on F ∗ E

 (f

),(f

) :=

| f

, (1)

where f

⊂ F , e

⊂ E and  .,.  denotes the duality

product between F and its dual space E . A constant

Dirac structure on F is a linear subspace D ⊂ F ×E

with the property D = D

⊥

. A crucial property of the

Dirac structure is that the standard interconnection of

Dirac structures is again a Dirac structure (Duindam

et al., 2009). Thus the components of each system

will be described as energy-storing elements, resistive

elements with the formation of Dirac structure and

also called as internal ports. The energy exchanges

between the system and environment (other systems)

are described by external ports, interaction ports and

control ports speciﬁcally. So that the system con-

sisting of these components is still a Dirac structure,

so as a large-scale multi-domain system composed of

multiple subsystems which have the formulations of

Dirac structure.

The system energy function Hamiltonian H(x) is

used to build the mathematical model of the system.

The state space variables x reﬂect the system ﬂow

variables by the deﬁnition of ˙x = f and the system

effort variables e are given by the co-energy variables

∂ H(x)/∂ x. All the subsystems can be described in the

form of

˙x = (J

J(x) − R

R(x))(

∂ H

∂ x

(x))

+ G

G(x)u

y = G

G(x)(

∂ H

∂ x

(x))

(2)

which is a useful formulation because the matrix

J(x) is a skew-symmetric matrix and the compo-

nents of J

J(x) are smooth functions of the state vari-

ables. The matrix R

R has to be symmetric and positive

semi-deﬁnite R

R = R

≥ 0. A dynamic system with

such formation is called a Port-Hamiltonian system.

In order to model the whole boom system as Port-

Hamiltonian system, the space of power variables of

hydraulic system and boom structure will be deﬁned

with numerical Dirac structure. The representation of

the boom system is written as the combination of the

hydraulic system and the boom structure in the for-

mulations of a Port-Hamiltonian system. For the sake

of simplicity, the damping effect is momentarily ne-

glected in the following chapters.

2.2 Port-Hamiltonian Model of

Hydraulic System

2.2.1 Essential Equations and Assumptions

The lufﬁng cylinder of the boom system can be simply

considered as the hydraulic cylinder actuator of Fig.

(1). A 4/3-way proportional directional valve controls

the movement of the asymmetric cylinder, which ac-

tuates the lufﬁng movement of the boom system. The

directional valve is connected with a pressure pump

and a tank. The supply pressure p

is determined by

the relief valve, and the tank pressure is p

The volumetric ﬂows through the directional

valve, Q

and Q

, are simply given by

= k

∆p

,i = 1,2 (3)

: Minimal volume

in Chamber 1

: Minimal volume

in Chamber 2

Figure 1: Diagram of the hydraulic system of a hydraulic

cylinder.

with the position of the valve core x

, the pressure dif-

ference ∆p

and the valve coefﬁcient k

, which can be

considered as constant when the properties of the ﬂow

through the valve ports are constant and the type of

valve core is slide valve. Then, we assume that there

is no internal or external leakage ﬂows and the tem-

perature remains constant. The continuity equations

Modeling and Discretization of Hydraulic Actuated Telescopic Boom System in Port-Hamiltonian Formulation

of the cylinder chambers can be described as

(As) = Q

(αA(l − s)) = Q

. (4)

Using the linearized constitutive law of the con-

stant (isothermal) bulk modulus E

oil

= ρ

∂ p

∂ ρ

, (5)

we can rewrite Eq. (3) as a well-known formation

˙p

oil

+ As



−A

+ Q



˙p

oil

+ αA(l − s)



αA

− Q



(6)

with the piston’s momentum P and its mass m. And

the motion equations of the piston are considered as

the following form

S =

P = (p

A − p

αA − F).

(7)

Eq. (7) and (6) constitute a state model of the valve-

controlled hydraulic cylinder of Fig. (1) with the state

vector x

x = [s, P, p

, p

]

2.2.2 Energy Description of Isentropic Fluid

In the case of an isentropic process no heat transfer

between the environment and the considering system,

which performs only work of expansion. The system

energy can be simpliﬁed as

dU = −pdV. (8)

By deﬁning the speciﬁc internal energy u = U/M

and the speciﬁc volume v = V /M, with M the mass of

the ﬂuid in the system, we can get

du = −pdv =

dρ (9)

where ρ = 1/v is the density of the ﬂuid.

Now we introduce another important thermody-

namic quantity, the enthalpy H = U + pV and the

mass speciﬁc enthalpy h = H/M = u + pv . Combin-

ing the deﬁnition of h with (9), we get the following

relations for the isentropic scenario

h = u +

p = u +

∂ u

∂ ρ

∂

∂ ρ

(uρ)

(10)

Based on the deﬁnition of isothermal bulk mod-

ules, integrating along an isentropic process, Eq. (5)

yields

p(ρ) = p

+ E

oil





with p

= p(ρ

). (11)

Then, the speciﬁc energy of the ﬂuid obeying the

constitutive law (5) can be obtained form (9) in the

form:

u(p) =



oil

−(p+E

oil

((

−p

)

oil

)



with u

= u (p

(12)

For the sake of convenience we will subsequently

choose p

= 0 and u

= 0. Hence the internal energy

results in

U(p) =

z }| {

V ρ(p)u(p) = V



oil



(p/E

oil

)

− 1



− p



(13)

Using the above formulation, we can acquire the

energy function of the ﬂuid inside the hydraulic cylin-

der, which is essential to building the Hamiltonian

function of the system.

2.2.3 Port-Hamiltonian Representation of

Hydraulic Cylinder

The speciﬁc internal energy u(p) of the ﬂuid in the

two chambers of the hydraulic cylinder can be deter-

mined by (12), then the energy stored in the hydraulic

cylinder of Fig. 1 is given by

Hydr

= V

u(p

) +V

u(p

)

= E

oil

∑

i=1,2



oli

)

−

oil

− 1



= V

+ As,V

= V

+ αA(l − s),

(14)

the subscripts refer to the corresponding quantities of

the chamber 1 and chamber 2 (rob side). Assuming

that the kinetic energy of ﬂuid mass can be neglected

compared to the kinetic energy of the piston, the total

energy E

of the hydraulic cylinder is

= U

Hyd

. (15)

We can use the total energy E

as the Hamilto-

nian function H

, then the Port-Hamiltonian model

of the hydraulic cylinder takes the form as (2) with

the state vector x

x = [s, P, p

, p

]

and the input vec-

tor u

u = [F,Q

]

, the hydraulic cylinder can be de-

scribed as

∑



x = J

J(x)∂

+ g

g(x)u

y = g

g(x)

∂

(16)

The matrices J

J(x) and g

g(x) are

J(x) =







0 1 0 0

−1 0 E

oil

A/V

−E

oil

αA/V

0 −E

oil

A/V

0 0

0 E

oil

αA/V

0 0







(17)

SIMULTECH 2019 - 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

g(x) =







0 0 0

−1 0 0

0 E

oil

0 0 −E

oil







(18)

In order to illustrate the system output y

y, more de-

tails of the model is given by

∂ H

∂ x







αA (Γ

) − A (Γ

)

P/m



oil

)

− 1





oil

)

− 1









with Γ

= p

− E

oil

)

+ E

oil

,i = 1,2.

(19)

Then using the deﬁnition (13), the system output

y = g

g(x)

∂ H

∂ x





0 −1 0 0

0 0 E

oil

0 0 0 −E

oil





∂ H

∂ x







−p/m

oil



oil

)

− 1



−E

oil



oil

)

− 1













−v

−ρ





(20)

The change of the system energy can be obtained

by the product of input u

u and output y

y as

= y

u = h

|{z}

−h

|{z}

−v · F (21)

In Eq. (21), the ﬁrst two parts represent the en-

ergy changed by the ﬂuid mass ﬂows to or from the

chambers respectively,

, and the third part

represents the work transferred to the boom structure

by cylinder force F.

2.3 Port-Hamiltonian Model of 2-D

Timoshenko Beam

2.3.1 A Rotation Homogeneous Timoshenko

Beam

The boom structure can be simpliﬁed as an ideal

beam model in a plane, when the lateral and axial

loads can be neglected. In order to obtain a suitable

Port-Hamiltonian representation of the boom struc-

ture, we start with the rotating homogeneous Timo-

shenko beam formulation to describe the dynamic be-

havior of the boom structure.

In Fig. 2. , t is the time variable and z is the spa-

tial coordinate along the equilibrium position of the

beam, and w

(z,t) is the deﬂection of the beam from

 

w t z

 

t z

Figure 2: A rotation homogeneous Timoshenko beam.

the equilibrium position and Ψ

(z,t) is the rotation of

the beam’s cross section.

The boundary condition of the boom structure can

be considered as a free tip with a ﬁxed end, and the

ﬁxed end rotates around a ﬁxed axis with the angle

displacement θ (t). Then the original Timoshenko

beam model is transferred to a rotating Timoshenko

beam. New system variables are deﬁned as

w(z,t) = w

(z,t) + z · θ(t)

ψ(z,t) = ψ

(z,t) + θ (t)

(22)

which still fulﬁll the original PDEs in a new form

∂

∂t

− K

∂

∂ z

+ K

∂ ψ

∂ z

= 0

∂

∂t

− EI

∂

∂ z

+ K



ψ −

∂ w

∂ z



= 0

(23)

In (23), the coefﬁcients ρ, I

, E, I are the mass per

unit length, the mass moment of inertia of the cross

section, Young’s modulus and the moment of the in-

ertia of the cross section respectively. And in the co-

efﬁcient K = kGA, G is the modulus of elasticity in

shear, A is the area of cross section and k is a constant

depending on the shape of the cross section.

2.3.2 Port-Hamiltionian Model of Timoshenko

Beam

For a homogeneous Timoshenko beam, the coefﬁ-

cients are constant and its mechanical energy is given

as following form:

(t) =

Hdz

H =



ρ ˙w

+ I

+ K (ψ − ∂

+ EI (∂

ψ)



(24)

According to the mechanical energy formulation

(24) the potential elastic energy is the function of the

shear and bending deformations, which can be written

as:

(z,t) = ∂

w(z,t) − ψ(z,t)

(z,t) = ∂

ψ(z,t).

(25)

The associated co-energy variables are shear force

Kε

(z,t) and bending momentum EIε

(z,t). The ki-

netic energy is the function of the translational and

Modeling and Discretization of Hydraulic Actuated Telescopic Boom System in Port-Hamiltonian Formulation

rotational momenta which are given as

(z,t) = ρ ˙w(z,t),

(z,t) = I

ψ(z,t),

(26)

and the co-energy variables are translational velocity

(z,t))/ρ and rotational velocity p

(z,t)/I

According to the deﬁnition of new state variables,

the original PDEs can be rewritten in a form







˙p













0 0 ∂

0 0 1 ∂

∂

−1 0 0

0 ∂

0 0



















. (27)

We denote e ∈ E , f ∈ F as the effort and ﬂow

variables separately. They are related to the time

derivative of state variables f

f = −

x and the associ-

ated co-energy variables as

f =













=−







˙p







e =

























. (28)

The total energy (we neglect the gravity potential

energy and just focus on the quadratic energy func-

tions) becomes the following formulation:

∂

H ·

xdz = −

· fdz. (29)

Applying integration by parts on Eq. (29), one

obtains

= (e

+ e

)

. (30)

Deﬁning the boundary ﬂow and effort variables as







∂













∂ Z







, (31)

where e

∂ Z

denotes the restriction on the border of the

domain Z = [0, L]. Comparing the right hand sides

of (29) and (30), it is clear that the increase of the

total energy is equal to the power through the borders.

And the power continuity and conservation equation

is fulﬁlled as

· fdz +



∂

· e

∂

+ f

∂

· e

∂





∂ Z

= 0. (32)

Finally, Eq. (27) can be rewrote shortly as

− f

f = J

J(z)∂

H = J

J(z)e

e, (33)

where J

J(z) is a skew-symmetric differential operator

as that in Eq.(2). Using the deﬁnition of ﬂow, ef-

fort variables (28) and the system state equations (33),

one can deﬁne a bilinear geometric structure, a Dirac

structure D:

D =

{

( f

f , f

, f

∂

e,e

∂

) ∈ F × E |

− f

f = J

J(z)e

e and Eq.(30) holds }.

(34)

2.3.3 Structure-preserving Spatial Discretization

In order to solve the distributed parameter model

of Timoshenko beam, we need to transfer its Port-

Hamiltonian model into a lumped-parameter model.

The skew-symmetric differential operator has to

be retained in the new discretized model, which

means that the discretization method should preserve

such certain (geometric or structural) property. In

(Moulla et al., 2012), a structure-preserving spa-

tial discretization method is applied to approximate

the inﬁnite-dimensional Timoshenko model by the

pseudo-spectral method. The discretization of the

Port-Hamiltonian system is completed with the ﬁnite-

dimensional approximation of the energy and the con-

stitutive relations based on the discretization of the

(Stokes-) Dirac structure. Furthermore, the bound-

ary port variables are preserved and speciﬁed (inter-

connection) port variables (inputs and outputs) in the

resulting lumped Port-Hamiltonian model during the

whole process.

The geometric discretization has proven to pro-

vide a good approximation of system properties in

(Wang et al., 2017), such as the spectrum of the dif-

ferential operators. Some essential steps are reviewed

in this section.

Based on the formulation (27), we rewrote the

Dirac structure (34) as

−f =







1 0

0 1

1 0

0 1







∂







0 0

1 0

0 −1

0 0







∗

(35)

so we can classify the effort vectors as e and e

∗

, de-

pending on whether it is subject to differentiation or

not.

According to the pseudo-spectral method pro-

posed for canonical systems of two conservation

laws, we deﬁne different approximation bases for

the ﬂows f

∈

{

, f

}

and the efforts e

∈

{

}

(t,z) ≈

N−1

∑

k=0

(z), e

∗

(t,z) ≈

N−1

∑

k=0

∗,k

(z),

(t,z) ≈

∑

i=0

(z).

(36)

The time dependent coefﬁcients are collected in

the vectors

∗

∈ R

and e

∈ R

N+1

,v ∈

{

, p

,ε

}

(37)

(z) and φ

(z) are the basis functions for ﬂows

and efforts, and satisfying the exact differentiation or

SIMULTECH 2019 - 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

compatibility condition (Vu et al., 2013):

E = span

{

,... ,φ

}

F = span

{

,... ,ϕ

N−1

}

∂

E = F .

(38)

Then we chose the interpolation Lagrange poly-

nomials of degree N and N −1 as suitable bases func-

tions for the efforts and ﬂows:

(z) =

N−1

∏

j=0, j6=k

z − z

− z

,φ

(z) =

∏

j=0, j6=i

z − ζ

− ζ

. (39)

∈ (0, L),k = 0,.. . ,N − 1 and ζ

∈ (0, L), i =

0,... ,N are the collocation points for ϕ

(z) and φ

(z)

respectively. In this paper, we choose the zeros of

Legendre polynomials to reduce the Runge’s phe-

nomenon (Cardoso-Ribeiro et al., 2016), i.e. the oc-

currence of numerical oscillations at the boundaries

of the interval with increasing number of collocation

points.

Denote φ

φ = [φ

,... ,φ

]

the vector of effort basis

functions and

Φ =



φ(0)

φ(L)



. (40)

Let f

t/r

∂

t/r

, f

t/r

and e

t/r

∂

t/r

the vectors of boundary ﬂows and efforts correspond-

ing to translational or rotational motion. Inserting

(36) into (35) and (31), one obtains the linear system

of equations

−



















D 0

0 D

D 0

0 D

























0 0

I 0

0−I

0 0













∗













∂































(41)

The elements of the derivative matrix D

D ∈

N×(N+1)

are obtained from the spatial derivative

of the effort bases functions at the ﬂow collocation

points:

k+1,i+1

= ∂

), (42)

with i = 0, ... ,N,k = 0, ... ,N −1. In accordance with

the distributed parameter model, an additional cou-

pling term with identity matrices I

I = I

appears on

the right hand side of (42).

Replacing the approximations of ﬂows and efforts

in the energy balance, we obtain

H ≈

∑

v∈

{

,ε

}

)

M f

(43)

with the elements of the non-square matrix M

M ∈

(N+1)×N

deﬁned as

i+1,k+1

(z)ϕ

(z)dz. (44)

The right hand side of (43) consists of degen-

erated bilinear forms between the vectors of dis-

crete ﬂows and efforts. Due to the degeneracy (the

kernel of M

M is non-empty), this bilinear form does

not qualify to deﬁne a Dirac structure on the ﬁnite-

dimensional bond space of reduced ﬂows and efforts

× E

with



∂



∈ E

∈ R

4N+8

and



, f

∂

, f

∂



∈ F

∈ R

4N+4

. It can,

however, be shown that the power continuity equation

(32) is approximated via

∑

v∈

{

,ε

}

)

M f

∑

u∈{t,r}



∂



M f

∂

= 0.

(45)

To obtain a non-degenerate power pairing, vectors of

reduced effort variables

∈ R

are deﬁned:

= M

. (46)

These shall be, we discretize the constitutive equa-

tion e

e = (δ

, derived from a discrete energy. Note

that

x = − f

f holds, i.e. states and ﬂows are discretized

with respect to the same basis. We can deﬁne

− f

and replace the approximation in H =

H dz

with Hamiltonian density. We obtain

H ≈

∑

)

, c

∈





, (47)

where the elements of S

S ∈ R

N×N

are given by

i+1, j+1

(z)ϕ

(z)dz. (48)

The required discretized constitutive relation is

simply



∂ H

∂ x



= c

, ∀v. (49)

On the other hand, the relation for the discretized

effort vectors e

∗

in the ﬂow (or state) bases becomes

∗

= c

= S

−1

= S

−1

. (50)

According to (Moulla et al., 2012), the discretised

Timoshenko beam can be formulated into an input-

/output (I/O) representation:

f =



0 J



· e

e (51)

Modeling and Discretization of Hydraulic Actuated Telescopic Boom System in Port-Hamiltonian Formulation

with

f =



,−e

∂ 0

, f

,−e

∂ 0

, f

∂ L

, f

∂ L



e =



, f

∂ 0

, f

∂ 0

∂ L











−D

−φ





−1



−S

−1





−1



−D

−φ





−1















−D

−φ





−1



−S

−1





−1



−D

−φ





−1







Indeed, J

= J

, i.e. the interconnection matrix

J ∈ R

(4N+4)×(4N+4)

is skew-symmetric.

We obtain the explicit state space model in linear

Port-Hamiltonian form

X = J

4N×4N

X +G

4N×4

Y = G

4N×4

4N×4N

+ D

4×4

(52)

The vector x

∈ R

is merged in the

overall state vector X

X ∈ R

, Q

4N×4N

blockdiag



S/ρ, S

S/I

S,K



is the overall

energy (Hessian) matrix, and U

U ∈ R

, Y

Y ∈ R

are

the vectors of boundary inputs and collocated, power

conjugated outputs. They are composed of the

elements of the boundary ﬂow an effort vectors. In

the terms of the physical boundary variables we have

U(t) =



















v(0)

ω(0)

Q(L)

M(L)







Y (t) =



















−Q(0)

−M(0)

v(L)

ω(L)







2.4 Port-Hamiltonian Model of the

Hydraulic Actuated Telescopic

Boom System

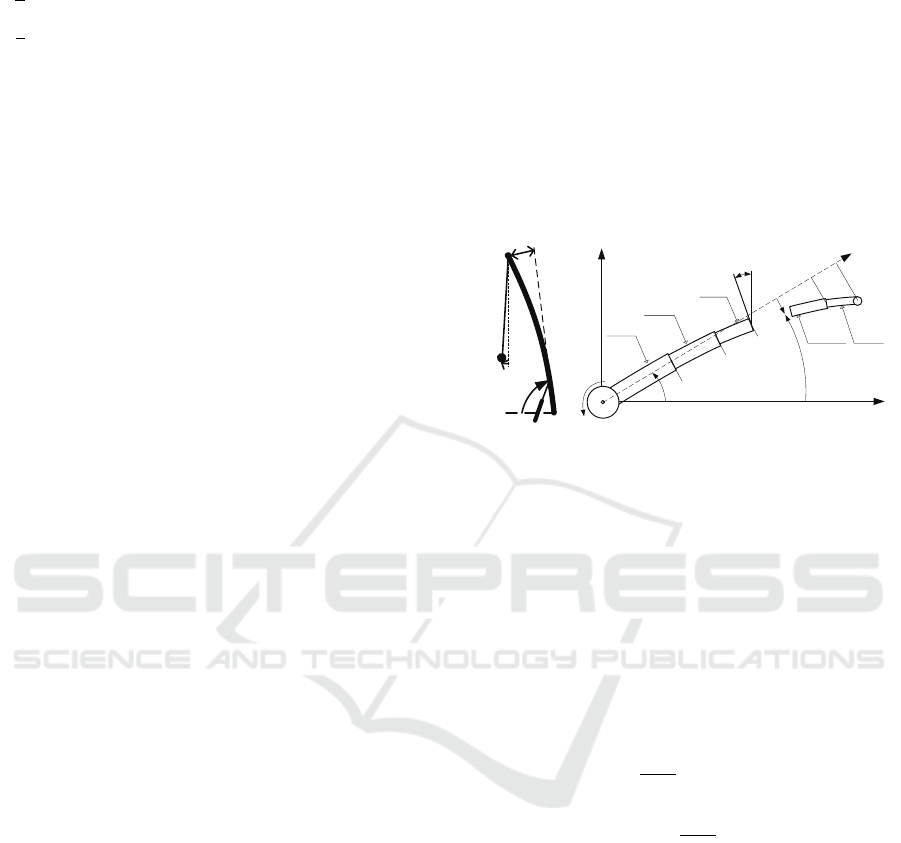
2.4.1 Modeling of the Telescopic Boom Structure

Based on the Timoshenko assumption and its Port-

Hamiltonian representation, it is not difﬁcult to build

a proper mathematical model for the structure of tele-

scopic boom. However there are some modiﬁcations

should be clariﬁed. The coefﬁcients ρ, I

, I of the

new beam model are not constant. Considering the

telescopic boom structure, its boom sections are de-

creasing so that all the other boom sections can be

pulled back into the ﬁrst boom, it means that a ho-

mogeneous beam model is no longer suitable. The

boom structure will be modeled as a N-stepwise beam

with different coefﬁcients for each boom section, a

non-homogeneous Timoshenko beam. However, each

boom section can be considered as a homogeneous

Timoshenko beam and the boundary conditions be-

tween each two sections are speciﬁed as ﬁxed accord-

ing to the continuous conditions. The overlapping

parts of the boom sections and the telescopic mech-

anism are neglected in the purpose of simpliﬁcation.









 

, ( , ) ( )

w z t w z t z t

 

( )

( , )

w z t

 

( , ) ( , )

z t z t t

\ \ T



Figure 3: Telescopic boom and its simpliﬁed model: the

rotating non-homogeneous Timoshenko beam.

According to the deﬁnition of power variables

(25) and (26), we can deﬁne the new power vari-

ables for the k

section of the N-stepwise beam in the

domain of D

:= [L

k−1

] as p

t,k

, p

r,k

,ε

t,k

,ε

r,k

,k =

1,... ,n. For each part of the stepwise Timoshenko

beam, a Dirac structure D

can be acquired by using

the corresponding power variables.

According to the Port-Hamiltonian form (51), the

I/O represent of the kth boom is represented as system

∑











= J



∂ H

∂ x



+ G







∂ H

∂ x



+ D



k−1



−Q

k−1

− M

k−1

(53)

the superscripts indicate that the variables are belong

to the k

beam and the subscripts represent the con-

nection points.

2.4.2 Interconnection between Subsystem

Now we focus on the interconnection between sub-

systems. The telescopic boom structure can be con-

sidered as the combination of multiple subsystem and

each of them is a homogeneous Timoshenko beam.

Based on the geometrical and mechanical continu-

ous conditions, the ﬂows and efforts of the k

and

SIMULTECH 2019 - 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

(k + 1)

beam sections through the connection point

have the following relations

t,k

∂

,t) = f

t,k+1

∂

,t)

r,k

∂

,t) = f

r,k+1

∂

,t)

t,k

∂

,t) = e

t,k+1

∂

,t)

r,k

∂

,t) = e

r,k+1

∂

,t)

(54)

which mean that the inputs and outputs of the adja-

cent two beams have similar relations at the connec-

tion point L

= U

k+1

= −U

. (55)

According to the I/O representation (53) as Port-

Hamiltonian system and the transfer characteristics

(55) between outputs and inputs, the boom structure

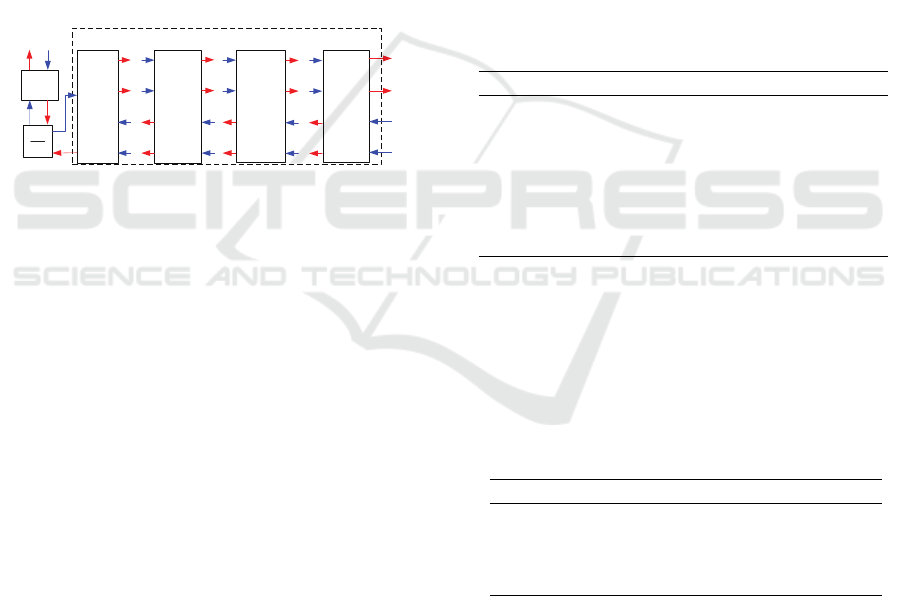
can be built as a series system showed in Fig. 4.



Q















...



Hydraulic

Cylinder

Telescopic boom structure

Loads

Responds

...

-1

...

i i

1k 

Figure 4: Interconnection of multiple beam and hydraulic

system.

The interconnection between the hydraulic sys-

tem and the boom structure is reﬂected by the luff-

ing mechanism, in which the hydraulic cylinder acts

as the actuator. The output of the hydraulic system,

the velocity of the piston, can be transferred to the

input angle velocity of the ﬁrst beam at its boundary

(z = 0).

Based on the measurements of lufﬁng mechanism

(the length of the cylinder and the positions of the

joints), the rotation of the boom can be expressed as

θ(t) = ∂

θ · s(t), the actuate force and moment have

the relation F = ∂

· θM. Therefor we have the I/O

transfer relation between the hydraulic system and the

boom structure as

r,1

= ω

= ∂

θ · ˙s = ∂

θ ·y

= F(t) = ∂

θ ·M

= ∂

θ ·Y

r,1

(56)

which illustrates the interconnection between the two

systems in different domains.

Finally, we have the complete model of the hy-

draulic actuated telescopic boom system. The load

at the boom’s tip and the hydraulic ﬂows though the

cylinder are the system inputs, the velocity responds

at the boom’s tip and the enthalpy change of the hy-

draulic system are the system outputs.

3 SIMULATION AND RESULTS

In order to evaluate the performance of our complete

model, we ﬁrstly evaluate the approximation quality

of the ﬁnite-dimensional model of non-homogeneous

Timoshenko beam based on the geometric discretiza-

tion.

We deﬁne a stepped beam model with three sets

of parameters as Table 1. The model of each part

of the beam are built as a Port-Hamiltonian system,

discretized and implemented in MATLAB/Simulink

individually. Then connect these three I/O represen-

tations as a series system. A ﬁnite element model

using the given parameters (Table 1) is also built

in NODYA, a dynamic ﬁnite element analysis pro-

gramme developed by our institute. An eigenvalue

analysis is applied ﬁrstly to check the frequencies, and

the next step is to check the respond behaviors by the

dynamic analysis.

Table 1: System parameters.

Parameter Beam 1 Beam 2 Beam 3

Length 0.3m

Width 0.02m 0.015 0.01

Depth 0.005m 0.004m 0.003m

Density 7850kg

Yong’s modulus 210GPa

Poisson’s ratio 0.33

Shear factor 5/6

The results of eigenvalue analysis is listed in Ta-

ble 2, the deviations between the two models are less

than 4% . It means that the Port-Hamiltonian system

representation and the corresponding geometric dis-

cretization are still suitable for the non-homogeneous

Timoshenko beam.

Table 2: Eigenvalue analysis results.

Mode num. FE- PH Deviation

1 7.37Hz 7.54Hz 2%

2 27.5Hz 28.2Hz 3%

3 65.9Hz 67.9Hz 3%

4 136.4Hz 141Hz 3%

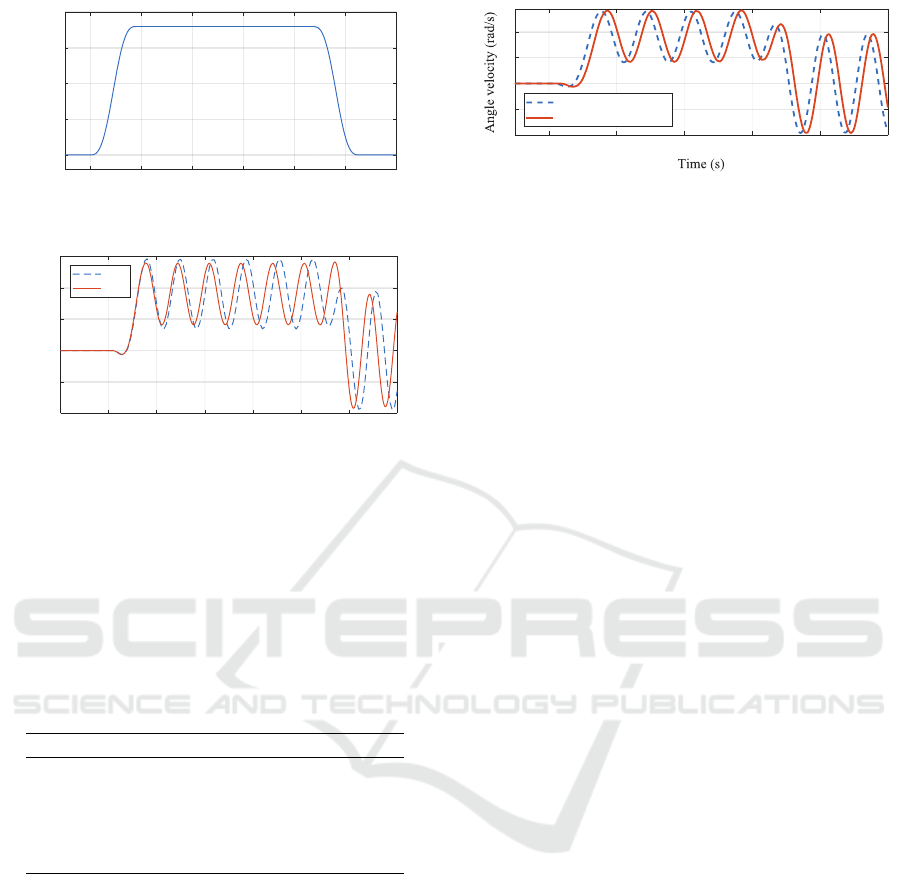
Fig. 5 (b) represents the transient responds of the

FE-model and the Port-Hamiltonian model, both have

the deﬁnition of input ω

as Fig. 5 (a) shows. The

amplitudes of the responding angle velocity at the

boom’s tip are very close. And the difference of the

eigenvalue is also reﬂected.

Next, we can evaluate the model of complete

telescopic boom system using the veriﬁed Port-

Hamiltonian model of boom structure. The model

Modeling and Discretization of Hydraulic Actuated Telescopic Boom System in Port-Hamiltonian Formulation

(a) Input angle velocity

(b) Output angle velocity

1 1.2 1.4 1.6 1.8 2 2.2

Time (s)

0.5

1.5

Angle velocity (rad/s)

0.8 1 1.2 1.4 1.6 1.8 2 2.2

Time (s)

-2

-1

Angle velocity (rad/s)

FEM

Figure 5: Desired input and the transient responds of FE-

model and Port-Hamiltonian model.

of the hydraulic system with the parameter set as

in Table 3 is also implemented in Matlab/Simulink.

Then the model can be easily connected to the model

of boom structure by the speciﬁed input and output

ports.

Table 3: Parameters of hydraulic system.

Parameters Value

Supply pressure 10bar

Tank pressure 0bar

Piston Area and area ratio 0.0001m

, 0.75

Bulk modulus 1.2GPa

Piston stoke length 0.25m

In order to avoid the strong nonlinear characteris-

tic of lufﬁng mechanism, we set the limit of the input

angle displacement to π/3. Fig. 6 shows the transient

responds of the boom structure model and the com-

plete model including the hydraulic system. In this

case, the inﬂuence of the hydraulic system is reﬂected

by the respond time delay.

4 CONCLUSION AND OUTLOOK

In this paper, we presented a modeling method and

a corresponding discretization method to describe the

hydraulic actuated telescopic boom system as a Port-

Hamiltonian system. The hydraulic cylinder and tele-

1 1.2 1.4 1.6 1.8 2

-2

-1

Beam only

With hydraulic system

Figure 6: Transient respond of the complete model of tele-

scopic boom, including hydraulic system.

scopic boom structure were modeled in PH formula-

tion respectively. A PH model of non-homogeneous

Timoshenko beam is proposed, in order to describe

the telescopic boom structure more accurately. The

model is proven by the comparison with ﬁnite element

model. Using the interconnection ports, these individ-

ual models could be integrated into a complete model,

which include the dynamic behavior of hydraulic sys-

tem in the dynamic simulation of telescopic boom

system. The future works are presented as following:

1. The complete model in this paper is still an open

loop system, the vibration responds of the boom

structure is not controlled. A closed loop system

will be developed. Some suitable controller will

be added to the hydraulic system for the purpose

of boom vibration suppression.

2. The 2-D bending vibration model of Timoshenko

beam is not good enough for the boom structure

modeling under some circumstances, especially

for the mobile cranes hoisting heavy loads. The

boom structure is bearing axial force when the

lufﬁng angle is large, in such case the axial de-

formation cannot be neglected. A more complex

beam model will be investigated and its PH for-

mulation will be presented.

ACKNOWLEDGEMENTS

The research is supported by Deutsche Forschungs-

gemeinschaft (FO 1180 1-1) and National Science

Foundation of China (11761131005).

REFERENCES

Cardoso-Ribeiro, F. L., Matignon, D., and Pommier-

Budinger, V. (2016). A power-preserving discretiza-

tion using weak formulation of piezoelectric beam

with distributed control ports. IFAC-PapersOnLine,

49(8):290–297.

Cheng, L., Ye, Z., and Tong, Z., editors (2016). Bond graph

modeling and simulation analysis of direct drive vol-

SIMULTECH 2019 - 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

ume control electro-hydraulic servo system with long

pipeline. IEEE.

Duindam, V., Macchelli, A., Stramigioli, S., and Bruyn-

inckx, H. (2009). Modeling and control of complex

physical systems: The port-Hamiltonian approach.

Springer Science & Business Media.

Gawthrop, P. J. and Bevan, G. P. (2007). Bond-graph mod-

eling. IEEE Control Systems Magazine, 27(2):24–45.

Guo, Y., Liu, D., Yang, S., Li, X., and Chen, J. (2016).

Hydraulic–mechanical coupling modeling by bond

graph for impact system of a high frequency rock drill

drifter with sleeve distributor. Automation in Con-

struction, 63:88–99.

Kugi, A. and Kemmetm

uller, W. (2004). New energy-

based nonlinear controller for hydraulic piston actu-

ators. European journal of control, 10(2):163–173.

Macchelli, A. and Melchiorri, C. (2004). Modeling and

control of the timoshenko beam. the distributed port

hamiltonian approach. SIAM Journal on Control and

Optimization, 43(2):743–767.

Macchelli, A., Melchiorri, C., and Stramigioli, S. (2009).

Port-based modeling and simulation of mechanical

systems with rigid and ﬂexible links. IEEE transac-

tions on robotics, 25(5):1016–1029.

Moulla, R., Lefevre, L., and Maschke, B. (2012). Pseudo-

spectral methods for the spatial symplectic reduction

of open systems of conservation laws. Journal of com-

putational Physics, 231(4):1272–1292.

Pertsch, A. and Sawodny, O. (2016). Modelling and con-

trol of coupled bending and torsional vibrations of an

articulated aerial ladder. Mechatronics, 33:34–48.

Pertsch, A., Zimmert, N., and Sawodny, O., editors (2009).

Modeling a ﬁre-rescue turntable ladder as piecewise

Euler-Bernoulli beam with a tip mass. IEEE.

Sawodny, O., Aschemann, H., and Bulach, A. (2002).

Mechatronical designed control of ﬁre-rescue

turntable-ladders as ﬂexible link robots. IFAC

Proceedings Volumes, 35(1):509–514.

Stadlmayr, R. (2009). On a combination of feedforward and

feedback control for mechatronic systems. Shaker.

Stadlmayr, R. and Schlacher, K., editors (2004). Modelling

and Control of a Hydraulic Actuated Large Scale Ma-

nipulator, volume 1. Wiley Online Library.

Sun, G. and Kleeberger, M. (2003). Dynamic responses of

hydraulic mobile crane with consideration of the drive

system. 38(12):1489–1508.

Sun, G., Kleeberger, M., and Liu, J. (2005). Complete

dynamic calculation of lattice mobile crane during

hoisting motion. Mechanism and Machine Theory,

40(4):447–466.

Sun, G. and Liu, J. (2006). Dynamic responses of hydraulic

crane during lufﬁng motion. Mechanism and Machine

Theory, 41(11):1273–1288.

Vu, N. M. T., Lefevre, L., Nouailletas, R., and Br

emond, S.

(2013). Geometric discretization for a plasma control

model. IFAC Proceedings Volumes, 46(2):755–760.

Wang, M., Bestler, A., and Kotyczka, P. (2017). Mod-

eling, discretization and motion control of a ﬂexi-

ble beam in the port-hamiltonian framework. IFAC-

PapersOnLine, 50(1):6799–6806.

Zuyev, A. and Sawodny, O. (2005). Stabilization of a ﬂexi-

ble manipulator model with passive joints. IFAC Pro-

ceedings Volumes, 38(1):784–789.

Modeling and Discretization of Hydraulic Actuated Telescopic Boom System in Port-Hamiltonian Formulation