
Optimal Trigger Sequence for Non-iterative Co-simulation
Franz Rudolf Holzinger
a
and Martin Benedikt
b
VIRTUAL VEHICLE Research Center, Inffeldgasse 21/A/II, Austria
Keywords:
Trigger Sequence, Execution Order, Calculation Order, Non-iterative Co-simulation, Travelling Salesman
Problem.
Abstract:
An execution sequence approach of interacting subsystem is presented for non-iterative co-simulation frame-
works. Local behaviour of coupling signals and subsystems are used to describe a general optimization prob-
lem of co-simulation. Therefore the linking matrix is weighted by analysis of the coupling signals within fuzzy
integrated expert knowledge. The weighted linking matrix is transferred to a directed co-simulation graph,
which can be interpreted as an appropriate travelling sales man problem. The solution of this co-simulation
graph provides an optimized trigger sequence of the subsystems.
1 INTRODUCTION
In the last decade co-simulation becomes a relevant
technique in diverse system development approaches,
especially in the automotive industry. The increas-
ing complexity of the systems forces engineers to di-
vide problems in several smaller sub-problems. These
subsystems are often modelled in specific simulation
environments and are solved by their own solvers.
Co-simulation is a technique to combine these sub-
models to an overall common system and allows
to run a holistic simulation (K
¨
ubler and Schiehlen,
2000).
The increasing number of subsystems and cou-
pling signals in co-simulation presents a significant
challenge for each co-simulation user and application
engineer. The individual subsystems and coupling
signals have different dynamic behaviour. These have
to be considered in the coupling by appropriate cou-
pling time steps of the several subsystems, proper
choice of extrapolation filters and suitable trigger se-
quence of the subsystems. In general, application en-
gineers have barely information about the subsystems
and so it is hardly possible to prepare a co-simulation
configuration with respect to simulation duration and
simulation accuracy. Therefore it is necessary to sup-
port the co-simulation user by an automatically con-
figuration of the co-simulation. This idea was al-
ready generally discussed by the authors (Benedikt
and Holzinger, 2016). An automated approach for ob-
a
https://orcid.org/0000-0003-3551-6579
b
https://orcid.org/0000-0003-2652-6812
taining the trigger sequence for co-simulation to im-
prove the simulation accuracy is discussed in the fol-
lowing.
The outline of this work is as follows. This pa-
per starts with an introduction into non-iterative co-
simulation. Reasonable information utilized for trig-
ger sequence determination is identified based on dis-
cussion on possible sources of coupling errors. An
approach to get a proper trigger sequence based on
the idea to solve travelling sales man problem is pre-
sented. This approach is illustrated and discussed by
an example.
2 NON-ITERATIVE
CO-SIMULATION
There are two well-established types of co-simulation
techniques: iterative and non-iterative. Compared to
iterative co-simulation the non-iterative one does not
allow to repeat a coupling step. In general this allows
a higher simulation performance in terms of simula-
tion duration. On the other hand the discretization
caused by the coupling mechanism is much higher.
Nevertheless the non-iterative co-simulation is com-
monly used in industry. Therefore one of the reasons
is that many simulation tools and subsystems do not
support external control reset of internal states and
thus repeating a time step is not possible.
In general, the induced coupling errors are higher
in the case of non-iterative co-simulation compared
to iterative co-simulation. Bidirectional dependencies
80
Holzinger, F. and Benedikt, M.
Optimal Trigger Sequence for Non-iterative Co-simulation.
DOI: 10.5220/0007833800800087
In Proceedings of the 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications (SIMULTECH 2019), pages 80-87
ISBN: 978-989-758-381-0
Copyright
c
2019 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved

between subsystems cause causality problems and so
extrapolation filtering is needed. Low order poly-
nomial extrapolation filters are generally used to es-
timate the input for the next simulation step. The
commonly used filter technique is the zero-order-hold
(ZOH) extrapolation. Here the last determined value
of the coupling signal is set as input signal during
the next calculation step. In addition to the error be-
tween the estimated signal and the actual determined
signal, the resulting staircase-shaped input signal can
also stimulate the subsystem and cause wrong results.
From the co-simulation configuration point of
view, there are mainly three different kinds of options,
to influence and improve the simulation quality:
• Coupling step size to define how often signals are
exchanged between the subsystems
• Extrapolation filter to solve the causality problem
of bidirectional dependent subsystems
• Trigger sequence to define the calculation order of
the subsystems
The topics of extrapolation filter and coupling time-
steps were already well discussed in the past (Busch
and Schweizer, 2011) (Benedikt et al., 2010). There
are still methods which compensate the extrapolation
error (Benedikt and Hofer, 2013), (Benedikt et al.,
2013). However a proper trigger sequence prevents
or at least reduces coupling errors at (some) inputs.
3 TRIGGER SEQUENCE
Co-simulation typically applies parallel scheduling
scheme in which all subsystems are calculated in par-
allel. This approach has a high simulation perfor-
mance (in terms of simulation duration) because the
subsystems can be calculated in the same time. Nev-
ertheless every input signal has to be extrapolated and
so in each coupling signal an error is induced. To re-
duce the coupling error subsystems can be executed
in sequential order, i.e. each subsystem is calculated
after the other.
The challenge is to define a trigger sequence or
calculation order, which minimizes the coupling ef-
fects. The number of possible sequences factori-
ally increases with the number of subsystems. A
simple co-simulation example with m = 4 subsys-
tems (see Fig. 1) leads already to m! = 24 differ-
ent possible configurations with respect to the exe-
cution order. The induced coupling error and thus
the results change depended on the defined trigger se-
quence. With increasing number of subsystems it is
(even for experienced co-simulation application engi-
S
1
y
11
u
21
y
12
u
41
S
2
y
21
u
31
S
3
y
31
u
42
S
4
y
41
u
32
y
42
u
11
Figure 1: Co-simulation Topology.
neers) hardly possible to define a well-defined trigger
sequence.
The connection between the inputs u
i
and outputs
y
i
of subsystems in the co-simulation is mostly the
single information which is available for the config-
uration problem. This relation can be described with
the linking matrix:
u = L · y. (1)
The linking matrix L is an orthogonal matrix
1
of the
dimension n, where n is the number of connections
between the subsystems. The connections of the co-
simulation topology from Figure 1 can be rewritten as
follows:
u
11
u
21
u
31
u
32
u
41
u
42
=
0 0 0 0 0 1
1 0 0 0 0 0
0 0 1 0 0 0
0 0 0 0 1 0
0 1 0 0 0 0
0 0 0 1 0 0
y
11
y
12
y
21
y
31
y
41
y
42
, (2)
where u = [u
1
, u
2
, . . . , u
n
]
T
is the input vector and y =
[y
1
, y
2
, . . . , y
n
]
T
represents the output vector. A fully
description of the co-simulation is given by this rela-
tion. Nevertheless this relation does not give any in-
formation about the dependencies between the several
subsystems. Therefore an other relation is needed,
which describes a formal relation between each sub-
system. Instead of the linking matrix L which de-
scribes the connection between inputs and outputs,
the matrix D describes the dependency of the sub-
systems themselves. This relation can be written as
follows:
D =
T
T
· L · S
T
. (3)
The matrix D is a m × m matrix and represents the
adjacency matrix, i.e. the dependency from one sub-
system to another. The matrices S and T are n × m
dimensional and describe the correlation between the
subsystems and the connections, where S describes
the allocation of the source y
i
to the subsystems and T
1
In order to ensure orthogonality for multiply connected
outputs, these outputs are duplicated, i.e. each output has
exactly one connection line.
Optimal Trigger Sequence for Non-iterative Co-simulation
81

the allocation of the target u
i
to the subsystems. The
columns represent the inputs of each subsystem and
the rows their outputs.
The linking matrix is a dependency description of
the connection, but it does not give any information of
the dependency of the subsystems. Regarding to the
trigger sequence the dependency of the subsystems is
more relevant.
The system dependency D of the co-simulation
topology from Figure 1 is given as follows:
D =
− 1 0 1
0 − 1 0
0 0 − 1
1 0 1 −
(4)
A more general description of the dependency matrix
D can be written as follows:
D =
T
T
· C · L · S
T
, (5)
with C as a diagonal matrix of weighted inputs
c
1
, c
2
, . . . , c
n
. With this additional weights c
i
it is pos-
sible to increase the relevance of several inputs y
i
and
thus to change the dependency between subsystems.
3.1 Extended TSP Problem
The co-simulation network can be interpreted as an
asymmetric, directed graph. The subsystems repre-
sent the nodes and the edges are the connections be-
tween the subsystems. The number of signals from
one subsystem to another weights the edge, and so
the number of extrapolated inputs. The co-simulation
graph is depicted in Figure 2. The nodes represent the
several subsystems from Figure 1 and the edges the
coupling signals between the subsystems.
c
12
c
14
c
41
c
23
c
34
c
43
S
1
S
2
S
3
S
4
Figure 2: Co-simulation Graph.
In this context the trigger sequence can be inter-
preted as a Hamiltonian cycle, where every node or
subsystem is exactly visited once. In the case that the
C is the identity matrix (i.e. all coefficients in the di-
agonal c
i j
= 1), the value of the edges represents the
number of extrapolated inputs. If for example node
3 is visited (i.e. subsystem S
3
is calculated) the sum
of incoming edges represents the number of extrapo-
lated coupling signals for this subsystem. Regarding
to the adjacency matrix D that means the sum of the
node’s column, see (6).
D =
0 c
12
0 c
14
0 0 c
23
0
0 0 0 c
34
c
41
0 c
43
0
(6)
If the node 3 is already visited, the subsystem has cal-
culated and the results are available. There is no ex-
trapolation needed any more for these coupling sig-
nals, i.e. the row of the node has to set to zero, see
(7).
D =
0 c
12
0 c
14
0 0 c
23
0
0 0 0 0
c
41
0 c
43
0
(7)
The more nodes or subsystems are visited the more
coefficients become zero and the less inputs have to
be extrapolated.
Based on the graph representation of the co-
simulation network, the optimal trigger sequence re-
sults from the shortest path between all nodes. A gen-
eral description of the optimization problem can be
written as follows:
min
∑
i
∑
j\i
∑
k\I
c
k j
x
i j
(8)
The coefficients c
i j
represent the value of the edges
and x
i j
is 0 or 1 according if a path or node is visited.
Starting from each row i, every column j is consid-
ered. The coefficients c
k j
along the column j are ac-
cumulated up. Already visited nodes or columns I are
not considered.
Nowadays a typical co-simulation problem in-
cludes less than ten subsystems and so in general the
dimension of the adjacency matrix is lower than ten.
Therefore the brute-force solving strategy for the co-
simulation graph is sufficient and can be solved with
low effort. With less than 5 nodes or subsystems
the brute-force solving method is faster than alterna-
tive solving algorithms. The calculation effort of the
brute-force and other algorithm to solve the TSP prob-
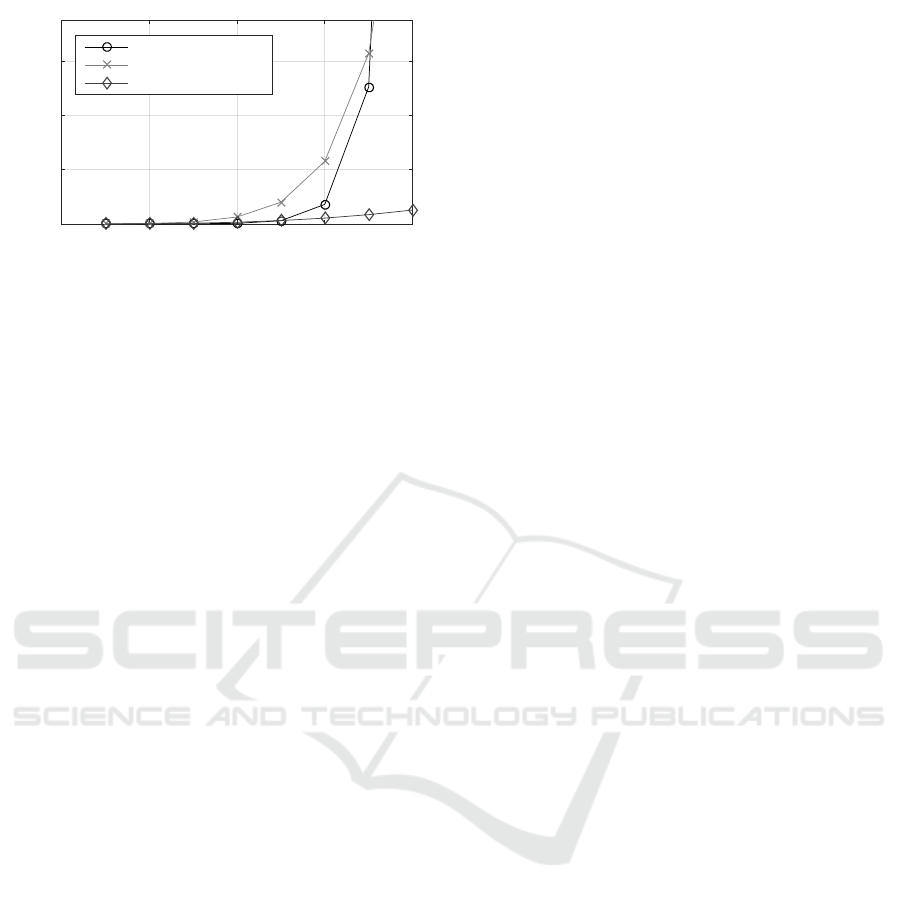
lem is compared in Figure 3.
Nevertheless, with an increasing number of sub-
systems the calculation effort of the brute-force ap-
proach significantly increases and so other solving
strategies with lesser computation effort should be
used.
SIMULTECH 2019 - 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications
82
0 2 4 6 8
Number of nodes
Calculation e,ort
Brute Force
Dynamic Programming
Nearst Neighbour
Figure 3: Calculation effort to solve a TSP problem.
However, it turns out that without any other in-
formation the subsystem within the lowest number
of inports should be calculated first (Holzinger and
Benedikt, 2019).
It is obvious that, if no more information is avail-
able, the minimal number of extrapolated inputs is a
desirable goal for a trigger sequence. To find this se-
quence the shortest way to connect all nodes has to
be found. The optimum trigger sequence can be de-
scribed as a modified travelling sales man problem
(TSP). In contrast to the original TSP, the (outgoing)
edges of already visited nodes have no impact to the
other nodes and thus these edges are set to zero.
3.2 Connection Properties
Depending on the relevance or impact of a connection
the weight of the signal c
i j
can influence the result
of the graph and consequently the trigger sequence.
This weighting can be influenced by different cou-
pling signal and subsystem properties (Benedikt and
Holzinger, 2016). In the following chapters some rel-
evant properties are discussed.
3.2.1 Subsequence
The subsequence is a subsystem property affected by
the subsystem solver, coupling time-step and the sub-
system interface. If the internal solving step size of a
subsystem, the so called micro step-size, is smaller
than the coupling step-size the subsystem provides
subsequence. Instead of a single value at the cou-
pling time-step, a sequence with the micro-steps is
given. These additional samples in the coupling sig-
nals generally allow more accurate simulation results
compared to single value coupling signals (coupling
time step equal micro time step).
3.2.2 Signal Types
The characteristic of the coupling signals is an impor-
tant information for the choice of a proper extrapo-
lation filter. The prediction of continuous signals is
easier than the estimation of a discontinuous or dis-
crete coupling signal. The most advanced extrapola-
tion and compensation techniques are based on con-
tinuous signal and so better to prevent the extrapola-
tion of discontinuous and discrete signals.
3.2.3 Direct Feed-through
The direct impact of an input to an output signal is one
of the most important properties of subsystem. Espe-
cially if the direct feed-through channels are formed
in a closed loop and affects an algebraic loop. An di-
rect feed-through indicates that an uncertainty at the
input signal (e.g. caused by an extrapolation filter)
means an uncertainty at the output signal. The ex-
trapolation of such inputs should be avoided.
3.2.4 Subsystem Dynamic
An other indicator for the extrapolation and trigger se-
quence of a subsystem is its dynamical behaviour. A
high system dynamic indicates that uncertainty at the
input of a system has a high impact to the output. And
on the other hand that low dynamic systems barely re-
act to any changes and discontinuities to the input. So
the extrapolation of inputs of slow input-output dy-
namics should be preferred instead of inputs of high
dynamic subsystems.
3.2.5 Coupling Signal Frequency
In addition to the system dynamic the frequency of the
coupling signal represents a further information for
the extrapolation filter setting and trigger sequence. It
is obvious that it is easier to extrapolate a signal with
low frequency components. However, coupling sig-
nals with high frequency components are not implic-
itly signals which should be prevented to extrapolate.
If the following system has a low pass characteristic,
high frequencies and uncertainty at the input signal
are not critical. The combined consideration of the
individual input-output dynamics and the signal fre-
quency determines if extrapolation shall be applied.
3.3 Contribution of Expert Knowledge
To combine the connection properties fuzzy logic is
used. The output of the fuzzy algorithm represents
the weight of the input signal c
i j
and for the inputs
the results of the signal quantities are used. Expert
Optimal Trigger Sequence for Non-iterative Co-simulation
83
Simulation Results
Subsequence
Signal Types
Feed-Through
Local Dynamics
Coupling Signal
Frequency
Subsystem Solver
···
Fuzzy Logic/ Expert Knowledge
c
i j
D = (T
T
· C · L · S)
T
Solve Optimization Problem
D
Set Co-Simulation Configuration
{
S
2
, S
1
, ...
}
Figure 4: Schematic procedure to get the trigger sequence.
knowledge is used to describe rules and transfer the
properties of each coupling signal to a weight of the
co-simulation graph.
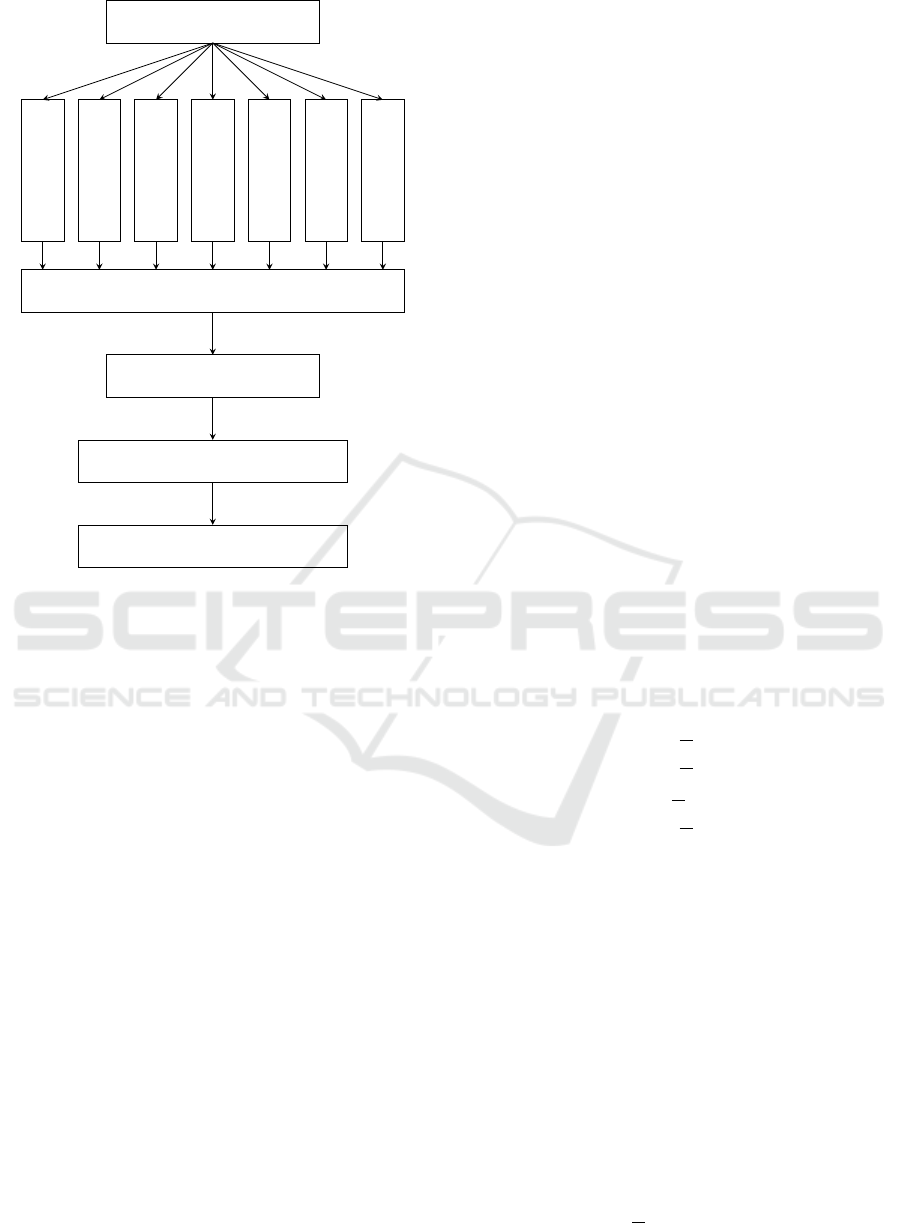
A schematic procedure to determine the trigger
sequence is shown in Figure 4. The procedure
starts with the results of a successfully simulated co-
simulation. The results are used for the signal analy-
sis, where the properties of the signals are computed,
like signal types, direct feed-through, etc. The in-
formation is merged into the fuzzy logic, where the
expert knowledge is integrated. The fuzzy algorithm
support a weight for every connection. The weighted
matrix delivers with the source and target transfer ma-
trix the adjacency matrix D, which is solved to get an
optimized trigger sequence.
In the case, that no results are available to calcu-
late the weight of the connections (e.g. after a new
configuration), all coefficients of the weighted matrix
C are set to a default value c
i j
= 1. The resulting de-
fault trigger sequence has minimized the number of
inputs to extrapolate.
4 EXAMPLE
The following section shows an example to demon-
strate the described approaches. Therefore three
strategies are used to determine trigger sequences.
The first strategy considers the number of extrapo-
lated inputs. The second uses the property of direct
feed-through to determine the trigger sequence and
the third strategy or advanced strategy weights the
coupling signals by subsystem and signal behaviour.
The example consists of four subsystems S
1
, S
2
, S
3
and S
4
which are connected to another as shown in
Figure 1. The subsystems S
1
, S
3
and S
4
are based
on an example in (Benedikt and Drenth, 2018). The
subsystems describe the behaviour of spring-damper-
mass systems. The additional model S
2
is a super-
posed controller, which controls the output y
42
of the
subsystem S
4
.
4.1 Subsystem Description
The four subsystems are solved with a fixed-step size
solver (Euler) and a step size δT = 0.1 ms. The cou-
pling time steps is constant for all subsystems ∆T =
1ms. The extrapolation filters for all coupling signals
is set to ZOH.
The mathematical description of the several subsys-
tems is as follows:
4.1.1 Subsystem 1
The subsystem S
1
is a second order linear model with
one input u
11
and two outputs y = [y
11
, y
12
]
T
. The
parameters are set c = 1000 and J
1
= 0.1. There is
a direct feed-through d = 44.27 from the input u
11
to
y
12
.
˙
x =
"
0 −
1
J
1
c −
d
J
1
#
x +
1
d
u
y =
"
0
1
J
1
c −
d
J
1
#
x +
0
d
u
(9)
4.1.2 Subsystem 2
The second subsystem describes a PI-controller with
the parameter k
p
= 100 and k
i
= 0.1 of the form:
˙x = k
i
· x +(r − u)
y = x +k
p
· (r − u)
(10)
The set value r = 10 and the initial states of all sub-
systems are zero.
4.1.3 Subsystem 3
The subsystem S
3
has two inputs u = [u
31
, u
32
]
T
and
an output y
31
. The model has an integrative be-
haviour, there is no direct feed-through from the in-
puts to the output. The parameter J
3
= 0.9.
˙x =
1
J
3
· x +
1 −1
u
y = x
(11)
SIMULTECH 2019 - 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications
84
S4S3S2S1
S4S3S1S2
S4S2S3S1
S4S2S1S3
S4S1S2S3
S4S1S3S2
S3S4S2S1
S3S4S1S2
S3S2S4S1
S3S2S1S4
S3S1S2S4
S3S1S4S2
S2S3S4S1
S2S3S1S4
S2S4S3S1
S2S4S1S3
S2S1S4S3
S2S1S3S4
S1S3S2S4
S1S3S4S2
S1S2S3S4
S1S2S4S3
S1S4S2S3
S1S4S3S2
Trigger Sequence Permutation
0
1
2
3
4
Objective Function
Default Strategy: Extrapolated Inputs
Default Strategy: Direct Feed-Through
Advanced Strategy
Figure 5: Evaluation of the objective function regarding different strategies: default strategy based on the extrapolated inputs,
default strategy based on the direct feed-through and advanced strategy.
4.1.4 Subsystem 4
The model S
4
has two inputs u = [u
41
, u
42
]
T
and two
outputs y = [y
41
, y
42
]
T
with a direct feed-through d =
44.27 from the input u
42
to y
41
and the parameter are
set c = 1000 and J
4
= 0.5.
˙
x =
"
0 −
1
J
4
c −
d
J
4
#
x +
0 1
−1 d
u
y =
"
c −
d
J
4
0
1
J
4
#
x +
0 d
0 0
u
(12)
4.2 Default Strategies
The default strategies describe the possibility to de-
fine a trigger sequence without knowledge or with
reduced knowledge of the subsystem behaviours.
Therefore no additional analysis of the coupling sig-
nals is needed to determine a trigger sequence for the
subsystems.
4.2.1 Extrapolated Inputs
In a first step without any additional information the
weight of all coupling signals is set to one, c
i
= 1, and
so the adjacency matrix D of the graph is given by (4).
In this case the linking matrix L is the single required
information to determine a trigger sequence. The
resulting trigger sequence represents the minimum
number of extrapolated inputs. The best solutions of
the default matrix are
{
S
4
, S
1
, S
2
, S
3
}
,
{
S
1
, S
2
, S
3
, S
4
}
,
{
S
1
, S
2
, S
4
, S
3
}
and
{
S
1
, S
4
, S
2
, S
3
}
. All of these so-
lution delivers a minimum according to (8). Each
for each of the resulting trigger sequences two inputs
have to be extrapolated in each simulation step.
4.2.2 Direct Feed-through
If the direct feed-through of a system is known by
the application engineer, this information can be
used as prior knowledge to define a trigger sequence.
Some subsystems also provide this information, e.g.
the FMI standard supports the information of direct
feed-through (Blochwitz et al., 2012).
The subsystems of the considered example have
three inputs with a direct feed-through to an output.
A reduced adjacency matrix D with the direct feed-
through is given as follows:
D =
− 1 0 0
0 − 0 0
0 0 − 1
1 0 0 −
, (13)
where each input with a feed-through to an output is
set to one. Based on the adjacency matrix D from
(13) the best solution is
{
S
3
, S
4
, S
1
, S
2
}
. The resulting
trigger sequence represents the minimum number of
inputs with a direct feed-through to an output. In the
example exits one configuration, where no input with
a direct feed-through has to be extrapolated.
4.3 Advanced Strategy
By the advanced strategy the default weights are mod-
ified based on the identified connection properties.
The adjacency matrix D can be rewritten as follows:
D =
− 1 0 0.35
0 − 0.25 0
0 0 − 0.72
0.67 0 0.25 −
(14)
The integrative behaviour of subsystem S
3
reduces the
coefficients c
23
= c
43
= 0.25. On the other hand, the
Optimal Trigger Sequence for Non-iterative Co-simulation
85
coefficients c
12
,c
34
and c
41
of the coupling inputs with
direct feed-through behaviour are still high.
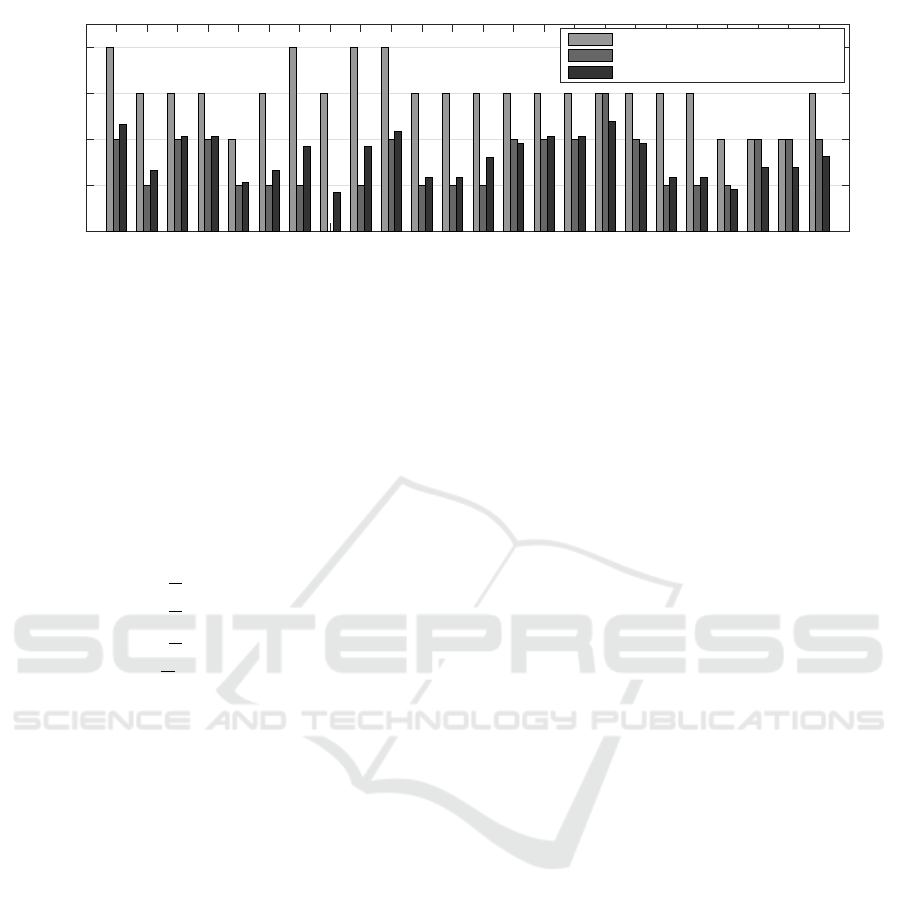
The cost to solve the objective function for all pos-
sible trigger sequences with respect to the three dis-
cussed strategies are illustrated in Figure 5. The two
default strategies show the evaluation of the objec-
tive function based on the minimum number of ex-
trapolated inputs with respect to (4) and the minimum
number of extrapolated direct feed-through channels
with the adjacency matrix (13). The advanced strat-
egy show the results of (14). The best solution deliv-
ers the trigger sequence
{
S
3
, S
4
, S
1
, S
2
}
.
The default strategy based on the direct feed-
through and the advanced strategy provides the same
trigger sequence. This is due the fact, that the sub-
system properties are mainly characterized by the di-
rect feed-through. Nevertheless, in examples with
more coupling signals or if the property of direct feed-
through is not known, the presented (advanced) ap-
proach helps to get a proper trigger sequence.
The determined solution
{
S
3
, S
4
, S
1
, S
2
}
is not
from the optimal set, which was calculated with
the default strategy based on the minimum number
of extrapolated inputs
{
S
4
, S
1
, S
2
, S
3
}
,
{
S
1
, S
2
, S
3
, S
4
}
,
{
S
1
, S
2
, S
4
, S
3
}
and
{
S
1
, S
4
, S
2
, S
3
}
. The solutions
from the default strategy have a minimal number of
extrapolated inputs, but all of the solutions extrap-
olate an input signal of a subsystem with a direct
feed-through property. The direct feed-through can
be interpreted as an additional extrapolation of corre-
sponding output signal and will cause additional cou-
pling errors.
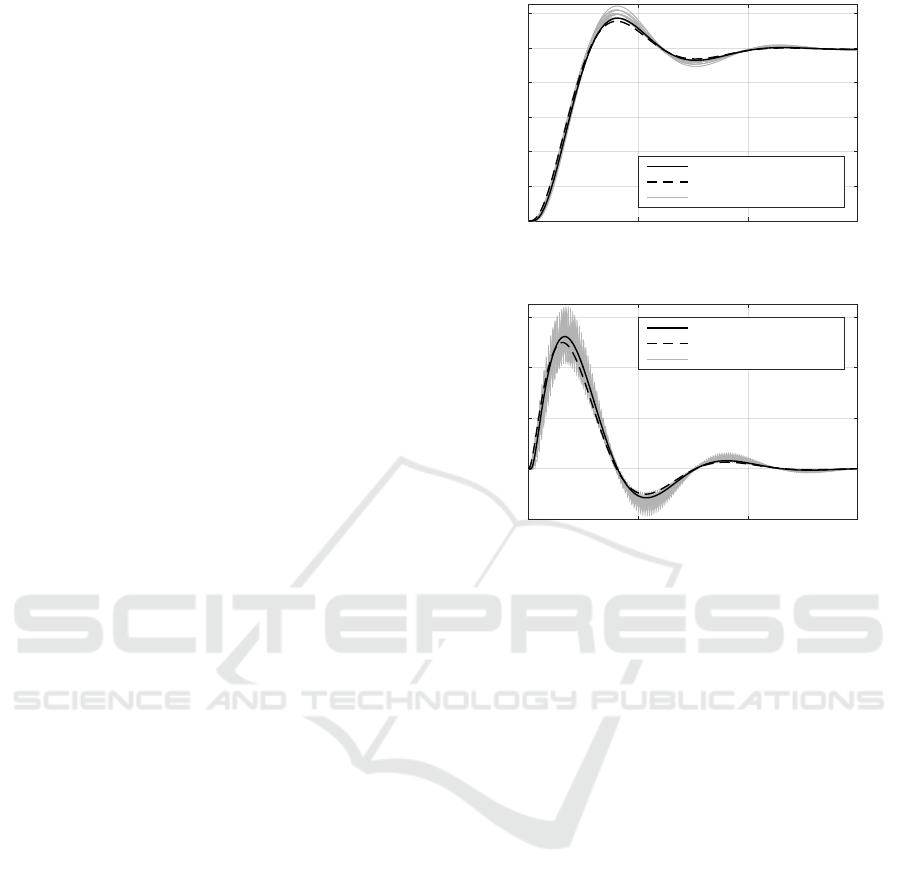
The results of the subsystem S
1
are shown in Fig-
ure 6. The dashed plot in both figures represents the
monolithic simulation result, where all subsystems
were simulated assembled and with one solver in a
single simulation environment. The grey lines show
the results of all possible permutations of the trigger
sequence. The black solide line is the result of the
trigger sequence
{
S
3
, S
4
, S
1
, S
2
}
from the presented
approach.
The result of the determined trigger sequence is
next to the monolithic simulation and has smaller
difference compared to the remaining permuted se-
quences. In the determined solution no input with di-
rect feed-through behaviour was extrapolated and so
the coupling error was reduced although that an ad-
ditional input has to be extrapolated compared to the
default solutions.
The other way around the solutions with high ef-
forts depicts a discrepancy to the monolithic solu-
tion. Nevertheless, even the best solution show dis-
crepancy between the monolithic solution caused by
the delay of the extrapolation filter. To compen-
0 0.05 0.1 0.15
Simulation Time in s
0
2
4
6
8
10
12
S
1
, y
11
Optimal Trigger Sequence
Monolithic Simulation
Permuted Trigger Sequence
0 0.05 0.1 0.15
Simulation Time in s
-20
0
20
40
60
S
1
, y
12
Optimal Trigger Sequence
Monolithic Simulation
Permuted Trigger Sequence
Figure 6: Simulation results of the permutation, monolithic
simulation and the optimal trigger sequence {S
3
, S
4
, S
1
, S
2
}.
sate these remaining difference advanced extrapola-
tion techniques can be used (Benedikt and Hofer,
2013) (Benedikt et al., 2013).
The presented approach provides an optimized
trigger sequence for sequential co-simulation. Nev-
ertheless it is obvious that the simulation duration for
sequential co-simulation is higher compared to par-
allel co-simulation, where each subsystem can cal-
culate at the same time. On the other hand, gener-
ally, sequential co-simulation delivers better simula-
tion results, due the fact, that less inputs have to be
extrapolated. Hierarchical co-simulation is a method
to get a trade-off between simulation accuracy from
and simulation duration. A configuration approach
for hierarchical co-simulation based on the number of
extrapolated inputs and calculation effort of the sev-
eral subsystems was already discussed by the authors
(Holzinger and Benedikt, 2019).
5 CONCLUSIONS
The article addresses an approach to determine
an optimized trigger sequence for non-iterative co-
simulation. Therefore expert knowledge e.g. in form
of fuzzy rules is used to assess the extrapolation qual-
SIMULTECH 2019 - 9th International Conference on Simulation and Modeling Methodologies, Technologies and Applications
86

ity of each input, based on the properties of the cou-
pling signals. The so resulting weighted connections
are used to describe a co-simulation graph. The so-
lution of the graph delivers an optimized trigger se-
quence.
If no additional information in terms of simulation
results is available to calculate the connection proper-
ties, the approach delivers a default solution related
to the minimum number of extrapolated coupling sig-
nals. After each simulation run the simulation results
can be used to calculate the connection properties and
finally weight the graph to get an optimal trigger se-
quence. This information can also be used to set the
time-step and the extrapolation filter or at least to as-
sess the co-simulation.
In a further work, the presented approach will
be extended for the configuration of hierarchical co-
simulation, so that some subsystems can be calcu-
lated in parallel and others sequentially. This allows a
trade-off between simulation accuracy and simulation
duration.
REMARK PATENT
The presented work describes a part of a novel au-
tomatic configuration approach for co-simulation of
distributed components. Protected by a pending Eu-
ropean patent (Benedikt et al., 2016) the outlined
schemes are supported by the co-simulation platform
Model.CONNECT
TM
(AVL, 2018) from AVL.
ACKNOWLEDGMENT
This work was accomplished at the VIRTUAL VE-
HICLE Research Center in Graz, Austria. The au-
thors would like to acknowledge the financial sup-
port of the COMET K2 - Competence Centers for
Excellent Technologies Programme of the Austrian
Federal Ministry for Transport, Innovation and Tech-
nology (bmvit), the Austrian Federal Ministry of Sci-
ence, Research and Economy (bmwfw), the Austrian
Research Promotion Agency (FFG), the Province of
Styria and the Styrian Business Promotion Agency
(SFG).
REFERENCES
AVL (2018). Model.CONNECT
tm
, the neutral model inte-
gration and co-simulation platform connecting virtual
and real components. (https://www.avl.com/-/model-
connect). [Online; accessed 31.01.2019].
Benedikt, M., Bernasch, J., Holzinger, F., and Watzenig, D.
(2016). Method for configuration a co-simulation for
a total system. EP3188053 (A1) — 2017-07-05.
Benedikt, M. and Drenth, E. (2018). Relaxing stiff sys-
tem integration by smoothing techniques for non-
iterative co-simulation. In IUTAM Symposium on Co-
Simulation and Solver-Coupling.
Benedikt, M. and Hofer, A. (2013). Guidelines for the ap-
plication of a coupling method for non-iterative co-
simulation. In Modelling and Simulation (EUROSIM),
2013 8th EUROSIM Congress on, pages 244–249.
Benedikt, M. and Holzinger, F. R. (2016). Automated con-
figuration for non-iterative co-simulation. In 2016
17th International Conference on Thermal, Mechan-
ical and Multi-Physics Simulation and Experiments
in Microelectronics and Microsystems (EuroSimE),
pages 1–7.
Benedikt, M., Stippel, H., and Watzenig, D. (2010). An
adaptive coupling methodology for fast time-domain
distributed heterogeneous co-simulation. In SAE 2010
World Congress and Exhibition. SAE International.
Benedikt, M., Watzenig, D., Zehetner, J., and Hofer, A.
(2013). Macro-step-size selection and monitoring of
the coupling errof for weak coupled subsystems in the
frequency-domain. In Proceedings, pages 1–12. .
Blochwitz, T., Otter, M.,
˚
Akesson, J., Arnold, M., Clauss,
C., Elmqvist, H., Friedrich, M., Junghanns, A.,
Mauss, J., Neumerkel, D., Olsson, H., and Viel,
A. (2012). Functional mockup interface 2.0: The
standard for tool independent exchange of simulation
models. In Proceedings of the 9th International Mod-
elica Conference, pages 173–184. The Modelica As-
sociation.
Busch, M. and Schweizer, B. (2011). An explicit approach
for controlling the macro-step size of co-simulation
methods. In Bernadini, D., editor, Proceedings of
the 7th European Nonlinear Dynamics Conference
(ENOC 2011): July 24 - 29, 2011, Rome, Italy, pages
1–6.
Holzinger, F. R. and Benedikt, M. (2019). Hierarchi-
cal Coupling Approach Utilizing Multi-Objective Op-
timization for Non-Iterative Co-Simulation, volume
1348, pages 735–740.
K
¨
ubler, R. and Schiehlen, W. (2000). Modular simulation
in multibody system dynamics. Multibody System Dy-
namics, 4.
Optimal Trigger Sequence for Non-iterative Co-simulation
87
