Convexiﬁcation of Semi-activity Constraints Applied to Minimum-time

Optimal Control for Vehicles with Semi-active Limited-slip Differential

Tadeas Sedlacek

1,2 a

, Dirk Odenthal

1 b

and Dirk Wollherr

2 c

BMW M GmbH, Daimlerstr. 19, 85748 Garching near Munich, Germany

Chair of Automatic Control Engineering, Department of Electrical and Computer Engineering, Technical University of

Munich, Theresienstr. 90, 80333 Munich, Germany

Keywords:

Minimum Lap Time, Semi-active Limited-slip Differential, Convexiﬁcation, Time Optimal Control,

Hermite-Simpson Collocation, Vehicle System Dynamics.

Abstract:

Semi-active actuators provide a good compromise between low energy consumption and high performance.

Thus, they are deployed in many engineering applications, often combined with other actuators into complex

systems requiring an integrated control concept for optimal performance. Optimal control can be used to

objectively evaluate the performance of such systems as well as to deduce optimal control input trajectories

and optimal passive system designs. We present a novel approach which enables considering a broad class

of semi-active actuators in optimal control problems via convex sets. This procedure is exemplarily depicted

for semi-active limited-slip differentials which are used in automotive applications for lateral torque distribu-

tion. The performance beneﬁt gained by installing a semi-active limited-slip differential at the rear axle of

a vehicle is objectively quantiﬁed by numerically computing time-optimal trajectories on a racetrack via di-

rect optimal control with Hermite-Simpson collocation. Although the overall problem remains nonconvex for

this particular application, this procedure is a ﬁrst step towards a fully convex implementation. By iteratively

increasing the upper boundary for the differential torque in multiple optimisations, we identify the smallest

upper differential torque boundary for optimal laps and determine the lap time sensitivity regarding this limit.

1 INTRODUCTION

Semi-active actuators are used in many engineer-

ing applications since they provide a good com-

promise between low energy consumption and high

performance (Savaresi et al., 2010). For instance,

semi-active dampers are deployed in automotive sus-

pension systems (Savaresi et al., 2010), in landing

gears for aircrafts (Kr

uger, 2000), in robotics to im-

prove running (Kim et al., 2018), in architectural and

bio-mechanical structures and many more (Poussot-

Vassal et al., 2010). Further examples for semi-active

actuators in the ﬁeld of automotive engineering are

semi-active limited-slip differentials (SLDs) for lat-

eral torque distribution, semi-active transfer cases for

longitudinal torque allocation and airsprings for an

adjustable stiffness (Cheli et al., 2006; Savaresi et al.,

2010). This paper focusses on SLDs which enable

transferring torque from the faster spinning wheel to

https://orcid.org/0000-0002-6191-6173

https://orcid.org/0000-0002-6651-8369

https://orcid.org/0000-0003-2810-6790

the slower one. Since only the amount of torque trans-

ferred by the differential can be controlled, whereas

the direction of the torque transfer is dictated by

the wheel speed difference, these actuators represent

semi-active components. SLDs improve the corner-

ing performance of vehicles by enhancing accelera-

tion potential and yaw agility (Cheli et al., 2006). Lap

times on racetracks can be used as a metric to objec-

tively evaluate these beneﬁts in vehicle performance.

The time-optimal input trajectories for the multiple

actuators of a vehicle under consideration of actuator

limitations and track boundaries can be deduced by

optimal control methods.

Optimal control has been successfully applied to

compute input trajectories for limited-slip differen-

tials (LDs) aiming at minimum lap times on race-

tracks. Passive LDs have been analysed via opti-

mal control methods in (Kelly, 2008; Perantoni and

Limebeer, 2014; Limebeer et al., 2014; Limebeer

and Perantoni, 2015; Tremlett et al., 2015), whereas

optimal trajectories for SLDs have been identiﬁed

in (Tremlett and Limebeer, 2016). All approaches

Sedlacek, T., Odenthal, D. and Wollherr, D.

Convexiﬁcation of Semi-activity Constraints Applied to Minimum-time Optimal Control for Vehicles with Semi-active Limited-slip Differential.

DOI: 10.5220/0009593600150025

In Proceedings of the 17th International Conference on Informatics in Control, Automation and Robotics (ICINCO 2020), pages 15-25

ISBN: 978-989-758-442-8

have in common that the torque transmitted by the dif-

ferential is depicted as a function of the wheel speed

difference. In the simplest form, the wheel speed dif-

ference is scaled by a factor which is either ﬁxed for

passive LDs or represents a system input when SLDs

are considered. The computed differential torque has

been either inserted into the differential equations of

the system (Kelly, 2008) or has been considered by

equality constraints (Perantoni and Limebeer, 2014;

Limebeer et al., 2014; Limebeer and Perantoni, 2015;

Tremlett et al., 2015; Tremlett and Limebeer, 2016).

Except for (Kelly, 2008; Tremlett et al., 2015), only

quasi steady-state wheel dynamics have been consid-

ered for the wheel speed to simplify the optimisation

problem.

In this paper, we revisit the optimal control prob-

lem (OCP) introduced in (Sedlacek et al., 2020b) and

augment the baseline vehicle model by a SLD at the

rear axle. Since the rotational wheel speeds are deci-

sive variables for LDs, the corresponding differential

equations are included into the system dynamics. Fur-

thermore, we consider maximum differential torque

boundaries for the SLD. Formulating the contem-

plated OCP via the methods used in previous papers

would result in either quadratic equality constraints or

nonconvex sets, both prohibiting a fully convex OCP

beforehand (Boyd and Vandenberghe, 2004). Using

the SLD as an example, we present a novel OCP-

modelling approach for semi-active components with

convex subsets in the two quadrants dictated by the

passivity constraint (Savaresi et al., 2010). The non-

convex set is transformed into two convex ones by

separate consideration of positive and negative con-

trol inputs. The downside of this method is the re-

quirement of an extra input and a generally higher

number of constraints. However, this convexiﬁcation

procedure is a ﬁrst step towards a fully convex OCP

for such vehicles. The correctness of the approach is

conﬁrmed by analysing the resulting optimal trajec-

tories. Finally, we identify the smallest upper differ-

ential torque boundary for optimal laps on a speci-

ﬁed racetrack and determine the lap time sensitivity

regarding this limit.

The remainder of this paper is organised as fol-

lows. The considered track model and vehicle model

are presented in section 2. The OCP aiming at com-

puting trajectories for minimum lap time is formu-

lated in section 3 and its solution is discussed in sec-

tion 4. Section 5 concludes the paper and gives an

outlook for future work. The appendix contains fur-

ther modelling details and parameter values.

2 VEHICLE MODEL

A nonlinear two-track vehicle model in combination

with a ﬂat track model is used to investigate opti-

mal lap trajectories for the Nuerburg-ring Grand-Prix

course. The topview of the vehicle model with re-

spect to the racetrack is illustrated in ﬁgure 1. We in-

troduce the index k ∈ K

= {1, 2,3,4} to distinguish

the individual wheels and make use of several co-

ordinate frames. Vectors given in the body coordi-

nate frame, which is coupled with the centre of grav-

ity (COG) of the vehicle and rotated by the yaw angle

ψ around the z-axis of the inertial frame, are marked

with the superscript b. Analogously, the wheel coor-

dinate frame w is the result of a rotation by the front

steering angle δ

. Calligraphic symbols denote vector

components given in the corresponding wheel coordi-

nate frame.

Drive torque generated by a combustion engine

is transmitted via a gear box and a SLD to the rear

wheels. The wheel-based engine torque T

considers

gear ratios and constant efﬁciencies of transmission

and SLD. The drive torque transferred laterally by

the SLD is denoted with T

. The front wheels are

mounted on individual axles and are thus decoupled.

Wheel-independent brake torques T

br,k

facilitate a lon-

gitudinal and lateral brake torque allocation. We as-

sume that the differential only transfers drive torque

and brake torque is solely allocated via the braking

system. This yields the individual wheel torques



















−T

br,1

−T

br,2

+ T

) − T

br,3

− T

) − T

br,4







. (1)

As will be shown in section 3.2.3, it is beneﬁcial to

split the differential torque into a positive and negative

tot

1/κ

x,3

y,3

x,4

y,4

x,1

y,1

x,2

y,2

Figure 1: Top view of vehicle model on a racetrack with

centre line R and track border B (Sedlacek 2020a).

ICINCO 2020 - 17th International Conference on Informatics in Control, Automation and Robotics

part according to

= T

+ T

−

. (2)

The total torque and total brake torque are computed

tot

∑

k=1

and T

br,tot

∑

k=1

br,k

, (3)

respectively. Furthermore, we assume δ

= δ

and δ

≡ 0 ≡ δ

. The n

= 8 system inputs are accu-

mulated in the input vector



−



∈ R

(4a)

with T



br,1

br,2

br,3

br,4



. (4b)

The longitudinal and lateral vehicle speed at the

COG in the body reference frame are denoted with

and v

, respectively. The yaw rate of the vehi-

cle and the rotational wheel speeds are represented

ψ and ω

, respectively. The vehicle motion is

described using wheel forces which are represented

by F



x,k

y,k



and F



x,k

y,k



in the

body and the corresponding wheel coordinate frame,

respectively. The wheel forces depend on the wheel

loads and wheel slips, as speciﬁed in the appendix.

The longitudinal drag force F

air,x

and the rolling resis-

tance torque T

roll,y,k

are given by (36) and (37), respec-

tively. In the subsequent equations, we use following

parameters: vehicle mass m, vehicle inertia J

, dis-

tance from COG to front axle l

or to rear axle l

, track

width of front axle b

or rear axle b

, dynamic rolling

radius of the corresponding tire r

and inertia of the

corresponding wheel-unit J

. Applying Newton’s sec-

ond law (Schramm et al., 2014) provides equations for

the translational motion



˙v





∑

k=1

x,k

+ F

air,x

∑

k=1

y,k



| {z }



ψv

−

ψv



(5a)

and the yaw movement of the vehicle

ψ =

− Σ

+ ∆

(5b)

= F

y,1

+ F

y,2

and Σ

= F

y,3

+ F

y,4

(5c)

∆

= F

x,2

− F

x,1

and ∆

= F

x,4

− F

x,3

(5d)

as well as for the rotational wheel motion



− F

x,k

+ T

roll,y,k



∀k ∈ K. (5e)

For the modelling of the SLD, we deﬁne the wheel

speed difference of the rear axle and the correspond-

ing differential equation as

= ω

− ω

and

−

, (5f)

respectively. In order to avoid an algebraic loop when

computing the wheel loads via (38a), delayed accel-

erations, given in the body reference frame, are in-

troduced using ﬁrst-order lag elements with time con-

stant τ

acc

˙a

acc



− a



∀ j ∈ {x, y}. (5g)

These low-pass ﬁlters can be motivated by neglected

chassis dynamics (Bianco et al., 2019) and the dy-

namic tire force generation (Sedlacek et al., 2020b).

The centre line of the racetrack is denoted with R

and characterised by its curvature κ

over the arc

length s

. The track boundary B is described using

the arc length-dependent track half-width d

. The

connection between vehicle model and track is estab-

lished via curvilinear coordinates using (Sharp et al.,

2000)

˙s

cos(Θ) − v

sin(Θ)

1 − d

, (6a)

= v

sin(Θ) + v

cos(Θ) and (6b)

Θ =

ψ − κ

˙s

. (6c)

The difference between vehicle orientation and tan-

gent angle of the reference line Θ

is given by the

tangent error angle Θ = ψ − Θ

. The lateral devia-

tion d

describes the normal distance of the tangent

to the COG of the vehicle.

Using (5) and (6), the system behaviour is char-

acterised by a continuous, input nonafﬁne, nonlinear

differential equation system

x = f(x,u,p) ∈ R

(7)

with the state vector

x =



veh

ref



with x

ref





, (8a)

veh



ψ ω



(8b)

and ω





. (8c)

Furthermore, the values of the model parameters p are

given in table 2.

3 OPTIMAL CONTROL

PROBLEM

In this section, we present the nonconvex OCP for

completing a lap in minimum time using the model

introduced in section 2. Before formulating the con-

straints and cost function of the OCP in sections 3.2

and 3.3, several preliminaries are outlined in sec-

tion 3.1. Model approximations are introduced to pro-

vide smooth system behaviour and avoid singularities,

Convexiﬁcation of Semi-activity Constraints Applied to Minimum-time Optimal Control for Vehicles with Semi-active Limited-slip

Differential

the free ﬁnal time OCP is transformed into an OCP

with ﬁxed ﬁnal arc length and the OCP is scaled to

enhance convergence rate.

The OCP is transcribed via Hermite-Simpson col-

location and posed using the domain-speciﬁc mod-

elling language JuMP (Dunning et al., 2017) for

mathematical optimisation embedded in the program-

ming language Julia. The resulting nonlinear pro-

gram is solved with IPOPT which uses a primal-dual

interior-point strategy with a ﬁlter line-search and fur-

ther features for performance enhancement (W

achter

and Biegler, 2006). Forward Mode Automatic Differ-

entiation is embedded in the JuMP-framework yield-

ing the necessary gradients and Hessians in advance

in machine precision.

3.1 Preliminaries

We adopt the smooth approximations introduced

in (Sedlacek et al., 2020a) to provide a differentiable

system for the computation of the derivatives. On the

one hand, we smooth the longitudinal slips (42a) via

x,k

≈

∆

v,k



v,k

∆

v,k

+ ε

λ,x



(9a)

with ∆

v,k

= ω

− v

x,k

and (9b)

v,k

= ω

+ v

x,k

∀k ∈ K. (9c)

On the other hand, the total slips in (43c) are replaced

≈

n,x,k

+ λ

n,y,k

+ ε

λ,tot

∀k ∈ K (9d)

which also eliminates the singularity in (43d).

Minimum-time OCPs have a free ﬁnal time. To

generate a ﬁxed ﬁnal arc length problem, we change

the independent variable to the arc length of the ref-

erence line associating all inputs and states explicitly

to the position on the racetrack (Perantoni and Lime-

beer, 2014). This transformation requires a scaling of

the differential equation system according to

) =

∂x

∂s

˙s

f =

f. (10)

After the transformation, the elapsed time is retrieved

by the differential equation

) =

∂t

∂s

˙s

with t(s

R,0

) = 0. (11)

With transformation (10) in mind, we exchange the

independent variables in the state vector (8) resulting



veh

t d



. (12)

The corresponding differential equation in (7) is re-

placed with (11) and the right-hand side of the differ-

ential equation system is adapted according to (10).

The OCP is scaled according to (Sedlacek et al.,

2020a) to improve convergence speed. This includes

adapting the states and inputs, which represent deci-

sion variables when collocation is used, to lie within

the the range [−1,1]. Furthermore, constraints are

normalised to the domain [−1, 0]. The interrelations

between the scaled and non-scaled variables are given

x = S

˜x

x + k

˜x

⇔

x = S

−1

˜x

(

x − k

˜x

) (13a)

u = S

u + k

⇔ u = S

−1

(

u − k

) (13b)

u) = S

˜x

u). (13c)

Adequate values for the scaling matrices S

˜x

and S

as well as the shifting vectors k

˜x

and k

are given

in (Sedlacek et al., 2020a).

3.2 Constraints

This section presents the constraints for the contem-

plated optimisation problem which are used to con-

sider the system dynamics, set initial values, capture

non-modelled physical effects and avoid numerical

problems.

3.2.1 Collocation Constraints

We use Hermite-Simpson collocation to consider the

continuous system dynamics (13c) in the OCP. Thus,

the system inputs and the right-hand sides of the

differential equations are approximated by piecewise

quadratic polynomials. The integration interval s

∈

R,0

R, f

] is divided into n

seg

segments with n

coll

seg

+1 collocation points. Simpson quadrature is ap-

plied on each segment i = 0,. . .,n

seg

− 1 yielding the

collocation constraints

i+1

−

(

+ 4

i+1

) (14a)

(

i+1

) +

(

−

i+1

) (14b)

with the segment width h

= s

R,i+1

−s

R,i

(Betts, 2010;

Kelly, 2017). The margins of each segment repre-

sent collocation points with the corresponding values

(·)

and (·)

i+1

. Values at the midpoint are marked as

(·)

. All following constraints, except for the initial

conditions, are set at each collocation point and mid-

point but we omit the index for readability. Once the

optimal values at the collocation points and midpoints

are computed, the intermediate values for the inputs

and states are determined by piecewise quadratic and

ICINCO 2020 - 17th International Conference on Informatics in Control, Automation and Robotics

piecewise cubic polynomials, respectively. The cor-

responding formulas can be found in (Sedlacek et al.,

2020a).

3.2.2 Initial Values

The vehicle starts at the centre of the racetrack in

alignment with the reference line yielding d

= Θ =

ψ = 0. The ﬁrst track section is straight, thus lateral

speed, yaw rate and initial steering angles are set to

zero. By setting the initial longitudinal speed to a

small value v

= v

x,k

= 1

, we avoid the singularity

of the tire slip angles (42b) at vanishing speed. We

presume that the vehicle is initially in steady-state, no

brake torque is applied, the SLD is open and aerody-

namic forces are compensated by the rear tire forces.

Thus, accelerations are zero resulting in only static

wheel loads. Then, (5a) and (5e) enable the compu-

tation of the front and rear tire forces as well as the

engine torque according to (Sedlacek et al., 2020b)

x,1

roll,y,1

= F

x,2

, (15a)

x,3

(−F

air,x

− F

x,1

− F

x,2

) = F

x,4

, (15b)

= F

x,3

+ F

x,4

− T

roll,y,3

− T

roll,y,4

. (15c)

Using the tire forces (15a)-(15b), the longitudinal slip

can be computed from (43f) and the initial rotational

wheel speeds can be identiﬁed via (42a).

3.2.3 Input Constraints

Due to actuator limitations, box constraints are set for

the brake torques

0 ≤ T

br,k

≤ T

br,k

∀k ∈ K (16)

as well as for the front steering angle

− δ

≤ δ

∀k ∈ {1,2}. (17)

The wheel-based engine torque depends on the en-

gine characteristic as well as the gear ratios and efﬁ-

ciencies of transmission and SLD. Since we assume

a rigid connection between engine and rear axle, the

torque constraint is formulated utilising the rotational

speed of the rear axle

≈

(ω

+ ω

). (18)

Assuming constant mechanical efﬁciencies and an au-

tomatic selection of the optimal gear, the admissible

set for the wheel-based engine torque T

is approxi-

mated by the constraints

0 ≤ T

≤ T

and T

≤

e,pow

(19a)

using the maximum engine torque T

and maximum

engine power P

(Sedlacek et al., 2020b; Gillespie,

1992). We presume that the vehicle has a launch

control functionality which immediately provides the

maximum torque T

at the race start. Thus, the com-

bined constraint

e,con

= min(T

e,pow

) (20)

represents the upper boundary for the engine torque.

3.2.4 Semi-activity Constraint

Semi-active components do not introduce energy

into systems and thus fulﬁll the passivity con-

straint (Savaresi et al., 2010). In this section, we

present a novel convexiﬁcation procedure for semi-

active components which have a convex subset in

each of the two quadrants dictated by the passivity

premise. The approach is depicted using the SLD as

an example, whereas we compare our method with

previous approaches.

The SLD enables a lateral distribution of torque,

however the direction of the torque transfer depends

on the rotational speed difference ω

within the SLD.

As mentioned in section 1, previous approaches have

modelled the differential torque via

= ξ

(21)

or a more complicated version with trigonometric

functions. If T

and ξ

are chosen as system inputs,

the SLD can be included into the OCP using the con-

straints

= ξ

(22a)

−T

≤ T

(22b)

−T

≤ T

(22c)

with T

denoting the maximum differential torque of

the SLD. With ξ

and ω

being an input and state,

−ω

−T

−

Figure 2: Convexiﬁed set for SLD (exaggerated).

Convexiﬁcation of Semi-activity Constraints Applied to Minimum-time Optimal Control for Vehicles with Semi-active Limited-slip

Differential

both represent optimisation variables. Thus, equal-

ity constraint (22a) is quadratic in the optimisation

parameters and would prevent a convex OCP before-

hand (Boyd and Vandenberghe, 2004).

Alternatively, (21) can be inserted into the differ-

ential equations with ξ

being a system input. How-

ever, this would result in the nonconvex sets

−T

≤ ξ

≤ T

(23a)

−T

≤ ξ

≤ T

. (23b)

In this paper, we suggest splitting the differential

torque T

into a positive part T

and a negative

one T

−

which enables the formulation of the convex

sets

−T

≤ T

+ T

−

≤ T

(24a)

= {(T

,ω

)|T

∈



0,T



∧ g

≤ 0} (24b)

with g

= −T





+ ε

(24c)

−

= {(T

−

,ω

)|T

−

∈



−T



∧ g

−

≤ 0} (24d)

with g

−

= −T

−



−



+ ε

. (24e)

Figure 2 depicts sets (24b) and (24d). Being conﬁned

to the ﬁrst and third quadrant, the passivity constraint

is satisﬁed. By selecting a sufﬁciently small parame-

ter ε

= 10

−10

, the excluded area for simultaneously

small wheel speed differences ω

and small differen-

tial torques T

is negligible. Notice that with (24b)-

(24e) from ω

= 0 follows T

= T

= −T

−

which

results in T

= 0. However, we point out that this con-

vexiﬁcation comes at the price of a larger OCP due to

one extra input and additional constraints.

Theorem 1. The set given by (24) is convex.

Proof. Convexity of set (24) is proven using theorems

from (Boyd and Vandenberghe, 2004). Set (24a) rep-

resents a half-space which is convex. We denote the

graph associated with the epigraph (24c) by

f (T

)

−

. (25a)

The second derivative of (25a)

) =

2ε

> 0 ∀T

> 0 (25b)

is positive for the parameter range T

∈ T



0,T



given in (24b). Thus, the graph is convex in this

region and consequently (24c) represents a convex

set for T

∈ T

. Furthermore, the box constraint

∈ T

in (24b) deﬁnes a polyhedron which is also

convex. The intersection of convex sets results in a

convex set. Thus, set (24b) is convex. The convexity

proof of (24d) is equivalent, since sets (24b) and (24d)

are established analogously. Finally, the intersection

of the convex sets (24a), (24b) and (24d) yields a con-

vex set.

Remark 1. It is tempting to choose the set

= {(T

,ω

)|T

∈



0,T



∧ T

≥ 0}.

However, this set is nonconvex since it includes ar-

bitrary ω

, such as ω

= −c < 0, if T

= 0. If we

exclude T

= 0 by choosing the convex set

= {(T

,ω

)|T

∈



0,T



∧ T

≥ 0},

we still cannot guarantee that T

= 0 ∀ω

= 0.

Remark 2. The presented approach is applicable to

other semi-active actuators with convex subsets in

the ﬁrst and third quadrant. Considering semi-active

dampers with damper speed v

, a possible convex set

can be approximated by splitting the damper force ac-

cording to F

= F

+ F

−

and using the constraints

≤ F

≤ k

and F

≤

d,0

d,1

with analogous constraints for F

−

. Therein we use

coefﬁcients k

and k

to restrict the admissible area

yielding F

= 0) = 0 and employ an upper bound-

ary using a linear constraint with parameters

d,0

and

d,1

. Note that by using different constraint parame-

ters for F

and F

−

, differing characteristics for com-

pression and rebound can be implemented without

discarding smoothness of the OCP. Other convex sub-

sets for semi-active dampers are depicted in (Savaresi

et al., 2010).

3.2.5 State Constraints

Since driving backwards is not expedient on race-

tracks, positive longitudinal speeds and wheel speeds

are enforced by the box constraints

0 < v

≤ v

and 0 ≤ ω

≤ ω ∀k ∈ K. (26)

The yaw rate and steering angles are generally small

enough, especially on racetracks, that (26) results

in v

x,k

> 0 (Sedlacek et al., 2020b). This enables

the simpliﬁcation made in (42a) and avoids the singu-

larity in (42b).

In order to keep the vehicle on the racetrack, the path

constraints

− d

veh

≤ d

−

veh

(27)

limit the lateral deviation of the vehicle employing the

arc length-dependent track half-width d

and chassis

width b

veh

For an efﬁcient tire usage, we restrict the tire

ICINCO 2020 - 17th International Conference on Informatics in Control, Automation and Robotics

forces (43) to stay within the region of adhesion

which excludes sliding and thus decreases tire wear.

Since tire wear reduces the tire force potential, this

constraint is eligible. This is accomplished by the

load-dependent friction ellipse constraints for the tire

slips

x,k

y,k

− 1 ≤ 0 ∀k ∈ K (28)

using (42a), (42b) and (44b) (Sedlacek et al., 2020b).

When wheels lift off, no tire force can be gener-

ated. To avoid introducing additional discontinuities

as in (Perantoni and Limebeer, 2014) and since the

lift-off of wheels is undesired for industrial sports

cars, we enforce positive wheel loads (38a) with

z,k

> 0 ∀k ∈ K. (29)

Furthermore, the additional constraint

˙s

≥ ε

˙s

> 0 (30)

is employed to avoid dividing by zero when using the

transformation (10). Since we exclude driving back-

wards, this assumption is legitimate.

3.3 Objective

The cost function is comprised of three objectives ac-

cording to

J = J

+ J

˙u

+ J

. (31)

The main goal is minimising the required lap time

which is accomplished with

1dt =

R, f

R,0

˙s

= t(s

R, f

). (32)

To avoid non-unique solutions and therefore improve

convergence, a small regularisation term which is

quadratic in the inputs can be added to the objective

function (Kelly, 2017). Using Simpson quadrature

and the input derivative vector

), we include the

regularisation term

˙u

= ε

˙u

∑

j=1

seg−1

∑

i=0

˙u,ji

(33a)

˙u,ji

= ˆu

R,i

)

+ 4 ˆu



R,i



ˆu

R,i

+ h

)

(33b)

which generates a preference for smooth input solu-

tions. Smooth inputs are generally desirable for hu-

man drivers which justiﬁes the penalty term (33a).

The computation of the input derivatives is shown

in (Sedlacek et al., 2020a).

To enhance efﬁciency and durability, simultaneous

drive and brake torques are avoided by adding the ob-

jective (Sedlacek et al., 2020b)

norm

seg

−1

∑

i=0

η,i

(34a)

η,i

= T

R,i

) + 4 T



R,i



R,i

+ h

)

(34b)

R,i

) = T

R,i

br,tot

R,i

) (34c)

norm

= T

+ T

br,r

. (34d)

The unscaled torques are used in (34c), since they are

limited to positive values. Scaling factors ε

˙u

and ε

are chosen sufﬁciently small so that the modiﬁed ob-

jective (31) is nearly time-optimal.

4 RESULTS

The OCP presented in section 3 is solved using a dis-

cretisation mesh of 1000 equally spaced collocation

points yielding a mesh interval of about 2.5 metres.

The optimal trajectories for the vehicle with an open

differential at the rear axle instead of the SLD, which

we name setup C0, have been computed in (Sedlacek

et al., 2020b). Conﬁguration C1 represents a vehicle

with SLD at the rear axle and T

= 1250Nm. Us-

ing (38b), we deﬁne the torque shift variable as

= −

z,0,r

with F

z,0,r

= ρ

. (35)

It represents the lateral torque shift of the SLD nor-

malised by the static rear axle load. The torque shift

variable (35) is displayed in ﬁgure 3 together with

the acceleration and braking points which are char-

acterised by sign changes in T

tot

. The ﬁrst subplot of

ﬁgure 4 illustrates the track curvature and the lap time

advantage progression ∆t of C1. The wheel speed

difference and differential torque for C1 are shown

in the second subplot. The third subplot considers

setup C1 and depicts the individual wheel torques,

the total torque and the upper boundary for the engine

torque (20).

As expected, acceleration starts at the corner

apexes and high speed corners like



and



are

passed without braking. The lateral wheel load

shift during cornering results in different optimal

slips (44b) between the left and right wheel of an

axle. Thus, the SLD enhances traction conditions dur-

ing cornering by applying a higher drive torque to the

outer wheel at the expense of a lower drive torque at

the inner wheel. Only small torques are transmitted

Convexiﬁcation of Semi-activity Constraints Applied to Minimum-time Optimal Control for Vehicles with Semi-active Limited-slip

Differential

Figure 3: Normalised torque shift variable for lateral drive torque distribution via SLD at the rear axle with T

= 1250 Nm.

Symbols: ◦ 500m marks, M start acceleration, O start braking.

Figure 4: Track curvature, progression of lap time beneﬁt, wheel speed difference and torques.

by the SLD when driving on paths with small curva-

ture and no differential torque is applied during brak-

ing due to (24a) and (34a). The lateral torque distribu-

tion, either by the SLD or the braking system, affects

the yaw motion of the vehicle: An additional yaw mo-

mentum is generated by the difference in longitudinal

tire forces between the left and right side. When ac-

celerating after corner apexes, the lateral drive torque

allocation by the SLD increases agility, whereas a

stabilising momentum is induced by the braking sys-

tem before corners. The lateral drive torque distribu-

tion by the SLD is primarily performed after corner

apexes to enhance acceleration and agility. With a lap

time of 146.68 seconds, an overall improvement of

2.37 seconds or 1.59% is achieved, whereas lap time

beneﬁts due to the SLD occur primarily when exiting

corners.

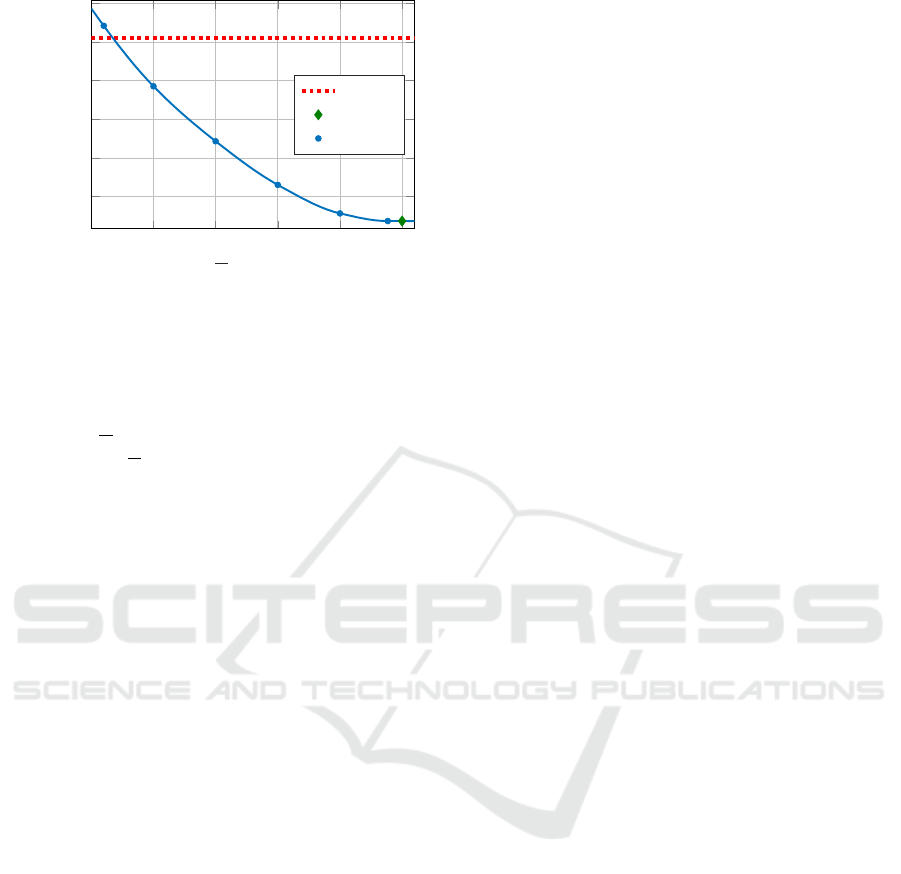
The lap time sensitivity regarding the maximum

torque of the SLD is depicted in ﬁgure 5. The sensi-

tivity curve is constructed via cubic spline interpola-

tion of computed sampling points which are generated

by solving the OCP for several values of T

. Gener-

ICINCO 2020 - 17th International Conference on Informatics in Control, Automation and Robotics

250 500 750

1000

1250

147

147.5

148

148.5

149

149.5

in Nm

in s

sample

Figure 5: Lap time over maximum torque of SLD.

ally, the increased vehicle weight and inertia due to

the SLD, implemented according to (Sedlacek et al.,

2020b), is detrimental for cornering. The beneﬁt of

lateral drive torque allocation outweighs this disad-

vantage for T

> 87.3Nm, whereas lap time reduces

with increasing T

. Since for the current track and ve-

hicle setup |T

| ≤ 1193Nm holds, lap time reductions

due to the SLD only occur up this threshold value.

However, this result strongly depends on the consid-

ered track and vehicle setup.

5 CONCLUDING REMARKS

A novel OCP-modelling approach for a broad class

of semi-active actuators has been presented. The pro-

cedure transforms the originally nonconvex set dic-

tated by the passivity constraint into multiple con-

vex sets. However, this convexiﬁcation requires an

extra input and generally a higher number of con-

straints. The method has been applied to compute

minimum-lap-time trajectories for a vehicle with rear-

wheel drive and SLD at the rear axle. The OCP

has been solved using Hermite-Simpson collocation

implemented in an open-source framework. Com-

pared to a vehicle with open differential instead of

the SLD, lap time is greatly reduced by 2.37 seconds

or 1.59% with lap time beneﬁts primarily occurring

when exiting corners. Although the overall OCP re-

mains nonconvex for the considered application, the

presented convexiﬁcation measure is a ﬁrst step to-

wards a fully convex OCP for such vehicles which

we will address in the future. Considering our pre-

vious work (Sedlacek et al., 2020b; Sedlacek et al.,

2020a), the proposed method will be used to com-

pare different powertrain topologies for vehicles with

combustion engine or electric machines while simul-

taneously identifying the respective optimal passive

vehicle setups. A detailed experimental comparison

of the proposed modelling-method with the existing

methods presented in section 3.2.4 is subject of future

work.

REFERENCES

Betts, J. T. (2010). Practical Methods for Optimal Control

and Estimation Using Nonlinear Programming. So-

ciety for Industrial and Applied Mathematics, second

edition.

Bianco, N. D., Bertolazzi, E., Biral, F., and Massaro, M.

(2019). Comparison of direct and indirect methods for

minimum lap time optimal control problems. Vehicle

System Dynamics, 57(5):665–696.

Boyd, S. and Vandenberghe, L. (2004). Convex Optimiza-

tion. Cambridge University Press, Cambridge, sev-

enth edition.

Cheli, F., Pedrinelli, M., Resta, F., Travaglio, G., Zanchetta,

M., and Zorzutti, A. (2006). Development of a new

control strategy for a semi-active differential for a

high-performance vehicle. Vehicle System Dynamics,

44(sup1):202–215.

Dunning, I., Huchette, J., and Lubin, M. (2017). Jump:

A modeling language for mathematical optimization.

SIAM Review, 59(2):295–320.

Gillespie, T. (1992). Fundamentals of Vehicle Dynamics.

SAE International.

Kelly, D. (2008). Lap time simulation with transient vehicle

and tyre dynamics. PhD thesis, Cranﬁeld University.

Kelly, M. (2017). An introduction to trajectory optimiza-

tion: How to do your own direct collocation. SIAM

Review, 59(4):849–904.

Kim, Y., Kwon, C., Moon, H., Kim, K., Cho, J., and Kong,

K. (2018). Optimization of semi-active pneumatic ac-

tuators for an exoskeleton robot for running. 2018

15th International Conference on Ubiquitous Robots

(UR), pages 119–124.

uger, W. (2000). Integrated design process for the de-

velopment of semi-active landing gears for transport

aircraft. PhD thesis, University of Stuttgart.

Limebeer, D., Perantoni, G., and Rao, A. (2014). Optimal

control of formula one car energy recovery systems.

International Journal of Control, 87(10):2065–2080.

Limebeer, D. J. N. and Perantoni, G. (2015). Optimal con-

trol of a formula one car on a three-dimensional track-

part 2: Optimal control. Journal of Dynamic Systems,

Measurement, and Control, 137(5).

Perantoni, G. and Limebeer, D. J. (2014). Optimal control

for a formula one car with variable parameters. Vehi-

cle System Dynamics, 52(5):653–678.

Poussot-Vassal, C., Savaresi, S. M., Spelta, C., Sename, O.,

and Dugard, L. (2010). A methodology for optimal

semi-active suspension systems performance evalua-

tion. In 49th IEEE Conference on Decision and Con-

trol (CDC), pages 2892–2897.

Savaresi, S. M., Poussot-Vassal, C., Spelta, C., Sename, O.,

and Dugard, L. (2010). Semi-Active Suspension Con-

trol Design for Vehicles. Elsevier, ﬁrst edition.

Convexiﬁcation of Semi-activity Constraints Applied to Minimum-time Optimal Control for Vehicles with Semi-active Limited-slip

Differential

Schramm, D., Hiller, M., and Bardini, R. (2014). Vehi-

cle Dynamics - Modeling and Simulation. Springer,

Berlin, Heidelberg.

Sedlacek, T., Odenthal, D., and Wollherr, D. (2020a).

Minimum-time optimal control for battery electric ve-

hicles with four wheel-independent drives considering

electrical overloading. Vehicle System Dynamics, Sub-

mitted for publication.

Sedlacek, T., Odenthal, D., and Wollherr, D. (2020b).

Minimum-time optimal control for vehicles with ac-

tive rear-axle steering, transfer case and variable pa-

rameters. Vehicle System Dynamics, pages 1–29.

Sharp, R., Casanova, D., and Symonds, P. (2000). A mathe-

matical model for driver steering control, with design,

tuning and performance results. Vehicle System Dy-

namics, 33(5):289–326.

Tremlett, A., Massaro, M., Purdy, D., Velenis, E., Assadian,

F., Moore, A., and Halley, M. (2015). Optimal control

of motorsport differentials. Vehicle System Dynamics,

53(12):1772–1794.

Tremlett, A. J. and Limebeer, D. J. N. (2016). Optimal tyre

usage for a formula one car. Vehicle System Dynamics,

54(10):1448–1473.

achter, A. and Biegler, L. T. (2006). On the implementa-

tion of an interior-point ﬁlter line-search algorithm for

large-scale nonlinear programming. Math. Program.,

106(1):25–57.

APPENDIX

Subsequently, further modelling details are addressed.

We assume windless conditions and consider the lon-

gitudinal drag force (Schramm et al., 2014)

air,x

= −

air

air,x

air

+ v

. (36)

The parameters are air density ρ

air

, drag coefﬁ-

cient c

air,x

and vehicle crossspan area A

air

. Due to

rolling resistance forces, the tires are subject to load-

dependent torques opposed to the rolling direction

roll,y,k

= − f

roll,0

z,k

(37)

with the rolling resistance coefﬁcient f

roll,0

For simplicity, quasi steady-state conditions are pre-

sumed for the wheel load computation. Assuming

that the roll momentum is allocated via the roll mo-

ment distribution factor ξ

roll

, the wheel loads are com-

puted via (Sedlacek et al., 2020b)







z,1

z,2

z,3

z,4







= ρ







g − h a

g + h a







+ ρ







−ξ

roll

−1

1−ξ

roll







(38a)

with ρ

2(l

+ l

)

and ρ

= m h a

. (38b)

The velocity of the wheel centre points in the

body coordinate frame is denoted with v



x,k

y,k



∀k ∈ K and computed by



−

ψl



, v



ψl



, (39a)



−

ψl



and v



−

ψl



. (39b)

These speeds are transformed into the corresponding

wheel coordinate frame via



x,k

y,k



= R

(δ

)



x,k

y,k



∀k ∈ K. (40)

Therein the two-dimensional rotation matrix

(γ)



cos(γ) sin(γ)

−sin(γ) cos(γ)



(41)

performs a mathematical rotation by the angle γ

around the z-axis of the inertial frame. Assuming

x,k

≥ 0 ∀k ∈ K, the longitudinal wheel slips are

deﬁned as

x,k

− v

x,k

max



x,k



. (42a)

The wheel slip angles are given by

y,k

= δ

− arctan

y,k

x,k

. (42b)

We employ the tire model presented in (Sedlacek

et al., 2020b) which is based on (Kelly, 2008). For

readability, we only list the basic equations for the

longitudinal tire forces and omit the index k. Equa-

tions for the lateral tire forces are constructed analo-

gously. The tire parameters differ in the longitudinal

and lateral direction as well as for the front and rear

wheels. The linear equations

max,x

b,x

−

a,x

−



−



a,x

(43a)

b,x

−

a,x

−



−



a,x

(43b)

approximate the load-dependent tire slip optimum

and the nonlinear wheel load degressivity, respec-

tively. The variables in (43a) and (43b) marked as

(·)

represent ﬁxed tire parameters. The normalised slips

and the combined slip coefﬁcient are deﬁned as

n,x

max,x

and λ =

n,x

+ λ

n,y

, (43c)

respectively. Using the tire shape parameter C

, the

tire force shape curve is characterised by

shape,x

n,x

sin



arctan(B

λ)



(43d)

with B

2 arctan(C

)

. (43e)

ICINCO 2020 - 17th International Conference on Informatics in Control, Automation and Robotics

With (38a), (43b), (43d) and the road friction coefﬁ-

cient µ, the longitudinal component of the tire force is

given by

= µ F

shape,x

. (43f)

The total tire force F





reaches its peak

values at the friction limit which can be represented

by the ellipse equation









= 1. (44a)

The positive optimum slip values are given by

tan







−

)

b,x

− (F

−

)

a,x



(

−

)

(44b)

With F

∀k ∈ K denoting the tire force vector in the

corresponding wheel coordinate frame, the tire force

components in the body reference frame are given by

= R

(−δ

∀k ∈ K. (45)

Parameter values for the considered OCP and the ve-

hicle model are listed in table 1 and 2, respectively.

Table 1: Parameter values for OCP.

variable value

optimisation

br,1/2

br,3/4

7024 Nm/4032 Nm

10.5 kNm/390.6 kW

0.6981 rad

0.9 ms

−1

/83.3 ms

−1

ω 277.8 rad/s

˙u

/ε

2 · 10

−2

/2 · 10

−4

˙s

1.0 ms

−1

λ,x

/ε

λ,tot

−6

/10

−8

Table 2: Parameter values for model.

variable value

env.

g 9.81 ms

−2

µ 1.0

air

1.2041 kgm

−3

tires

2000 N/6000 N

a,x,1/2

b,x,1/2

0.124/0.108

a,y,1/2

b,y,1/2

0.144/0.133

a,x,1/2

b,x,1/2

1.560/1.396

a,y,1/2

b,y,1/2

1.603/1.258

x,1/2

y,1/2

1.949/1.941

a,x,3/4

b,x,3/4

0.111/0.099

a,y,3/4

b,y,3/4

0.109/0.099

a,x,3/4

b,x,3/4

1.898/1.597

a,y,3/4

b,y,3/4

1.945/1.515

x,3/4

y,3/4

1.949/1.858

vehicle

1/2

3/4

0.3429 m/0.3474 m

veh

1.626 m/1.594 m/1.90 m

/h 1.479 m/1.503 m/0.540 m

m/J

1988 kg/3485 kgm

1/2

3/4

2.20 kgm

/6.95 kgm

air

2.44 m

air,x

/ f

roll,0

0.31/0.0031

acc

0.03 s

Convexiﬁcation of Semi-activity Constraints Applied to Minimum-time Optimal Control for Vehicles with Semi-active Limited-slip

Differential