Investigation of the Dynamic Loads on Tower Cranes During

Slewing Operations

Manuel St

olzner, Michael Kleeberger, Marcel Moll and Johannes Fottner

Chair of Materials Handling, Material Flow, Logistics, Technical University of Munich,

Boltzmannstr. 15, 85748 Garching, Germany

Keywords:

Tower Cranes, Static and Dynamic Analysis, Finite Element Method, Vibration Model, Dynamic Factors.

Abstract:

For decades, tower cranes have been the most important hoisting device used in building construction. In order

to avoid security risks at the construction site, it is essential to analyse the dynamic behaviour of these cranes

during the work process as the crane motions cause dynamic forces on the supporting structure. According

to the current standards, stress calculations for tower cranes are carried out based on static approaches. The

standards stipulate the use of special dynamic factors in the static calculation to estimate the dynamic loads.

This article analyses the dynamic behaviour of a tower crane during the important work process of slewing.

The results of the approach according to the standards are compared with the results of the nonlinear dynamic

ﬁnite element calculation. In addition, a newly developed vibration model based on a two-mass model will

be presented in this paper. The model helps to estimate the dynamic loads more accurately than the methods

currently used. However, the computation time required to perform the stress calculation is only slightly

increased.

1 INTRODUCTION

Tower cranes are very versatile and widely used. A

range of tower cranes is available extending from

small cranes, often used for the construction of de-

tached houses, to large tower cranes with special

climbing facilities for the construction of high-rise

buildings. Their ﬁeld of application currently in-

cludes a hoisting capacity of about 100 metric tonnes,

and a hoisting height of more than 100 metres. In

order to meet the different requirements on construc-

tion sites, most tower cranes are designed modularly,

which enables a large number of boom conﬁgurations.

The fundamental motions of tower cranes are

slewing, hoisting and they also allow either a lufﬁng

motion or load travel. During operation, crane mo-

tions cause dynamic forces on the hoist load and on

the crane’s structure. In order to guarantee the safety

of cranes, exact calculations of the boom system are

absolutely essential throughout the developing pro-

cess. Consequently, one main objective of the stress

calculation is to reproduce the system’s dynamic be-

haviour as realistically as possible.

According to the current international standard

ISO 8686 and the European standards EN 13001

and EN 14439, static approaches are used to perform

stress calculations for tower cranes. In order to con-

sider the dynamic effects during the working process,

rigid body approaches are used and special dynamic

factors are deﬁned. These factors are either based on

the experience of the crane manufacturers or alterna-

tively they are selected from tables in the standards.

In this publication, the approaches in the international

standard are evaluated by comparing the calculation

results with the results of other calculation methods.

Some scientiﬁc studies on tower cranes are al-

ready available in the literature. However, recent pub-

lications often deal with questions of control engi-

neering to reduce the effects of load swing, e.g. (Le

et al., 2013; Blajer and Kołodziejczyk, 2011; Doc¸i

et al., 2016). In almost all cases, models with rigid

or simple elastic bodies are used. Although these

simpliﬁed modelling approaches help to solve control

engineering problems, they are not adequate for in-

vestigating the dynamic effects on the structure in the

stress calculation.

Only a few publications exist that deal with the dy-

namic behaviour of tower cranes taking into account

elastic deformation, or that evaluate the approaches

in the standard. Some papers (Ju and Choo, 2005; Ju

et al., 2006) focus on frequency investigations for dif-

ferent applications. An earlier research project has al-

Stölzner, M., Kleeberger, M., Moll, M. and Fottner, J.

Investigation of the Dynamic Loads on Tower Cranes During Slewing Operations.

DOI: 10.5220/0009816300590067

In Proceedings of the 10th International Conference on Simulation and Modeling Methodologies, Technologies and Applications (SIMULTECH 2020), pages 59-67

ISBN: 978-989-758-444-2

ready investigated the dynamic loads of tower cranes

when hoisting grounded loads (Maier, 2000). For the

crane analysed, this research project showed that the

dynamic factor for hoisting, according to EN 13001,

describes the loads more accurately than the speciﬁca-

tions in the standard DIN 15018, which is now obso-

lete. However, this work provides no comparison with

the standards for crane movements involving major

displacement of the supporting structure, such as the

process of lufﬁng or slewing.

Since the standards for mobile cranes use similar

approaches for the design, the applicability of the dy-

namic factors has been evaluated in some publications

on mobile cranes, such as (Kleeberger et al., 2014;

olzner et al., 2018; St

olzner et al., 2019). These

articles have shown that the simpliﬁed approaches of

the calculation methods currently used are often inap-

propriate for reproducing the true dynamic effects on

mobile cranes. As it can be assumed that the calcula-

tion approaches of the standards have similar deﬁcits

for tower cranes as for mobile cranes, the dynamic

behaviour of tower cranes is analysed in more detail

below. Since the publications mentioned above show

that the greatest inaccuracies occur in the calculation

of slewing processes, this article focuses on the dy-

namic loads on tower cranes during slewing.

The nonlinear dynamic ﬁnite element method can

characterise the dynamic effects on cranes very accu-

rately. In this article, the results calculated using the

approach from the international standard ISO 8686

are evaluated by means of a comparison with the re-

sults of the nonlinear dynamic ﬁnite element calcula-

tion, as this method can be considered reliable.

Although the dynamic ﬁnite element method al-

lows exact and reliable calculations, it is very rarely

used by crane manufacturers. The main disadvantage

of this calculation method is the increased computing

effort compared to a static calculation. There is also

no reasonable way to consider the standards’ partial

safety factors in a dynamic calculation.

Alternatively, the standards permit the use of other

methods to calculate the dynamic factors. In order to

achieve a more accurate calculation of the dynamic

behaviour of tower cranes without a disproportionate

increase in computing time, a special vibration model

is presented in this publication. This new method al-

lows the dynamic factors for the slewing process to

be calculated in a more precise way than the current

methods. The applicability of the vibration model and

the calculation standard is shown for a tower crane

with different loads and load positions. In order to

assess the accuracy of the model and the standard,

several angular velocities and angular accelerations of

the tower crane are taken into account.

2 STATE of the ART

The international standard ISO 8686-1 (ISO 8686-1,

2012) deﬁnes general rules for the stress calcula-

tion of cranes. With regard to the load assumptions,

the general European standard for crane calculation

EN 13001-2 (EN 13001-2, 2014) is mostly identi-

cal to the international one. The design principles

for loads and load combinations of tower cranes are

speciﬁed in the product-speciﬁc international stan-

dard ISO 8686-3 (ISO 8686-3, 2018) and in the Eu-

ropean standard EN 14439 (EN 14439, 2010).

In the standards, the approach for considering dy-

namic loads is based on a rigid body kinetic analysis

and uses quasi-static calculation methods. In order to

take the effect of the dynamic forces into considera-

tion, the use of dynamic factors is suggested. How-

ever, the standard ISO 8686-3 also allows other val-

ues to be used for dynamic factors “when determined

by recognized theoretical analysis or practical tests”

(ISO 8686-3, 2018). The nonlinear dynamic ﬁnite el-

ement calculation and the vibration model presented

here (see Section 3) both belong to the accepted theo-

retical methods of analysis mentioned above.

To carry out the stress calculation of tower cranes,

the version of the European standard EN 14439 that

is currently valid refers to the now-obsolete stan-

dard DIN 15018 (DIN 15018, 1984) and the guide-

line FEM 1.001 (FEM 1.001, 1998). The interna-

tional standard provides ﬁxed values for the dynamic

factors, whereas the guideline FEM 1.001 deﬁnes an-

other method of describing dynamic loads during the

horizontal movements of tower cranes. This guide-

line proposes an approach based on a two-degree-of-

freedom model, which allows the factors to be calcu-

lated depending on the crane conﬁguration (see Sec-

tion 2.2).

In addition to the dynamic factors, both the inter-

national and the European standards prescribe the use

of partial safety factors in order to compensate for un-

certainties in the load assumptions. Since the aim of

this publication is not to evaluate safety factors but to

assess the quasi-static loads from the vibration model

and the calculation standards, no safety factors are

used. Nevertheless, the partial safety factors can be

considered in the same way as prescribed in the stan-

dards when this vibration model is used.

2.1 ISO 8686

The standard ISO 8686-3 “gives speciﬁc require-

ments and values for factors to be used at the struc-

tural calculation” (ISO 8686-3, 2018) of tower cranes.

The current draft of the product-speciﬁc European

SIMULTECH 2020 - 10th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

tower crane standard EN 14439 (prEN 14439, 2018)

corresponds to the approaches of this standard.

Based on ISO 8686-1, the loads are classiﬁed as

regular loads, occasional loads and exceptional loads.

The occasional loads include all forces caused by

skewing or loads due to climatic and environmental

inﬂuences such as loads from wind, snow or tem-

perature variation. Exceptional loads are test loads

and other loads that occur very rarely during regular

crane operations, for instance loads caused by emer-

gency off or loads due to unintentional loss of the

hoist load. Among regular loads are the forces caused

by “acceleration of all crane drives including hoist

drives” (ISO 8686-1, 2012). The dynamic loads re-

sulting from slewing operations fall into this category

of forces.

According to ISO 8686, the forces applied on

the structure during acceleration and deceleration are

calculated with rigid-body models. The calculation

includes both centrifugal forces and inertial forces.

Since a rigid-body analysis does not reﬂect the elastic

effects, the changes in the forces are multiplied by a

dynamic factor. To estimate the dynamic loads, the

dynamic factor Φ

is deﬁned and some default values

of this factor are proposed in the standard. To con-

sider the inertia forces, the general rules in the stan-

dard ISO 8686-1 provide a range between 1 and 2 for

this factor. The product-speciﬁc part ISO 8686-3 de-

ﬁnes a factor of Φ

= 1.5. The centrifugal forces are

considered by a factor of Φ

= 1.

In addition to the proof of strength, ISO 8686-3

also requires a proof of fatigue. As the aim of this

article is not to analyse fatigue, but rather to evalu-

ate and improve the underlying load assumptions, the

proof of fatigue is not considered.

2.2 FEM 1.001

For the proof of strength, the European standard

EN 14439 refers to the FEM guideline 1.001. This

guideline distinguishes between dynamic forces due

to vertical and horizontal movements of the crane. For

the horizontal movements, which include the slewing

process, the guideline deﬁnes different dynamic fac-

tors in the stress calculation related to the hoist load

and the supporting structure.

In contrast to ISO 8686, the dynamic factor for the

hoist load is calculated using a two-degree-of freedom

model of the crane (see Figure 1). In this model, m

the mass of all translatory and rotary moving parts of

the drive system and the supporting structure, reduced

to the load suspension point, while m

represents the

mass of the hoist load. The driving force F acts on m

The stiffness k

of the load pendulum is calculated

with the equation

· g

, (1)

where g is the acceleration due to gravity and l is the

length of the pendulum.

Deﬁning the coordinate z = y − x leads to the equa-

tions of motion

· (¨x + ¨z) = k

· z

· ¨x = k

· z − F.

(2)

Solving and evaluating these equations gives rise to a

dynamic factor by which to multiply the inertial force

on the hoist load.

Furthermore, the FEM guideline prescribes a value of

2 as the dynamic factor related to the supporting struc-

ture. To determine this parameter, the square root of

the square sum of the model’s two natural frequencies

and ω

are calculated ω

+ ω

and com-

pared with the time for deceleration.

Figure 1: Model according to FEM 1.001.

When applied to the elastic lattice structures of tower

cranes with modern drive systems, this simpliﬁed

model has two major disadvantages:

1. The elasticity of the supporting structure is not

considered.

2. The controlled drives currently used for tower

cranes follow the accelerations and decelerations

speciﬁed by the crane control very closely. Thus,

the movement of the mass m

is determined en-

tirely by the crane control and the two-mass model

is consequently reduced to a one-mass model of

the hoist load’s pendulum.

For these reasons, this simpliﬁed model cannot ac-

curately represent the dynamic loads on tower cranes

and the approach is consequently not adopted in the

current draft of EN 14439. Therefore, a modiﬁed

two-mass model is presented in the following section

in order to determine the dynamic loads during slew-

ing with an increased accuracy.

3 VIBRATION MODEL

Figure 2 shows the modiﬁed vibration model for

calculating the dynamic factors. In comparison to

Investigation of the Dynamic Loads on Tower Cranes During Slewing Operations

(t)

Figure 2: Modiﬁed vibration model for calculating dynamic

factors of tower cranes.

Figure 1, an additional spring with the stiffness k

added to describe the stiffness of the supporting struc-

ture. Furthermore, the displacement x

(t) replaces

the driving force F. When the load case slewing is

calculated, x

(t) is proportional to the time course of

the slewing angle of the crane slewing gear, which is

determined by the crane control. The dynamic be-

haviour of the supporting structure and the hoist load

can thus be expressed by the equations of motion

· ( ¨x

+ ¨x

) + k

· x

= −m

· ¨x

(t)

· ¨x

− k

· x

+ k

· x

= −m

· ¨x

(t)

(3)

with x

= y

− x

. The mass m

again repre-

sents the mass of the hoist load, the stiffness k

deﬁned by Eq. 1.

The mass m

and the stiffness k

are determined

by the results of a frequency analysis of the crane’s

ﬁnite element structure. Numerical investigations

about mobile cranes have shown that the vibrations

caused during the slewing process of cranes contain

only few natural frequencies (St

olzner et al., 2018).

During the process of slewing, tower cranes exhibit a

similar dynamic behaviour (see chapter 4.2). Conse-

quently, only the ﬁrst two lowest natural frequencies

corresponding to the modeshapes, which are orthog-

onal to the plane of the supporting structure, are de-

cisive in analysing the slewing process. ω

is usually

the lowest natural frequency of the crane structure at

which the tip of the boom and the hoist load swing

in phase, while ω

is the lowest natural frequency at

which the tip of the boom and the hoist load swing out

of phase. The characteristic equation

det



0 m



· λ



−k



= 0, (4)

with λ

= −ω

and λ

= −ω

is used to calculate the

values m

and k

. The mass m

and the stiffness k

are ﬁnally determined with the equations

= −

· m



− m

· ω





− m

· ω



(5)

= −

· m

· ω



− m

· ω





− m

· ω



. (6)

The differential equations (3) of the two-mass model

can easily be solved analytically. For that reason,

no numerical methods are required for calculating

the dynamic factor, and consequently the proposed

method only needs slightly more computing time than

the method proposed by the standard.

The dynamic factor related to the hoist load and the

supporting structure are calculated with the solutions

(t) and x

(t) of Eq. 3 for the associated working cy-

cle. The solution of the static displacement x

1,m

and

2,m

for a constant averaged acceleration a

of the

drive system results in the equations

1,m

= −

· a

(7)

2,m

= −

+ m

) · a

. (8)

The displacement solution x

(t) is proportional to the

lateral deﬂection of the boom. As the maximum lat-

eral deﬂection of the boom is again proportional to the

maximum bending moment in the boom, the largest

absolute value of x

(t) at the point in time t = t

max

is,

therefore, relevant for calculating the dynamic factor.

max

) is the corresponding value of the hoist load’s

lateral displacement. The new dynamic factors for the

static stress calculation result from the quotient of the

calculated dynamic displacements x

max

), x

max

)

and the static solutions x

1,m

and x

2,m

. The dynamic

factor applied on the supporting structure Φ

5,s

and the

factor for the hoist load Φ

5,p

are calculated with the

relations

5,s

max

)

2,m

(9)

5,p

max

)

1,m

. (10)

Both dynamic factors refer to the inertial forces act-

ing on the crane structure and the hoist load at the

constant mean accelerations a

of the slewing drive.

Based on the standard ISO 8686-3, the inertial forces

are still calculated with a rigid-body kinetic model

of the crane, and the dynamic behaviour is estimated

by applying the determined factors Φ

5,s

and Φ

5,p

on the inertia forces. To take the centrifugal forces

into account, the factor of Φ

= 1 recommended in

ISO 8686-3 is maintained.

The basic requirement of this vibration model is a

constant natural frequency during the slewing pro-

cess, which is satisﬁed for both bottom slewing and

top slewing tower cranes. In the case of the bot-

tom slewing tower cranes, the entire structure moves,

which means that the stiffness, and thus also the nat-

ural frequencies of the crane system, do not change.

SIMULTECH 2020 - 10th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

For top slewing tower cranes, the natural frequencies

also remain constant, since the tower consists of a

rectangular cross-section and the moment of inertia

is constant in all directions.

4 NUMERICAL ANALYSIS

According to ISO 4306-3 (ISO 4306-3, 2016), the

characteristics of tower cranes can be classiﬁed as

assembly, slewing level, type of boom and conﬁg-

uration. In the following calculations, a stationary,

top slewing crane with horizontal boom is analysed.

The subsequent sections present the crane with its

analysed parameters (see Section 4.1) and show the

numerical results using different calculation methods

(see Section 4.2).

4.1 Modelling

The calculation of the dynamic loads is based on a

crane with a maximum hoisting capacity of 12 metric

tonnes. In order to investigate the dynamic behaviour

of different crane conﬁgurations, the evaluation com-

prises different vertical and horizontal positions of the

hoist load. To analyse the inﬂuence of the hoist load’s

vertical position, four different hoisting rope lengths

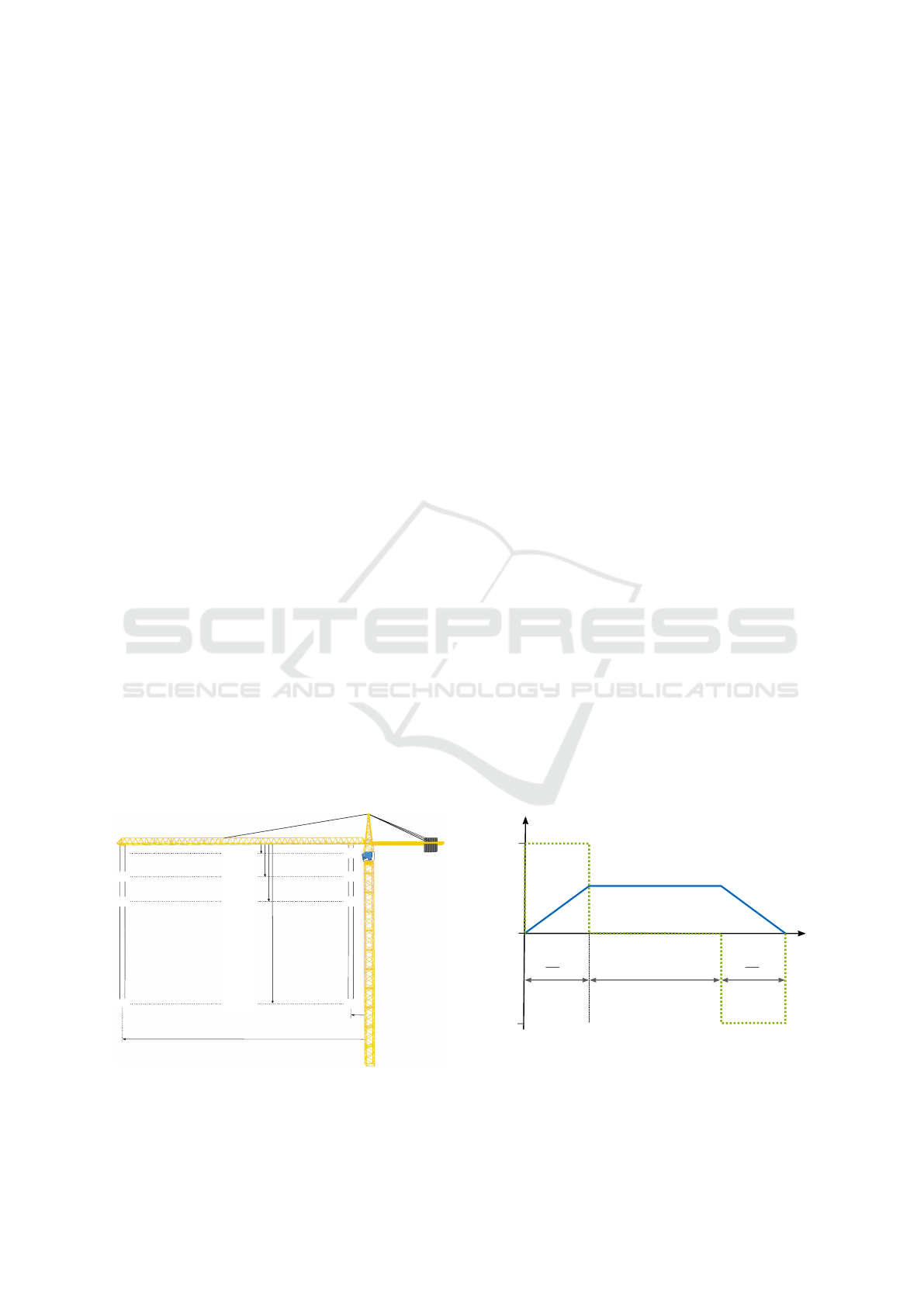
(0.7 m, 5 m, 10 m, 46 m) are selected (see Figure 3).

No damping is considered in the numerical calcula-

tions because the objective of the investigations is to

assess the accuracy of different approaches for the

stress calculations and not to analyse the inﬂuence of

damping on the supporting structure.

The ﬁnite element program NODYA, which was espe-

cially developed for the crane calculation, is used to

carry out the calculations. The original focus of this

0.7 m

5 m

10 m

46 m

5 m

75 m



Figure 3: Modelling of the tower crane and analysed posi-

tions of the hoist load.

software was the dynamic and static stress calculation

of mobile cranes, but it is also capable of calculat-

ing the lattice boom structures of tower cranes (Maier,

2000). It provides special features for the crane cal-

culation such as a cable element and a superelement.

The load cycle for analysing the dynamic effects

includes the phases acceleration, constant velocity

and deceleration. Linear time courses for the accel-

eration and velocity are assumed, choosing the same

time for acceleration and deceleration (see Figure 4).

The point in time t

max

of the maximum lateral

movement of the boom system is detected with a cho-

sen simulation time of 60 s. To identify the maxi-

mum stress, the calculations are carried out with 11

different time durations of constant velocity t

, rang-

ing from 0 s to 20 s. Crane manufacturers often only

consider one acceleration phase, as the crane operator

should commonly choose a convenient braking point

to achieve minimal vibration of the supporting struc-

ture and the load. However, in the modelled process

including the phase of deceleration, the inﬂuence of

the crane operator is disregarded as this represents the

more conservative load case.

As described in Section 2.1, the loads calculated

according to the international standard are mainly

dominated by the inertia forces and, therefore, depend

almost entirely on the acceleration of the supporting

structure. To verify this approach and to show possi-

ble dependencies of the dynamic loads on velocity or

acceleration, different combinations of angular speed

and angular acceleration are analysed in the calcula-

tions (see Table 1). As the FEM guideline recom-

mends an acceleration time between 5 s and 10 s, a

time close to this range is selected. In addition, it is

ensured that the drive torques do not exceed a value

of about 400 kN m.

Acce-

leration

Constant Velocity Dece-

leration

−a

a(t), v(t)

a(t)

v(t)

Figure 4: Analysed time course of the angular acceleration

and angular velocity of the slewing drive.

Investigation of the Dynamic Loads on Tower Cranes During Slewing Operations

Table 1: Analysed combinations of angular velocity and ac-

celerations.

ω in rad s

−1

ω in rad s

−2

ω/

ω in s

0.0105 0.0015 7

0.0105 0.0021 5

0.0105 0.0026 4

0.021 0.0021 10

0.021 0.003 7

0.0315 0.00263 12

0.042 0.0028 15

4.2 Results

The nonlinear dynamic ﬁnite element calculation is

the calculation method that can be considered the

most realistic for cranes. For this reason, the results

obtained from the calculation standard and the vi-

bration model are evaluated by means of comparison

with the results of the dynamic ﬁnite element calcula-

tion.

4.2.1 Dynamic Calculation and ISO 8686

Figure 5 shows an exemplary comparison between

the dynamic calculation and ISO 8686-3. The ﬁgure

shows the time courses of the lateral deformation of

the boom’s tip regarding 11 different times t

between

0 s and 20 s. The maximum values depend strongly

on the point in time at which breaking is initiated and

on the duration of the steady state time t

. However,

it is not possible to standardise the most crucial time

since the time courses change for different crane

conﬁgurations and different angular velocities or ac-

celerations. The results of the static calculation ac-

cording to ISO 8686-3 are shown for a positive and

a negative direction of slewing. In the crane conﬁgu-

ration considered, the results of the static calculation

are lower than the absolute maximum of the dynamic

calculation.

Figure 6 shows the results of the lateral deformation

of the crane’s boom tip for all load positions ¬-¯ and

Ê-Í of Figure 3. The diagram shows the results for

the combination of velocity and acceleration at which

the maximum lateral displacement of the boom tip oc-

curs. When the angular velocities and accelerations

resulting in the maximum lateral displacement of the

dynamic calculation are analysed, it is noticeable that

the maximum always occurs at the highest considered

acceleration of 0.003 rad s

−2

. This indicates that the

dynamic crane loads depend mainly on the angular

acceleration and not on the angular velocity of the

tower crane, which is analysed more precisely in Sec-

tion 4.2.2. For all velocities and accelerations consid-

ISO 8686-3

Displacement in m

Time in s

0 20 40 60

Figure 5: Time courses of the boom tip including dif-

ferent times t

, ω = 0.0105 rad s

−1

ω = 0.0026 rad s

−2

= 12 t, length of hoisting rope: 10 m, horizontal load

position: 5 m.

Dynamic calculation

ISO 8686-3

Displacement in m

Ê Ë Ì Í¬  ® ¯

Load positions according to Figure 3.

Figure 6: Maximum lateral displacement of all analysed

crane systems, calculated with the dynamic calculation and

according to ISO 8686-3. The maximum is always reached

with ω = 0.021 rad s

−1

and

ω = 0.003 rad s

−2

ered, the calculation according to ISO 8686-3 results

values that are too low for the dynamic loads. Fur-

thermore, it is apparent that the differences between

the calculation according to the standard and the dy-

namic ﬁnite element calculation occur for all investi-

gated positions of the hoist load.

When we study the dependency of the maximum

lateral displacement on the pendulum length, a de-

creasing crane load is obtained with increasing pen-

SIMULTECH 2020 - 10th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

dulum length. For this reason, the requirement of

the standard, which prescribes a load position “at

the top of the jib or immediately below the crab”

(ISO 8686-1, 2012) could be conﬁrmed by these nu-

merical tests.

4.2.2 Dynamic Calculation and Vibration Model

Figure 7 shows a Fourier transformation of the dy-

namic time course of the hoist load’s lateral displace-

ment. In the frequency domain it becomes clear that

the vibration is mainly dominated by two frequencies,

which correspond to the ﬁrst and the fourth natural

frequency of the crane. Since other frequencies in-

ﬂuence the vibration signal only very slightly, the vi-

bration can be simulated with the developed two mass

model.

Absolute value Fourier coefﬁcient in m

0.4

0.8

1.2

Frequency in Hz

0 0.05 0.1 0.2 0.3

Figure 7: Fourier transformation of the hoist load’s lat-

eral displacement, ω = 0.0105 rad s

−1

ω = 0.0026 rad s

−2

=10 s, m

= 2.5 t, length of hoisting rope: 46 m, horizon-

tal load position: 75 m.

Figure 8 shows the time course of the lateral displace-

ment of the hoist load’s suspension point for both the

dynamic calculation and the vibration model. The vi-

bration model reproduces the time course of the dy-

namic ﬁnite element calculation with high accuracy.

Both frequency and amplitude are reproduced almost

exactly as the higher natural frequencies make no no-

ticeable contribution to the vibration. For this rea-

son, the use of only two natural frequencies in the

vibration model shown leads to almost the exact re-

construction of the nonlinear dynamic ﬁnite element

calculation.

Dynamic calculation

Vibration model

Displacement in m

Time in s

0 20 40 60

Figure 8: Time course of the hoist load’s suspension point,

ω = 0.021 rad s

−1

ω = 0.003 rad s

−2

, t

= 14 s, m

= 2.5 t,

horizontal load position: 75 m.

Table 2 shows the dynamic factors related to the

hoist load and the supporting structure according to

the Eqs. 9 and 10. The dynamic factors vary consid-

erably, in part, when the different velocities and accel-

erations are used. In all cases, the dynamic factor re-

lated to the hoist load is higher than the factor for the

supporting structure, as an increased inertia force acts

on the hoist load due to the pendulum. Furthermore,

a dependency of the dynamic factor on the angular

acceleration of the crane can be derived, while the

dependency on the angular velocity is considerably

lower. In an earlier publication about mobile cranes, it

was already possible to determine dependency condi-

tions for velocity and acceleration based on the break-

ing time and the period of vibration (Kleeberger et al.,

2014). Since the selected angular acceleration is rel-

atively low in each case, the dependency on the an-

gular acceleration is more signiﬁcant in the presented

analyses. The analysis of special working conditions,

Table 2: Dynamic factors calculated with the vibration

model, m

= 2.5 t, length of hoisting rope: 5 m, load-lifting

radius: 75 m.

ω t

5,s

5,p

in rads

−1

in rads

−2

in s

0.0105 0.0015 0 1.999 2.017

0.0105 0.0021 2 1.889 1.935

0.0105 0.0026 18 1.752 1.83

0.021 0.0021 12 1.889 1.916

0.021 0.003 0 1.999 2.017

0.0315 0.00263 12 1.717 1.726

0.042 0.0028 18 1.526 1.578

Investigation of the Dynamic Loads on Tower Cranes During Slewing Operations

such as the load case emergency stop with its high ac-

celerations, would result in other dependencies. Over-

all, it can be seen that the recommended value of

=1.5, which is speciﬁed in ISO 8686-3, cannot lead

to satisfactory results in most cases. The value 1.5 is

only suitable for the longest acceleration time of 15 s.

In Figure 9, the maximum lateral displacements

of the boom’s tip resulting from the dynamic calcula-

tion are compared with the quasi-static calculations.

In order to investigate the quality of the load assump-

tions derived from the vibration model with those of

ISO 8686-3, the results of both methods are included

in the diagram. The quasi-static calculations using

the vibration model are based on the dynamic fac-

tors listed in Table 2. It is obvious that the dynamic

factors based on the vibration model allow a much

more accurate representation of the dynamic calcula-

tion than using the default factor of 1.5 which is spec-

iﬁed in the standard. The dynamic calculation can be

reproduced with high accuracy for all angular veloci-

ties and accelerations by using the calculated dynamic

factors. This clearly shows that the approach of the

quasi-static calculation leads to precise results when

appropriate dynamic factors are used.

Dynamic calculation

Vibration model

ISO 8686-3

Displacement in m

ω = 0.01050 rad s

−1

ω = 0.00150 rad s

−2

ω = 0.01050 rad s

−1

ω = 0.00210 rad s

−2

ω = 0.01050 rad s

−1

ω = 0.00260 rad s

−2

ω = 0.02100 rad s

−1

ω = 0.00210 rad s

−2

ω = 0.02100 rad s

−1

ω = 0.00300 rad s

−2

ω = 0.03150 rad s

−1

ω = 0.00263 rad s

−2

ω = 0.04200 rad s

−1

ω = 0.00280 rad s

−2

Figure 9: Maximum lateral displacement, m

= 2.5 t,

length of hoisting rope: 5 m, horizontal load position: 75 m.

5 CONCLUSION AND OUTLOOK

In this paper, the dynamic loads on tower cranes dur-

ing the slewing operation were analysed. The ac-

curacy of the standard ISO 8686-3 was evaluated by

comparing the results with the results of the nonlinear

dynamic ﬁnite element calculation. For the crane in-

vestigated in this publication, the dynamic loads were

usually underestimated with the default dynamic fac-

tor stipulated in the standard.

In order to describe the dynamic behaviour of the

tower crane during the slewing operation more ac-

curately, a newly developed vibration model, based

on a two-degree-of-freedom system, was presented in

this article. With the help of this model, the elas-

tic deformations of the supporting structure could be

considered in the stress calculation. It was possi-

ble to determine dynamic factors that allow a more

accurate description of the dynamic loads on tower

cranes than the commonly used factors from the stan-

dard. Concurrently, a similar computing time could

be achieved, since the vibration model is based on an

analytical solution of the two-degree-of-freedom sys-

tem. The comparisons included different angular ve-

locities and accelerations of the driving system and

different positions of the hoist load to determine the

inﬂuence of these parameters on the dynamic effects.

By analysing typical working cycles, the risks and

potentials of the current tower crane calculation dur-

ing the slewing process could be shown. The knowl-

edge of the dynamic loads on tower cranes can pro-

vide information about the quality of the standard’s

load assumptions.

To verify the statements on the dynamic properties

and the applicability of the vibration model, system-

atic comparisons including different types of cranes

should be carried out in the future. Furthermore, new

measurements of currently used tower cranes are nec-

essary to obtain further results regarding the accuracy

of the modelled crane and the different calculation

methods. An important question also arises from the

applicability of the vibration model to different work-

ing processes, such as hoisting or lufﬁng.

REFERENCES

Blajer, W. and Kołodziejczyk, K. (2011). Improved

DAE formulation for inverse dynamics simulation of

cranes. Multibody System Dynamics.

Doc¸i, I., Hamidi, B., and Lajqi, S. (2016). Dynamic analy-

sis and control of jib crane in case of jib lufﬁng motion

using modelling and simulations. International Fed-

eration of Automatic Control-PapersOnLine.

Ju, F. and Choo, Y. S. (2005). Dynamic Analysis of Tower

Cranes. Journal of Engineering Mechanics-ASCE.

Ju, F., Choo, Y. S., and Cui, F. S. (2006). Dynamic response

of tower crane induced by the pendulum motion of the

payload. International Journal of Solids and Struc-

tures.

SIMULTECH 2020 - 10th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

Kleeberger, M., Schneidler, S., and G

unthner, W. A. (2014).

Untersuchung der dynamischen Beanspruchungen bei

Gittermast-Fahrzeugkranen und Vergleich mit der

quasistatischen Auslegung der Norm. In Tagungs-

band 22. Kranfachtagung. Otto von Guericke Univer-

sity Magdeburg.

Le, T. A., Dang, V.-H., Ko, D. H., An, T. N., and Lee, S.-G.

(2013). Nonlinear controls of a rotating tower crane

in conjunction with trolley motion. Journal of Systems

and Control Engineering.

Maier, K. J. (2000). Untersuchung zur nichtlinearen

Berechnung dynamischer Belastungsvorg

ange an Tur-

mdrehkranen. PhD thesis, Technical University of

Munich.

DIN 15018 (1984). German Institute for Standardization:

Cranes - principles for steel structures - stress analysis.

EN 13001-2 (2014). European Committee for Standardiza-

tion: Crane Safety - General design - Part 2: Load

actions.

EN 14439 (2010). European Committee for Standardiza-

tion: Cranes - Safety - Tower cranes.

FEM 1.001 (1998). Federation Europeenne de la Manu-

tention (FEM): Rules for the design of hoisting ap-

pliances.

ISO 4306-3 (2016). International Organization for Stan-

dardization: Cranes - Vocabulary - Part 3: Tower

cranes.

ISO 8686-1 (2012). International Organization for Stan-

dardization: Cranes - Design principles for loads and

load combinations - Part 1: General.

ISO 8686-3 (2018). International Organization for Stan-

dardization: Cranes - Design principles for loads and

load combinations - Part 3: Tower cranes.

prEN 14439 (2018). European Committee for Standardiza-

tion: Cranes - Tower cranes (Draft).

olzner, M., Kleeberger, M., G

unthner, W. A., and Fottner,

J. (2018). Vibration model to describe the dynamic

behaviour of lattice boom mobile cranes and loader

cranes. In Logistics Journal: Proceedings. WGTL.

olzner, M., Kleeberger, M., G

unthner, W. A., and Fottner,

J. (2019). Calculating the dynamic behaviour of lattice

boom mobile cranes during hoisting with a vibration

model. In XXIII International Conference on Material

Handling, Constructions and Logistics - MHCL 2019.

Vienna University of Technology (Institute for Engi-

neering Design and Product Development) together

with University of Belgrade (Faculty of Mechanical

Engineering).

Investigation of the Dynamic Loads on Tower Cranes During Slewing Operations