
Congestion-Aware Stochastic Path Planning and Its Applications in Real
World Navigation
Kamilia Ahmadi and Vicki H. Allan
Computer Science Department, Utah State University, Logan, Utah, U.S.A.
Keywords:
Stochastic Path Planning, Multi-Agent Systems, Congestion-Aware Modelling, Non-Linear Objective, Route
Planning under Uncertainty.
Abstract:
In the realm of path planning, algorithms use edge weights in order to select the best path from an origin point
to a specific target. This research focuses on the case where the edge weights are not fixed. Depending on the
time of day/week, edge weights may change due to the congestion through the network. The best path is the
path with minimum expected cost. The interpretation of best path depends on the point of view of car drivers.
We model two different goals: 1) drivers who look for the path with the highest probability of reaching the
destination before the deadline and 2) the drivers who look for the best time slot to leave in order to have a
smallest travel time while they meet the deadline. Both of the goals are modelled based on the cost of the
path which is highly dependent on the level of congestion in the network. Minimizing the paths’ cost helps in
reducing traffic in the city, alleviates air pollution, and reduces fuel consumption. Findings show that using our
proposed intelligent path planning algorithm which satisfies users’ goals and picks the least congested path is
more cost efficient than picking the shortest-length path. Also, we show how agents’ goals and selection of
cost function impacts paths’ choice.
1 INTRODUCTION
Path planning finds a path from a specific origin to
a destination over a network of road segments. Path
planning algorithms use the road segment costs in or-
der to come up with the best path. If the road seg-
ments’ costs are fixed, planning the best path through
the network is a well understood task via the algo-
rithms like Dijkstra and A* algorithms (Dijkstra and
W., 1959; Hart et al., 1968). However, in real world
navigation problems, depending on the level of con-
gestion on the road segments, the cost associated with
the legs of the trip changes over time. Also, it is not
feasible to use an adaptive algorithm in every step
due to the urgency in having a quick response to the
queries and hesitancy of drivers to change their route
frequently.
In modeling a city scale graph, congestion
changes throughout the day which results in having
uncertain costs on the road segments (Nikolova and
Karger, 2008; Rus, 2020; Geisberger et al., 2010;
Yaoxin Wu et al., 2016). Congestion is highly af-
fected by the path selection of drivers in the network.
In addition, there are many factors that affect the
congestion pattern such as road conditions, drivers’
path choice, time of the day, weather conditions, and
events throughout the city (Rus, 2020; Geisberger
et al., 2010; Wilkie et al., 2011; Sigal et al., 1980; Pi
and Qian, 2017; Niknami and Samaranayake, 2016).
We consider expected travel time on the road seg-
ments as the cost of that segment. The variabil-
ity of congestion level on road segments makes it a
stochastic network. Minimizing the paths costs, ul-
timately results in reducing the city scale congestion
by picking less congested paths. Reducing conges-
tion throughout the city has the benefits of decreased
pollution, fewer accidents, less wasted time, and less
fuel costs (Chiabaut et al., 2009; Fan et al., 2005; Rus,
2020; Pi and Qian, 2017; Yaoxin Wu et al., 2016).
This paper focuses on path planning over a
stochastic network which is a graph of a city. The
challenge is to find the best paths under uncertainty
and the constraints of real world domain. Agents are
car drivers which can pursue different goals: first, the
ones who are not willing to take risk and look for the
path with highest probability of reaching destination
before a desired arrival time, even if it may take them
longer. Second, the agents who are open to take a
riskier decision if it helps them in having the small-
est en-route time. These agents are flexible in leaving
Ahmadi, K. and Allan, V.
Congestion-Aware Stochastic Path Planning and Its Applications in Real World Navigation.
DOI: 10.5220/0010267009470956
In Proceedings of the 13th International Conference on Agents and Artificial Intelligence (ICAART 2021) - Volume 2, pages 947-956
ISBN: 978-989-758-484-8
Copyright
c
2021 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved
947

anytime while they still need to make the trip.
To make it clearer, one good example of these kind
of agents’ goals is in the context of a package de-
livery system. For example, suppose that we guar-
antee the delivery of a package by 4 PM, otherwise
the customer doesn’t accept the delivery and we lose
the shipping costs. In that case, we are interested in
picking a path that has the highest chance of reach-
ing destination before the deadline to avoid losing the
shipping cost. The other possible case is delivering
perishable products. For example, if we promised the
delivery of perishable products before 6 PM to the
customers, we are interested to pick a path that has
the smallest en-route time due to the nature of our
package. In this case, we are flexible in leaving any-
time, but we do need to have the smallest en-route
path while still making the destination before 6 PM.
As mentioned earlier, the definition of best path
differs based on the goal of the agents. For finding the
best path, queries have an origin, a destination and the
desired arrival time (deadline) along with the agents’
goals. Our proposed path planning framework, mod-
els the city as a stochastic network, utilizes pruning
techniques to reduce the size of search space, defines
the path costs, aligns them with agents goals and picks
the minimum cost path.
2 LITERATURE REVIEW AND
CONTRIBUTION
Miller-Hooks and Mahmassani (Miller-Hooks and
Mahmassani, 2000) consider travel costs as edge
weights of a navigation graph in their model. Costs
depend on travel times, and their goal is to find the
least expected travel time in peak and non-peak time
of the day. Then they solve an equivalent determinis-
tic problem. The main concern with this framework is
there has been little work on considering uncertainty,
congestion awareness and time dependency of edge
weights in finding the optimal path.
Fan, Kalaba and Moore (Fan et al., 2005) consider
a special monotone increasing cost based on the prob-
ability of arriving late and suggests that the Gamma
distribution is natural for modelling stochastic edge
travel times. The probability calculation requires
computing a continuous-time convolution product.
Therefore, it makes the path planning a computation-
ally expensive and time consuming task.
Niknami et al (Niknami and Samaranayake,
2016), present a model to compute the route that max-
imizes the probability of on-time arrival in stochas-
tic networks. Their method uses a heuristic for the
optimal path that chooses the direction at every in-
tersection based on the current state by evaluating
zero-delay convolution on the path probability and ex-
pected travel time. However, they assume that travel
time distributions are exogenous (not impacted by in-
dividuals routing choices) which makes it not desir-
able as in realistic domain path choices are affected
by other drivers’ decisions as a major source of con-
gestion on stochastic networks.
Zhiguang (Cao, 2017), proposed the Probability
Tail model based on a cardinality minimization prob-
lem by directly utilizing travel time data on each
road link. Then, the minimization problem is ap-
proximately solved via relaxing the cardinality by
L1-norm, and formulating it as a mixed integer lin-
ear programming problem. For extracting the edge
weights, it uses travel time samples on each arc as in-
put and adopts some random distributions to generate
the weights. As the result, this model doesn’t consider
traffic patterns for different times of a day.
Rus et al. (Rus, 2020) proposes stochastic path
planning method where edge weights are represented
as linear combination of mean and variance of travel
time (mean+ λ * variance) controlled by a λ parame-
ter. In their model, the key property is that the optimal
path occurs among the extreme points of the convex
hull containing all the path points. Then λ is used
to prune the search regions and selects only a small
number of λ values. The best path is found by Di-
jkstra (Dijkstra and W., 1959) based on minimizing
the cost function of two modelled goals: a) probabil-
ity tail model and b) mean risk model. Since, Rus’s
model uses exhaustive enumeration for path selection,
it’s run time in average is O(n
2
log
4
(n)).
In our work, we propose a path planning model
which uses Rus’s work (Rus, 2020) as a conceptual
framework but provides practical improvements on
top of it. Firstly, we do not linearly combine mean
and variance of travel time on edge weights. We con-
sider travel costs in intervals of 10 minutes for each
day of a week and extract the typical mean and vari-
ance on that edge for the specific time slots. Means
and variances are as short as 10 minutes time seg-
ments to present the variation in any given point of
day/time accurately. Also, we study three options of
cost functions (linear, exponential, and step cost func-
tion) to have a better understanding of main classes
of cost modelling and their impact in path selection.
However, the model is general enough to include any
cost function. (details of cost functions are explained
in 3.5)
Our proposed path planning algorithm has two
main steps: a) pruning search region to select few can-
didate paths among all possible paths, and b) Planning
an optimal path from the candidate paths in step a. In
ICAART 2021 - 13th International Conference on Agents and Artificial Intelligence
948

pruning phase, a node is expanded if expected mean
of the travel time of the approximate path through the
node is less then the user’s deadline. Proposed prun-
ing algorithm utilizes graph clustering and approxi-
mation techniques (explained in detail in 3.4). Major-
ity of pruning work utilizes pre-computation, which
makes it very efficient and practical to real work do-
main. The second part of proposed path planning al-
gorithm focuses on picking the path which has the
minimum cost aligned with agents’ goals.
The last contribution of this paper focuses on
modelling agents’ goals. First group of agents are
looking for the path that maximizes the probability
of reaching a destination before the deadline (highest
probability path). Second group, look for the best de-
parture time slot in order to have the least travel time
and arrive at the destination before deadline, these
agents are interested to take riskier decision if it pro-
vides them shortest en-route time. Details of agents’
goal modelling is discussed in 3.6.
3 MODEL DESCRIPTION
3.1 Open Street Map Data
For building the city graph, we used Open Street Map
data (Haklay and Weber, 2008). Open Street Map is a
collaborative open source project which creates a free
editable map that can be used widely. Open Street
Map represents physical entities on the ground like
buildings, roads, intersections, bridges and so on. It
uses the basic data structure of entities and tags for
describing the characteristics of that entity. The data
structure includes nodes, ways, and relations. A node
is a single point in space defined by its latitude, lon-
gitude, and node id. A way is a list of nodes used to
represent linear features such as a series of roads. A
relation is a multi-purpose data structure that relates
two or more data elements like a route, turn restric-
tion, traffic signal or an area. We used map matching
techniques to match the OSM data to our logged traf-
fic data.
3.2 City Graph Edge Weights
The city is modelled as a directed graph consisting a
set of vertices, V , which represent road intersections
and edges, E, that represent road segments between
vertices. We consider the city graph to be planar (i.e.,
edges intersect only at their end points) (Rus, 2020;
Nikolova and Karger, 2008). Associated with each
edge of the graph is edge weight (W ) which is not
fixed, and it is represented by an expected travel time
random variable in terms of mean and variance of the
delay on that edge at the specific time shown in Equa-
tion 1.
W
edge
(t) = (m
edge
(t), v
edge
(t)) (1)
We compute time segments in the intervals of 10
minutes for each day of a week. For finding the mean
and variance of each edge in time segments of a week,
we summarized yearlong traffic data based on 10 min-
utes time segments for each day of a week. The tar-
get city in this model is Salt Lake City, Utah, and we
use monitored traffic data from Utah Department of
Transportation (UDOT) to extract edge weights of the
city graph.
Travel time of each edge is an independent Gaus-
sian random variable (Ahmadi and Allan, 2017; Rus,
2020; Nikolova and Karger, 2008; Long et al., 2006;
Wilkie et al., 2011; Sigal et al., 1980; Fan et al.,
2005). Since the sum of independent Gaussian ran-
dom variables is also a Gaussian random variable, the
travel time for the whole path is also Gaussian (shown
in Equation 2).
t
path
∼ Normal(m
path
, v
path
) (2)
We consider edge weights to be independent from
each other as the time dependent variance on edges
represents the dependency of the congestion on adja-
cent edges (Rus, 2020; Nikolova and Karger, 2008;
Wilkie et al., 2011; Sigal et al., 1980; Campbell et al.,
2011; LAU et al., 2012; Niknami and Samaranayake,
2016). For example, suppose that edge e takes 30 per-
cent longer than when congestion free in a specific
time slot, an adjoining edge is likely to take 30 percent
longer than when congestion free in the same time
slot. Then for the specific edge and its adjoining edge,
the variance reflects all of these changes throughout
different time slots of the day. It is also possible to
consider the stochastic dependency between edges by
transforming the graph in a way to add a new edge be-
tween two dependent edges with mean equals to 0 and
variance equals to covariance of the weights of two
dependent edges (Rus, 2020; Nikolova and Karger,
2008; Long et al., 2006; Fan et al., 2005). However,
since this makes the city graph even more intercon-
nected and complex, we model edge weights as in-
dependent while variance on edges represents the de-
pendencies.
The mean of a path is the sum of the means of all
edges included in the path considering sliding time
window (δ) (Equation 3).
m
path
(t) =
∑
e∈path
m
e
(t + δ) (3)
Congestion-Aware Stochastic Path Planning and Its Applications in Real World Navigation
949

Variance of the path is the sum of variance values
of all edges included in the path from an origin O to
destination D including sliding time window δ (Equa-
tion 4) (Rus, 2020; Nikolova and Karger, 2008; Chi-
abaut et al., 2009; Campbell et al., 2011; LAU et al.,
2012).
v
path
(t) =
∑
v∈path
v
e
(t + δ) (4)
If we consider each edge as an independent ran-
dom variable, then the sum of variances is derived
from (Equation 5). Since we assume edge weights
are independent from each other, then cov(X
i
, X
j
)=0
for i 6= j and Equation 6 is the result. Based on Equa-
tion 6, the variance of a path is the sum of variance of
all edges included in the path shown in Equation 4.
var(
n
∑
i=1
X
i
) = E([
n
∑
i=1
X
i
]
2
) −[E(
n
∑
i=1
X
i
)]
2
(5)
var(
n
∑
i=1
X
i
) =
n
∑
i=1
n
∑
j=1
cov(X
i
, X
j
) =
n
∑
i=1
cov(X
i
, X
j
)
=
n
∑
i=1
var(X
i
) (6)
For finding the mean and variance of a path, a slid-
ing time window has been considered. A sliding time
window implies that the cost of each edge in the path
depends on the amount of time that took to reach it,
not just the initial departure time. For example each
m
e
(t) is actually m
e
(t + δ) in which delta is the esti-
mated arrival time from source node to edge e.
3.3 Agents
We consider drivers as agents. Agents get suggested
directions from a central path planner by entering
source, destination, deadline and their goal. Defini-
tion of best path may be different from the point of
view of one agent to another. Having the origin (O),
target (T ), and deadline (D), here are the two main
questions that clarifies agents’ goals in this model.
• What is the path with the maximum probability
of reaching destination before the deadline? (the
most secure path, hence might be longer)
• What is the best time to leave in order to have the
smallest travel time and reach the target before the
deadline? (riskier decision, while getting smallest
travel time path)
3.4 Pruning Heuristic in Path Finding
In a city scale graph with interconnected nodes, there
are many possible paths between a source node (S) to
a destination node (D). Considering all of those paths
is computationally intractable and lots of them are not
aligned with the agent’s deadline and goal. Thus, we
need to prune the search region in order to consider
the paths with the closest characteristics to the de-
sired path. For finding the candidate paths between
a source (S) to a destination (D), we start from the
source node and expand the connected nodes until we
reach the destination. In expanding phase, we use a
heuristic that for each node considers an approximate
path from source to destination through that node, and
if the mean of that path is greater than the provided
deadline in query time, then the node is not expanded.
The path from source to destination through node
N is the combination of the path from source to the
node (P
SN
) and the approximate shortest-length path
from the node to destination (P
ND
). For each node
in expansion phase, P
SN
is known from the history
of previous expansion steps. For finding P
ND
, we
consider an approximate shortest-length path from
that node to destination as finding the actual shortest-
length path from N to destination is also computation-
ally intractable due to the large branching factor in
each step of the city scale graph.
Figure 1: P
SN
is the part of the path from source to node N
and it is retrieved from the history of previous expansion
steps. P
ND
is the approximate shortest-length path from
node N to destination. If the summation of mean of P
SN
and P
ND
is greater than the provided deadline, node N is
not getting expanded.
For approximating the P
ND
, we use city partition-
ing. Each partition includes a set of nodes and it is
represented by its exemplars. Exemplar of each par-
tition is one of the main nodes with highest traffic in
that partition. For city partitioning, We tried few com-
munity detection methods on the graph of Salt Lake
City (Infomap (Edler et al., 2017), Leading Eigenvec-
tor (Ruaridh Clark, 2018), Label propagation (Garza
and Schaeffer, 2019), and Multilevel (Yang et al.,
2016)) and among those Multilevel divides the city
to 157 communities and in average each community
includes 200 to 400 nodes in it. For the city like Salt
Lake City, this distribution of nodes and number of
partitions is reasonable. Figure 2 shows the distri-
bution of communities of multilevel approach on the
graph of Salt Lake City.
ICAART 2021 - 13th International Conference on Agents and Artificial Intelligence
950

Figure 2: Left: Distribution of communities in Multilevel
approach. Right: Visualization of communities on SLC
graph. The dots represent communities.
Approximate shortest-length path (P
ND
) is found
by using A* algorithm on exemplars, i.e. instead of
considering all the nodes from N to D, only exem-
plars are considered. In each step of A*, the next
exemplar is picked based on the smallest g(n) + h(n)
value, where g(n) is the shortest-length path from cur-
rent exemplar to the neighboring exemplar and h(n) is
the direct path from the neighboring exemplar to the
destination. Shortest-length paths between adjacent
exemplars are pre-computed and they are retrieved to
build the approximate shortest-length path.
Figure 3: Finding an approximate shortest-length path from
N to destination using A∗ algorithm through exemplars.
As it can be seen from Figure 2, Salt Lake City
has 157 partitions, therefore, pre-computing and stor-
ing the shortest-length paths between the adjacent ex-
emplars is not a complex task. Also pre-computation
of shortest-length path between exemplars is a one
time task as the shortest-length path between exem-
plars doesn’t change over time.
After finding the approximate shortest-length
path, mean of both P
SN
and P
ND
are found considering
the query time and if the summation of their means
is greater than the provided deadline, the node is not
expanded. This heuristic helps us to prune the path
finding search region and find the potential paths with
reasonable mean aligned with provided deadline.
As mentioned earlier, edge weights are repre-
sented based on mean and variance of the traffic flow
on that edge at the query time. Also, each path is the
finite sequence of edges. Therefore, paths from a spe-
cific source (C) to a destination (D) are presented as
nodes (m
p
,v
p
) in the mean-variance plane (Figure 4).
In the mean-variance plane, the horizontal axis rep-
resents the mean and the vertical axis represents the
variance. Each small rectangle represents one of the
candidate paths for a specific source, destination pair.
Figure 4: Paths from a specific origin (O) to a target (T) are
presented as nodes (m
p
,v
p
) in the mean-variance plane.
Paths are in a convex hull and the best path is
somewhere in the convex hull between the extreme
points. Paths may vary from the one with highest vari-
ance and lowest mean (marked as b) to a path with
highest mean and lowest variance (marked as a)in the
mean variance domain shown in Figure 4. Convex-
ity certifies that in the search region, there can be
only one optimal solution which is globally optimal
(Nikolova and Karger, 2008; Rus, 2020; Niknami and
Samaranayake, 2016). Then based on the cost func-
tion and agent’s goal, one of these paths is selected as
the best path which we explain in further sections.
3.5 Cost Function
Since there may be more than one candidate path be-
tween two nodes and the main objective is to find a
path with minimum expected cost, we need to have
a function which models each path’s expected cost
which is found using Equation 7. Modelling cost
function is extended from our previous work (Ahmadi
and Allan, 2017)
ExpectedCost(t) = cost(t) ∗ f
path
(t) (7)
For modelling paths’ cost Cost(t), we studied
three main classes of cost functions: a) linear, b) ex-
ponential, and c) step cost functions and we discuss
the characteristics of each one in the subsequent sec-
tions. Obviously, modelling paths’ cost is not lim-
ited to the cost functions we discuss here and the
model is general enough to handle any cost function
of choice. Either by combining linear, exponential,
and step function or by directly putting Cost(t) in
Equation 7.
Since the main cost on paths is travel time and
travel time on edges is a continuous random vari-
able which follows a normal distribution (Nikolova
and Karger, 2008; Rus, 2020; Fan et al., 2005; Sigal
et al., 1980), Probability Density Function (PDF) is
used (Bachman et al., 2000) to define the probability
of travel time random variable at each specific time
(described in Equation 8). The parameter m
path
, v
path
are calculated based on Equation 3 and Equation 4.
f
path
(t|m
path
, v
path
) =
1
p
2πv
path
e
−
(t−m
path
)
2
2v
path
(8)
Congestion-Aware Stochastic Path Planning and Its Applications in Real World Navigation
951

3.5.1 Linear Cost Function
In the linear cost function model, the cost of the path
increases linearly by travel time (Equation 9). The
longer the travel time (t), the more expensive the path
is. The expected cost is calculated using Equation 10.
cost(t) = t (9)
ExpectedCost(t) =
Z
+∞
−∞
t f
path
(t)dt = m
path
(10)
Therefore, if we model cost as linear, expected cost
of the paths are equal to average travel time of those
paths. In that case, neither deadline and nor agent’s
goal plays a role here. It even removes the effect of
variance of travel time of paths.
3.5.2 Exponential Cost Function
Exponential cost function refers to the case where the
cost of a path increases rapidly by travel time. Equa-
tion 11 shows the exponential cost model based on
travel time (t), and Equation 12 is used for calculating
the expected cost. In Equation 11, k is the steepness
of the exponential cost increase.
cost(t) = e
k∗t
(11)
ExpectedCost(t) =
Z
+∞
−∞
e
k∗t
f
path
(t)dt = e
k(m
path
+
v
path
2
)
(12)
Based on the Equation 12, minimizing the ex-
pected cost of the exponential cost function depends
on minimizing the linear combination of mean and
variance in accordance with cost steepness parameter
k.
Even though modelling cost as exponential con-
siders the effect of variance in path planning, hence
it always picks the path with minimum m
path
, v
path
at
query time and other parameters such as deadline and
agents’ goals are not in the picture of decision mak-
ing.
3.5.3 Step Cost Function
Another way of modeling the cost function is to pe-
nalize the paths which reach the destination after the
deadline. In this case, a step function is used to model
the cost (Equation 13 and Equation 14 ). In Equa-
tion 13, u represents a step function (Bachman et al.,
2000), d stands for the desired arrival time, and t is
travel time random variable. Then expected cost is
found using Equation 15.
cost(t, d) = u(t −d) (13)
u(t −d) =
1 i f t > d
0 i f t < d
(14)
ExpectedCost(t) =
=
Z
+∞
−∞
u(t −d) f
path
(t)dt (15)
Since the step function does not consider any
penalty if the agent reaches the destination before
deadline, the cost in the interval of [−∞, d] is zero and
Equation 15 is re-written as Equation 16. Equation 16
is equal to Cumulative Density Function (CDF) of
Standard Normal Distribution (Bachman et al., 2000).
CDF generates a probability of the random variable
(travel time in this case) when distribution is normal
to be less than a specific value which is d (deadline)
here. Based on Equation 16, when there is a set of
paths from a specific origin to a destination, the path
with minimum expected cost is the path that maxi-
mizes Equation 17.
ExpectedCost(t) =
Z
+∞
d
f
path
(t)dt = 1 −Φ(
d −m
p
√
v
p
)
(16)
Φ(path) =
deadline −m
path
√
v
path
(17)
In the step cost model, in order to select the best
path, we need to consider deadline, agents’ goals and
query time in the objective function as shown in Equa-
tion 17.
3.6 Modelling Agents’ Goals
As mentioned in 3.3, two agents’ goals have been con-
sidered in this work and per the discussion in 3.5,
if we model paths’ cost as linear and exponential,
agents’ goals are not considered in expected cost min-
imization. Therefore, we focus on step cost function
as one of the possible cost functions which considers
the agents’ goals.
3.6.1 Highest Probability Path
If we model the cost as step function the expected cost
can be found by Equation 16. Then, in order to min-
imize the expected cost we need to maximize Equa-
tion 17 which is the path with highest probability of
reaching the destination before deadline. For find-
ing the best path, we need to consider the set of all
candidates paths from origin (O) to destination (D) in
the mean-variance domain based on the approach ex-
plained in 3.4 in order to select the path which maxi-
mizes Equation 17.
ICAART 2021 - 13th International Conference on Agents and Artificial Intelligence
952

3.6.2 Shortest En-route Time
For finding the shortest en-route time, cost function
is modelled as the step cost. Then having the desired
arrival time and the probability of making the trip be-
fore that, we are looking for the specific time t
G
for
departure which results in the shortest en-route time.
Therefore, the departure time is not fixed and it is a
specific time t
G
bounded in the interval of query time
τ1 and desired arrival time τ2. For simplicity of refer-
ral, we call the [τ1, τ2] interval as the trip interval.
For this model, we modify the deadline variable in
Equation 17 as the difference of desired arrival time
and departure time. The goal is to find the depar-
ture time t
G
in a way that the travel duration is mini-
mized. In Equation 18, φ is the argument of the Cu-
mulative Distribution Function (CDF) that makes the
CDF equal to the given probability of making the trip
before τ2 and it is fixed here.
desired arrival time −departure time =
m
path
+ Φ(path) ∗
√
v
path
i f departure time ⊂[τ1, τ2] (18)
Here is the summary of the steps need to be done
for this scenario.
• Find the latest time (τ
L
) that if agent departs it can
still reaches destination before deadline.
• Divide trip interval [τ1, τ
L
] to sub-intervals of
[t
1
,t
2
,t
3
,t
4
,t
5
, . . . , t
n
] in accordance with time seg-
ment definition (10 minutes each).
• For each of the sub-intervals k which is [t
(k−1)
,t
k
]:
− Find the paths from an origin (O) to destina-
tion (D) in a case that if they start their trip in
[t
(k−1)
,t
k
], they can make the trip before dead-
line.
− From the set of paths found in last step, select
the one which minimizes the expected cost of
the objective function described in Equation 18.
• Now for each time segment k we have one path
which is the best for that time segment. Then, se-
lect the interval which has the path with minimum
travel time.
4 EXPERIMENTS AND RESULTS
4.1 Path Planning based on Agents’
Goals
In this experiment, we study how agents’ goals in path
planning affect the paths selection in different times
of the day. For this reason, we pick some source, des-
tination pairs with the distance in the range of seven
to ten miles.
4.1.1 Highest Probability Path
Given the deadline (set as the estimated travel time
for the two selected nodes in this experiment) and the
goals of selecting the path with highest probability of
reaching destination, we aim to study the path selec-
tion for each of the introduced cost function (linear,
exponential and step cost function).
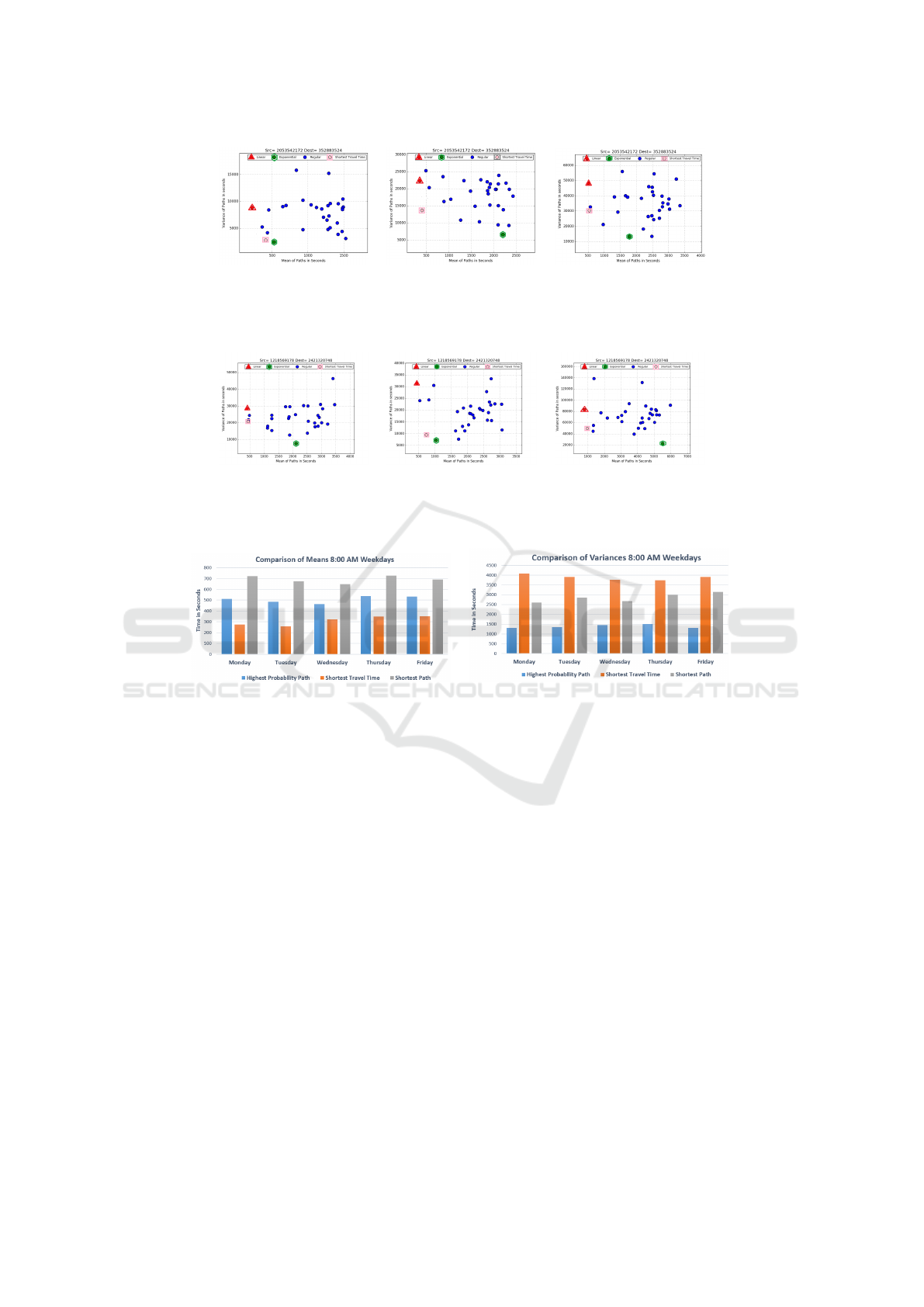
Figure 5 and Figure 6 show the results of this ex-
periment for three different time slots of Friday and
Tuesday for two sets of randomly picked nodes. Each
circle represents one of the possible paths between the
source and destination. Considering the set of paths
between a specific source and destination, a red tri-
angle identifies the path that satisfies the linear cost
function criteria. A green trapezoid is the best path
based on the exponential cost model and a black rect-
angle identifies the path with step cost function.
The results in Figure 5 and Figure 6 show that the
characteristics of paths for the same source and desti-
nation nodes changes in different times of the day de-
pending on the impact of travel level throughout the
city.
A pattern in both Figure 5 and Figure 6 shows that
when we query for best paths in rush hours, the differ-
ence between linear, exponential and step cost func-
tion which models highest probability path is large.
However, in non-rush hour times, there is not a signif-
icant difference between them. This means that hav-
ing a realistic cost function model along with consid-
ering the deadline helps in finding better paths in rush
hour. In non-peak times, since traffic is low, paths are
similar to each other and navigation might not be that
crucial. Having a good cost function modeling and a
wise criteria of picking the best path is crucial when
paths are congested.
Another interesting point is the way different
models pick the best path. Linear model (red trian-
gle) focuses on picking the path with minimum mean,
while in some cases like Figure 5.(a) the path might
have a high variance. Exponential model (green trape-
zoid) considers the mean and variance but it does not
consider the deadline. Therefore, in Figure 5.(a), Fig-
ure 6.(a), and Figure 6.(c) the paths selected by ex-
ponential model all violate the deadline. Step cost
function which demonstrates the highest probability
path, pick the least risky path which makes the dead-
line without a high variance which is the desired out-
come in this experiment.
Congestion-Aware Stochastic Path Planning and Its Applications in Real World Navigation
953

(a) (b) (c)
Figure 5: Selected paths for times of Friday for Source node=83590367 and Destination node=352876209 for each cost
function. Query times from left to right the times are: a) 8:00, b) 15:00, and c) 18:10 PM. Deadline is set as 1200 seconds.
(a) (b) (c)
Figure 6: Selected paths for different times of Tuesday for Source=358207657 and Destination=384734324 for each cost
function. Query times from left to right are: a) 7:30, b) 11:40, and d) 17:45. Deadline is set as 1400 seconds.
4.1.2 Shortest En-route Time
In this experiment, the agent wants to have the short-
est en-route time within a desired arrival interval. We
determine the best time to start the trip and which
path yields the shortest travel time. Figure 7 and Fig-
ure 8, show the results of this experiment for three dif-
ferent time slots of Monday and Wednesday for two
different sets of nodes with all three mentioned cost
functions. Each circle represents one of the paths be-
tween the source and destination. In each figure, pink
rectangle represents the path with smallest travel time
with the step cost function, green trapezoid is the best
path if the cost function is exponential, and red tri-
angle is the best path based on linear cost function
model.
Similar to the findings in the previous experiment,
Figure 7 and Figure 8 shows that in rush hours se-
lected paths for different models differ from each
other significantly, while in non-peak hours they are
almost the same. It emphasizes the effect of conges-
tion in busy hours and how it changes the weights on
edges of the graphs and impacts the path selection.
As in the previous experiment, the linear model picks
the path with smallest mean, while that path might
have a high variance like Figure 7.(b). Exponential
path does not consider deadline and it may pick a path
which does not make the trip within the desired ar-
rival. Step cost function which provides the shortest
en-route time path considers mean, variance, and de-
sired arrival time. Desired probability for this experi-
ment is considered as 85 percent. This means that we
are interested to find the paths that have the smallest
travel time and within the chance of 85 percent can
make the trip before deadline (85 percent is a number
we picked to keep the experiments consistent here, it
can be any probability).
Another finding indicates that picking the shortest
en-route path sometimes is a risky decision as it has a
high variance of reaching destination before deadline.
For example, in Figure 7 and Figure 8 the smallest
travel time path has the higher variance in comparison
to the path with exponential cost function.
4.2 How Do Selected Paths Compare
with Shortest-length Path?
In the realm of path planning, shortest-length path
is always a practical option. Hence we used it as a
baseline to see how our paths are different from the
shortest-length path. In this experiment, we com-
pare means and variances of shortest-length path with
highest probability path and shortest en-route time
path for 100 random pairs of source and destinations
with the distance of 10 to 12 miles in different areas
of Salt Lake City during morning rush hours. The re-
sults for each of the goals are averaged over all 100
pairs in weekdays (Monday through Friday). Desired
travel time for the pairs is considered as 2200 seconds
(based on the average time takes to get from a source
to destination with the distance for 10 to 12 miles in
rush hour) and desired probability for this experiment
is considered as 85 percent. Figure 9 is the demonstra-
tion of means and variances for each day of the week.
As it can be seen from the graphs, shortest-length path
is not performing well as it just tries to pick the mini-
ICAART 2021 - 13th International Conference on Agents and Artificial Intelligence
954
(a) (b) (c)
Figure 7: Selected paths for different times of Monday for Source=2053542172 and Destination=352883524. Times from left
to right are: a) 6:40, b) 8:10, and d) 18:00. Desired arrival time is within the 1600 seconds after query time. Best time for
start the trip to have the smallest travel time is as follow: a) 6:40, b) 8:23, and c) 18:21.
(a) (b) (c)
Figure 8: Selected paths for different times of Wednesday for Source=1218569178 and Destination=2421320748. Times from
left to right are: a) 7:40, b) 11:10, and d) 17:30. Desired arrival time is within the 2000 seconds after query time. Best time
for start the trip to have the smallest travel time is as follow: a) 8:01, b) 11:10, and c) 18:09.
(a) (b)
Figure 9: Comparison of average mean and variance of highest probability path, shortest en-route path and shortest-length
path in 8:00 AM of Weekdays for 100 different source and destinations.
mized length path even if it is congested. Shortest en-
route time paths have smaller means in comparison
to highest probability paths while they have higher
variance which makes highest probability paths more
secure options but longer travel time. Even though
shortest-length paths have reasonable variance, their
high mean value makes them not a good option to
pick.
5 CONCLUSION AND FUTURE
WORK
This research represents path planning in the real
world domain in which edge weights are not fixed
but are stochastically affected by the time of the
day/week. Inspired by time-dependent traffic situ-
ation, we parameterize these distributions by time,
which allows us to speak of time-dependent path costs
and study the problems of reaching a goal by a dead-
line, and delaying departure to minimise traversal-to-
goal time. The best path is the path with lowest cost
and the cost is based on travel time which highly de-
pends on the level of congestion in different time of
the day/week. Since the graph is interconnected, op-
timizing for lowest cost on all possible paths is not
feasible, therefore, we propose a pruning technique to
shrink the search region. Agents can pursue two main
goals: 1) picking the least risky path and 2) picking
the smallest travel time along with awareness of when
to start the trip.
Results show that during rush hour, utilizing an
intelligent path planning approach is crucial. In ad-
dition, we demonstrate that a suitable path planning
approach must consider path’s mean, path’s variance,
agents’ goals and the deadline to provide optimal op-
tions. We also compared the mean and variance of
highest probability paths and shortest en-route paths
with shortest-length paths at the same query time.
This experiment proves that during rush hour shortest-
Congestion-Aware Stochastic Path Planning and Its Applications in Real World Navigation
955

length path is not a good option. Another finding in-
dicates that, highest probability path has less variance
as it takes the most secure path, while the smallest
travel time has the lowest mean and might have a high
variance.
Possible future work is to consider salable ap-
proaches that enables this framework to handle large
number of path planning queries at the same time.
Also, we can expand the model to offer paths that are
optimal for alleviating the overall congestion of the
city rather than just the best path for each agent (opti-
mal decisions versus selfish decisions).
REFERENCES
Ahmadi, K. and Allan, V. H. (2017). Stochastic Path Find-
ing under Congestion. In 2017 International Confer-
ence on Computational Science and Computational
Intelligence (CSCI), pages 135–140.
Bachman, G., Narici, L., and Beckenstein, E. (2000).
Fourier and Wavelet Analysis. Universitext. Springer
New York, New York, NY.
Campbell, A. M., Gendreau, M., and Thomas, B. W.
(2011). The orienteering problem with stochastic
travel and service times. Annals of Operations Re-
search, 186(1):61–81.
Cao, Z. (2017). Maximizing the probability of arriving on
time : a stochastic shortest path problem.
Chiabaut, N., Buisson, C., and Leclercq, L. (2009). Fun-
damental Diagram Estimation Through Passing Rate
Measurements in Congestion. IEEE Transactions on
Intelligent Transportation Systems, 10(2):355–359.
Dijkstra, E. W. and W., E. (1959). A note on two problems
in connexion with graphs. Numerische Mathematik,
1(1):269–271.
Edler, D., Guedes, T., Zizka, A., Rosvall, M., and Antonelli,
A. (2017). Infomap Bioregions: Interactive Mapping
of Biogeographical Regions from Species Distribu-
tions. Systematic Biology, 66(2):197–204.
Fan, Y. Y., Kalaba, R. E., and Moore, J. E. (2005). Arriving
on Time. Journal of Optimization Theory and Appli-
cations, 127(3):497–513.
Garza, S. E. and Schaeffer, S. E. (2019). Community detec-
tion with the Label Propagation Algorithm: A survey.
Physica A: Statistical Mechanics and its Applications,
534:122058.
Geisberger, R., Kobitzsch, M., and Sanders, P. (2010).
Route planning with flexible objective functions.
Haklay, M. and Weber, P. (2008). OpenStreetMap: User-
Generated Street Maps. IEEE Pervasive Computing,
7(4):12–18.
Hart, P. E., Nilsson, N. J., and Raphael, B. (1968). A for-
mal basis for the heuristic determination of minimum
cost paths. IEEE Transactions on Systems Science and
Cybernetics, 4(2):100–107.
Lau, H. C., Yeoh, W., Varakantham, P., and Nguyen, D. T.
(2012). Dynamic Stochastic Orienteering Problems
for Risk-Aware Applications. Uncertainty in Artificial
Intelligence: Proceedings of the Twenty-Eighth Con-
ference: August 15-17 2012, Catalina Island, United
States.
Long, D., ICAPS 2006 (16 2006.06.06-10 Windermere, U.,
and International Conference on Automated Planning
and Scheduling (16 2006.06.06-10 Windermere, U.
(2006). Optimal route planning under uncertainty.
AAAI Press.
Miller-Hooks, E. D. and Mahmassani, H. S. (2000). Least
Expected Time Paths in Stochastic, Time-Varying
Transportation Networks. Transportation Science,
34(2):198–215.
Niknami, M. and Samaranayake, S. (2016). Tractable
Pathfinding for the Stochastic On-Time Arrival Prob-
lem. pages 231–245. Springer, Cham.
Nikolova, E. and Karger, D. R. (2008). Route Planning un-
der Uncertainty: The Canadian Traveller Problem.
Pi, X. and Qian, Z. S. (2017). A stochastic optimal control
approach for real-time traffic routing considering de-
mand uncertainties and travelers’ choice heterogene-
ity. Transportation Research Part B: Methodological,
104(Supplement C):710 – 732.
Ruaridh Clark, M. M. (2018). Eigenvector-based commu-
nity detection for identifying information hubs in neu-
ronal networks | bioRxiv.
Rus, S. L. B. K. G. R. M. (2020). Method and apparatus for
traffic-aware stochastic routing and navigation.
Sigal, C. E., Pritsker, A. A. B., and Solberg, J. J. (1980).
The Stochastic Shortest Route Problem. Operations
Research, 28:1122–1129.
Wilkie, D., Van Den Berg, J., Lin, M., and Manocha, D.
(2011). Self-Aware Traffic Route Planning.
Yang, Z., Algesheimer, R., and Tessone, C. (2016). A
Comparative Analysis of Community Detection Algo-
rithms on Artificial Networks. Scientific Reports, 6.
Yaoxin Wu, Wei Chen, Xuexi Zhang, and Guangjun Liao
(2016). Improving the performance of arrival on time
in stochastic shortest path problem. In 2016 IEEE
19th International Conference on Intelligent Trans-
portation Systems (ITSC), pages 2346–2353. IEEE.
ICAART 2021 - 13th International Conference on Agents and Artificial Intelligence
956
