Boundary Burr Phenomenon for Long-path Time Domain OCT

Tatsuo Shiina

Graduate School of Engineering, Chiba University, 1-33 Yayoi-cho, Inage-ku, Chiba-shi, Chiba 263-8522, Japan

Keywords: OCT, Long Path, Boundary, Diffraction.

Abstract: Long-path OCT measurement system has been developed for industrial use. We aim to measure time change

of the concentration and refractive index of the target solution with a certain volume. As the fundamental

experiment, we put the glass plate in the solution to obtain the glass position and its thickness, and got the

interesting result. That is, the vibration mitigating waveform like a diffraction pattern by a knife-edge was

observed. The OCT measurement consists of inline signals from the back-reflected (scattered) light, the

diffraction pattern by a knife-edge is appeared on a screen. Although this phenomenon causes the boundary

burr on the target detection, while the diffraction pattern has the information of the refractive index difference

of the target. In this study, the characteristics of the boundary burr phenomenon is examined. Its origin is

considered experimentally and analytically.

1 INTRODUCTION

The optical coherence tomography: OCT technology

is the low coherent interferometer and it obtains the

cross-sectional image by non-invasive and non-

destructive measurement. It is invented by Tanno and

Fujimoto (Tanno N., 1990 and Huang D., 1991)

Mainly it is developed for ophthalmology (Danielson

1991, Brezinski, 1999) After that, it is adapted to the

industrial use.(Song 2012) The combination of super

luminescent diode: SLD and optical fiber

interferometer adds the flexibility of measurement to

the device and also compactness.

In this study, a portable OCT scanner has been

developed for industrial use.(Shiina T., 2003, 2009,

2014, Yoshizawa T., 2015, Saeki K., 2020) The long

path TD-OCT was aimed to measure the refractive

index difference due to the solution condition such as

temperature and density change or convective flow.

The system has a measurement range of up to 100mm

with 5-digit accuracy. And the target is not a drop of

liquid, but a certain volume of the solution to catch

the partial change in a volume and its temporal

change. Long optical path measurement leads the

sensitive detection to enlarge the small difference.

Recently, we conducted the fundamental

experiment to evaluate the refractive index change

due to the solution temperature (Shiina T. 2019) and

https://orcid.org/0000-0001-9292-4523

the aqueous solution of the ethanol was examined too

with the unique characteristics of its refractive index

variation. To expand the measurement to the

concentration change and erratic distribution of

solution, as an initial approach, a glass plate was

inserted into a pure water to obtain the glass position

and its thickness. There, the interesting result was

observed, that is, the vibration mitigating waveform

like a diffraction pattern by a knife-edge was

observed. The OCT measurement consists of inline

signals from the back-reflected (scattered) light,

while the diffraction pattern by a knife-edge is

appeared on a screen. This phenomenon causes the

boundary burr on the target detection, while the

diffraction pattern has the information of the

refractive index difference of the target. We shift the

experiment to more simple arrangement to examine

this unique phenomenon. With the actual knife-edge

diffraction conditions, we discussed the boundary

burr phenomenon of the OCT signal. The numerical

analysis approach was started, too.

In this report, the characteristics of the boundary

burr phenomenon is examined and analyzed. Its

origin is considered experimentally and analytically.

The influence and benefit of this boundary burr

phenomenon was discussed, too.

Shiina, T.

Boundary Burr Phenomenon for Long-path Time Domain OCT.

DOI: 10.5220/0010327201150121

In Proceedings of the 9th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2021), pages 115-121

ISBN: 978-989-758-492-3

115

2 LONG-PATH TD-OCT SYSTEM

The time-domain OCT is classical and scanning

speed is lower than the current trend Spectral Domain

OCT, but it has a simple structure and linear signal

magnification, and higher flexibility of optical probe

design independent to the other OCT specification

such as scan speed, wavelength and scanning range.

The optical setup of the long path TD-OCT is

illustrated in Fig.1. The SLD (Super Luminescent

Diode) light source of 800nm-band is installed into

the long path TD-OCT. The cylindrical can-package

designed original SLD has been developed for our

industrial OCT. Peltier device for cooler was

excluded in this SLD device to keep the cheaper cost.

Even without a cooler, its optical intensity and

operation are stable for industrial use. It is produced

by Anritsu Co, Ltd. The interference signal is

detected as the Gaussian envelope through the

specialized amplifier and filter circuit.

The long path TD-OCT utilizes the rotational

optical path change mechanism. (Shiina, 2002) This

scanning mechanism consists of a rotating corner

reflector and a fixed mirror. The rotation radius and

its speed decide the measurement range and scan rate,

respectively. The fixed mirror reflects the thrown

beam to the same path. The optical path change

becomes approximately linear motion. The distortion

is about 1 – 2% within the rotation angle of +/-20

degrees. It causes the beat frequency change, too, as

shown in Fig.2. The long-path OCT has a rotation

disk of 60mm radius, of which maximum

measurement range reaches 100mm. Here it is

restricted to 80mm by the reflector size. The rotation

speed is 200rpm. A servo motor is installed to

stabilize the rotation. The rotation disk has a ballast

weight to keep a valanced rotation. Even that, the

rotation sometimes fluctuates slightly as a rotation

jitter due to the timing of the excitation control. The

interference signal was examined via a PC automatic

measurement program. Such a fluctuated signal was

trimmed away and took an average to minimize the

standard deviation by the program. As a result, the

total error restricted within 1μm.

In the last time, the OCT measurement probe was

set to enter the small tank within the measurement

range. The interference signals of the small tank were

obtained at four positions from its glass walls (each

side of the walls). The refractive index was calculated

by the optical path length between the inner water

sides of the small tank walls. The temperature was

controlled to observe the change of refractive index

and estimate the concentration of the solution.

The boundary burr could be observed when the

additional glass plate inserted into the tank solution.

This time, the experimental condition was simplified

to make the boundary burr phenomenon clear

compared with the usual knife-edge pattern.

Figure 1: Structure of long path TD-OCT.

Table 1: Specification of long-path TD-OCT system.

SLD

Anritsu AS8E210GP30M

Center wavelength 830nm

Spectral Half Width 15nm

Optical Output Power 1.2mW

Fiber Assembly 2 x 2 coupler with Collimator

Scanning

Mechanism

DC Servo motor with

(Chiba Motor)

Rotation Disk Diameter 120mmf

Rotation Speed 200 rpm

Resolution 7μm for layer recognition

1μm for positioning accuracy

Scanning Range 100mm with +/- 20 degrees

Figure 2: Optical path length and beat frequency with

optical scanning mechanism at 200rpm.

PHOTOPTICS 2021 - 9th International Conference on Photonics, Optics and Laser Technology

116

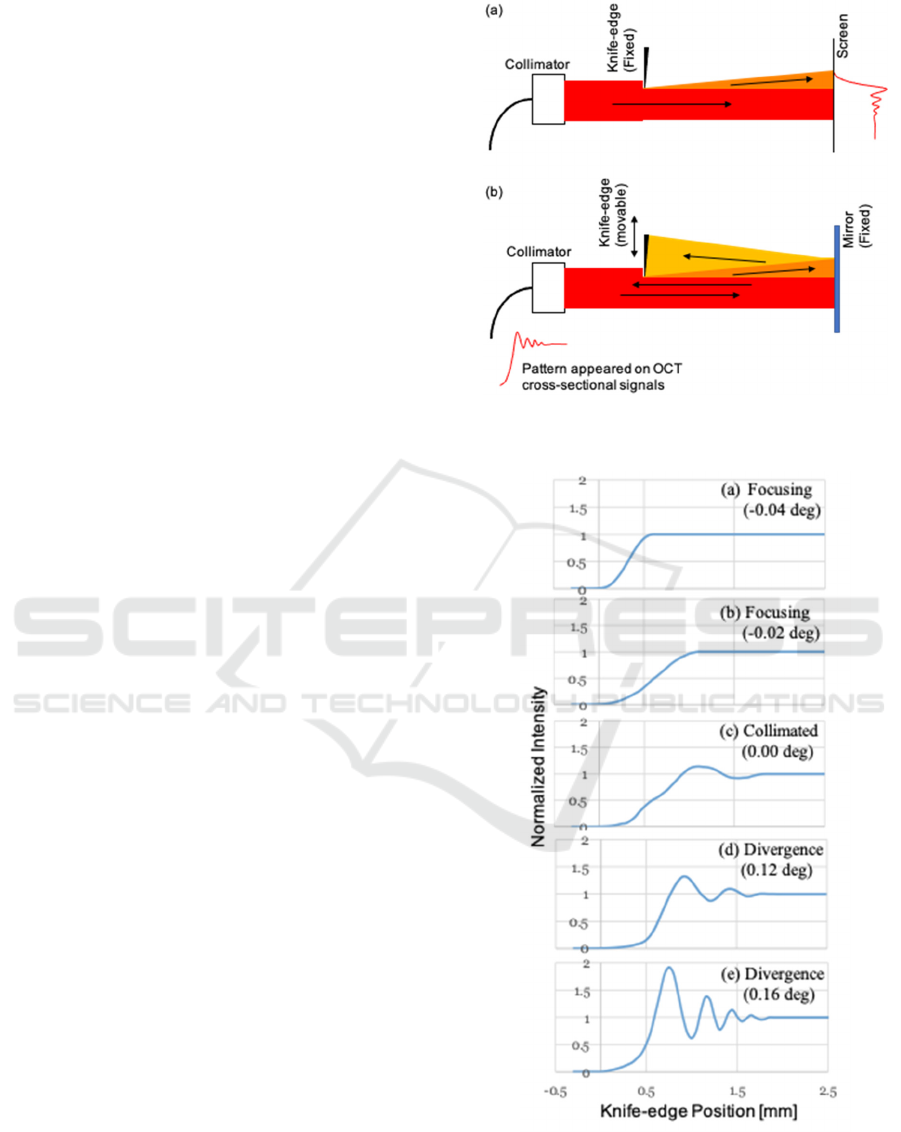
3 EXPERIMENT

To make this boundary burr phenomenon clear, the

water tank was removed and put the knife-edge, for

which was substituted a movable thin mirror, and the

fixed mirror plate to return the light to the probe. The

experimental setup is shown in Fig. 3. Figure 3(a) is

the usual setup to observe the knife-edge diffraction

and Figure 3(b) is this experimental setup to observe

the boundary burr phenomenon with the OCT probe.

In the usual knife-edge observation, the knife-edge is

fixed and the diffraction pattern is appeared on the

screen, which is set after the knife-edge. The distance

between the knife-edge and the screen decide the

diffraction pattern enlargement. On contrary, the

OCT measurement is a point measurement and it

catches the back reflected light. The collimator is

utilized as a transmitting and receiving aperture. To

obtain the pattern, the knife-edge changes its position

by crossing the OCT beam.

The divergence of the optical probe beam was

controlled with the adjustable collimator. The

divergence was adjusted from focusing condition to

spreading condition. The measurement was

conducted by shifting the knife-edge mirror to be

crossed the beam orthogonally. At each knife-edge

mirror position, the OCT interference signal returned

from the fixed mirror was stored. The diffraction like

vibration mitigating waveform will be appeared in the

cross-sectional graph. In the experiment, the direction

crossing the beam was changed appropriately. The

knife-edge position was changed during the optical

probe collimator and the fixed mirror, too.

Figure 4 shows cross-sectional waveforms at each

beam divergence. The horizontal axis was started

with fully covered with the knife-edge mirror as

initial position of “0” and when the knife-edge mirror

was shifted fully from the beam, the intensity

becomes constant as the right side of the graph. The

interference intensity was normalized with the

constant intensity.

It is clear that the boundary burr waveforms ware

appeared at the beam spreading conditions. The beam

focusing condition of Fig. 4(a)-(c), the propagating

beam size should be smaller at the fixed mirror

position, and the waveform was rising up earlier when

the beam focusing becomes stronger. The beam

divergence condition of Fig. 4(c)-(e), the vibration is

getting stronger due to the beam divergence. Their

rising up is getting earlier due to the divergence, too.

In general, the distance between the knife-edge and

the fixed mirror is constant, and the diffraction pattern

of knife-edge never change its rising up condition at

any crossing position of the knife-edge on the beam

Figure 3: Experimental setup for boundary burr observation

with long-path OCT.

Figure 4: Observation Results due to the beam divergence.

As the rising up is getting earlier, the vibration

was getting higher, while the vibration period is

Boundary Burr Phenomenon for Long-path Time Domain OCT

117

getting shorter. This feature is different from the

diffraction pattern of knife-edge.

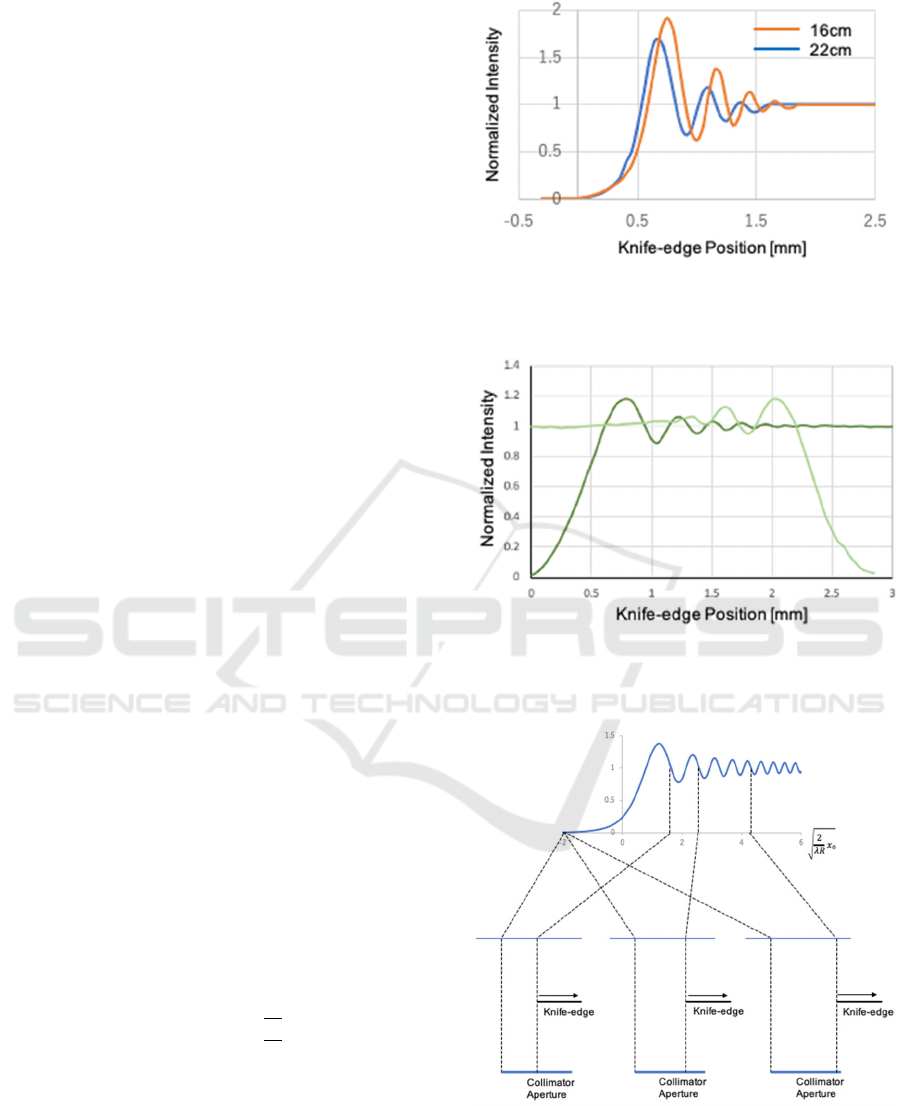
Figure 5 shows the pattern difference due to the

distance between the knife-edge and the collimator.

The beam divergence is 0.16 degrees. This result has

the same feature with the diffraction pattern of knife-

edge, that is, the distance is getting longer, the pattern

is enlarged and the peak intensities of the vibration is

smaller. The vibration period is followed with the

same manner, too.

For check, the pattern observation was conducted

with both of left and right side scan of the knife-edge.

The result is shown in Fig. 6. The beam divergence

was 0.16 degrees. It is natural that both of the

boundary burr patterns are same vibration, while we

can discuss the beam size. It will burr the boundary of

the target. It will be important to distinguish the time

variation of the refractive index and concentration.

How this boundary burr phenomenon will occur?

We considered the experimental condition with the

long-path TD-OCT. Off course, it is particular

condition on long-path OCT. Beam divergence will

be effective on the longer optical path when it is

detected on the inline collimator aperture. When the

knife-edge will start from the covered position, the

interference intensity rises up due to the uncovered

area of the collimator aperture. Then the diffraction

pattern is reflected at the fixed mirror and goes back

to the collimator. At that time, as the first peak area

occupies on the collimator aperture as shown in

Fig. 7(i), the interference intensity rises up at

maximum. The diffraction pattern of knife-edge

repeats the vibration peaks equally plus and minus

against the average intensity. When the knife-edge

position gradually uncovers the collimator aperture

area like fig. 7(ii), the interference intensity generated

by the reflected beam just passed through the

uncovered area will causes the vibration, too. When

the number of the peaks increases, the interference

intensity reaches to the average, that is, settle down to

the constant (Fig. 7(iii)). The distance R between the

knife-edge and the collimator via the fixed mirror,

wavelength 𝜆 and the adequate knife-edge position 𝑥



to cover the collimator aperture, that is, forming the

non-unit diffraction parameter







𝑥



will decide the

boundary burr phenomenon. The long-path OCT had

such parameters balance and observed those patterns.

Figure 5: Pattern variation due to the distance between the

knife-edge and the fixed mirror. The beam divergence is

0.16 degrees.

Figure 6: Left and right sides boundary burr patterns. The

distance between the knife-edge and the collimator was

22cm.

Figure 7: Principle of boundary burr phenomenon on long-

path OCT observation.

(i)

(ii)

(iii)

PHOTOPTICS 2021 - 9th International Conference on Photonics, Optics and Laser Technology

118

4 SIMULATION

To make this boundary burr phenomenon clear, we

conducted the numerical simulation. The

experimental condition is considered in the

simulation, for example, wavelength, knife-edge

setup, propagation distance, aperture size, etc. The

diffracted beam propagates to a certain distance. This

diffracting intensity distribution is visualized. We set

the orbicular beam and the orbicular collimator

aperture, and calculate the 2 dimensional intensity

distribution. The Gaussian distribution was used in

the calculation. Larger the intensity to renter into the

aperture is, Stronger the interreference intensity is.

The parameters are propagation distance between the

knife-edge and the collimator via the fixed mirror.

The core size of the optical fiber was considered with

the focal length of the collimator lens, too.

The intensity distribution at variation of the knife-

edge position is shown in Fig.8. Each image indicates

4𝜇𝑚 × 4𝜇𝑚 cross sectional area at the focal point of

the collimator. The center circle indicates the core

area, which estimates the intensity passed through the

core. The back arrow with a line represents the

shadow of the knife-edge, that is, the knife-edge

inserted from the left side. Its position is shown in the

unit of radius R. The distance between the knife-edge

and the collimator via the fixed mirror was adjusted

to reflect the experimental condition.

When the knife-edge is placed at the position of

0.5R, where 3/4 area was covered. Although 1/4 of

the aperture area is still remained, the intensity will

not rise up. The peak intensity is clear, while it shifts

outside from the knife-edge position. From the knife-

edge position of 0.25R, the returned beam could pass

through the collimator. When the knife-edge position

of -0.25R, the vibration of intensity was started.

Figure 9 shows the intensity distribution due to the

knife-edge position. The same boundary burr pattern

can be simulated, while the concrete matching is not

discussed with intensity ratio and knife-edge

positions. Nevertheless, the feature of the pattern

reflects the phenomenon. The intensity rises up when

the reflected beam passes through more than 1/4 area

of the aperture. The intensity peak was obtained at

0.25R, where the peak intensity in the distribution

reach at the center. After that, the small vibration still

remained but soon settle down to the average. The

rising up period is matched with the experimental

result. The vibration period looks similar to the

experiment, but the second peak is small. The pattern

variation was discussed with the change of beam

divergence and the combination of the collimator lens

focal length and the core size of the optical fiber.

Figure 8: Intensity distributions at each knife-edge position.

Figure 9: Intensity distribution of simulated result.

5 DISCUSSIONS

As the result of this experiment, for sensing purpose,

when the target in the media tank will be detected, its

size and boundary will be burr with this experimental

condition. Such situation is depicted in Fig. 10(a). In

such a case, the beam can be focused to avoid the

Boundary Burr Phenomenon for Long-path Time Domain OCT

119

Figure 10: Step up procedure of the experiment.

boundary burr phenomenon. The focused beam,

however, change the resolution, especially it should

be careful in the long-path measurement. The

boundary burr phenomenon is not only observed at

the fixed mirror, but also at the target reflected signal

itself. It is caused by the propagation of the target

reflected signal to the collimator. It is observed at the

experimental result (Shiina, 2020).

The boundary burr pattern has the information of

the optical property conditions such as refractive

index, target hitting angle, at so on. The known

material target is inserted into the solution to reveal

the phenomenon about the combination of target

material and the solution as shown in Fig.10(b). The

refractive index of the target will change the

diffraction condition. It is helpful to tie up with the

numerical analysis with the theory.

The goal of this project is to visualize the

distribution of the refractive index and concentration

of the target solution as shown in Fig.10(c). They

change due to the temperature and chemical reaction.

The boundary burr pattern reflects them. The

sensitivity and resolution is quite high, and this

system can catch the small difference of the ignition

of change such as freezing reaction and convective

flow, and so on.

6 CONCLUSIONS

In this report, we have developed the long-path TD-

OCT with the positioning accuracy of 1μm and

measurement range of >80mm. With this

experimental set up, the vibration mitigating

waveform like a diffraction pattern by a knife-edge

was observed. The boundary burr phenomenon is

caused by the propagation of the diffraction pattern.

It is proved experimentally and analytically.

The goal of this project is to visualize the

distribution of the refractive index and concentration

of the target solution. Now the experiment shifts to

the next step, that is, the target is inserted into the

solution to obtain its distribution with the information

of refractive index, position and concentration change

due to the temperature and chemical reaction.

REFERENCES

Brezinski M. E. and Fujimoto J. G., 1999, “Optical

Coherence Tomography: High-Resolution Imaging in

Nontransparent Tissue”, IEEE J. Quant. Electron.,

Vol. 5, No. 4, pp.1185-1192.

Danielson B. L. and Boisrobert C. Y. 1991, “Absolute

optical ranging using low coherence interferometry”,

App. Opt. Vol. 30, No.21 pp.2975-2979.

Harvey A. H., Gallagher J. S., and J. M. H. L.,

1998, ”Revised Formulation for the Refractive Index

of Water and Steam as a Function of Wavelength,

Temperature and Density”, J. Phys. Chem. Ref. Data,

Vol.27, pp.761-774.

Huang D., Swanson E. A., Lin C. P., Schuman J. S., Stinson

W. G., Chang W., Hee M. R., Flotte T., Gregory K.,

Puliafito C. A., J. G. Fujimoto, 1991, “Optical

Coherence Tomography”, Science, Vol. 254,

pp.1178- 1181.

Saeki K., Huyan D., Sawada M., Sun Y., Nakamura A.,

Kimura M., Kubota S., Uno K., Ohnuma K., and

Shiina T., 2020, “Measurement algorithm for real

front and back curved surfaces of contact lenses”,

Applied Optics, Vol. 59, No. 28, pp.9051-9059.

Shiina T., Moritani Y ., Ito M., and Okamura Y ., 2003,

"Long optical path scanning mechanism for optical

coherence tomography", Applied Optics, Vol.42, No.

19, pp.3795-3799.

Shiina T., Miyazaki H., and Honda T., 2009, “Factory built-

in type simplified OCT system for industrial

PHOTOPTICS 2021 - 9th International Conference on Photonics, Optics and Laser Technology

120

application”, IEEJ C、Vol.129, No.7, pp.1276-1281,

(Japanese)

Shiina T., 2014, “Optical Coherence Tomography for

industrial application” Handbook of Optical

Metrology 2

Edition, CRC Press, Chapter 30,

pp.769 – 790.

Shiina T. 2019, “Solution Concentration and Temperature

Measurements by Long-path Optical Coherence

Tomography”, Photoptics 2020, 20-RP-33.

Song G. and Harding K G, 2012, “OCT for industrial

applications”, Proc. Of SPIE, 8536.

Tanno N. 1990, Japanese Patent 2010042.

Yoshizawa t. Eds, 2015, Handbook of optical metrology:

principles and applications, second edition, CRC

Press.

Boundary Burr Phenomenon for Long-path Time Domain OCT

121