Modelling and Simulation of an Aerosol-on-Demand Print Head

with Computational Fluid Dynamics

Martin Ungerer

, David Zeltner

, Achim Wenka

, Ulrich Gengenbach

and Ingo Sieber

Institute for Automation and Applied Informatics, KIT, Hermann-von-Helmholrz-Platz1, 76344 Egg.-Leo, Germany

Institute for Micro Process Engineering, KIT, Hermann-von-Helmholrz-Platz1, 76344 Egg.-Leo, Germany

Keywords: Computational Fluid Dynamics, Modelling, Simulation, Additive Manufacturing.

Abstract: In this paper we present the functional validation of a newly developed concept of a print head for aerosol-

on-demand printing using fluid dynamical modelling and simulation. In our concept of the aerosol-on-demand

print head, the ink is atomised by ultrasonic excitation and focussed by a sheath gas in a converging nozzle.

The special feature of this new concept is aerosol generation directly in the print head thus allowing for on-

demand operation. Using computational fluid dynamics (CFD), a pre-manufacturing study is being carried

out to validate the operation of the concept as well as to find a design-for-manufacture.

1 INTRODUCTION

Novel devices and systems with special chemical,

physical or optical properties can be realised by

printing processes using functionalized inks

(Sirringhaus and Shimoda 2003; Sieber, Thelen, and

Gengenbach 2020, 2021; Magdassi 2010). Drop-on-

demand inkjet printing has achieved a high level of

development in printing functional structures

(Sirringhaus and Shimoda 2003). In contrast to drop-

on-demand inkjet printing, aerosol jet printing has the

advantages of potentially printing finer structures

with higher resolution and the capability to print on

three dimensional structures (Mette et al. 2007,

Neotech 2021), or even bond multiple chip layer

together, thus replacing the need for wire bonding

(Hedges and Marin 2012).

Aerosol jet printing is a continuous printing

process where a fine spray of atomised ink is focussed

hydrodynamically by means of a sheath gas flow.

This results in a stable, and over a range of several

millimetres well-collimated aerosol jet (Ganz et al.

2016; Gupta et al. 2016). A currently unsolved

problem in aerosol jet printing is, that it cannot be

operated in on-demand mode. This is because a run-

in time of the aerosol generation is required, since in

current aerosol jet printers the generation of the

aerosol takes place in an atomisation unit which is

https://orcid.org/0000-0001-9762-0019

https://orcid.org/0000-0003-2811-7852

detached from the nozzle. Thus, switching the jet

generation on and off for on-demand operation is not

possible (Chang, Facchetti, and Reuss 2017; Hedges

and Marin 2012).

To address this point we develop a new principle

for an aerosol jet-on-demand (AoD) print head

(Ungerer et al. 2018). Centerpiece of this concept is

the integration of the atomisation unit into the print

head. The aerosol is generated by means of ultrasonic

atomisation of the ink in the capillary with the aid of

a piezo actuator. Aerodynamical focusing of the

aerosol jet is based on the sheath gas mass flow, the

aerosol mass flow and the outlet nozzle. Thus, a

compact system design can be developed which will

allow for printing operation in all spatial directions, a

widely tunable nozzle-to-substrate distance, as well

as a jet-on-demand mode of operation (Ungerer

2020; Sieber et al. 2022).

This paper will focus on the proof-of-concept

based on CFD simulations. In the simulations the

functional ink is modelled as distilled water. This is

justified, since the aerodynamic focusing is

independent of the dynamic viscosity of the fluid or

the particle content in the fluid. Hence, an

aerodynamic focusing of all fluids that can be

atomised in the capillary is possible. For modelling of

the print head the limitations of the manufacturing

processes used (design-for-manufacturing) are taken

Ungerer, M., Zeltner, D., Wenka, A., Gengenbach, U. and Sieber, I.

Modelling and Simulation of an Aerosol-on-Demand Print Head with Computational Fluid Dynamics.

DOI: 10.5220/0011258100003274

In Proceedings of the 12th International Conference on Simulation and Modeling Methodologies, Technologies and Applications (SIMULTECH 2022), pages 44-51

ISBN: 978-989-758-578-4; ISSN: 2184-2841

into account. The organisation of this paper is as

follows: Section 2 addresses the design-for-

manufacturing of a laboratory setup of the print head.

In Section 3, modelling of the print head is presented.

Herein a brief theoretical description of the used

model approach is presented as well as the geometry

model and the meshing. Simulation and its results are

presented in Section 4, the paper concludes with a

discussion of the results and a brief outlook in

Section 5.

2 DESIGN-FOR-MANUFACTURING

Fig. 1 shows the schematic of the principle design of

the inner contour of the aerosol print head. The

aerosol is generated by ultrasonic atomisation of the

ink in the capillary with the aid of a piezo actuator.

The tip of the capillary is located in a rotationally

symmetric chamber, the mixing chamber, into which

a sheath gas is injected to aerodynamically focus the

atomised ink in the nozzle.

Figure 1: Schematic of the principle design.

In order to obtain a uniform, rotationally

symmetric aerosol jet, a uniform flow of the sheath

gas around the capillary is necessary. To achieve this,

the velocity profile of the sheath gas, which flows in

from four inlets evenly distributed around the

circumference, is homogenised in a plenum chamber,

denoted as antechamber. The concept of a plenum

chamber for homogenisation is well known in fluid

mechanics (Guha, 2010) and rules of thumb exist to

achieve good mixing and thus homogenisation using

a large volume, many baffles and the longest possible

distance in the antechamber. Hence, the antechamber

is designed as a meandering structure, which makes

good use of the given volume, has a long distance and

sharp deflections at the bending, thus leading to

homogeneous flow around the capillary.

Downstream of the mixing a nozzle follows in

which the ink droplets are aerodynamically focused.

Due to the aerosol generation inside of the mixing

chamber, discontinuous operation is possible and thus

enables the feature of AoD printing.

For manufacturing of a functional model of the

aerosol print head for future use in a laboratory setup,

a lathing process is available at our institute. Lathing

is a process well suited to manufacture the almost

completely rotationally symmetrical geometry of the

print head. Also, surfaces with sufficiently low

roughness can be achieved by lathing. The

application of the lathing process results in a number

of requirements which must be taken into account in

the design of the print head. For example, the shallow

angles required for aerodynamic focusing cannot be

produced with the conventional lathing tool due to its

limited insertion depth. This leads to a separation of

the nozzle geometry into two sections: One with a

nozzle angle of α

= 45°, which can be produced with

the standard lathing tool, and a tip with a nozzle angle

of α

= 15°, which is used for focusing the aerosol.

This tip is manufactured with a conical milling tool.

The minimum diameter of the nozzle exit, which can

be manufactured with the conical milling tool, is 1

mm. The design parameters resulting from the

process limitations are summarised in Table 1.

Table 1: Design parameters of the fabrication process.

Parameter Value

Nozzle angle α

[°]

Nozzle angle α

[°]

Nozzle exit diameter d [mm]

As material for the print head, the aluminum alloy

AlMgSi1 is selected. These are the boundary

conditions resulting from the fabrication processes

used to be considered in geometrical modelling of the

print head.

3 MODELLING

Modelling of the AoD-print head is on the basis of

CFD, a numerical technique used to solve fluid

mechanical problems. We are using Ansys Fluent in

the versions R19.3 and R20.1 as CFD tool.

3.1 Theory

Our chosen modelling approach also takes turbulent

flows into account. In principle, turbulence is

described by the Navier-Stokes equations. However,

a direct numerical simulation based on the Navier-

Modelling and Simulation of an Aerosol-on-Demand Print Head with Computational Fluid Dynamics

Stokes equations is not possible in most cases and

averaging techniques are used to filter out all or at

least parts of the turbulent spectrum (Ansys 2021).

Ansys Fluent offers a large number of different

turbulence models, none of which can be regarded as

universally valid. For the work presented, we have

used the Reynolds-averaged Navier-Stokes equations

(Eqs. 1, 2).

𝜕𝜌

𝜕𝑡

𝜕

𝜕𝑥





𝜌𝑢





(1)

𝜕

𝜕𝑡



𝜌𝑢





𝜕

𝜕𝑥



𝜌𝑢



𝑢



=

−

𝜕𝑝

𝜕𝑥



𝜕

𝜕𝑥



𝜇



𝜕𝑢



𝜕𝑥



𝜕𝑢



𝜕𝑥



−

𝛿



𝜕𝑢



𝜕𝑥





+

𝜕

𝜕𝑥





−𝜌𝑢





𝑢







(2)

Eq. 1 is the continuity equation, describing the

conservation of mass where ρ is the density and u

the mean velocity. The conservation of momentum is

represented by Eq. 2 where p is the static pressure and

the symbol 𝛿



denotes the Kronecker-Delta. The

Reynolds-averaged Navier-Stokes equations

equations are generally favourable in terms of

computational effort and time and are thus very well

suited for the calculation of complex turbulent flows

(Ansys 2021). By eliminating all turbulent structures

from the flow, a uniform flow of the averaged

velocity and pressure fields is achieved. Based on the

Reynolds-averaged Navier-Stokes equations

equations a large variety of engineering applications

can be modeled. For our approach, we use the k-𝜔-

𝑆𝑆𝑇 model (shear stress transport) which is a

compressible turbulence model. Here, two additional

transport equations are solved, one for the turbulence

kinetic energy 𝑘 (Eq. 3) and one for the specific

dissipation rate 𝜔 (Eq. 4).

𝜕

𝜕𝑡



𝜌𝑘



𝜕

𝜕𝑥





𝜌𝑘𝑢





𝜕

𝜕𝑥







𝜕𝑘

𝜕𝑥





+ 𝐺



−𝑌



+ 𝑆



(3)

𝜕

𝜕𝑡



𝜌𝜔



𝜕

𝜕𝑥





𝜌𝜔𝑢





𝜕

𝜕𝑥







𝜕𝜔

𝜕𝑥





+ 𝐺



−𝑌



+ 𝐷



+ 𝑆



(4)

With 𝐺



representing the production of turbulence

kinetic energy 𝑘 and 𝐺



the generation of the specific

dissipation rate 𝜔 . 𝛤



and 𝛤



give the effective

diffusivity of 𝑘 and 𝜔, respectively, while 𝑌



and 𝑌



imply the dissipation of 𝑘 and 𝜔 due to turbulence.

𝐷



is the cross-diffusion term, 𝑆



and 𝑆



are user-

defined sources, respectively. Detailed information of

the calculation of this parameter can be found in

Wilcox (2006) and Menter (1994).

The main advantage of the k-𝜔 model is that the

boundary layers are also modelled thus leading to

better results near the walls. In addition, on the basis

of k-𝜔 models boundary layer flows with

unfavourable pressure gradient and dissipation can be

better predicted. In the k-ω models of the SST,

elements of the ω-equation and the ε-equation are

combined to avoid the sensitivity with respect to free

flow inherent to the standard k-ω model.

Furthermore, the SST model is calibrated to

accurately calculate flow separation from smooth

surfaces.

Modelling of the aerosol takes place with respect

to the Euler-Lagrange consideration as discrete

phases. In the Discrete Phase Model the droplet tracks

are calculated inside of the velocity field of the

continuous phase. The Euler-Lagrange approach

neglects particle-particle interactions, and this

requires that the discrete phase occupies only a low

volume fraction (Ansys 2021). Since we estimate a

volume fraction of the discrete phase of less than 10%

of the total volume, the Euler-Lagrange consideration

is well suited for efficient calculation of the

individual droplets due to the small total number of

droplets per volume fraction. The source

characteristic of the aerosol generation is modelled

using the cone injection model of Ansys Fluent which

describes a conic-shaped particle injection for the

aerosol where the following input parameters are

available: Origin, particle distribution, temperature,

cone axis, aerosol velocity, cone angle, particle

diameter, mass flow, and the azimuth. The properties

set in the model are shown in Tab. 2.

Table 2: Injection properties.

Parameter Value

x-Position [m]

y- Position [m]

z- Position [m]

Particle distribution

Particle diameter [m]

Temperature [K]

Cone axis

Cone angle [°]

Azimuthal start angle [°]

Azimuthal stop angle [°]

Velocity magnitude [m/s]

Mass flow [kg/s]

uniform

2⋅ 10

-5

300

(1/0/0)

360

aramete

3.2 Geometry

The geometry model must strictly follow the

conditions and design rules of the manufacturing

processes as defined in Section 2 and summarised in

Tab. 1. Geometry parameters, which do not have a

direct impact on the focussing of the aerosol jet, as

long as they are within specific boundaries, are:

SIMULTECH 2022 - 12th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

 dimensions of the mixing chamber and

 position and angle of the influx channel.

Length and width of the mixing chamber can be

freely chosen, as long as they are sufficiently large to

avoid a wetting of the inner walls by insufficient

focussing. Manufacturing restriction on the other

hand would prefer a short and compact design – these

contradictory requirements must be well balanced

and validated by simulations.

With respect to the inlet channel of the

antechamber, free parameters are positioning and

inlet-angle. The momentum transfer between aerosol

and sheath gas depends on the vectorial difference of

the velocities, i.e. focusing of the aerosol jet can be

achieved on the one hand by a higher absolute

difference in the velocities and on the other hand by

different flow directions. The highest deflection of

the aerosol is achieved by a sheath gas flow

perpendicular to the droplet tracks. This can be

obtained by placing the influx channel in the center of

the mixing chamber at the height of the capillary tip.

This positioning will cause eddies. To achieve an

even, eddy-free flow in the mixing chamber the influx

channel should be placed ahead of the capillary tip in

flow direction. Furthermore a sharp edged transition

from channel to chamber must be avoided, since

again such a geometrical feature would cause eddies.

For those reasons the inlet channel from the

antechamber to the mixing chamber is tilted by an

angle of 60° with respect to the cylindrical chamber

wall. This will result in an eddy-free flow of the

sheath gas around the capillary. Figure 2 shows the

geometry model of the print head.

Figure 2: Geometry model of the print head.

3.3 Meshing

The result of a CFD simulation depends not only on

how dense the mesh is in the area of large velocity

gradients (e.g. on the walls) but also on the type of

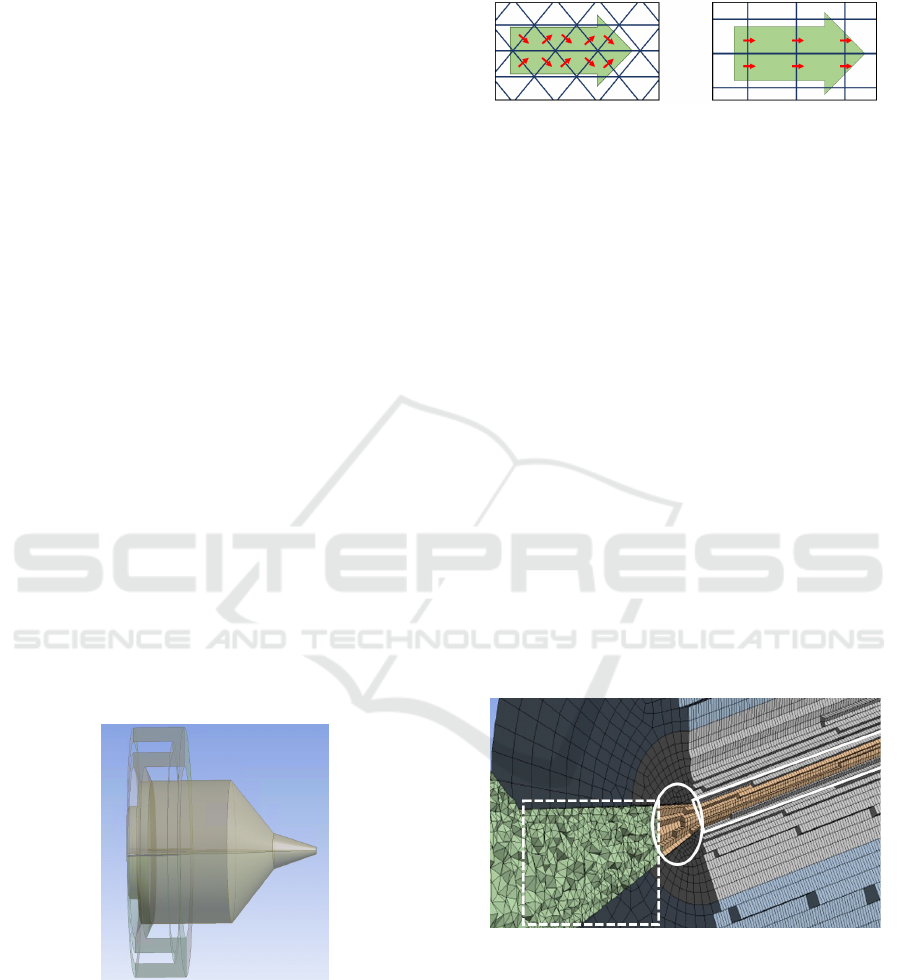
elements and symmetries in the meshes (see Fig. 3).

Interfaces that are not perpendicular to the largest

mass flows (Fig.3, left) enhance numerical diffusion.

Figure 3: Mass flow in different meshes. Physical flow

direction through the model (green) and flow through the

interfaces of the elements (red).

Particularly critical areas with respect to meshing

are the nozzle and the free jet. In the conical region of

the nozzle, the element size decreases with the nozzle

diameter. Thus, the mass flow through successive

elements remains approximately the same, which

reduces numerical diffusion. In this case, usage of a

tetrahedral mesh is appropriate (see Fig. 4, left,

dashed frame). In the transition zone between nozzle

and free jet at the tip of the nozzle, a transfer from

tetrahedral elements to hexahedral elements is

implemented (see Fig. 4, center, solid ellipse).

A free jet does not always have a unique,

mathematically stable solution, so numerical

diffusion must be minimised to improve convergence.

This is achieved by selecting the mesh in such a way

that the boundary surfaces of the elements are as

perpendicular as possible to the largest mass flows

(see Fig. 4, right, solid frame). This would not be the

case for elements in tetrahedral form or asymmetric

meshes. Here the generation of asymmetric solutions

due to numerical diffusion are more likely.

Figure 4: Section through the mesh used; decreasing

element size in the area of the nozzle (left, dashed frame),

symmetrical meshing in the area of the free jet (right, solid

frame) and transition zone (middle, solid ellipse).

In turbulent flows, special attention must be paid

to the boundary conditions at the walls, since different

layers form here. The transition from the fully

turbulent to the wall can be divided into three layers:

the purely viscous bottom layer, the transition layer

and the overlap layer (Schlichting and Gersten 2006).

Modelling and Simulation of an Aerosol-on-Demand Print Head with Computational Fluid Dynamics

The k-𝜔-𝑆𝑆𝑇-model used also models the boundary

layers and requires a much finer meshing at the wall.

Comparability of the boundary layers in different

flows is achieved by the de-dimensioned wall

distance (Eq. 5).

𝑦



= 𝜌𝑢



𝑦

𝜇

(5)

with 𝑢









Here ρ denotes the density, u

the shear stress rate,

µ the viscosity, and τ the shear stress in that layer.

The required resolution of the meshing thus depends

on both the choice of turbulence model and the

velocity gradients due to the de-dimensioned wall

spacing (Ansys 2021). This means that for the same

geometry and different flow velocities, different

densities of meshing must be chosen.

In our model, the wall layers are meshed at a very

fine resolution, so that the first mesh element is in the

range 𝑦+ ≈ 1. Thus, the influences of the purely

viscous sublayer are fully represented (Ansys 2021).

This resolution is achieved by using prism elements

in this layers.

Furthermore, since the Euler-Lagrange model

used involves a particle-related consideration of the

discrete phase, it must be ensured that in principle a

particle can be located completely within a mesh

element. Therefore, care must be taken that the

minimum size of the mesh elements is chosen in a

way that it reaches a multiple of the particle size. The

mesh consists of 4,107,235 elements with 1,097,103

nodes and a minimum edge length of 60 µm.

Generation of the prism layers is conducted with a

transition rate of 0.272 and a growth rate of 1.2.

4 SIMULATION

The simulation is carried out on a workstation. The

processor used is the AMD Ryzen Threadripper

3970X with 32 cores, 64 threads at 3.7 GHz, 128 GB

RAM and an Nvidia Titan RTX graphics processor

with 24 GB.

4.1 Modelling of Ink and Sheath Gas

The ink is modelled as distilled water so that the

discrete phase consists of atomised droplets.

Replacing the functional ink with distilled water in

the model is permissible because aerodynamic

focusing does not depend on the dynamic viscosity of

the ink or the particle content in the ink. Hence, once

the simulative functional proof of aerodynamic

focusing has been provided, it is basically possible for

all liquids that can be atomised in the capillary. If the

droplets of functional inks have a different

momentum than the droplets of distilled water due to

their density or diameter, the sheath gas mass flow

can be adjusted in such a way that focusing of the

aerosol jet is achieved.

The droplets make up only a small volume

fraction in the mixing chamber (< 10 % of the total

volume), thus the particle-particle interaction of the

droplets can be neglected. However, a coupling of the

discrete phase with the continuous phase is

established so that a momentum transfer of the

aerosol to the sheath gas, which is not negligible for

large aerosol mass flows, is taken into account. Table

3 shows the simulation parameter of the aerosol at the

outlet of the capillary (Zeltner 2020).

Table 3: Simulation parameters of the aerosol at the outlet

of the capillary.

Parameter Value

max. exit angle [°]

max. diameter of droplets [µm]

max. exit velocity [m/s]

max. aerosol mass flow [kg/s]

1.21 ⋅ 10

-5

The sheath gas is the continuous phase in the

Discrete Phase Model. As sheath gas Argon is

modelled as an ideal gas. The parameters are dipicted

in Table 4.

Table 4: Modelled properties of Argon.

Parameter Value

Specific heat [J/(kg K)]

Thermal conductivity [W/(m K)]

Viscosity [kg/(m s)]

Molecular weight [kg/kmol]

520.64

0.0158

2.125 ⋅ 10

-5

39.948

4.2 Determination of the Operating

Point

Based on the modelling of the entire print head,

simulations of the printing process are carried out. In

the first step, the operating points are determined at

which the aerosol can be focused by means of the

sheath gas without contact with the wall. To

determine these working points, the mass flow of the

sheath gas is changed step by step for varying mass

flows of the aerosol until no contact of the aerosol

with the nozzle walls occurs.

Figure 5 shows the relationship between sheath

gas mass flow, aerosol mass flow, and wall contact.

The red diamonds depict mass flow combinations

resulting in a wall contact, while blue dots denote

mass flow combinations without wall contact. The

SIMULTECH 2022 - 12th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

region of operation without wall contact can be

separated by a straight line from the region with wall

contact.

Figure 5: Relationship between sheath gas mass flow and

aerosol mass flow. Blue dots: no wall contact; red

diamonds: wall contact; green encircled cross: chosen

operating point.

Since the Reynolds number is linearly dependent

on the sheath gas mass flow, it is also possible to

directly infer the Reynolds number for the process

window. With the help of the diagram in Fig. 5, it is

possible to determine a maximum aerosol mass flow

at a specified sheath gas mass flow. For proper

operation of the nozzle, a laminar flow of the ink is

required. The operating point of the nozzle is

therefore selected to achieve a low Reynolds number

to allow for laminar flow. Hence, an operating point

at a Reynolds number of Re = 1200 is chosen for the

further simulations, which corresponds to a sheath

gas mass flow of 2 ⋅ 10

-5

kg/s. If the momentum of the

aerosol mass flow is too large, eddies will form due

to mass conservation, which will deflect some of the

droplets towards the nozzle wall (Fig. 6 b).

Figure 6: Eddy generation in the nozzle at Re = 1200 and

increasing aerosol mass flow. Droplet tracks (black) and

flow of the sheath gas (green). a): no wall contact, aerosol

mass flow 1 ⋅ 10

-6

kg/s; b): light wall contact, aerosol mass

flow 2 ⋅ 10

-6

kg/s; c): strong wall contact, aerosol mass flow

5 ⋅ 10

-6

kg/s.

Furthermore, if the aerosol mass flow is too large,

the aerosol cannot be deflected sufficiently towards

the axis of symmetry, so that wetting of the nozzle

walls occurs (Fig. 6 c). If the mass flows are in the

range above the straight line of Fig. 5, no eddies form

and the aerodynamic focusing is large enough to

prevent wetting of the nozzle walls (Fig. 6 a). Hence,

the operating point is specified with a sheath gas mass

flow of 2 ⋅ 10

-5

kg/s (Re = 1200) and an aerosol mass

flow of 1 ⋅ 10

-6

kg/s (marked by the green encircled

cross in Fig. 5) to ensure an operating point with no

wall contact.

4.3 Steady-state and Transient

Considerations

As the operating point of the print head has been

determined, the functionality of the printing process

can be examined. The free jet is included in the

investigations, as the focusing of the jet outside the

nozzle is to be examined. At the boundary of the

control space, a constant pressure to the environment

is set as a boundary condition. Inflow and outflow is

allowed for all modeled phases (pressure outlet).

Figure 7 shows the static pressure distribution for the

model used.

Figure 7: Static pressure.

In order to deliver reproducible results, a time-

independent, i.e. steady, behaviour of the printing

process is necessary. Therefore, steady-state

simulations are carried out first. The settings listed in

Tables 3 and 4 are used for this purpose. Results of

the steady-state simulations at the operating point are

a focussed aerosol jet with a diameter of approx. 0.8

mm when leaving the nozzle and approx. 0.15 mm at

the focal point, located at a distance of 4.2 mm from

the nozzle (Fig. 8 b). In the nozzle no wall contact

occurs and the flows are rotationally symmetrical.

Modelling and Simulation of an Aerosol-on-Demand Print Head with Computational Fluid Dynamics

Hence, in steady-state, the proper functioning of the

AoD print head is validated by simulation.

Time-resolved transient simulations are also

performed to investigate whether truly stable steady-

state solutions are present or whether they are merely

averages of a transient solution.

Figure 8: Simulation of the droplet tracks for the entire

system. The rectangular region in a) is the region of interest.

b) steady-state simulation, c) transient simulation.

Due to the transient observation, another particle

model has to be used, which is called unsteady

particle tracking in Ansys Fluent. In this particle

model, individual droplets have a position and a

velocity in every time step. No continuous trajectories

are calculated, but only the position of the droplets is

updated. The number of newly generated droplets per

time step is set to 20 to limit the computational effort.

A very fine time increment is necessary for the

droplets after they leave the capillary. The fine time

increment leads to extremely slow movements of the

droplets elsewhere in the model, so that in the

simulation over 1000 time increments are necessary

until a steady aerosol flow reaches the substrate. This

makes the time-resolved simulations very

computationally intensive. The required time

increment for the droplets was set at 0.1 ms per

increment. The total simulated time span is approx.

0.5 s.

The time-resolved simulations give similar results

to the steady-state simulations (see Fig. 8 c). Again,

no wall contact occurs in the nozzle. The position of

the focal point and the velocities of the droplets are

identical in both approaches (see Fig. 8 and Table 5).

However, the diameter of the focal point is larger

in the transient simulations than in the steady-state

simulations. A deflection or defocusing of the aerosol

jet due to turbulence in the free jet is neither observed

in the steady-state nor in the time-resolved

simulations.

As a result, it can be stated that steady-state

solutions form at the free jet. These results confirm

the assumption of a steady-state behaviour, which is

of existential importance for functional printing.

Thus, all requirements for AoD-printing are

fulfilled and the newly developed concept has been

validated by simulation.

Table 5: Properties of steady and transient simulations.

5 CONCLUSIONS

In this article we present the proof-of-concept of a

new principle of an aerosol jet-on-demand print head

for functional printing by means of CFD simulations.

Aerodynamic focusing of the aerosol jet is based on

properly adjusting the mass flows of the sheath gas

and the aerosol and the appropriate design of the

outlet nozzle. Design considerations are made with

respect to generation of a homogenised sheath gas

flow and the manufacturing processes available at our

institute, thus resulting in a design-for-manufacturing

approach. Modelling and meshing of the print head is

discussed for the critical areas to avoid numerical

diffusion and to improve the convergence.

Simulation of the operation of the print head is

done by modelling the functional ink as distilled

water. Since aerodynamic focusing is independent of

the dynamic viscosity of the fluid or the particle

content in the fluid, this approach is permissible. In

principle, aerodynamic focusing of all fluids that can

be atomised in the capillary is possible. If, due to

density or diameter, the droplets of functional inks

have a different momentum than the droplets of

distilled water, the mass flows of the sheath gas and

the aerosol have to be adjusted to achieve focusing of

the aerosol jet.

There are two conditions which must be met for

reliable function of the aerosol jet-on-demand print

Pro

ert

stead

transient

Reynolds number

max. velocity sheath gas

max. velocity droplets

velocity droplets @ nozzle exit

Diameter aerosol jet @ nozzle

exit

Diameter aerosol jet @ focus

focus position

1200

18.3 m/s

13.6 m/s

7 m/s

0.8 mm

0.15 mm

4.2 m

1200

18.3 m/s

13.6 m/s

7 m/s

0.9 mm

0.35 mm

4.2 m

SIMULTECH 2022 - 12th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

head. These are on the one hand the generation of a

stable and focused aerosol beam, and on the other

hand the prevention of wetting of the inner nozzle

wall by the aerosol. The fundamental operating

parameters ensuring these conditions are found by

CFD simulations. In a first step the relationship

between the mass flows of the sheath gas and the

aerosol and the wetting of the inner wall is

investigated leading to an operating point at

Re = 1200 ensuring a non-wetting condition. Since a

time-continous operation of the print head is a

prerequisite of a reliable function of aerosol-on-

demand printing, steady-state as well as transient

simulations are performed to investigate for time

dependency of the solutions. The transient

simulations give identical results as the steady-state

simulations concerning the position of the beam focus

as well as the velocity distribution. Thus, all

requirements for aerosol-on-demand printing are

fulfilled and the newly developed concept has been

validated by simulation.

In future studies, the simulative findings will be

experimentally evaluated and validated by realising

the design-for-manufacture as experimental setup.

REFERENCES

Ansys 2021. Ansys Fluent User’s Guide, Release 2021 R2,

ANSYS, Inc. Canonsburg, PA, USA

Chang, J.S., A.F. Facchetti, and R. Reuss. 2017. A circuits

and systems perspective of organic/printed electronics:

Review, challenges, and contemporary and emerging

design approaches. IEEE Journal on emerging and

selected topics in circuits and systems 7, : 1–21. doi:

10.1109/JETCAS.2017.2673863.

Ganz, S., H.M. Sauer, S. Weißenseel, J. Zembron, R. Tone,

E. Dörsam, M. Schaefer, M. Schulz-Ruthenberg. 2016.

Printing and Processing Techniques. Nisato, G., Lupo,

D., and Ganz, S. (editors): Organic and Printed

Electronics: Fundamentals and Applications: 48-116.

Singapore: Pan Stanford Publishing.

Gupta, A.A., A. Bolduc, S. G. Cloutier and R. Izquierdo.

2016. Aerosol Jet Printing for printed electronics rapid

prototyping, IEEE International Symposium on

Circuits and Systems (ISCAS), Montreal, QC: 866-869,

doi: 10.1109/ISCAS.2016.7527378.

Guha, A. and Smiley, B. 2010. Experiment and analysis for

an improved design of the inlet and nozzle in Tesla disc

turbines. Proceedings of The Institution of Mechanical

Engineers Part A-journal of Power and Energy - PROC

INST MECH ENG A-J POWER 224. doi:

10.1243/09576509JPE818.

Hedges, M. and A. B. Marin. 3D Aerosol Jet Printing -

Adding Electronics Functionality to RP/RM. Direct

Digital Manufacturing Conference (Berlin) 2012. url:

https://optomec.com/wp- content/uploads/2014/04/Opt

omec_NEOTECH_ DDMC_3D_Aerosol_Jet_Printing

.pdf. (accessed: 24.02.2020).

Magdassi, S. 2010. The Chemistry of Inkjet Inks. Singapore:

World Scientific Publishing.

Menter, F.R. 1994. Two-Equation Eddy-Viscosity

Turbulence Models for Engineering Applications.

AIAA Journal. 32(8): 1598–1605.

Mette, A., P. L. Richter, M. Hörteis, S. W. Glunz. 2007.

Metal Aerosol Jet Printing for Solar Cell Metallization,

Progress in Photovoltaics 15, 621–627. doi:

10.1002/pip.759.

Neotech. 2021. 3D Printed Electronics applications realised

by Neotech AMT. url: https://neotech-amt.com/

applications. (accessed: 19.10.2021).

Schlichting, H. and K. Gersten. 2006. Grenzschichttheorie.

10. Auflage. Berlin, Heidelberg: Springer. doi:

10.1007/3-540-32985-4.

Sieber, I. R. Thelen, and U. Gengenbach. 2020. Assessment

of high-resolution 3D printed optics for the use case of

rotation optics. Opt. Express 28: 13423-13431.

Sieber, I., R. Thelen, and U. Gengenbach. 2021.

Enhancement of High-Resolution 3D Inkjet-printing of

Optical Freeform Surfaces Using Digital Twins.

Micromachines 12(1): 35. https://doi.org/10.3390/mi1

2010035.

Sieber, I., Zeltner, D., Ungerer, M., Wenka, A., Walter, T.,

Gengenbach, U. (2022). Design and experimental setup

of a new concept of an aerosol-on-demand print head.

Aerosol Science and Technology. Taylor & Francis.

DOI: 10.1080/02786826.2021.2022094

Sirringhaus, H. and T. Shimoda. 2003. Inkjet Printing of

Functional Materials. MRS Bulletin 28(11): 802–806.

doi: 10.1557/mrs2003.228.

Wilcox, D.C. 2006. Turbulenc Modelling for CFD, 3rd

edition). La Canada, California: DCW Industries, Inc.

Ungerer, M., A. Hofmann, R. Scharnowell, U. Gengenbach,

I. Sieber, and A. Wenka. 2018. Druckkopf und

Druckverfahren [Print head and printing method].

Patent: DE 10 2018 103 049.5.

Ungerer, M. 2020. Neue Methodik zur Optimierung von

Druckverfahren fur die Herstellung funktionaler

Mikrostrukturen und hybrider elektronischer

Schaltungen [New methodology for optimising printing

processes for the production of functional

microstructures and hybrid electronic circuits].

Dissertation, Karlsruhe, Germany: Karlsruhe Institute

of Technology (KIT).

Zeltner, D. 2020. Auslegung und Evaluierung einer

Dosiereinheit zur additive Fertigung funktionaler

Strukturen [Design and evaluation of a dispensing unit

for additive manufacturing of functional structures].

Karlsruhe: Karlsruhe Institute of Technology, Institute

for Automation and Applied Informatics.

Modelling and Simulation of an Aerosol-on-Demand Print Head with Computational Fluid Dynamics