Modelling Cyber Vulnerability using Epidemic Models

Bao Nguyen

Defence Research Development Canada and University of Ottawa, School of Mathematics and Statistics, Canada

Keywords: Cyber Defence, Epidemic Models, Biological Diseases, SIR (Susceptible – Infected – Removed) Model.

Abstract: This paper documents an epidemic model known as SIR (Susceptible – Infected – Removed units). We

derive an approximated solution to the differential equations that define the SIR model. Unlike the exact

SIR solution, the approximate solution is analytical and has a closed form expression. We use this

approximate model as an inspiration to cyber defence. Such a model allows us to investigate the

characteristics of the propagation of electronic viruses. That is, we can determine the number of susceptible

units, the number of infected units and the number of removed units as a function of time. This information

will eventually permit the defence to find ways to eradicate a virus attack and to show how viruses affect the

defence effectiveness.

1 INTRODUCTION

“Infectious diseases have been a part of the human

condition since time immemorial” (Smith? 2008a).

Note the “?” is part of the last name “Smith?”.

Nowadays, we also encounter electronic viruses

which can attack computers and networks. The

nature of data communication allows electronic

viruses to propagate data rates ranging from kilobits

per second to gigabits per second. Hence a network

could be infected in a matter of minutes. To prepare

defence against viruses, we need to be able to model

the process of infection. Our inspiration is owed to

the modelling of epidemiology.

“Mathematical epidemiology has its roots in

1760, when Daniel Bernoulli formulated and solved

a model for smallpox. In 1906, Hamer used a

discrete-time model of measles to understand

recurrent epidemics” (Smith? 2008b). Clearly, there

is an available body of knowledge in the

mathematics of infectious diseases.

We encounter computer viruses every day and in

every field of work. There are lots of speculations on

the potential damages of a cyber-attack. Below is a

list of examples.

a. A car’s accelerator can be disabled (Greenberg,

2016a);

b. A car can unintentionally accelerate, brake or

steer (Greenberg, 2016b);

c. A sniper rifle can be deactivated or change its

target (Greenberg 2016c);

d. The fact that North Korea’s missile launches

were failing too often may be due to US cyber-

attacks (Sanger 2017).

Some of the above examples may be real and

some of them may not be accurate. But whatever

their veracities are, cyber defence is real. It was even

mentioned in the presidential debate between Hilary

Clinton and Donald Trump (Blake 2016). It is not

hard to imagine what would happen if a defence

system is infected. The impact could range from

minor nuisances to catastrophic failures. For

example, the defence system can fire in the wrong

direction, at the wrong target and at the wrong time.

The economic impact of crimes in cyberspace is

also speculated. Below are two examples.

a. The cost of crimes in cyberspace is estimated

to be 445 billion USD (World Economic

Forum 2016) and

b. US, China and Germany, three of the four

largest economies in the world, lost more than

200 billion USD (Centre for Strategic and

International Studies 2016).

In addition to the extent of a cyber-attack, it is

common knowledge that such an attack does not

necessarily require a lot of resources as cited from

(Kesan and Hayes 2012) below:

“Cyberattacks are not resource-intensive, which

renders them even more dangerous because no

practical requirement exists to limit the attackers to

being members of organized and well-funded

sources such as a nation’s military.”

232

Nguyen, B.

Modelling Cyber Vulnerability using Epidemic Models.

DOI: 10.5220/0006401902320239

In Proceedings of the 7th International Conference on Simulation and Modeling Methodologies, Technologies and Applications (SIMULTECH 2017), pages 232-239

ISBN: 978-989-758-265-3; ISSN: 2184-2841

 2023 by His Majesty the King in Right of Canada as represented by the Minister of National Defence and SCITEPRESS – Science and Technology Publications, Lda. Under

CC license (CC BY-NC-ND 4.0)

This is also recognized officially by NATO as

cited from (NATO fact sheet, 2016) below:

“Cyber threats and attacks are becoming more

common, sophisticated and damaging. The Alliance

(NATO) is faced with an evolving complex threat

environment. State and non-state actors can use

cyber-attacks in the context of military operations.”

Given the frequency and extent of cyberattacks,

we investigate the infection of viruses on a network

using an epidemic model. It is certainly not the first

time that cybersecurity is modelled by epidemiology

(Krishnan et al. 2013). There are several such

models. To name a few: the SEIR model

(Susceptible-Exposed-Infectious-Recovered), the

SIR model (Susceptible-Infectious-Recovered), the

SI model (Susceptible-Infectious) and the SIS model

(Susceptible-Infectious-Susceptible) (Keeling and

Rohani 2007). Each model is named after the

sequence of phases an entity is in when infected by a

virus.

The difference between the first two, the SEIR

model and the SIR model, is that the former

simulates the exposed phase where an individual can

be infected but is not infectious. It is often possible

to remove the exposed phase from the model which

leads to the SIR model (Keeling and Rohani 2007)

where an individual can be susceptible, infected or

recovered. This can be done when the population

scale is small meaning that every individual can be

infected in a short time. Susceptible units are those

that can be infected. Infected units are those that can

infect other units. And Removed units are those that

are no longer infected (recovered units).

In contrast to the SIR model, the SI model does

not account for the recovered phase. The SI model is

usually appropriate for plants. Once the plants are

infectious, they will remain infectious and

eventually die (Keeling and Rohani 2007). The

remaining model i.e. the SIS model is appropriate

for sexually transmitted diseases. Once an individual

recovers, he/she is again susceptible to infection

(Keeling and Rohani 2007). This could be applicable

for computer viruses as well. However, for a short

time scale, we assume that the defence will not be

attacked by the same virus or that once the virus is

known; the defence will recognize its signatures and

will stop the known virus before any infections

occur.

Based on the nature of the cyber defence

scenarios that we consider: suitability of the level of

details, rapid dissemination of the infection (time

scale is short) (Hethcote 2000) and the fact that a

recovered unit is not susceptible to infection once

the virus is known and there is a software that can

neutralize the virus, we choose to examine the SIR

model as a cyber defence model.

Similar to most of the epidemic models, the SIR

model does not have an analytical solution. Hence,

it only has numerical solutions which make it

inconvenient (but not impossible) to analyze and to

predict the extent of the infection. However, we

were able to find an approximate solution that is

analytical. And we will show in future work that the

approximated SIR model is useful in planning

against cyberattacks. (Morris-King and Cam 2015)

also makes use of the SIR model to examine cyber

vulnerabilities but from an agent based simulation

perspective.

Section 2 presents the SIR model. Section 3

derives an approximated differential equation to the

SIR model. Section 4 derives an approximated

solution which is a solution to the approximated

differential equation. Section 5 analyses the results.

Section 6 provides the characteristics of the

approximated solution. We conclude in Section 7.

Before we delve into the details of the report, we

state below the assumptions:

a. It is possible for a red force to hack into the

defence system and put a virus in the defence

system;

b. The defence is partially disabled if not

completely during the infection;

c. The nature of computer viruses can be

simulated by biological epidemic models and

d. Further studies/experiments can determine the

parameters of the epidemic models.

Note that the epidemic models described above are

simple and deterministic. There are also stochastic

models (Bailey 1975) but they are even more

complicated mathematically and are not necessarily

better for our purpose than the SIR model. In fact,

there are a multitude of computer viruses such as

benevolent viruses, file infectors, macro viruses, etc.

(Horton and Seberry 1997). Each of them behaves

differently. It would be impossible to model all of

them.

We ultimately aim to determine the effects of a

cyber-attack on the effectiveness of the defence and

not the details of the infection in the sense that we

are looking for orders of magnitudes for the number

of susceptible units, the number of infected units and

the number of removed units as well as the duration

of the infection. In essence, if there is a virus in the

system and if there is a remedy to that virus and both

of them can be modelled or bound by the parameters

in the SIR model then the solution to the SIR model

can be useful to the planning of cyber defence. This

solution will enable the comparison of the efficiency

Modelling Cyber Vulnerability using Epidemic Models

233

between cyber defence software against known

viruses. Knowing the magnitudes of the duration of

the infection and the magnitudes of the number of

components that are affected will help determine the

changes in defence effectiveness. This is critical

especially against an astute enemy who could launch

a missile attack at the same time as a cyber-attack.

It is not hard to imagine how things can go wrong to

a net centric defence when the command and control

is infected even if for a short time. Key measures of

effectiveness in such a missile defence scenario will

definitely be affected and will likely show losses in

effectiveness.

2 SIR MODEL OF EPIDEMICS

The SIR model is well understood, (Smith? 2008c).

It is assumed in the SIR model that there is

homogeneous mixing within the population. This

could happen if any unit is in contact with all other

units. This interpretation can be seen when we

consider a finite population for example four units in

which one of them is infected. If the infection rate is

the same for all susceptible units then all units must

be in contact with all other units. Otherwise, by

changing the initial infected unit to another unit, we

will not have the same infection rate. This

corresponds to a complete graph (Bondy and Marty

2008) which is a graph where every node is linked to

all other nodes. In other words, this is a totally

connected network. Clearly, the spread of a virus

depends on the topology of the network (Ganesh et

al. 2005 and Chakrabarti et al. 2008). That is,

infections could occur only if an infected node is

connected to another node. Therefore, we can

consider the SIR model as the worst case scenario

i.e. an infected node can infect any other nodes. We

could also think of the SIR model as an attack at the

central node which is connected to all of the other

nodes or any susceptible unit is in contact with other

infected units in a way that each susceptible unit has

an identical rate of infection. It is defined by a set of

differential equations as shown below:

S aSI

aSI bI

RbI

=−

(1)

where

S is the number of units that are susceptible

to infections,

is the number of units infected and

R is the number of units removed from infection

i.e. they are no longer infected;

is the rate of

infection and

b is the rate of recovery.

NSIR=++ is a constant. That is, the total

population is fixed. We scale

/, / /SSNIINandRRN←← ←. Hence,

0,, 1SIR≤≤ and 1SIR++ =. In the context of

computer viruses,

S is the number of susceptible

units,

is the number of infected units and R is

the number of removed (recovered) units.

Figure 1: An SIR model.

In spite of the simplicity of Equations (1), there

are no known analytical solutions. However, we

could infer from Equations (1) that there are two

equilibrium points where the RHS of Equations (1)

are equal to zeroes. The first equilibrium point

occurs when

0II==, SSN=≤ and

RRNS==−. The second equilibrium occurs

when

0aS b−=

/SSba== which implies

that

'0I = which makes

IN=≤ but S is

decreasing due to

/dS dt

. Therefore it is not a

stable equilibrium.

S is the initial value of

at time zero and

/Sba> then there will be an epidemic as

'0I >

The method of determining the equilibrium points

for ordinary differential equations is well

understood. It makes use of the Jacobian matrix and

its eigenvalues (Smith? 2008d). An equilibrium

point is stable if all eigenvalues are negative (or

zero).

3 APPROXIMATED

DIFFERENTIAL EQUATIONS

TO THE SIR EPIDEMIC

MODEL

We note that from Equations (1),

is uniquely

determined by

. So we focus on

and

because

once we solve for

and

, we can readily solve

for

. The first two equations of Equation (1) can

be combined to give:

''ISbI=− − (2)

SIMULTECH 2017 - 7th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

234

We define

() ()

ft Itdt=≥



(3)

Integrating Equation (2), we get:

SIbfC=− − ⋅ + (4)

where

C is a constant of integration. Since

()

'/ ln 'SS S aI= =− (5)

We get

SfbfCAe

−

=− − + = (6)

where

is a constant parameter. If we assume that

there is

infection at time zero and there are no

removed units then these are the boundary

conditions:

() ()

()

fItdt

fI I



(7)

This means that

(8)

Hence,

bf S e

−

=− −

(9)

There are two roots to the RHS of Equation (9):

ff W e

ba b

ff W e

ba b

−

==+ −

−

==+













(10)

where

W is the Lambert function. The Lambert

function is shown in Figure 2. For real

, there are

two branches. The first branch is shown in blue and

corresponds to

()

0,Wx

while the second branch is

shown in yellow and corresponds to

()

1,Wx−

Since the arguments of

()

for

and

are

negative, we can infer that the

embedded in

and

are also negative based on Figure 2. Simple

calculus dictates that

Sue S e

−

−≥−

where

/uab=

. From Equations (1), there are two cases.

First, if

()

1abu<<

then the number of infected

units will decrease right away. That is, the infection

will die out with time. Second, if

()

1abu≥≥

then

the number of infected units will increase at least at

time zero. Therefore, we will focus on the second

case because the virus will infect the system which

is the scenario that we are interested in. Since

1S ≤ , we reason that:

()

0, 1

WSue

−

−=−≥− (11)

Hence

ff W e

ba b

abu

ab bu

λλ

−

==+

−−

==≥







(12)

From (Higham et al. 2015), the second order

approximation of

()

1,Wx−

is given by:

()

1/1

1, 1

We z

−−

−− =−− (13)

Equating

1/1

eSue

−− −

= (14)

We obtain:

()

2ln ln 1zSuu=− + −+ (15)

1S  then by using a Taylor expansion, we get

()

zu Ou

−

=−+ + − (16)

As a result

()

1/1

1, 1 / 3

We uu

−−

−− −− − (17)

0 1 2 3 4

Lambert function

Figure 2: Lambert function.

Modelling Cyber Vulnerability using Epidemic Models

235

Hence

()

ff W e

ba b

−

==+ −

−

=− <







(18)

The above holds in general for

01S<≤. We

observe that the RHS of Equation (9) is concave.

That is,

() ()()

RHS RHS x RHS y

≥+







(19)

Equivalently,

()

()(){}

()

/2 /2

ax ay

bSe

bx S e by S e

eee

ee e

−

−−

−

−−

−

−−

≥−− +−−

−≥− +

≤− +

≤−







(20)

Because the RHS of Equation (9) is concave, we

approximate it by a quadratic function. That is,

()()

bfe cff ff

−

−− − −

(21)

where

and

are given by Equations (10).

Additionally, we determine

c by minimizing the

i.e.

()()

()

min

cf f f f

bf e

−⋅

⋅−⋅− −

⋅

−⋅ − +









(22)

which is the same as

()()

()

()()

()

()()

cf f f f

bf S e

cf f f f

df ff ff

bf S e

−

−−

−− − +

−−

−−=

−− − +















()()

()

cf f f f

df ff ff

bf S e

−

−−

−− − =

−− +

















(23)

This yields:

()()

()

()()

{}

ff ff

bf S e

df f f f f

−

−−

−− +

−−













(24)

There is actually a closed form expression for

c .

It can be obtained by performing the integrals in the

numerator and in the denominator above. However,

it is not particularly illuminating so we keep

Equation (24) the way it is. Observe that the

integrals in Equation (24) are integrated from

0f =

0ff=> since we know that

()

0ft≥ as

shown in Equation (3). By doing so, we discard all

negative values of

which are not physical values.

That is, the value of

is not affected by the value of

when

is negative.

We plot the exact

/df dt

in Equation (9) and the

quadratic function in Equation (21) that

approximates

/df dt

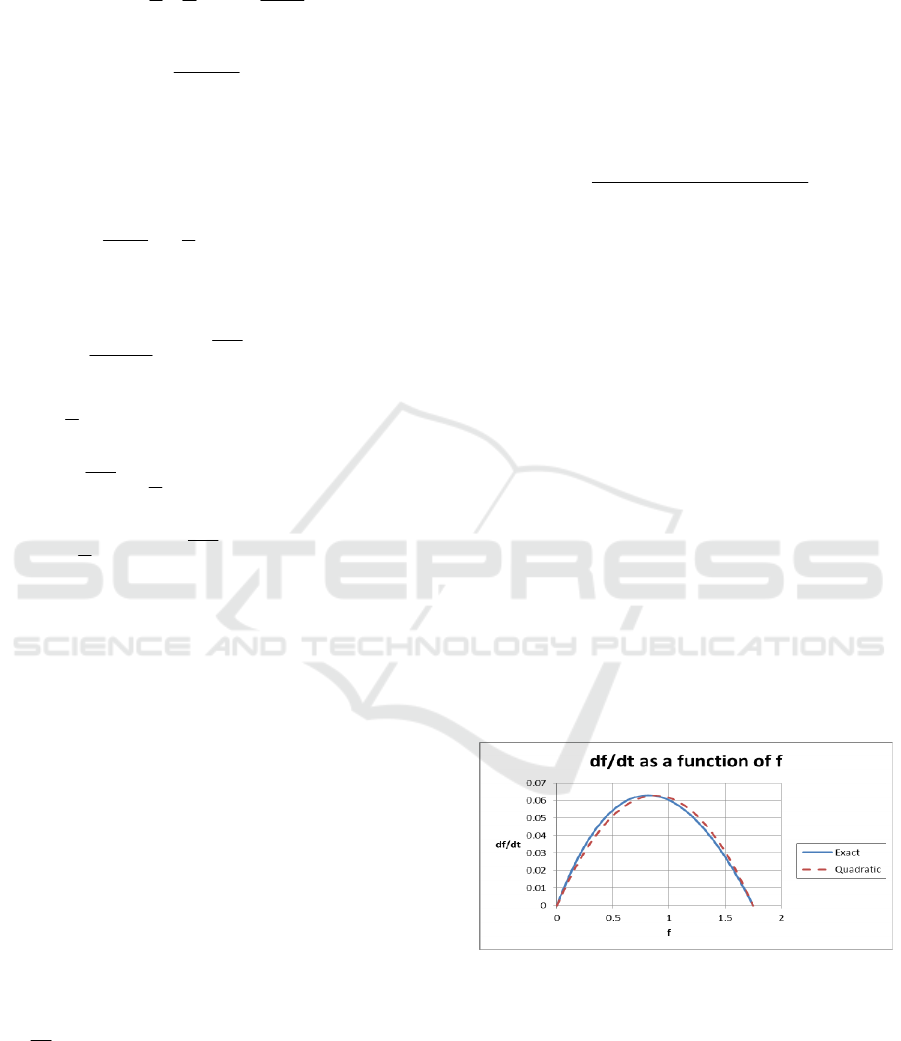
in Figure 3. It can be seen that

the approximation is very similar to the exact

/df dt

. Both of them are concave functions with a

maximum between

and

Figure 3: Derivative of

For illustration, we assume the following values in

Figure 3:

1/ 2, 1/3

0.99999

5.99991 10 1.74847

−

=− ⋅ =

(25)

SIMULTECH 2017 - 7th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

236

4 APPROXIMATED SOLUTION

TO THE SIR EPIDEMIC

MODEL

We now solve for

()

t using the quadratic

approximation:

()()

cf f f f−−= (26)

This is a simple differential equation that can be

solved using:

()()

cf f f f

−−

(27)

(Gradshteyn and Ryzhik 1979) integrating:

−







(28)

where

C is a constant parameter and

()

0cf fΔ= − > assuming that

0c <

0f <

and

0f > . Raising Equation (28) as a power of an

exponential, we get:

()()

ff f f Ae

−−=⋅ (29)

where

is a constant parameter. Since

()

00f =

this yields:

=−

(30)

Solving for

f :

()

−+

(31)

We can now obtain

()

t :

() ()

()

12 1 2

cf f e f f

It f t

ffe

−

(32)

From Equation (5) and the boundary conditions in

Equations (7), we get an expression for

()

St:

()

af t

St Se

−

= (33)

From Equation (1) and the boundary conditions in

Equations (7), we get an expression for

()

Rt:

() ()

Rt bf t= (34)

To investigate the long term effects of the system,

we evaluate the SIR as time tends to infinity.

()

12 1 2

lim lim 0

cf f e f f

ffe

→∞ →∞

−

(35)

()

21 2

lim lim

fe af

S t Se Se

−+

−

−+ −

→∞ →∞

== (36)

()

lim lim

Rt b bf

ffe

→∞ →∞

−+

(37)

5 RESULTS

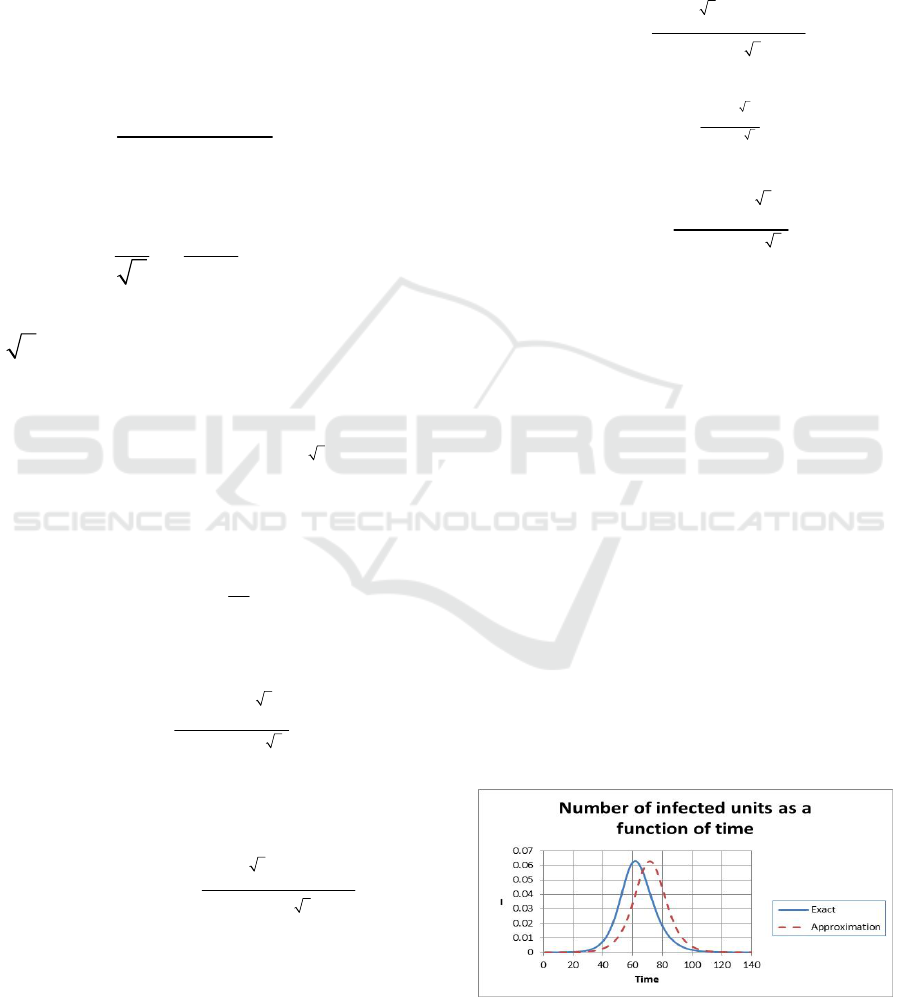
We plot

as a function t in Figure 4.

increases

as a function of time then reaches a maximum and

then decreases as a function of time. The blue curve

corresponds to the exact solution obtained

numerically while the red curve corresponds to the

approximated solution. The two have the same

shape and the same asymptotic behaviours as time

gets large. In addition, the approximated solution is

slightly shifted to the right. The maximum number

of infected units is about

6.2 percent of the

population as

is normalized. The input parameters

are shown in Equation (25). Note that we did not

give a unit for the time as we do not know the

coupling parameters a and

b . Once we obtain the

values for the coupling parameters, we will be able

to extract the unit of time. This will be done in the

future.

Figure 4: Number of infected units as a function of time.

Modelling Cyber Vulnerability using Epidemic Models

237

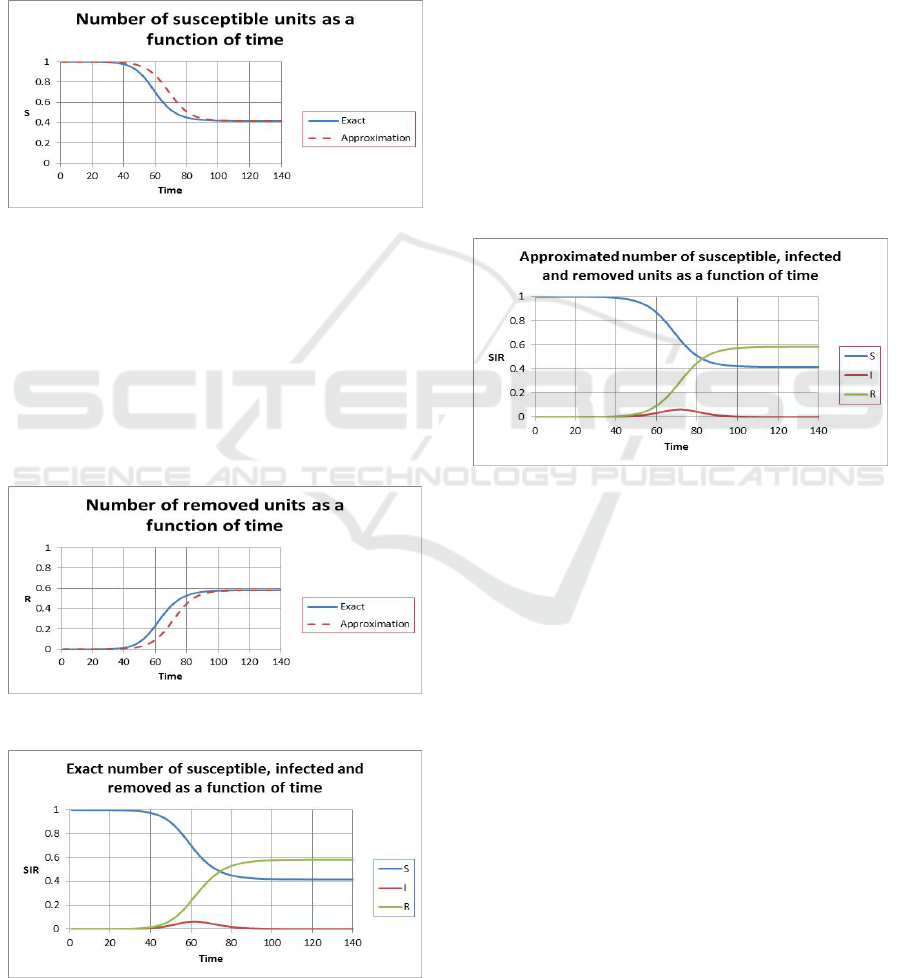
Similarly, we plot

S as a function of t in Figure 5.

It is a decreasing function of time. The blue curve

corresponds to the exact solution while the red curve

corresponds to the approximated solution. The two

have the same shape and the same asymptotic

behaviours as time gets large. That is,

S reaches a

constant value that is non-zero for large time. In

addition, the approximated solution is slightly

shifted to the right.

Figure 5: Number of susceptible units as a function of

time.

The same behaviours occur when we plot R as

a function of

t as shown in Figure 6. It is an

increasing function of time and reaches a non-zero

value as time gets large. We plot the SIR units as a

function of time for the exact model in Figure 7 and

for the approximate model in Figure 8. As time gets

large, the SIR units in both cases reach steady

values.

Figure 6: Number of removed units as a function of time.

Figure 7: Number of susceptible, infected and removed

units as a function of time.

6 CONCLUSIONS

In this paper, we have derived an approximated SIR

model and found the corresponding analytical

solution. We could consider the approximated SIR

model itself a SIR model. After all, the exact SIR

model is a man-made model where the couplings

among the susceptible units, the infected units and

the removed units are parts of the modelling.

Unlike the exact SIR model and in spite of its

simplicity, the analytical nature of the approximate

solution allows one to determine the long term

characteristics of the SIR units, the maximum

number of infected units and the time when this

occurs with only three parameters

,,cf f and the

boundary conditions.

,,cf f are obtained from the

couplings

,ab

of the exact SIR model and the

boundary conditions.

Figure 8: Number of susceptible, infected and removed

units as a function of time.

This allows us to plan for cyber-attacks.

Knowing

,,cf f, we can determine the extent of

the damage i.e. the number of infected units, the

number of susceptible units and the number of

removed units as functions of time. These numbers

are illustrated in Figure 4, Figure 5 and Figure 6

respectively. They show how long the system takes

to recover e.g. when the number of infected units

reaches a minimum acceptable value after attaining a

maximum value. If it takes a long time relative to

the time scale of a simultaneous missile attack then

clearly the defence may not be effective especially if

critical defence systems are infected and the defence

loses its net centric capabilities for example. What is

more, if the number of infected units keeps

increasing with time then we know that our cyber

defence is absolutely not effective. These

qualitative features and their orders of magnitudes

will be useful to the defence planning.

SIMULTECH 2017 - 7th International Conference on Simulation and Modeling Methodologies, Technologies and Applications

238

A contribution to this paper is the simplicity of

the approximated and analytical solution. We require

only the three parameters of a quadratic

function

,,cf fto model a generic virus infection

and its remedy.

Our next step is to conduct experiments and/or

investigations to determine these parameters that are

specific to the scenario. To do that, we would also

need to consider the number of platforms, the

number of computers, the network topology, etc.

ACKNOWLEDGEMENTS

I would like to thank Prof. Suruz Miah of Bradley

University and Dr. Kevin Ng of Defence R&D

Canada – Centre for Operational Research and

Analysis (DRDC CORA) for discussions. The

content of this paper comes from an internal

document of DRDC.

REFERENCES

Bailey, Norman T. J., 1975, The mathematical theory of

infectious diseases and its applications, Charles Griffin

& Company LTD, 2nd edition, pp. 39-42.

Blake A., The first Trump-Clinton presidential debate

transcript, the Washington Post 26 Sep 2016 (online),

https://www.washingtonpost.com/news/the-fix/wp/

2016/09/26/the-first-trump-clinton-presidential-deba

te-transcript-annotated/ (Access date: 27 Oct. 2016)

Bondy J. A. and Marty U. S. R., 2008, Graph theory.

Springer, p. 4.

Chakrabarti D., Wang Y., Wang C., Leskovec J., and

Faloutsos C., 2008. Epidemic thresholds in real

networks, Association for Computing Machinery

Transaction Information System Security. 10:4,pp.1–26.

Ganesh A., Massoulie L., and Towsley D., 2005, The

effect of network topology on the spread of epidemics,

Proceedings of IEEE Infocom.

Gradshteyn, I. S., and I. M. Ryzhik, 1979. Tables of

integrals, series, and products, 6th ed. Academic Press,

San Diego, CA. 6th Ed., p. 1100.

Greenberg, A., 2016a. Hackers remotely kill a Jeep on the

highway – with me in it, https://www.wired.com/2015/

07/hackers-remotely-kill-jeep-highway/ (Access date:

26 Oct. 2016).

Greenberg, A., 2016b. The Jeep hackers are back to prove

car hacking can get much worse, Andy Greenberg,

https://www.wired.com/2016/08/jeep-hackers-return-

high-speed-steering-acceleration-hacks/ (Access date:

26 Oct. 2016).

Greenberg, A., 2016c. Hackers can disable a sniper rifle or

change its target, Andy Greenberg, https://www.

wired.com/2015/07/hackers-can-disable-sniper-rifleor-

change-target/ (Access date: 26 Oct 2016).

Hethcote, H., 2000. The mathematics of infectious

diseases, SIAM Review, Vol. 42, No. 4, pp. 599-653.

Higham, N. J. etal, 2015. The Princeton companion to

applied mathematics, Princeton University Press, pp.

151-154.

Horton J and Seberry J, 1997, Computer Viruses: an

Introduction, Proceedings of the Twentieth

Australasian Computer Science Conference eb. 1997, -

Aust. Computer Science Communications, Vol. 19,

No. 1, pp. 122-131.

Keeling Matt J. and Rohani, P., 2007. Modeling Infectious

Diseases in Humans and Animals, Princeton

University Press, p. 4.

Kesan J, and Hayes C., 2012. Mitigative counterstriking:

self-defense and deterrence in cyberspace, Harvard

Journal of Law and Technology (forthcoming,

available at SSRN: http://ssrn.com/abstract=1805163).

Krishnan G. S. S. et al., 2013. Computational intelligence,

cybersecurity and computational models: proceedings

of ICC3, Springer.

Morris-King, J. and Cam, H., 2015. Ecology-inspired

cyber risk model for propagation of vulnerability

exploitation in tactical edge, Proceedings of the IEEE

2015 Military Communications Conference

MILCOM'2015, pp. 336-341.

Sanger, D., 2017. A Eureka moment for two times

reporters: North Korea’s missile launches were failing

too often, the New York Times, Mar 06 2017.

Smith? R., 2008a. Modelling disease ecology with

mathematics, American Institute of Mathematical

Sciences, p 1.

Smith? R., 2008b. Modelling disease ecology with

mathematics, American Institute of Mathematical

Sciences, p 1.

Smith? R., 2008c. Modelling disease ecology with

mathematics, American Institute of Mathematical

Sciences, 2008, pp. 14-16.

Smith? R., 2008d. Modelling disease ecology with

mathematics, American Institute of Mathematical

Sciences, 2008, pp. 25-30.

Xu S., Lu W., and Li H., 2015. A stochastic model of

active cyber defense dynamics, Internet Mathematics

Vol. 11, pp. 28–75.

NATO fact sheet (online), 2016. http://www.nato.int/

nato_static_fl2014/assets/pdf/pdf_2016_07/20160627

_1607-factsheet-cyber-defence-eng.pdf (Access date:

26 Oct 2016)

Net losses: estimating the global cost of cybercrime,

Centre for strategic and international studies, Jun 2014

(online), http://www.mcafee.com/ca/resources/reports/

rp-economic-impact-cybercrime2.pdf (Access date: 27

Oct. 2016).

Norton Report (online), 2013. http://www.symantec.com/

region/reg_eu/resources/virus_cost.html (Access date:

26 Oct. 2016).

The global risks report 2016, 11th edition, World

Economic Forum (online), http://reports.weforum.org/

global-risks-2016/executive-summary/ (Access date:

26 Oct. 2016).

Modelling Cyber Vulnerability using Epidemic Models

239