
The Process of Intraconnection and Interconnection in Mathematical
Problem Solving based on Stages of Polya
N. Tasni
1,3
, T. Nusantara
1
, E. Hidayanto
1
, Sisworo
1
, E. Susanti
2
and Subanji
1
1
Pascasarjana Universitas Negeri Malang, JL. Semarang No. 5 Malang, Indonesia
2
Universitas Negeri Islam Maulana Malik Ibrahim Malang, JL. Gajayana No. 50 Malang, Indonesia
3
STKIP YPUP Makassar, JL. Andi Tonro No. 17Makassar, Indonesia
ellysusanti@mat.uin-malang.ac.id
Keywords: Problem Solving of Polya, Mathematical Connections, Connective Thinking, Complete Connective Thinking
Networks.
Abstract: One of the factors that inhibit the success of students in constructing the problem-solving process is that
students are not able to identify the type of mathematical connection that should be built in the problem-
solving process. Therefore, the purpose of this study is to discuss the types of mathematical connections that
occur in the stages and between stages of Polya. Identification of the type of connection in each stage and
between stages of solving the Polya problem is defined as intraconnection and mathematical interconnection.
The purposive sampling technique was used to select two students who had a tendency to productive
connective thinking with complete connective thinking networks. Worksheets and recordings of the three
students' thinking are analyzed using a qualitative descriptive approach. In the intraconnection process can be
described the formation of a network of understanding connections, hierarchical connections, connections if
so, equivalent representation connections, and procedural connections. Whereas in the interconnection
process there is the formation of network connection planning, syntax or plan execution, and connection
evaluation. The conclusion of the research results is the formation of five connection networks in the
intraconnection process and three connection networks in the interconnection process.
1 INTRODUCTION
The problem-solving process requires establishing a
connection between stages problem solving, as an
effort to find solutions based on knowledge owned
(Xenofontos & Andrews, 2014). The strategy of
finding solutions to problems scientifically involves
estimating, observing, analyzing information and
forming results (Hong & Diamond, 2012). This
strategy involves a problem-solving process that
simultaneously develops students' skills in high-level
thinking, one of which is to build mathematical
connections (Hou, 2011). Students who have the
tendency of productive connective thinking can
always generalize their ability to establish
mathematical connections at each stage of problem-
solving, especially the solving of Polya's problems.
But what inhibits students from being able to
construct Polya problem solving is the inability of
students to identify mathematical connections that
occur within and between each Polya stage.
Through identification of the mathematical
connection process of students who tend to produce
productive thinking in each stage or between stages
of problem-solving, Polya can know the ideas built by
students when linking mathematical concepts. So that
the results of this identification can be a reference for
teachers to overcome student difficulties in
establishing connections. Students can take
advantage of connections in problem-solving, so they
do not have to rely on their memory alone to
remember too many isolated concepts and procedures
when doing problem-solving processes (Hung & Lin,
2015). Students only need to know the relevant
concepts in mathematics that can be used in other
domains. To fulfill this goal students must have
knowledge about connections in each stage and
connections between stages in problem-solving
according to Polya. This indicates that it is very
important to identify the connection process that
occurs in the Polya problem-solving process.
328
Tasni, N., Nusantara, T., Hidayanto, E., Sisworo, ., Susanti, E. and Subanji, .
The Process of Intraconnection and Interconnection in Mathematical Problem Solving based on Stages of Polya.
DOI: 10.5220/0008521503280335
In Proceedings of the International Conference on Mathematics and Islam (ICMIs 2018), pages 328-335
ISBN: 978-989-758-407-7
Copyright
c
2020 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved

Exploring the connection process that occurs in
each stage and between stages of solving Polya's
problem, is expected to lead to a positive attitude
towards mathematics so that the students' awareness
and thinking will be more open to mathematics, not
only focused on the particular material being studied
(Hendriana, Slamet, & Sumarmo, 2014) Identifying
the type of connection in stages and between stages
of solving Polya's problem, is expected to help
students find the right strategy in solving
mathematical problems, especially the application of
mathematics in everyday life. At the same time
improve mathematical connection capabilities, so that
it can be used in the development and improvement
of mathematics learning processes. The practical
implications of the results of this study are expected
to add to the scientific repertoire, especially the
application of mathematical connections in solving
mathematical application problems in everyday life
so that in the end the essence of the mathematics
learning objectives can be achieved.
Previous studies only looked at the mathematical
connections that occurred in the general problem-
solving process, either through assignments or
through the learning process. (Eli, Mohr-Schroeder,
& Lee, 2013; Hendriana et al., 2014; L. Suominen,
2015; Mhlolo, Venkat, & Schfer, 2012a; Stylianou,
2013) identification of connections in verbal problem
solving has been done but has not been described in
detail the types of connections that occur in the stages
and between stages of solving the problem Polya. So
that this paper is directed to describe how connections
are in the problem-solving process of students who
tend to have productive connective thinking or have
complete connective thinking networks? This study
aims to describe the specific connection that occurs in
the Polya problem-solving process for students who
tend to productive connective thinking or have a
complete network of connective thinking. Includes
connections at each stage, as well as connections
between stages in Polya's steps which are categorized
as mathematical interconnections and
intraconnections.
2 REVIEW LITERATURE
Mathematical connections as a relation of several
concepts or ideas, whether the relationship of
concepts or ideas in mathematics and between one
mathematical unity with other disciplines (Jaijan,
2012; Ozturk & Guven, 2016) Therefore
mathematical connections should enable students to
(1) recognize and use connections between
mathematical ideas, (2) understand how
mathematical ideas are interconnected and construct
one another, (3) recognize and apply mathematics in
an outside context mathematics. (Hsu & Silver,
2014).
(Businskas, 2008) which explains the types of
mathematical connections is a process that occurs in
the minds of learners. Earlier (Hiebert, J., &
Carpenter, n.d, 1999) explained that structured
networks such as spider webs, where points or
vertices can be considered as the pieces of
information represented, and the series between them
as connections. This indicates that new knowledge is
built on existing knowledge, or a mathematical
connection must be established between pre-existing
schemes so that unknown mathematical ideas can be
understood by the learner. A connection exists in
every part of mathematics and the learners must
engage in building activities or identifying such
connections and recognizing the coherent nature of
mathematics that includes: multiple representations,
problem solving, verification, modeling and
application of mathematics in the real world (Hsu &
Silver, 2014)
The mathematical connection is one of the
standard curriculum of elementary and middle school
mathematics learning (Hendriana et al., 2014). In
order to make the process of solving the problem,
must first understand the problem and to be able to
understand the problems must be able to make
connections with related topics. Bruner(Permana &
Sumarmo, 2007) states that there is no concept or
operation in mathematics that is not connected with
other concepts or operations in a system, because of a
fact that the essence of mathematics is something that
is always associated with something else. This
indicates that when students connect mathematical
ideas, their understanding is deeper and more lasting,
and they will see mathematics as a whole (Hsu &
Silver, 2014)
In general, the connection is the relationship
between ideas, concepts or procedures (Businskas,
2008). In the problem-solving process, students will
connect ideas, concepts, or procedures to understand
problems, plan strategies, resolve problems as
planned, and re-examine the results obtained. In these
four stages, students will certainly engage in
mathematical activities that require them to build
connections between existing knowledge and new
ideas that not known. Therefore, the mathematical
connections in this study will be observed from two
perspectives i.e. connections that occur in every stage
of Polya, and the connections that occur between
these stages.
The Process of Intraconnection and Interconnection in Mathematical Problem Solving based on Stages of Polya
329

In this research, will be observed connection
process that happened in problem solving step
according to Polya namely:
2.1 Understanding the Problem
The first step is to understand the problem, the student
may not be able to solve the problem correctly, if not
understand the problem given. Students should be
able to show the parts of the principle of the problem,
the question, the known, the prerequisites.
2.2 Planning a Solution
This second step relies heavily on student experience
in solving problems. In general, the more varied their
experiences are, the more creative the students tend to
be in preparing a problem-solving plan.
Understanding the problem for a solving plan may be
long and tortuous. The ultimate success of solving
problems is the idea of a plan. This idea may appear
gradually, or after a failed experiment and doubt may
occur suddenly, as a "brilliant idea". A good idea can
be based on previous experience or knowledge.
2.3 Solving Problems to Plan
To think about a plan, understanding the idea of
completion is not easy. The teacher should ask firmly
to the student to check each step, by asking Are you
sure that step is right?
2.4 Checking Back Results Obtained
A good student, when he or she has got a problem
solving and written down an answer neatly, he will
check again the results obtained. Teachers can ask
students with questions: Can you check the results?
Can you check the argument? To provide challenges
and satisfaction in solving problems ask Can you get
results in different ways?
The process of thinking examined in this study
relates to the association of ideas that arise when
establishing mathematical connections in the process
of solving Polya problems. (Holyoak, K.J and
Morisson, 2012) explains that building a
mathematical connection involves three cognitive
processes, namely building new ideas from previous
ideas, building relationships among topics in
mathematics itself, and applying mathematical ideas
to other sciences or everyday life. (Susanti, 2015)
states that connective thinking is a process of thinking
in making the association between mathematical
ideas when connecting mathematical concepts.
Furthermore, Susanti classifies connective thinking
into 3 categorization which is simple connective
thinking, semi-productive connective thinking, and
productive connective thinking.
In this study will be focused on the type of
connection that is formed on the network of complete
or productive thinking in the solving of Polya
problem solving. Thinking productively connective is
the ability to think in building many connections from
relevant ideas which arose based on the information
provided, then formed a generalization to conclude
the general rule until the formation of a knowledge
reconstruction. (Susanti, 2015). Therefore, in this
research, will be identified connection process that
occurs in complete connective thinking network or
productive in Polya problem solving process. The
connection process that occurs will be identified in
the intraconnection and interconnection process in
solving Polya problem.
Some research related to establishing
mathematical connections in problem solving has
been widely practiced. Stylianou., D, 2013 examines
the mathematical connections in the troubleshooting
process of high school students. In his research, he
describes the connection between the justification
process and the representation. Jaijan & Loipha, 2012
establishes a mathematical connection with an open-
ended transformation, which is through open-ended
problem solving. Next (Angeli & Valanides, 2012)
looks at how connections of epistemological beliefs
and student reasoning when thinking about problem-
solving ill-structure. Open-ended or ill-structured
problems often arise in real-world situations.
However, students' awareness to use mathematical
connections in problem solving, in particular, solves
the problem of mathematical applications in the world
real low (Baki, Çatlioǧlu, Coştu, & Birgin, 2009)
3 METHOD
The purposive sampling technique is used to select
two students to research students. The two students of
the FA and the AM were selected based on the results
of a written test conducted by thinking a load and
semi-structured interviews. Students with a network
of productively connective thinking are selected to
obtain a complete picture of the connection process
that occurs in each stage and between stages in
solving Polya problems. The work and recording of
think aloud of the two students were analyzed by the
qualitative descriptive approach. The semi-structured
interview process was conducted to deepen the
analysis of the connection process that occurred at the
ICMIs 2018 - International Conference on Mathematics and Islam
330

stage of understanding, planning, implementation of
the plan, and evaluation and connection process
between the four stages to obtain the conclusion of
the research results. The problem-solving sheet used
in collecting data is as follows:
“ Four students will take part in an innovative
work competition. For that, a fee of Rp. 900,000.00 is
required. Because each has a different financial
condition, the amount of each student's contribution
is not the same. Student A contributes half the
contribution of three other students. Student B
contributed one-third of the contributions of three
other students. Student C contributes a quarter of the
contribution of the other three students, calculate
how much contribution to student D”
4 RESULT AND DISCUSSION
Connections that occur in each stage and between the
stages in the problem-solving step according to Polya
in this study are categorized as intra-connection and
interconnection. The description of the intra-
connection and interconnection of the two students is
explained as follows: At the stage of understanding,
FA students demonstrate their ability to identify each
element that is known and asked if the problem given.
Every student needs a different time to understand the
problem given. This understanding arises after
students write down the elements that are known and
asked in the question. The process can be seen from
the results of transcripts of interviews with FA
students as follows:
R : What can you understand after reading the questions
given?
FA: here there are four students who take part in the
competition, namely students A, B, C, and D
R : What will be done by the four students?
FA : The four students will contribute to the
innovative work competition.
R : What are the contributions of the four students?
FA : Its contribution, namely student A contributed
half of the contribution of three other students,
student B contributed one third of the contribution of
three other students, student C contributed a quarter
of the contribution of three other students and the
total cost was 900,000
R : What is student D?
FA: what is asked in the question is the contribution
of student D?
Based on the results of the transcript of interviews
with FA students it was found that in order to
understand the problem, the FA students identified
the elements known and asked in the questions. The
FA is able to identify concepts that will be used as the
initial idea to develop a plan for solving the problem
given. The process indicates the ability of FA students
to connect each element known and asked through
connection understanding. (Tasni, Nurfaidah, 2017)
examines the barriers of productive connective
thinking of students in solving mathematical (Tasni &
Susanti, 2016)problems and finds that one of the
factors that inhibits students' ability to think
productively is the inability to establish complete
connections at the stage of understanding. However,
in the conditions shown by the FA students, he was
able to establish a complete connection at the
understanding stage, so he was able to plan better at
the planning stage. In the interconnection process
students make connections of understanding while if
observed in the intra-connection aspects students
make planning connections. explains that connection
understanding, that is, connections that are built based
on the ability of the subject to identify the elements
that are known and asked in the question, to find out
the concepts and procedures that will be used as a
settlement strategy. It is also explained by (Hsu &
Silver, 2014) that the ability of students to recognize
connections is directly related to mathematical
understanding.
At the planning stage, the first student FA reviews
what is known and asked in the question then attempts
to translate it into a mathematical equation. In this
process, FA students plan to use the concept of
comparison to formulate an equation that shows the
amount of money from each student. Next, the FA
students think to define the solution of the equation
using the two-variable linear equation system
concept, by selecting the elimination and substitution
methods to determine the value of each variable. In
the intra-connection process students make
hierarchical connections, (Tasni & Susanti, 2016)
explains that hierarchical connections namely
connections are built on a hierarchical relationship
between two concepts or one concept is a component
of another concept. This condition can be observed
when students use the concept of comparison to
formulate a mathematical model of the problem being
solved. Furthermore, students use procedural
connections by selecting the elimination and
substitution methods to determine the value of each
variable. As (Nakamura, 2014) explained that
knowledge can be built through the construction of
hierarchical concepts in mathematics.
The second student, AM, developed a more
mature settlement plan. In this case, AM students plan
to use the maximum number concept to form the
general equation form of the total costs that must be
The Process of Intraconnection and Interconnection in Mathematical Problem Solving based on Stages of Polya
331

spent by the four students based on the problem
given, then use the concept of comparison to damage
the mathematical equation of each statement in the
problem. In this condition AM students make
connections if it is, that is by building a connection to
the question questions that want a lot of contributions
from wrong attacks and the maximum amount of
costs needed to participate in innovative work
activities. As explained by (Mhlolo, Venkat, &
Schfer, 2012b) that doing mathematics with
reasoning, students must look at the eye or the
relationship between hypotheses and conclusions. To
simplify the form the equations that have been
compiled, AM Students use the concept of fractions.
The process is reflected in the work of AM students
as follows:
Figure 1: Work Results I of AM Student.
The following are the results of AM students' aloud
transcripts in designing problem solving.
“Here I use the concept of maximum number, the
concept of comparison and the concept of
fractions. And to compile the equation model I will
use the concept of comparison because the
equation is still in fraction, so I will simplify it
using the fraction concept "
Based on the results of AM student work and
fragments of interview transcripts, it was found that
students who used hierarchical relationships between
fraction and comparison concepts. Hierarchy
relationships occur when a concept is a component or
contained in another concept. This was identified by
the process carried out by AM students in compiling
mathematical equations using the concept of
comparison. This process is carried out by students to
avoid the fraction of equations arranged, without
changing the value of each equation. In this condition
AM students make equal representation connections.
In the previous study (Tasni & Susanti, 2016)
explained that Equivalent Connection
Representation, namely connections are built on
concepts that are represented in different ways and
forms but have the same value. In this study shows
the equality of verbal representation to symbolic. The
same thing was stated by (Businskas, 2008) that is in
the same form is an equivalent representation.
At the stage of implementation of the plan, FA
Students carry out the stages of implementing the
plan according to the draft arranged in the previous
stage. In the intra-connection process, FA students
make procedural connections. (Businskas, 2008)
explain that a concept can be a type of procedure or
method used to connect when working with other
concepts. Furthermore, in the interconnection
process, FA students make syntax connections or
implement plans. According to (Paper & Ribeiro,
2016) connection syntax is formed by using the basic
nature of a concept to construct a new concept used
in problem-solving.
The next completion step is that the FA student
determines the value of variable D which is the core
question of the question given. Based on the results
of his work in determining the value of each variable
and confirmation through the interview process can it
is known that FA students use one of the concepts that
has dependency logical to the other concepts. Or
show a relationship if then between the two concepts.
This was identified when FA students used logical
reasoning in the process determine the values of
variables A, B, C, and D. Where each equation is seen
as a premise, while the results or values of variables
are obtained from the process of elimination is a
logical conclusion. (Mhlolo et al., 2012b) explains
that the characteristics of the connection if it is when
students prove each guess and make conclusions
based on facts previously known.
In the Evaluation Stage. Every student has
different abilities in investigating the truth of the
problem solving that has been done. At the evaluation
stage the FA students focus on the question, is there
another procedure that can be used to obtain the same
answer. So that FA students believe that elimination
and substitution procedures are the only way to
determine the solution of each equation. FA students
assume that another method, in this case the
graphical method cannot be used because each
equation that is composed contains four variables that
cannot be described in dimension two. Therefore, FA
students choose the substitution method as another
procedure to verify the answers obtained. In the intra-
connection process FA students make equal
connection connections while simultaneously
evaluating connections on the interconnection
process. As explained in (Tasni & Susanti, 2016) that
connection is Justification and Representation, that is
a connection that is built when the subject evaluates
the truth of the answers obtained, with the concepts
ICMIs 2018 - International Conference on Mathematics and Islam
332
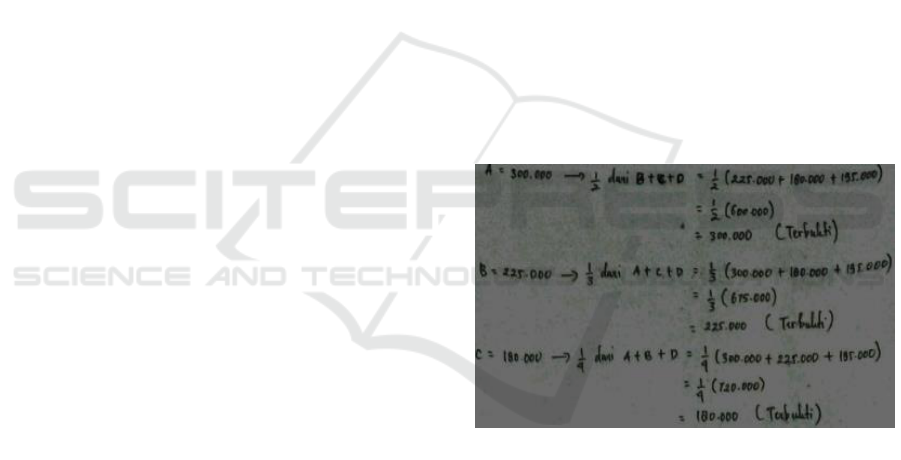
and procedures used. The process can be seen from
the FA students' think aloud transcripts as follows:
"To prove the truth of the answers I got, I will use a
different method, namely the substitution method."
Interconnection is the process of connection that
occurs between each stage of Polya. In the interview
process the FA and AM students showed a good
understanding of the questions given. This was
identified by their ability to write down the elements
known and asked in the questions. With their good
understanding, they are able to develop a problem
solving strategy, which is to develop a mathematical
model of the elements known in the problem.
(Mackrell & Pratt, 2017) explains that by having
adequate strategic knowledge, students will design
appropriate strategies to solve problems. Through the
understanding they have of the questions given, they
are also able to identify the concepts that will be used
In developing problem solving strategies. Among
other things, FA students plan use the concept of a
two-variable linear equation system to solve
problems and the concept of comparison to form a
mathematical model. (Plaxco & Wawro, 2015)
explains that understanding in linear algebra can
make it easier for students to do mathematical
solutions.
Both FA and AM students make planning
connections. Planning connection is a process of
interconnection that occurs from the understanding
stage to the planning stage, namely the idea of
completion that appears in the minds of students after
understanding the problem. This can be noted from its
ability to identify the concepts to be used and compile
resolution strategies. As shown in the FA student
think aloud transcripts as follows:
“I simplify each equation that is formed by using
the fraction concept, where to change the form of
fractions into integers, I multiply it by the same
number in the denominator, after the simple form
I use the elimination and substitution methods to
determine the value of each variable”
Based on the fragments of the interview transcript,
it can be said that there are interconnections carried
out by students from the planning stage to the
completion stage of the settlement. This is identified
by the ability of students to use the previously
mentioned concepts to simplify the model of equality
that has been compiled. In this case the researcher
identifies the interconnection process that occurs
from the planning stage to the implementation stage
is the connection implementation plan (syntax
connection). Connection implementation plan or
syntax occurs because there is a relationship between
the strategy designed and the implementation of the
strategy. By compiling a mature resolution strategy,
students will succeed in the process of implementing
the strategy in solving problems, as explained by
(Anthony & Walshaw, 2009) when students have the
strategic knowledge needed to correct existing
problems but, applying them ineffectively, will fail to
use the right strategy, the same thing is explained by
(Sulak, 2010) that students who are able to develop
sound strategies will succeed in solving problems.
Furthermore, the interconnection process that
occurs at the implementation stage of the plan to the
evaluation stage is also influenced by the stage of
understanding carried out by students. This can be
seen from the evaluation process carried out by AM
students. In order to be sure of the correctness of the
answers obtained, AM students re-match the values
of each variable obtained from the implementation
stage, with mathematical models arranged based on
the elements known in the problem. The process is
illustrated by the following work results of AM
students:
Figure 2: Work Results II of AM Student.
Based on the results of the AM student's work and
the interview process, it is known that AM students
connect evaluation. Evaluation connections are the
interconnections shown through the relationship
between representation and justification in the
problem-solving process. Students must have the
ability to use different methods in the evaluation
process to have the ability to solve problems (Esen &
Belgin, 2017). (Eli et al., 2013) explains that there is
a connection between representation and justification,
namely the ability of students to find connections
between the final results obtained with
representations based on the data obtained at the
understanding stage will lead students to obtain the
appropriate problem solutions.
The Process of Intraconnection and Interconnection in Mathematical Problem Solving based on Stages of Polya
333

5 CONCLUSIONS
The Intraconnection process that occurs in the Polya
problem-solving stage begins from the understanding
stage. Students demonstrate the ability to identify
elements that are known and asked in the matter,
these conditions are identified as understanding
connections. Furthermore, in the planning stage
students demonstrate the ability to build a hierarchical
relationship between two concepts or one of the
concepts that are components of another concept, this
condition is identified as a hierarchical connection. In
the implementation stage of the student plan shows
the ability to identify a concept that has a logical
dependency on another concept, this condition is
identified as a connection if then. In addition, at the
planning stage also identified procedural connection
occurred. Procedural connections are demonstrated
by students' ability to use a concept when working
with a particular method or procedure. Subsequent
connections that occur at the stage of the
implementation of the plan is equivalent
representational connections, this connection is
indicated by the ability of students to represent a
concept with a variety of relevant representations.
The interconnection process that occurs between
the Polya problem-solving steps is started from the
coherence built between the understanding stage and
the problem-solving planning stage. These
connections are identified as planning connections,
these connections are demonstrated through a
relationship of the understanding level to the maturity
of the completion strategy to be developed. Further
connections that occur between the stages of planning
and stages. The implementation of the plan i.e.
connection syntax. Syntax connection shows the
realization of the concept or procedure from the
planning stage to the implementation stage of the
plan. Further connections that arise between the
evaluation stage and the understanding stage of the
evaluation connection. The evaluation connection
shows the relationship of justification by checking the
conformity of the solution obtained with the
representation of mathematical models arranged
based on known elements at the understanding stage.
ACKNOWLEDGMENTS
The authors would like to express our biggest
gratitude to DP2M Dikti as research funder.
Furthermore, words are powerless to express our
gratitude to all civitas of UPT unit of education
district Bulukumba South Sulawesi which give the
research permit to conduct the research.
REFERENCES
Angeli, C., & Valanides, N. (2012). Epistemological beliefs
and Ill-structured Problem-solving in Solo and paired
contexts. Educational Technology and Society, 15(1),
2–14.
Anthony, G., & Walshaw, M. (2009). Characteristics of
effective teaching of mathematics: A view from the
West. … of Mathematics …, 2(2), 147–164.
https://doi.org/10.21831/JPE.V1I2.2633
Baki, A., Çatlioǧlu, H., Coştu, S., & Birgin, O. (2009).
Conceptions of high school students about
mathematical connections to the real-life. Procedia -
Social and Behavioral Sciences, 1(1), 1402–1407.
https://doi.org/10.1016/j.sbspro.2009.01.247
Businskas, A. M. (2008). CONVERSATIONS ABOUT
CONNECTIONS : How secondary mathematics
teachers conceptualize and contend with mathematical
connections by THESIS SUBMITTED IN PARTIAL
FULFILLMENT OF THE REQUIREMENTS FOR
THE DEGREE OF In the Faculty of Education.
Eli, J. A., Mohr-Schroeder, M. J., & Lee, C. W. (2013).
Mathematical Connections and Their Relationship to
Mathematics Knowledge for Teaching Geometry.
School Science and Mathematics, 113(3), 120–134.
https://doi.org/10.1111/ssm.12009
Esen, E., & Belgin, B. (2017). THE EVALUATION OF
THE PROBLEM SOLVING IN MATHEMATICS
COURSE ACCORDING TO, 01012.
https://doi.org/10.1051/itmconf/20171301012
Hendriana, H., Slamet, U. rahmat, & Sumarmo, U. (2014).
Mathematical connection ability and self-confidence
(an experiment on Hunior High School students
through Contextual Teaching and learning with
Mathematical Manipulative). International Journal of
Education, 8(1), 1–11. https://doi.org/10.17509/
IJE.V8I1.1726
Hiebert, J., & Carpenter, T. P. (1992). (n.d.). Learning and
teaching with understanding. In Handbook of research
on mathematics teaching and learning: A project of the
National Council of Teachers of Mathematics (pp. 65–
97). New York (NY): England: Macmillan Publishing
Co, Inc.
Holyoak, K. J and Morisson, R. (2012). Thinking and
Reasoning: A reader’s guide. New York (NY): Oxford
University Press.
Hong, S. Y., & Diamond, K. E. (2012). Two approaches to
teaching young children science concepts, vocabulary,
and scientific problem-solving skills. Early Childhood
Research Quarterly, 27(2), 295–305. https://doi.org/
10.1016/j.ecresq.2011.09.006
Hou, H. T. (2011). A case study of online instructional
collaborative discussion activities for problem-solving
using situated scenarios: An examination of content and
behavior cluster analysis. Computers and Education,
ICMIs 2018 - International Conference on Mathematics and Islam
334

56(3), 712–719. https://doi.org/10.1016/j.compedu.
2010.10.013
Hsu, H.-Y., & Silver, E. A. (2014). Cognitive complexity
of mathematics instructional tasks in a Taiwanese
classroom: An examination of task sources. Journal for
Research in Mathematics Education, 45(4), 460–496.
https://doi.org/10.5951/jresematheduc.45.4.0460
Hung, C.-H., & Lin, C.-Y. (2015). Using concept mapping
to evaluate knowledge structure in problem-based
learning. BMC Medical Education, 15(1), 212.
https://doi.org/10.1186/s12909-015-0496-x
Jaijan, W. (2012). Making Mathematical Connections with
Transformations Using Open Approach, 3(1), 91–100.
L. Suominen. (2015). Abstract Algebra and Secondary
School Mathematics: Identifying and Classifying
Mathematical Connections. The University of Georgia.
Mackrell, K., & Pratt, D. (2017). Constructionism and the
space of reasons. Mathematics Education Research
Journal, 29(4), 419–435. https://doi.org/10.1007/
s13394-017-0194-6
Mhlolo, M. K., Venkat, H., & Schfer, M. (2012a). The
nature and quality of the mathematical connections
teachers make. Pythagoras, 33(1). https://doi.org/10.
4102/pythagoras.v33i1.22
Mhlolo, M. K., Venkat, H., & Schfer, M. (2012b). The
nature and quality of the mathematical connections
teachers make. Pythagoras, 33(1), 1–9. https://doi.org/
10.4102/pythagoras.v33i1.22
Nakamura, A. (2014). Hierarchy Construction of
Mathematical Knowledge, 2(2), 203–207.
https://doi.org/10.12720/lnit.2.2.203-207
Ozturk, T., & Guven, B. (2016). Evaluating students’
beliefs in problem solving process: A case study.
Eurasia Journal of Mathematics, Science and
Technology Education, 12(3), 411–429.
https://doi.org/10.12973/eurasia.2016.1208a
Paper, C., & Ribeiro, M. (2016). Towards a Topology of
Mathematical Connections in Teacher Knowledge,
(August).
Permana, Y., & Sumarmo, U. (2007). Melalui
Pembelajaran Berbasis Masalah. Educationist, I(2),
116–123.
Plaxco, D., & Wawro, M. (2015). The Journal of
Mathematical Behavior Analyzing student
understanding in linear algebra through mathematical
activity. Journal of Mathematical Behavior, 38, 87–
100. https://doi.org/10.1016/j.jmathb.2015.03.002
Stylianou, D. A. (2013). An Examination of Connections in
Mathematical Processes in Students’ Problem Solving:
Connections between Representing and Justifying.
Journal of Education and Learning, 2(2), 23–35.
https://doi.org/10.5539/jel.v2n2p23
Sulak, S. (2010). Effect of problem-solving strategies on
problem solving achievement in primary school
mathematics, 9, 468–472. https://doi.org/10.1016/j.
sbspro.2010.12.182
Susanti, E. (2015). Proses Berpikir Siswa Dalam
Membangun Koneksi Ide-ide Matematis Pada
Pemecahan Masalah Matematika, (1), 2–3.
Tasni, Nurfaidah, et all. (2017). Obstacles to students’
productive connective thinking in solving mathematical
problems. JIP MIPA UPI, 22(2).
https://doi.org/http://dx.doi.org/10.18269/jpmipa.v22i2
.9100
Tasni, N., & Susanti, E. (2016). Membangun Koneksi
Matematis Siswa dalam Pemecahan Masalah Verbal.
Beta, 10(1), 103–116.
https://doi.org/http://dx.doi.org/10.20414/betajtm.v10i
1.108
Xenofontos, C., & Andrews, P. (2014). Defining
mathematical problems and problem solving:
Prospective primary teachers’ beliefs in Cyprus and
England. Mathematics Education Research Journal,
26(2), 279–299. https://doi.org/10.1007/s13394-013-
0098-z
The Process of Intraconnection and Interconnection in Mathematical Problem Solving based on Stages of Polya
335
