Crime-related Threats on Transportation Safety: A Mathematical

Model of Risk Assessment

I. G. Emel’yanov

1,2,3

and E. I. Dumanskaya

Ural State University of Railway Transport, Yekaterinburg, Russia

Ural Federal University, Yekaterinburg, Russia

Institute of Engineering Science, Russian Academy of Sciences (Ural Branch), Yekaterinburg, Russia

Ural State Law University, Yekaterinburg, Russia

Keywords: Criminal behavior, mathematical model, integral equation, crime risk assessment, criminological security in

transport.

Abstract: The article is devoted to the crime risks assessment as a possible option to counter threats to security in

transport. The model used is demonstrated on the example of a specific criminal case. The problem in question

developed mathematical model which describes the danger of a person. It is an attempt to solve an problem

by numerical methods – to made the model of individual criminal behavior. This makes it possible to prevent

recidivism of persons who have been sentenced to probation.

1 INTRODUCTION

The transport security system predetermines and

interconnects several security systems of a lower

rank, including criminological security.

Transport sector, including rail transport. provides

the basic conditions for the life of society, but crime

as a negative social and legal phenomenon is also an

integral part of public life. Socio-economic

transformations associated with the development of

technology entail the transformation of crime, and

this pattern is clearly manifested in the relationship

between crime and transport.

Accordingly, in modern realities, the task is to

increase the effectiveness of the impact on the sources

of threats to criminological security - crime in all its

manifestations, including the personality of the

offender and criminogenic factors. In this paper, the

authors focused on the study of the identity of the

offender as a carrier, the subject of this threat in order

to predict the risks of criminal behavior.

In the natural sciences, the task of researching

any phenomenon or process is usually reduced to

solving algebraic, differential, or other equations,

which contain a large amount of quantitative

information about the process under study. An

https://orcid.org/0000-0002-9733-5485

https://orcid.org/0000-0002-2066-1012

accurate description of the process under study

usually allows one to model the process for other

possible conditions as well.

Recently, mathematical models have become

widely used in various not only technical sciences,

but also in economics, medicine, biology, and in the

humanities. Much less publications using

mathematical models are found in legal sciences

(Srivastav, 2020; Syed, 2013). It seems that the use of

mathematical models and methods will help solve a

significant class of problems that arise in the social

system, in particular, in the field of criminological

prevention. In criminology, mathematical methods

for processing statistical information (Farrington,

2016) and correlation analysis began to be used to

assess the possibility of relapse. There are also

probabilistic predictive approaches to assessing the

risk of relapse (Skeem, 2016). It is known that

criminological studies reveal that some personality

attributes are associated with criminal behavior

(Miller, 2001).

Although the connection between past and future

criminal behavior has been found to some extent

(Farrington, 2003), in practical terms, predicting the

individual criminal behavior of a person is an

exceptionally difficult task. This is since human

272

Emelâ

Zyanov, I. and Dumanskaya, E.

Crime-related Threats on Transportation Safety: A Mathematical Model of Risk Assessment.

DOI: 10.5220/0011583700003527

In Proceedings of the 1st International Scientiﬁc and Practical Conference on Transport: Logistics, Construction, Maintenance, Management (TLC2M 2022), pages 272-277

ISBN: 978-989-758-606-4

 2023 by SCITEPRESS – Science and Technology Publications, Lda. Under CC license (CC BY-NC-ND 4.0)

behavior is determined by a plurality of factors that

have different influences on it. It should be

emphasized that a criminological forecast is always

probabilistic in nature, since with respect to any

person it is impossible to say with an absolute

guarantee that he will commit or not commit a crime.

But the main factor influencing the possibility of

committing a crime, including recidivism, is the

individual danger to the personality of the offender,

which is formed during life, which consists not only

in the fact that the person turned out to be able to

commit an already actually committed crime, but also

in the fact that he is able to under certain conditions

and continue to do so.

In this work, the proposed mathematical model

proceeds from the fact that some aspects of the

complex behavior of an individual can be formalized

in some system from a multitude of simultaneously

interacting factors that affect the personality.

Individual behavior can be formalized on the basis of

very simple rules for the interaction of independent

components that negatively affect the personality and

determined using mathematical equations. For this, a

hypothesis is introduced that the probability of

individual criminal behavior of a person is

proportional to the total recorded number of crimes in

the period of interest. This hypothesis allows us to

create an equation in which the unknown is the

personality dangerousness depending on time. Time

discretization of the process of observing a person

allows the resulting integral equation to be reduced to

linear algebraic equations that are easily solved.

2 FORMULATION OF THE

PROBLEM AND ACCEPTED

CONCEPTS

To model individual criminal behavior, we introduce

several functions that have a quantitative value. Let

us introduce the personality function C

(Characteristic of Criminal) as a negative

characteristic of the subject's personal qualities that

increase the likelihood of relapse. Let us assume that

the function C

will be determined by several known

values obtained from information during observation

in a certain period about the person under study. Let

us assume that the value of this function may vary

within

10 ≤≤

We also introduce the function S

(Social

determinants). This function will also have a known

quantitative value and will change within

0 ≤≤

The S

function will be determined by the influence

of negative social processes taking place in society,

affecting the subject and increasing the likelihood of

relapse. We assume that the function S

will be

determined by several known values obtained from

statistical sources.

The introduction of the function C

and S

, which

vary from zero to one, is like the damage function in

the mechanics of materials, which is used in the study

of a damaged material. It is known that the influence

of various types of damage on the characteristics of

the deformation process is carried out with the help of

a scalar function ω – a measure of damage. The value

of ω, which varies from the value ω=0 for an

undamaged material, ω=1 – for a destroyed material.

For mathematical modeling of individual criminal

behavior, we introduce the function D (Personality

dangerousness). This function will determine the

danger of a person, i.e. propensity to commit a crime.

We accept that the function will be determined from

the solution of the problem of modeling the process

of the criminal behavior of the subject, before and at

the time of the commission of the crime.

Since the personality of a criminal can differ in

different suggestibility – susceptibility to various

social processes, we introduce the coefficient s

(Suggestibility). We accept that this coefficient is

determined by the psychophysiological

characteristics of the subject's personality and will

have a quantitative value within

10 ≤≤ s

. At the same

time, we assume that at s=0 the subject is not

receptive, but at s=1 it is strongly receptive to various

social processes taking place in society.

Thus, any person at every moment of time will be

under the influence of negative formed personal

qualities and several social negative processes taking

place in society. Given the different susceptibility of

the individual, the negative "load" on a person can be

represented as a sum of influences

)(

sSC +

that

affects a person at any given time.

3 ACCEPTED HYPOTHESES

AND RESOLUTION

EQUATIONS

Let us introduce the concept of risk assessment of the

commission of a crime R (Risk assessment) as a

product of functions

RsSCD

=+× )(

. The meaning

of this concept lies in the fact that under the same

external conditions, a crime will be committed by a

person who has a greater function of the danger of

Crime-related Threats on Transportation Safety: A Mathematical Model of Risk Assessment

273

personality D. Since the risk of a crime is a

probabilistic value that depends on time t, then we

define it as the probability of committing a crime

under the prevailing conditions, and, therefore,

𝐷(𝑡) × [𝐶



(𝑡) + 𝑠𝑆



(𝑡)] = 𝑃



(𝑡)

(1)

Consider the ultimate crime scenario. If a crime

has occurred, then, therefore, the probability of its

commission is equal to one

1)( =tP

(2)

Suppose that a set of formed personal qualities

and a spectrum of social negative processes has the

highest possible importance, therefore

.2)]()([ =+ tsStC

(3)

We normalize equation (3), dividing it to 2

.12)]()([

=×+

−

tsStC

(4)

Considering equations (1) and (4) we obtain that

the function of personality dangerousness D at the

time of the crime under the most unfavorable external

conditions is equal to one

.1)( =tD

(5)

Since all parameters of equation (1) depend on the

time t, integrating it

.)()]()([(2)(

dttPdttsStCtD



−

=+×

(6)

Here

is the period of observation of the subject.

Equation (6) is an integral equation that contains

unknown functions personality dangerousness D and

the probability of committing a crime

It is known that in mechanics and physics in

solving problems that are described by differential

and integral equations, research objects are divided

into a few elements in which constant mathematical

values are taken or some known functions. This

technique allows you to solve many scientific and

applied problems (Grigorenko, 1999; Emelyanov I.

G. 2009; Emelyanov I. G. 2018).

It is known that the integral equation can be

represented as a system of linear algebraic equations.

We divide the period of monitoring the subject of

on N steps over time (day, month, year, ...) in which

the functions entered above will have a constant

value. Thus, the time step will be equal

1−

×Δ= NTt

)....1( Ni =

(7)

For each step in time, we write equations (6)

iRiidicii

tPtsSCD =+×

−

][(2

(8)

Accepting a permanent and dimensionless time

step, equation (8) will take the for

Ridicii

PsSCD =+×

−

][(2

(9)

In the system (9) in the right part, the likelihood

of committing a crime within each time period is

presented. Since we will explore cases with an

intentional crime at the end of the observation period,

the probability of its offensive will be equal to one.

Therefore, we will have an additional equation



(10)

In system (9), on the right side, the probabilities

of committing a crime by some subject from a certain

group of offenses that occurred in the period of

interest are presented. We accept the hypothesis that

the probability of a single crime from a certain

observation group is proportional to the probability of

the totality of crimes committed in a certain industry

over a certain period of time. Therefore, one can write

iRi

kpP =

(11)

Here k – unknown coefficient of proportionality,

– the probability of committing crimes committed

in a certain industry for a certain period. The

probability of committing crimes in a certain territory

for a certain period can be defined as

Popu

(12)

Here

– the number of intentional crimes in a

certain industry in a certain time,

– the total

population.

Considering the expression (11) and (12), system

(9) will have the form

OPU

dicii

sSCD =+×

−

][(2

(13)

From equation (10) one can determine the

coefficient k for the right side of equation (13)



OPU

(14)

Considering relations (14), system (13) takes the

form

TLC2M 2022 - INTERNATIONAL SCIENTIFIC AND PRACTICAL CONFERENCE TLC2M TRANSPORT: LOGISTICS,

CONSTRUCTION, MAINTENANCE, MANAGEMENT

274



=+×

−

sSCD

][(2

(15)



=+×

−

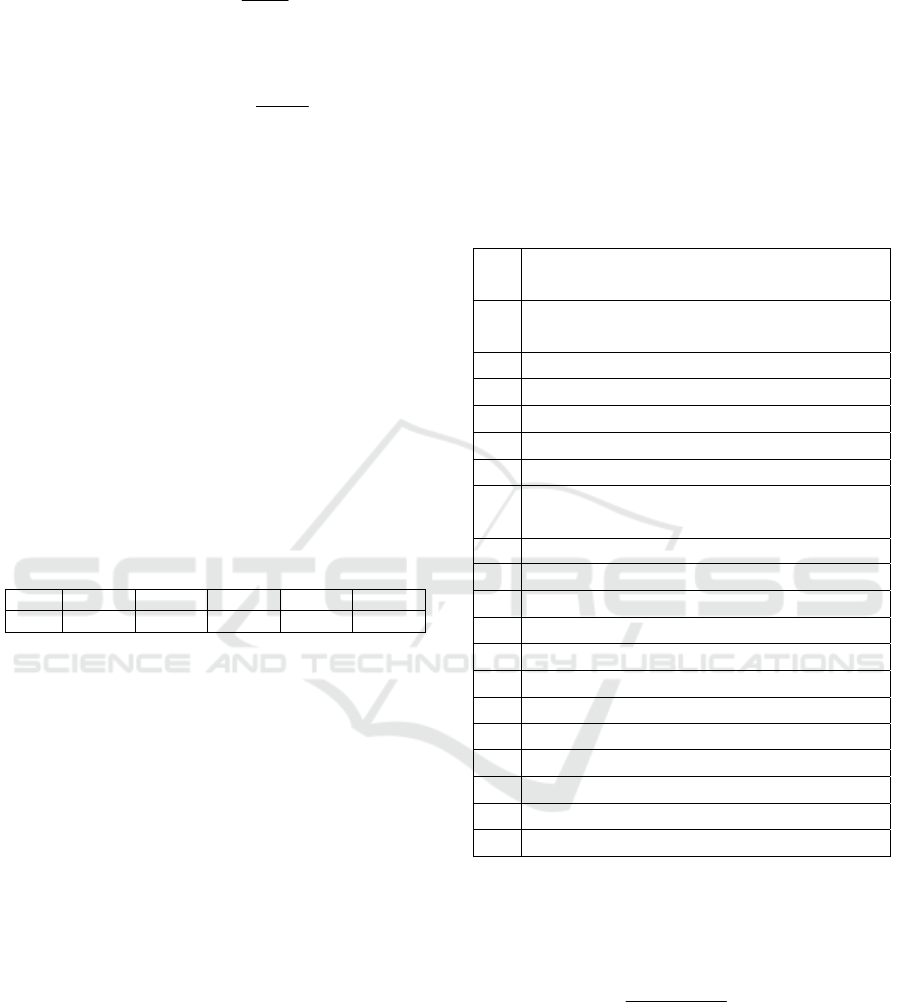
dNcNN

sSCD

][(2

4 EXAMPLE OF USING THE

PROPOSED METHOD

Let us define the function personality dangerousness

D of the offender for the criminal case committed in

2014. Criminal case № 1-649/2014

https://sudact.ru/regular/doc/O6J6wFXEKtrt/.

The period of observation of the subject 2010 -

2014,

= 5 years.

=1 year is a dimensionless time

for collecting statistical information.

Table 1 shows the state of crime in the Russian

Federation in thousands of registered crimes.

Table 1: The state of crime in the Russian Federation.

Year 2010 2011 2012 2013 2014

2628,8 2404,8 2302,2 2206,2 2166,4

To determine the personality function Cc, we will

assume that the personal qualities of the subject under

study will be characterized by a certain set of diskette

values obtained from statistical sources. For this

case, we assume that a person can be characterized by

20 (

= 20) values that contribute to a crime and are

presented in Table 2. Since the maximum value of

=1, then, assuming the hypothesis of the same

influence of each factor on the commission of a

crime, each component will "weigh"

−

×kC

= 0.05. The accepted hypothesis means that in the

presence of all 20 negative factors, a person has the

maximum probability of an offense.

Therefore, we will assume that the personal

qualities of the subject during five years of

observation are constant and equal to Сс=0.35. It

should be added that the quantitative values in this

table 2 may change during the observation period,

which can be considered in equation (15).

We accept that the function of the influence of

negative social processes on the personality of the

subject under study Sd will be characterized by a set

of diskette values obtained from statistical sources.

For this case, we will assume that four (

= 4)

negative social factors contributing to crime can

influence a person. These are international migration,

lack of cash income, the number of unemployed aged

15-72 and urbanization. Information on these

processes for the observation period from 2010 to

2014 is available on the website

https://www.gks.ru/statistic.

Table 2: Characteristics of the criminal

The presence of psychopathy and psychopathic

conditions – 0

Organic lesions of the central nervous system,

brain contusions, mental anomalies – 0

Aldolization and narcotization – 0.5

Emotional instability – 0

Social degradation, marginalization – 0

Ethnic, religious intolerance – 0

Belonging to a criminal subculture – 0

Belonging to other marginal groups, informal

groups of an illegal orientation – 0

Low level of material security – 0.5

10.

Lack of work– 0.05

11.

Employment in unskilled labor –0.5

12.

Not married –0.5

13.

Lack of secondary education –0.5

14.

The presence of a criminal record – 0

15.

Bringing to criminal liability – 0

16.

Lack of housing –0

17.

Belonging to the urban population – 0.5

18.

Cynicism, legal nihilism – 0

19.

Unresolved life conflicts – 0

20.

Facts of domestic violence – 0

Statistical information on international migration

in the number of people for 2010-2014 is calculated

using the formula

minmax

min

−

(16)

Here M

, M

max

, M

min

– the current, maximum, and

minimum value of international migration

Table 3 shows the normalized distribution of

international migration calculated by formula (16) (a

function of negative social processes occurring in

society S

Crime-related Threats on Transportation Safety: A Mathematical Model of Risk Assessment

275

Table 3: International migration.

Yea

2010 2011 2012 2013 2014

0. 0.413 0.566 0.727 1.

Similarly to international migration, statistical

information is processed on the deficit of money

income (in billion rubles), the number of unemployed

aged 15-72 years (in thousands of people) and

urbanization (in thousands of people). Table 4 - Table

6 provides information on the normalized distribution

of the deficit of money income, the number of

unemployed and urbanization (total urban population

growth) calculated by formula (16), respectively.

Table 4: Deficit of income

Yea

2010 2011 2012 2013 2014

0.04 0.477 0. 0.415 1.

Table 5: Number of unemployed

Yea

2010 2011 2012 2013 2014

1. 0.624 0.145 0.149 0.

Table 6: Urbanization

Year 2010 2011 2012 2013 2014

0.355 0. 0.505 1. 1.

For the maximum factor Sd to be equal to one, as

defined above, the values in tables 3-6 must be

reduced by a factor, therefore

−

× kS

Considering the above calculations and setting a

low coefficient of the subject's susceptibility to social

factors s=0.25, system (15) takes the form

4.11708

8.2628

]348.025.035.0[(2

=×+×

−

4.11708

8.2404

]378.025.035.0[(2

=×+×

−

4.11708

2.2302

]304.025.035.0[(2

=×+×

−

4.11708

2.2206

]572.025.035.0[(2

=×+×

−

4.11708

4.2166

]75.025.035.0[(2

=×+×

−

(17)

Figure 1 shows the law of change function

personality dangerousness D over the observation

period from 2010 to 2014. Curves 1,2,3 correspond to

the calculation for the susceptibility coefficient

s=0.25 s=0.5 s=0.75.

Thus, it can be stated that for the subject of the

considered criminal case, the function personality

dangerousness D for not repeating the crime, under

equal external conditions, should be less than

D<0.405.

Figure 1: The law of change function personality

dangerousness D over the observation period

5 DISCUSSION OF RESULTS

SOLUTIONS AND

CONCLUSIONS

In this article, the function personality dangerousness

was found based on a specific crime. It should be

emphasized that we propose to extend our model only

to cases of probation, that is, in this model there are

no changes in environmental factors, the offender is

not imprisoned.

The paper proposes a mathematical model that

describes the function personality dangerousness.

Quantifying this function makes it possible to assess

the risks of relapse in probationers based on

extrapolation of social determinants influencing

crime and predictions of the state of crime, as well as

data on changes in personality traits.

Based on the extrapolation of social determinants

influencing the crime, and forecasts of the state of

crime, as well as data on the individual in the time -

the term of probation, knowledge of the function

personality dangerousness will make it possible to

assess the risks of relapse. If the function exceeds

D>0.405, this dramatically increases the risk of

recurrence and requires the subjects to respond

proactively and take preventive measures.

Thus, numerical modeling of individual criminal

behavior through a comprehensive account of

external and internal factors affecting the individual's

behavior can be an effective way to predict such

processes. The method can be used to counter security

threats in transport.

2010 2011 2012 2013 2014

0.25

0.5

0.75

T, yaer

TLC2M 2022 - INTERNATIONAL SCIENTIFIC AND PRACTICAL CONFERENCE TLC2M TRANSPORT: LOGISTICS,

CONSTRUCTION, MAINTENANCE, MANAGEMENT

276

REFERENCES

Srivastav, A. K., Athithan, S., 2020. Modeling and analysis

of crime prediction and prevention, Social Network

Analysis and Mining 10:26, p. 21.

Syed, F. S., Li, D., Zhang, X., Guo, Z., 2013. Mathematical

Modelling in Criminology Malaysian Journal of

Mathematical Sciences, 7(1): pp. 125-145.

Farrington, D. P., MacLeod, J. F., Piquero, A. R., 2016.

Mathematical Models of Criminal Careers: Deriving

and Testing Quantitative Predictions, Journal of

Research in Crime and Delinquency, Vol. 53(3) pp.

336-355.

Skeem, J., Lowenkamp, C., 2016. Risk, race, and

recidivism: predictive bias and disparate impact.

Criminology, 54(4), pp. 680–712.

Miller, J., Lynam, X., 2001. Structural models of

personality and their relation to antisocial behavior: A

meta-analytic review. Criminology, 39, pp. 765-798.

Farrington, D. P., 2003. Developmental and life-course

criminology: Key theoretical and empirical issues—

The 2002 Sutherland award address. Criminology,

41(2), pp. 221–225.

Grigorenko, Ya. M., Vasilenko, A. T., Emel'yanov, I. G. et

al., 1999. Statics of structural elements Vol. 8 of the 12-

volume series Mechanics of composites. р. 379.

Emelyanov, I. G., 2009. Contact problems of the theory of

shells, p. 185.

Emelyanov, I. G., 2018. Mechanics of Shells and Its

Applications, Diagnostics, Resource and Mechanics of

materials and structures 2, pp. 6-28. http://dream-

journal.org/issues/2018-2/2018-2_165.html.

Crime-related Threats on Transportation Safety: A Mathematical Model of Risk Assessment

277