
Stability Analysis of Fuzzy Mathematical Measles Model
H. A. Bhavithra and S. Sindu Devi
*
SRMIST, Department of Mathematics, Ramapuram Chennai, India
*
Keywords: Stability Analysis, Basic Reproduction Number, Fuzzy Basic Reproduction Number, Euler Method.
Abstract: The well-known susceptible-infected-recovered (SIR) mathematical model is used in this work to investigate
the disease's spread utilising fuzzy parameters. We have demonstrated that when the reproduction number is
less than unity, the disease-free equilibrium point is locally asymptotically stable. In order to extend the
concept of basic reproduction number, we are creating a fuzzy basic reproduction number. We are examining
the approximate numerical solution of the fuzzy non-linear differential equation applying Euler method and
the outcome is examined with the basic reproduction number.
1 INTRODUCTION
One of the most aggressive bacterial illnesses, measles
is brought on by the measles virus, which is found in
the snot of affected people's throats and noses. Among
all animal species, this virus is solely present in the
human body and belongs to the paramyxovirus family
(genus morbilivirus). The infected person's spitting
and blowing can directly transmit this virus from one
person to another. High fever, coughing, cataracts,
allergies, little white spots on the body, and a rash are
the early symptoms of measles in infected individuals.
More vulnerable populations include people over the
age of 20 and those under the age of five who are more
at risk from this illness. Infections in the ears and
sinuses, oral ulcers, diarrhoea, and malnutrition are
among the consequences.
Measles outbreaks were reported on multiple
occasions in various locations throughout
Bangladesh, one of the South East Asia Region
(SEAR) countries, between 2000 and 2016. In
Bangladesh during this time, there were 33,213
recorded incidents and approximately 70,273
reported cases of the measles14. Despite the fact that
the Expanded Program of Immunization (EPI) began
in Bangladesh in 1979 to control and prevent measles.
The government has continued these efforts in 2014
with the introduction of the combined treatment of the
measles-containing vaccine (MCV2), with the goal of
eradicating the disease nationwide by 2018. As a
result, during the past few decades, measles cases
*
Corresponding author.
have decreased by up to 84%. However, since 2016,
there has been an increase in measles cases across the
country. Considering certain already-existing
obstacles, Rohingya refugees provide yet again
another difficulty in the fight against measles.
The study of communicable diseases
epidemiologic is heavily reliant on mathematical
modelling and simulation. A key factor in the
rigorous investigation, dissemination, and treatment
of the disease is the use of mathematical models.
Applying contemporary processing capabilities is a
straightforward way to achieve the desired results.
This is utilized to solve problems in many different
fields. The authors have created a model that
increases awareness through interactions with the
aware population and public awareness campaigns
(Agaba al. 2017). The developed framework is
predicted to be advantageous to the researchers and
medical professionals engaged in respiratory cancer
charities. Making plans and making decisions for
lung cancer prevention and treatment may help to
identify the underlying causes of lung cancer and take
the necessary steps to control them, thus enhancing
global public health (Ahmed et al. 2021). The
model's simulation results, according to the authors,
shows that, under certain circumstances, brucellosis
will entirely vanish or see a discernible drop
throughout the whole population of the Democratic
People's Republic of Congo (DRC) (Kasereka et al.
2020). Using data on pulmonary tuberculosis in
Malang, the results of hypothesis assessment and
Bhavithra, H. and Devi, S.
Stability Analysis of Fuzzy Mathematical Measles Model.
DOI: 10.5220/0012509500003739
Paper published under CC license (CC BY-NC-ND 4.0)
In Proceedings of the 1st International Conference on Artificial Intelligence for Internet of Things: Accelerating Innovation in Industry and Consumer Electronics (AI4IoT 2023), pages 423-428
ISBN: 978-989-758-661-3
Proceedings Copyright © 2024 by SCITEPRESS – Science and Technology Publications, Lda.
423

computation showed that cases spiked briefly before
declining in Makassar (Side et al. 2018). The
simulation results show that COVID-19 transmission
varies with corona virus load. The effects of
vaccination and adherence to rules governing health
have an equal influence on slowing or stopping the
spread of COVID-19 in Indonesia (Abdy et al. 2021).
The infected cases in India that a time series
projections model is utilized for, as well as the time
series predictions of actual cases against expected
instances in India, were both examined by Bimal
Kumar Mishra et al. (Mishra et al. 2021), as well as
a time series forecasting model for India's case
fatality rate. The major goal of this research is to
restrict the propagation of the viral by estimating the
reproduction number. The researchers have created
the SEIR-SEI mathematical model by taking a few
characteristics into consideration as fuzzy numbers
by sweatha.et.al (Sweatha et al. 2022) The presence
of backward bifurcation, as stated in Fuzzy SIR-
epidemic model for transmission of measels by
zaman.et.al (Zaman et al. 2017) The major goals of
this paper are to investigate the fuzzy SIR model for
malaria using stability analysis and backward
bifurcation by Yuyang Chen.et.al (Joshua et al. 2020,
Isaac et al. 2015, Kaiming et al. 2020).
2 PRELIMINARIES
2.1 Fuzzy Set
Let X be a nonempty crisp sets. A fuzzy subset S of
X is denoted by and is defined as:
Where
: X→ [0,1] is a membership function
associated with a fuzzy set
which describes the
degree of belongingness of x with X.
Here we use the membership function µ(x) to
indicate the fuzzy subsets
. Also µ(x) is called fuzzy
number if X is the set of real numbers.
2.2 Fuzzy Measure
Let Ω be a non-empty set and P(Ω) denote the set of
all subsets of Ω. Then µ: Ω → [0,1] is a fuzzy
measure if (Zaman et al. 2017):
1) µ(ϕ) =0 and µ(Ω) =1
2) for A,B P(Ω ) ,µ(A) ≤ µ(B) if A⸦ B
Let µ: Ω → [0,1] be an uncertain variable, i.e.) µ is a
fuzzy subset and µ a fuzzy measure on Ω. Then fuzzy
expected value (FEV) of µ is the real number, defined
by the Sugeno measure.
〗
Where
2.3 Fuzzy Mathematical Model
The core SIR model splits the population contributing
to the transmission of an ailment into three
epidemiology classes: Susceptible covers persons
who have the ability to contract the illness, infected
people who are contagious, and removed people who
have previously been associated with the disease's
spread. The SIR model's equation system is listed
below:
Susceptible: They are the people who are exposed to
the spread of the disease out of the overall population
(N).
Infected: People who exhibit infectious disease
symptoms are classified as to the infected population.
They can transmit the disease because they are also
contagious.
Recovered: Persons who have undergone treatment
or taken vaccination and recovered from the
infectious disease are termed as recovered population.
When developing the model for the transmission of
measles among people with the entire population, the
system of nonlinear ordinary differential equations is
taken into account (Zaman et al. 2017). Let says that
is the virus load.
= (1)
= (2)
= (3)
Where
• is the rate of transmission
• is the recovery rate
• is the rate of losing immunity
• is the virus load
2.4 Analysis of Fuzzy System
be the membership function this is a rising
function because as the population of people who are
susceptible grows, the risk of infection will also grow.
The transmission rate is selected to be fuzzy
membership function because it is more appropriate
than the alternatives. In order to build a fuzzy
membership function, we assume that the virus load
of the disease in an individual is quite low (
,
AI4IoT 2023 - First International Conference on Artificial Intelligence for Internet of things (AI4IOT): Accelerating Innovation in Industry
and Consumer Electronics
424
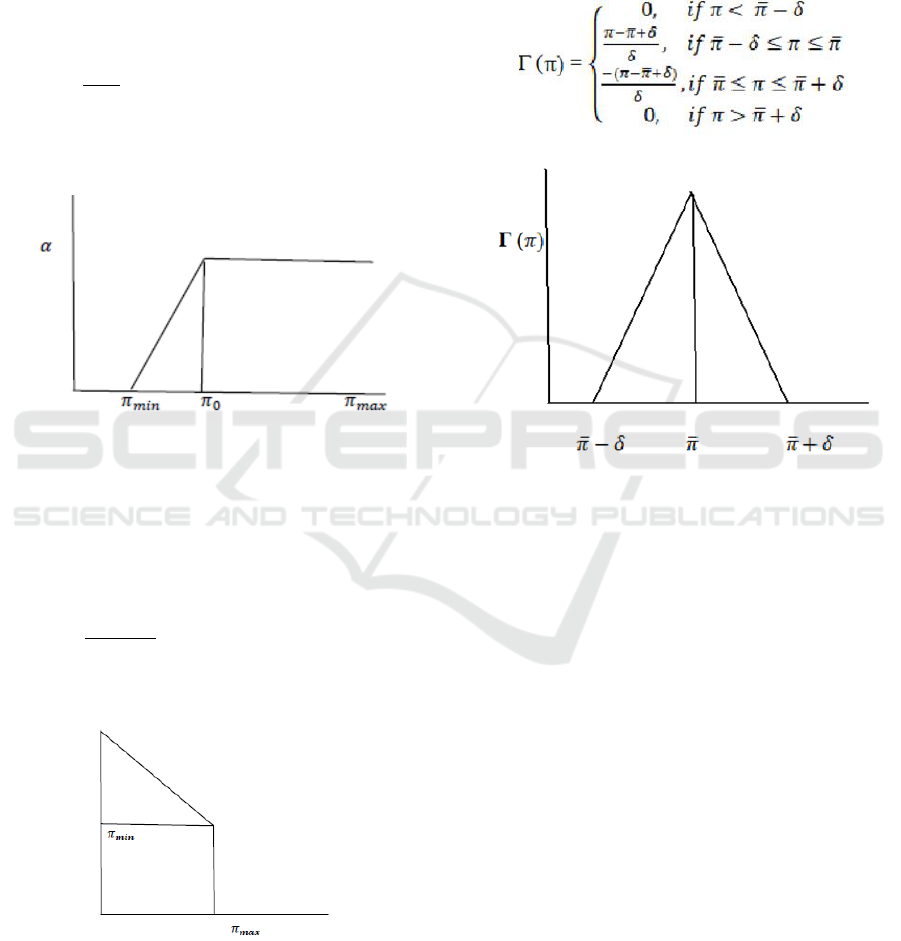
making the possibility of transmission negligible. The
least amount of virus load required to transmit the
disease is
. When the virus load is at its highest,
the viral spread rate reaches its optimum level and
reaches one. However, we presume that
is the
maximum permitted viral load that an individual can
have. We define as follows and fig:1 describes
the membership function of
as follows
(Sweatha et al. 2022):
(4)
Where
is the lowest infection rate.
Figure 1.
And is the fuzzy membership function
for the recovery rate. It is a decreasing function since
a higher viral load makes recovery from infection
take longer. The fuzzy membership function of
is represented in the following equation
=
The recovery rate that is lowest is
. Figure 2
illustrates how the membership function
works.
Figure 2.
2.5 Membership Function of Γ (
Additionally, as virus load is regarded as a linguistic
variable, we take into account the fact that different
individuals have varying viral loads. The members of
the linguistic variable are listed below (Sweatha et al.
2022):
Figure 3: Membership function of Γ (
The symbols and stand for the centre value
and dispersion of each fuzzy set assumed. There are
three levels for the linguistic variable: weak, medium,
and high. Every classification can be seen as a
triangular-shaped fuzzy number. The following
graphic provides a diagrammatic representation of the
membership function Γ ( . The figure 3 is the
membership function of Γ (
Equilibrium Points
The disease free equilibrium and endemic
equilibrium are the two equilibrium points in the
model. In order to find these two equilibrium points
each of the equations in (1),(2),(3) must equals zero.
Disease Free Equilibrium Points
The points of DFE implies that there is no
transmission of the disease, namely I =
= 0 and R
=
= 0. Thus the DFE is
S =
=
= (
= (
Stability Analysis of Fuzzy Mathematical Measles Model
425

Basic Reproduction Number
BRN is the average number of secondary infections
carried on by a single infectious person throughout
the lifetime of an infection. The BRN is determined
using next generation matrix method” (Isaac et al.
2015, Kaiming et al. 2020).
F = V =
=
(5)
3 STABILITY ANALYSIS
Theorem 1:
The DFE of our model is locally asymptotically stable
when
Proof:
The Jacobian matrix is
J =
At DFE
=
The characteristic equation at the disease-free
equilibrium point is
(6)
According to Routh-Hurwitz criteria the disease-free
equilibrium point is said to be asymptotically stable.
Global Stability
While considering Lyapunov function
by substituting the value of we
found that the lypnov function is 0 iff I is 0. Hence by
Lasalle’s invariance principle the disease is globally
asymptotically stable.
Fuzzy Basic Reproduction Number
The fuzzy basic reproduction number is given by
(Zaman et al. 2017),
(
R
0
())
where (
R
0
()) = sup {inf (τ, k(τ))}, 0
k(τ) =
which is a
fuzzy measure. To obtain (
R
0
()) we need to
define fuzzy measure which is given by
,Ɐ
From (
R
0
()), here R
0
() is not diminishing
with, where the set, X= [
, and π is the
solution to the underlying expression
Thus, k(π) =
= sup with
where k(0) = 1 and k(1) = (
.
The amount of virus in the population which
“was assumed as a linguistic meaning is classified
into three cases and all of them has fuzzy behaviour.
They are weak virus load
, medium virus load
(
) and strong virus load (
Case 1: Weak virus load
(i.e.) when
, we have
(
R
0
()) = 0 <
Thus, we can conclude that the disease will be extinct.
Case 2: Medium virus load” (
(i.e.) when
and
Therefore,
k(
So, if, k ( is continuous and diminishing
function with k(0) = 1 and k(1) = 0. Hence,
(
) is the fixed point of k and
(
))
AI4IoT 2023 - First International Conference on Artificial Intelligence for Internet of things (AI4IOT): Accelerating Innovation in Industry
and Consumer Electronics
426

As the function is increasing and continuous
function then by the intermediate value theorem there
exists with such that
R
0
() >
There exists virus load such that
and R
0
()
equivalent. Additionally, the average number of
secondary cases
is higher than the number of
secondary cases
due to the medium amount of
virus.
Case 3: Strong virus load” (
(i.e.) when
and
, then
k(
Similar to case 2, we have
(
R
0
())
Thus,
we can infer that the illness will be
widespread.
3.1 Numerical Simulation
In order to solve the numerical simulation, we made
the following assumptions: S = 500, I = 100, R = 0,
= 0.00000181, = 0.0009, = 0.2
a
Figure 4: Susceptible population using Euler.
b
Figure 5: Infected population using Euler’s method.
c
Figure 6: Recovered population using Euler’s method.
d
Figure 7: represents the graph for basic reproduction
number.
The figures 4, 5, 6 are the susceptible, infected and
recovered population which is depicted using Euler’s
method. Figure d shows the dynamical behaviour of
basic reproduction number. It also shows that when
R0 value less than unity, that is around 30 days
infection graph in fig b also falls down after 30 days
In order to solve the numerical simulation, we
made the following assumptions: S = 500, I = 100, R
= 0, α = 0.00000181, β = 0.0009, µ = 0.2. Using
Euler method we found first 20 values in the similar
manner we can find for next consecutive days.
In order to solve the numerical simulation, we made
the following assumptions:
S = 500, I = 100, R = 0, = 0.00000181, = 0.0009, = 0.2
4 CONCLUSION
The compartmental SIR epidemic model has been utilized
in this paper to examine the population spread. We
Stability Analysis of Fuzzy Mathematical Measles Model
427
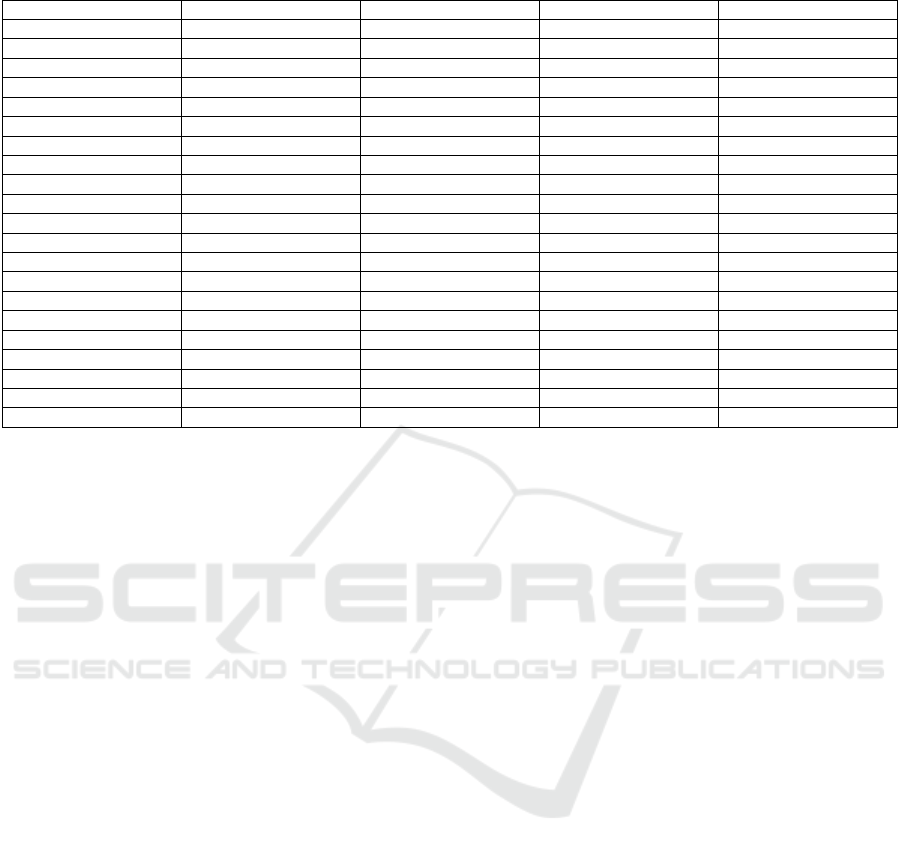
Table 1: is calculated using Euler’s meth.
X (Date)
Y (R0)
Y (Susceptible)
Y (Infectives)
Y (Recovered)
0
1.005555556
500
100
0
1
1.00537355
499.9095
100.0005
0.09
2
1.005191577
499.8190161
100.0009836
0.18000027
3
1.005009636
499.7285483
100.0014509
0.270000795
4
1.004827728
499.6380967
100.0019017
0.360001561
5
1.004645852
499.5476612
100.0023362
0.450002553
6
1.004464009
499.4572419
100.0027544
0.540003755
7
1.004282198
499.3668387
100.0031562
0.630005154
8
1.00410042
499.2764517
100.0035416
0.720006735
9
1.003918674
499.1860809
100.0039106
0.810008482
10
1.003736961
499.0957263
100.0042633
0.900010382
11
1.00355528
499.0053879
100.0045997
0.990012419
12
1.003373632
498.9150658
100.0049196
1.080014578
13
1.003192017
498.8247599
100.0052233
1.170016846
14
1.003010435
498.7344702
100.0055106
1.260019207
15
1.002828885
498.6441968
100.0057815
1.350021646
16
1.002647368
498.5539397
100.0060361
1.44002415
17
1.002465883
498.4636989
100.0062744
1.530026702
18
1.002284432
498.3734743
100.0064964
1.620029289
19
1.002103013
498.2832661
100.006702
1.710031896
20
1.001921627
498.1930742
100.0068913
1.800034507
determined the membership function and derived the
fuzzy parameters as a function of viral load. We have
identified the conditions for the local stability of the
endemic equilibrium and the disease-free equilibrium
of our model. Figure 7 shows the dynamical
behaviour of basic reproduction number. It also
shows that when R0 value less than unity, that is
around 30 days infection graph in fig. 5 also falls
down after 30 days. That is around 35 days the
infection rate reduced to 20% in the meantime
recovery rate gradually increases around 2%.
REFERENCES
Agaba, G. O., Y. N. Kyrychko, and KB3619199 Blyuss.
"Mathematical model for the impact of awareness on
the dynamics of infectious diseases." Mathematical
biosciences 286 (2017): 22-30.
Ahmed, Jobayer, and Md Haider Ali Biswas.
"Mathematical Modeling and Analysis the Effect of
Smoking for the Dynamics of Lung Cancer”.
Proceedings of the 11th Annual International
Conference on Industrial Engineering and Operations
Management Singapore, March 7-11, 2021.
Kasereka Kabunga, Selain, Emile F. Doungmo Goufo, and
Vinh Ho Tuong. "Analysis and simulation of a
mathematical model of tuberculosis transmission in
Democratic Republic of the Congo." Advances in
Difference Equations 2020.1 (2020): 1-19.
Side, S., A. M. Utami, and M. I. Pratama. "Numerical
solution of SIR model for transmission of tuberculosis by
Runge-Kutta method." Journal of Physics: Conference
Series. Vol. 1040. No. 1. IOP Publishing, 2018.
Abdy, Muhammad, Syafruddin Side, Suwardi Annas,
Wahyuddin Nur, and Wahidah Sanusi. "An SIR
epidemic model for COVID-19 spread with fuzzy
parameter: the case of Indonesia." Advances in
difference equations 2021, no. 1 (2021): 1-17.
Mishra, Bimal Kumar, Ajit Kumar Keshri, Dinesh Kumar
Saini, Syeda Ayesha, Binay Kumar Mishra, and Yerra
Shankar Rao. "Mathematical model, forecast and
analysis on the spread of COVID-19." Chaos, Solitons
& Fractals 147 (2021): 110995.
G. Zaman, Y. H. Kang, G. Cho, and I. H. Jung, “Optimal
strategy of vaccination & treatment in an SIR
epidemic model,” Mathematics and Computers in
Simulation 136, 63–77 (2017).
Sweatha Selvakumar, S.Sindu Devi (2022), Fuzzy
epidemic model for the transmission of Zika virus, ECS
Transacation, 107(1), 16851-16858.
Joshua Kiddy K.Asamoah, Farai Nyabadza ,Zhen Jin
,Ebenezer Bonyah, Muhammad Altaf Khan, Michael Y.
Li, Tasawar Hayat(2020),Backward bifurcation and
sensitivity analysis for bacterial meningitis
transmission dynamics with a nonlinear recovery rate,
Chaos, Solitons and Fractals,140,110237.
Isaac Mwangi Wangari Stephen Davis, Lewi Stone (2015)
backward bifurcation in epidemic models: Technical
Note, Applied Mathematical Modelling, 904.
Kaiming Bi, Yuyang Chen, Chih-Hang Wu, David Ben-
Arieh (2020), A Memetic algorithm for solving optimal
control problems of zika virus epidemic equilibriums
and backward bifurcation analysis, Communication in
Nonlinear Science and Numerical simulation, 5704.
AI4IoT 2023 - First International Conference on Artificial Intelligence for Internet of things (AI4IOT): Accelerating Innovation in Industry
and Consumer Electronics
428
