Developing a Sequential Mask Projection Technique for Micro-Lens

Generation Using Excimer Laser Micro-Structuring

Eric Syrbe, Sebastian Buettner, Michael Pfeifer and Steffen Weissmantel

Laserinstitut Hochschule Mittweida, Technikumplatz 17, Mittweida, Germany

Keywords: Excimer Laser, Micro-Optics, Micro-Lenses, Fluorine Laser, Direct Laser Fabrication, Micro Machining.

Abstract: Fluorine laser micro-structuring enables the generation of micro-optics in glasses and other wide band gap

materials. For the generation of micro-lenses, we developed a new micro-structuring method and the

appropriate hardware. The process is based on the mask projection technique and uses a set of different

circular masks, which are placed consecutively within the laser beam. This creates a ring-shaped ablation area

with variable inner radius. By using an appropriate set of masks, it is possible to generate a surface with a

defined spherical shape. The measured radii of curvature of the structured micro-lenses are in the range of

90 μm up to 250 μm with corresponding surface roughness values to below 100 nm. The entire process and

requirements are described, and the results are presented.

1 INTRODUCTION AND STATE

OF THE ART

Current technical innovations are often based on

improved efficiency or a more compact design. The

latter case requires a miniaturization of the included

parts, which is a constant challenge for the involved

manufacturing processes. One of the driving forces

behind are the computer and communication

technologies, because data transmission via the classic

copper cable is limited in terms of transmission speed

and becomes more susceptible to electromagnetic

interference fields the greater the volume of data

transmitted per second. One solution is offered by

optical data transmission, which is insensitive to these

effects and shows a low signal attenuation, so that

signals can be transmitted over long distances with

virtually no loss or errors. The coupling of the optical

signals into progressively smaller wave guiding

structures such as mono mode fibres require micro-

optics with best possible shape accuracy and low

surface roughness. A wide range of processes was

developed to fulfil these demands. Imprinting (Moore,

Gomez, & Lek, 2016) and additive techniques

(Bückmann, Schittny, Thiel, & Kadic, 2014; Kim,

Brauer, Fakhfouri, & Boiko, 2011) are especially used

to produce polymer micro-optics. But there is still a

great need for development regarding the production of

micro-optics in glass materials and in high-quality

fused silica, e.g. for integrating optics into a wafer.

Only a few processes are still suitable for processing

this material, with the most used being lithography.

However, the latest developments are increasingly

using femtosecond (Hua, Liang, Chen, Juodkazis, &

Sun, 2022) and CO

lasers (Zhao et al., 2022) or a

combination of both (Sohn, Choi, Noh, Kim, & Ahsan,

2019) to achieve the desired lens quality. The approach

we have been pursuing for some years now is to use

excimer lasers and the mask projection technique for

this purpose. It has been successfully demonstrated that

a wide variety of micro-optics can be produced using

the numerous structuring methods we have developed.

But as with the other laser structuring methods the

achievable quality is still a major challenge.

In this research we aim to reduce the influence of

re-deposition of debris at the ablation area during the

patterning process. The previously developed method

uses a rotating mask where the debris is always

pushed ahead into the following ablation area. This

results in irregularities in the structure and its surface

(Buettner, Pfeifer, & Weissmantel, 2020). To solve

this problem, we have changed the strategy for lens

structuring to a full area structuring method in which

the ablation area is gradually reduced and always lies

within the previous area. Therefore, almost no debris

should be found inside the ablation area after

structuring. To achieve this gradually changing mask

geometry we used a combination of a set of circular

masks and an outer aperture.

Syrbe, E., Buettner, S., Pfeifer, M. and Weissmantel, S.

Developing a Sequential Mask Projection Technique for Micro-Lens Generation Using Excimer Laser Micro-Structuring.

DOI: 10.5220/0012377400003651

Paper published under CC license (CC BY-NC-ND 4.0)

In Proceedings of the 12th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2024), pages 21-27

ISBN: 978-989-758-686-6; ISSN: 2184-4364

2 EXPERIMENTAL SETUP

2.1 Process

The used system consists of the pulsed fluorine laser

LPF 220i of Coherent Lasersystems GmbH & Co. KG

and the micro machining station EX-157. A detailed

description of the station is given in our previous

work (Buettner, Pfeifer, & Weissmantel, 2019), as the

system is identical. Due to its beam characteristics the

fluorine laser is not suited for the direct-writing

technique. Therefore, a mask projection technique is

applied. To get the laser pulse fluence H evenly

distributed over the mask area the laser beam is

homogenized. If the threshold laser pulse fluence of

the material to be structured is exceeded the

homogenization results in a uniform ablation depth.

Both the laser pulse duration and the used laser

wavelength influence the threshold laser pulse

fluence. The fluorine laser emits photons with a pulse

duration of 25 ns and a wavelength of 157 nm, which

corresponds to a photon energy of 7.9 eV. The photon

energy is of particular interest for the machining of

wide band gap materials, as the difference between

photon energy and energy band gap has a direct effect

on the processing quality. Higher photon energy, in

relation to the band gap energy of the material to be

structured, results in a better surface quality of the

treated material, due to the better absorption

conditions. On the other hand, the energy band gap

determines which optical material can be used as

mask material for the corresponding wavelength of

the laser.

As mentioned above, we targeted to realize a

whole area treatment, which requires a combination

of several masks. More precisely, the goal was to

create a ring-shaped ablation area with a variable

inner and a constant outer radius. The inner radius of

the ablation area is controlled by a set of special

circular masks. The fabrication of the masks is

described in the following subsection.

2.2 Mask Generation

As mentioned, the masks must be transparent for the

wavelength of the laser. We choose calcium fluoride

as mask substrate material, because its band gap

energy of 12.2 eV is higher than the photon energy.

Therefore, the material is transparent for the used

laser wavelength. The 50 mask substrates have a

diameter of 5 mm and a thickness of 0.5 mm. The

latter is very low, so that hardly any losses occur. To

obtain an inner boundary of the ring-shaped ablation

area, it is necessary to generate an opaque area on the

mask substrate. We used a pulsed laser deposited

tantalum coating for this purpose. The main challenge

in generating these opaque areas is to position them

very precisely on the substrate. For the generation of

micro-lenses, these areas are circles with different

radii and these circles must each be concentric to the

substrate. To solve this problem of precise

positioning, a mask holder for the coating process was

developed.

The holder consists of three metal plates, the back

plate, the sample plate, and the front plate with the

sample plate being pinched between the other two

plates. The sample plate is a 0.5 mm thick metal plate

with 56 holes of 5 mm diameter each so that the

calcium fluoride substrates fit ideally into these holes.

Other than the back plate, the front plate also got

56 holes of 4 mm diameter to expose one face of the

substrates for coating. To change the area to be

coated, an additional thin tantalum foil is placed

between the sample and the front plate. In this foil,

holes with different diameters were cut using an ultra-

short pulse laser. In the whole assembly the sample

plate, the tantalum foil and the front plate were

aligned and fixed on the back plate.

All three plates have adjusting holes for a precise

positioning using dowel pins. The adjusting holes

ensure that all coating areas are concentric regarding

the boundary of each substrate. Following, the pulsed

laser deposition coating process is applied to the

assembly. In Figure 1 the coated calcium fluoride

substrates are shown. Due to the coating mask

(tantalum foil) geometries, every area has an

individual inner radius generating ablation areas with

a radius starting from 1 μm up to 50 μm in 1 μm steps

as there are 50 different masks.

Figure 1: Coated calcium fluoride substrates on the sample

plate after the pulsed laser deposition coating process, with

the front plate and the coating mask removed.

PHOTOPTICS 2024 - 12th International Conference on Photonics, Optics and Laser Technology

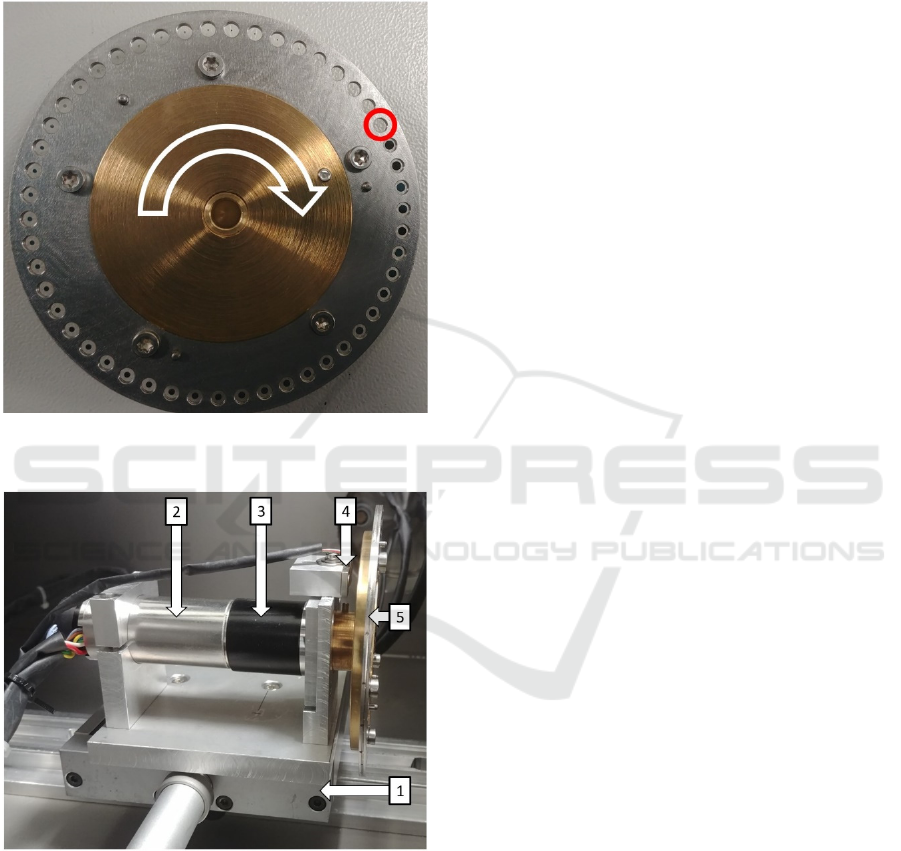
2.3 Hardware

The sequential ablation of material with different

mask geometries requires the fast change of the

masks within the laser beam. For this a mask wheel

was developed, shown in Figure 2.

Figure 2: Mask wheel (red: smallest mask, white: direction

of rotation).

Figure 3: Experimental setup consisting of: linear stage (1),

stepper motor (2), 33:1 gear (3), Hall sensor (4) and mask

wheel (5).

The coated substrates are again held using three

laser-cut plates: a front and back plate with holes of

4 mm diameter and an intermediate plate with holes

of 5 mm diameter. These 4 mm holes are responsible

for the fixed outline of each micro-optic and are as

such the before mentioned constant radius aperture.

The holes in the mask wheel are placed on a circle

with a radius of 50 mm.

The mask wheel is driven by a servo motor with a

33:1 gear. The used motor encoder has a precision of

4000 increments per revolution. Furthermore, a Hall

sensor is added to reference the rotary movement

(homing). Now the center of each mask can be moved

precisely to the same position within the laser beam.

This is important for the position of the ablation areas,

and therefore for the geometry of the target structure.

The whole assembly, shown in Figure 3, is placed on

a linear stage, which is used for adjusting the wheel

position to the laser beam height.

2.4 Calculation of the Process

Parameters

In principle, two different process methods are

possible. In method 1, the mask wheel is rotated

during the structuring process at a speed that is

synchronised with the laser pulse repetition rate.

Thus, a micro-optic can be completed in about 0.25 s

if all 50 masks are used, and the laser emits at its

maximum pulse repetition frequency of 200 Hz.

However, this method requires a mask set specially

made for the micro-optics to be structured. This mask

set then enables the fast industrial production of

identical lenses.

In method 2, the rotary motion is stopped at each

mask position and the calculated number of laser

pulses needed to form the target structure is applied.

Due to the acceleration and deceleration of the mask

wheel the processing times are about one minute per

micro-optic, which is significantly slower than

method 1. On the other hand, this second method is

more flexible since a mask can be used several times

and thus more masks with different diameters fit into

the mask wheel. Since many different micro-lenses

are to be structured, the second method is used for this

study.

The number of laser pulses and the necessary set

of masks are calculated using a variance comparison.

The starting point of each calculation is the target

radius of curvature (ROC) and the given laser pulse

fluence which determines the ablation depth per laser

pulse ∆𝑧. As the lens is rotationally symmetrical the

calculation can be simplified to two dimensions. The

centre of the lens is always at a radius of r = 0 μm.

The target profile is then defined by a circle equation

with the target ROC. The calculation of the actual

profile starts with the radius value r

m,1

corresponding

to the first mask in the mask set being used. The

required ablation depth for each mask radius r

is the

difference between the profile depth for the current

Developing a Sequential Mask Projection Technique for Micro-Lens Generation Using Excimer Laser Micro-Structuring

mask radius, e.g. r

m,1

, and the profile depth for the

previous mask radius, e.g. r

m,0

. The number of laser

pulses results from the integer rounded ratio of the

necessary ablation depth to follow the target profile

and the ablation depth per laser pulse ∆𝑧. The result

of this step-by-step calculation procedure is shown as

an example in Figure 4.

Figure 4: Exemplary result of a variance comparison

calculation for a given target profile.

3 RESULTS

We used laser pulse fluences from H = 0.5 J/cm

up to

2.0 J/cm

and target ROC from 50 μm up to 250 μm to

determine the optimal parameter set for each target

structure. In principle, the setup described in the

previous chapter enables the generation of micro-

lenses, as demonstrated by the example in Figure 5.

Figure 5: Scanning electron microscope picture of a micro-

lens with H = 1.0 J/cm

and a ROC of 250 μm.

After the structuring process the micro-lenses are

measured with a laser scanning microscope. The

height data is then analysed using a python program

that fits a sphere to the data with the radius as the

variable fit parameter. This radius is the measured

ROC. Each parameter set was structured three times

to evaluate the process stability. In the following

figures every point represents the result for one

micro-lens.

Figure 6: Comparison of the measured ROC to the target

ROC of the lens structures for different laser pulse fluences.

The magenta line indicates the ideal ROC.

Figure 6 shows that the measured ROC of the

structures with H = 1.0 J/cm

deviate the least from

the target ROC with one structure from each

parameter set almost exactly showing the target ROC.

For the other laser pulse fluences the measured ROC

is significantly smaller. The ratio between measured

and target ROC remains approximately constant for

all parameter combinations: H = 0.5 J/cm

: 0.75;

H = 1.5 J/cm

: 0.84; H = 2.0 J/cm

: 0.88. These

constant deviations point to an error regarding the

laser pulse fluence measurement which could be

minimised by applying a correction factor for each

laser pulse fluence. This factor can be iteratively

adjusted by structuring a series of lenses with

different target ROC and calculating the ROC

deviation.

Figure 7: Standard deviation of each spherical fitted ROC

for every structured micro-lens.

The calculation of the standard deviation for each

fitted sphere, as shown in Figure 7, can be used as an

PHOTOPTICS 2024 - 12th International Conference on Photonics, Optics and Laser Technology

indicator for the shape accuracy of each lens. It can be

noticed that larger target ROC values result in lower

standard deviations. The lenses structured with

H = 1.0 J/cm

generally show the lowest standard

deviations meaning that they correspond most closely

to the shape of an ideal sphere. The lenses with

H = 1.5 J/cm

and H = 2.0 J/cm

show values of

standard deviation that are just a bit higher. The

H = 0.5 J/cm

lenses generally show the highest

standard deviations. In addition to being the farthest

away from the target ROC, this laser pulse fluence is

thus not suitable for structuring useful micro-lenses.

Figure 8: Scanning electron microscope picture of a micro-

lens with H = 1.0 J/cm

and a ROC of 50 μm. The red arrow

points at the clearly visible chipping.

Small values of target ROC result in high standard

deviations, because those lenses tend to chip making

them useless as an optical device. An exemplary

micro-lens is shown in Figure 8. The chipping is also

the reason for the measured ROC deviation for the

smallest target ROC of 50 μm and 70 μm, as shown

in Figure 9.

Figure 9: Deviation of the measured ROC from the target

ROC for different laser pulse fluences.

However, the chipping does not occur in any

micro-lens with a ROC of 90 μm and larger. This

structuring method can therefore be used to produce

micro-lenses with a radius of curvature ≥ 90 μm.

Figure 9 again shows that the laser pulse fluence of

H = 1.0 J/cm

is most suited for the generation of

micro-lenses closest to a given target ROC. To further

analyze these lenses regarding the repeatability of the

structuring process the deviation from the mean

measured ROC for each target ROC can be

calculated. The result is shown in Figure 10. The

lenses with a target ROC of 70 μm and 190 μm show

the largest deviation with 18 μm and 16 μm

respectively. The smallest deviation occurs with the

lenses with the largest target ROC of 230 μm and

250 μm with 3 μm each. This corresponds to a

deviation from the mean measured ROC of about 1 %

which indicates a good repeatability of the structuring

results for the respective process parameters.

Figure 10: Deviation from the mean measured ROC for

each target ROC using a laser pulse fluence of

H = 1.0 J/cm

. The magenta line indicates an ideal deviation

of 0 μm.

The laser pulse fluence of H = 1.0 J/cm

also

results in the smallest overall root mean square

surface roughness values at around S

= 80 nm, as

shown in Figure 11. This value is obtained by

convolving the height data with a gaussian filter using

a standard deviation value corresponding to a defined

cutoff wavelength of 𝜆



= 8 μm. Overall S

decreasing by increasing target ROC. The reason for

this is that fewer laser pulses per mask position are

required to produce micro-optics with a larger ROC.

The surface roughness for all target ROC greater than

170 μm is generally below 130 nm which in relation

to the design wavelength for optical data transmission

of 1310 nm equals 𝜆/10. The so far best shape lenses

are those structured with the laser pulse fluence of

H = 1.0 J/cm

. To further analyse their surface

roughness different cutoff wavelengths can be used

for the calculation, as shown in Figure 12 with

𝜆



= (2.5; 8.0; 25) μm. The smaller the cutoff value

the lesser the roughness. For the ROC of 250 μm the

three roughness values for the corresponding cutoff

Developing a Sequential Mask Projection Technique for Micro-Lens Generation Using Excimer Laser Micro-Structuring

wavelengths of (2.5; 8.0; 25) μm are (38; 74; 172) nm

respectively. 38 nm is less than 𝜆/30 in relation to the

design wavelength.

Figure 11: Calculated surface roughness S

for every

structured lens using a cutoff wavelength of 8 μm.

Yet another way of assigning a roughness value to

each structure is already given in Figure 7 through the

standard deviation of each spherical fitted ROC.

These values can be interpreted as the roughness that

remains when the ideally fitted lens shape is

subtracted from the measured height data as the

formula for the standard deviation is identical to the

formula for surface roughness. But these roughness

values do not involve a specific cutoff wavelength,

they are rather an indication of the wavefront error a

plane wave would get when passing through the

micro-lens.

Ultimately the calculation and the needed surface

roughness value is dictated by the application the

micro-optics are used for.

Figure 12: Calculated surface roughness S

for all lenses

structured with a laser pulse fluence of H = 1.0 J/cm

using

three different cutoff wavelengths.

Regarding a process optimisation, there are two

possible starting points. Firstly, we detected a mask

misalignment error while evaluating the generated

micro-lenses, as shown exemplary in Figure 13. This

error can be a result of backlash in the motor gearbox

which could be solved by attaching the motor encoder

directly onto the mask wheel. The error could also be

caused due to misalignment during the fabrication of

the mask wheel or during the mounting of the mask

wheel to the motor axis. Both could result in the masks

not being concentric to the motor axle which would

explain the mask misalignment. Solving this problem

would lead to lenses that are closer to the ideal shape

of a sphere and simultaneously also smoother,

depending on the used roughness calculation method.

Figure 13: Mask misalignment error. The red circles

indicate the inner edge of two ablation areas that are not

ideally concentric.

Secondly, the fluorine laser slowly loses power

while not emitting at a its maximum pulse repetition

frequency of 200 Hz. As the mask wheel has to stop

at each mask position to wait until the necessary

number of laser pulses is applied, the effective pulse

repetition frequency is much lower than 200 Hz. As a

result, the laser pulse fluence slowly decreases with

increasing duration of the structuring process. This

has a particular effect on the ablation areas with the

largest inner radius because these are processed last.

Therefore, once an optimal parameter set for a

specifically needed target ROC is found, the next step

is to reduce the total process time by using only the

necessary masks and applying process method 1 as

described in section 2.4. This should further decrease

the local deviations from the ideal lens shape as the

laser can now operate close to or even at its maximum

pulse repetition frequency.

4 CONCLUSIONS AND

OUTLOOK

The combination of sequential mask projection and

fluorine laser micro-structuring enables the generation

PHOTOPTICS 2024 - 12th International Conference on Photonics, Optics and Laser Technology

of rotationally symmetrical micro-optics. For this

purpose, the necessary masks were produced and the

experimental setup with a rotating mask wheel was

built. Each micro-optic to be structured is defined by

the used laser pulse fluence and a structure parameter

such as the target radius of curvature of a lens

geometry. The required mask sequence and the

number of laser pulses per mask are calculated using

a variance comparison. The evaluation of the

structures produced with the calculated parameter

sets shows that the calculation method leads in

principle to the generation of micro-lenses close to

the target geometries. The measured radii of

curvature of the micro-lenses produced with a laser

pulse fluence of H = 1.0 J/cm

deviate the least from

the respective target radius of curvature. One

structure from each parameter set almost exactly

matches the predefined target radius of curvature. The

surface roughness S

of those structures decreases

with larger target radius of curvature. The smallest

calculated S

values are around 80 nm using the cutoff

wavelength of 𝜆



= 8 μm.

Our next studies aim to reduce the process time

per micro-optic with a different motor-gear-

combination as well as the surface roughness by

applying a laser smoothing process as an

aftertreatment.

REFERENCES

Bückmann, T., Schittny, R., Thiel, M., & Kadic, M. (2014).

On three-dimensional dilational elastic metamaterials.

New Journal of Physics, 16(3).

Buettner, S., Pfeifer, M., & Weissmantel, S. (2019).

Manufacturing of Cylindrical Micro Lenses and Micro

Lens Arrays in Fused Silica and Borosilicate Glass

using F2-Laser Microstructuring. In M. Raposo, P. A.

Ribeiro, & D. Andrews (Eds.), PHOTOPTICS 2019

(pp. 66–72). Setúbal, Portugal: SCITEPRESS - Science

and Technology Publications Lda.

Buettner, S., Pfeifer, M., & Weissmantel, S. (2020).

Fabrication of Micro Spiral Phase Plates in Fused Silica

using F2-Laser Microstructuring. In P. Albella (Ed.),

PHOTOPTICS 2020 (pp. 114–121). Setúbal:

SCITEPRESS - Science and Technology Publications

Lda.

Hua, J.-G., Liang, S.-Y., Chen, Q.-D., Juodkazis, S., & Sun,

H.-B. (2022). Free ‐ Form Micro ‐ Optics Out of

Crystals: Femtosecond Laser 3D Sculpturing.

Advanced Functional Materials, 32(26).

Kim, J. Y., Brauer, N. B., Fakhfouri, V., & Boiko, D. L.

(2011). Hybrid polymer microlens arrays with high

numerical apertures fabricated using simple ink-jet

printing technique. Optical Materials Express, 1(2),

259.

Moore, S., Gomez, J., & Lek, D. (2016). Experimental

study of polymer microlens fabrication using partial-

filling hot embossing technique. Microelectronic

Engineering, 162, 57–62.

Sohn, I.-B., Choi, H.-K., Noh, Y.-C., Kim, J., & Ahsan, M.

S. (2019). Laser assisted fabrication of micro-lens array

and characterization of their beam shaping property.

Applied Surface Science, 479, 375–385.

Zhao, L., Cheng, J., Yin, Z., Yang, H., Liu, Q., Tan, C., et

al. (2022). Rapid CO 2 laser processing technique for

fabrication of micro-optics and micro-structures on

fused silica materials. Proceedings of the Institution of

Mechanical Engineers, Part B: Journal of Engineering

Manufacture, 236(1-2), 100–110.

Developing a Sequential Mask Projection Technique for Micro-Lens Generation Using Excimer Laser Micro-Structuring