Optimisation of Ceramic Kiln Loading Problem Using Multi-Objective

Genetic Algorithm

Derya Deliktas¸

and Ays¸e Kaygısız

utahya Dumlupınar University, Faculty of Engineering, Department of Industrial Engineering, K

utahya, Turkey

Keywords:

Ceramic Kiln Loading Problem, Ceramic Industry, Genetic Algorithm, Multi-Objective Optimisation,

Manufacturing Efﬁciency.

Abstract:

Efﬁcient resource utilisation is paramount for boosting productivity and competitiveness within industrial

contexts. In ceramic manufacturing, the Ceramic Kiln Loading Problem is critical, wherein the optimal ar-

rangement of ceramic products within kilns signiﬁcantly inﬂuences production efﬁciency. This study aims to

enhance efﬁciency by maximising the utilisation of the oven vehicle through optimal loading of the ordered

products. To achieve this objective, the Genetic Algorithm has been integrated with weighted sum and conic

scalarisation methods, and the results obtained from each method have been compared. Additionally, since

the algorithm’s parameters can signiﬁcantly inﬂuence its performance, parameter tuning has been conducted

using the irace method. The ﬁndings corroborate the superiority of results obtained by integrating the Genetic

Algorithm with weighted sum scalarisation.

1 INTRODUCTION

Cutting and packing represents two classic combina-

torial optimisation problems. Cutting problems in-

volve optimising the use of materials such as wood,

steel, and cloth, while packing problems focus on

maximising the use of available packing space. Ef-

ﬁcient utilisation of material and transport capacities

is crucial in production and distribution processes,

as it contributes to the economic use of natural re-

sources. Cutting and packing problems share a com-

mon structure: a set of large objects and small items

are provided, deﬁned exhaustively in one, two, three,

or more geometric dimensions. The goal is to select

some or all of the small items, group them into one

or more subsets, and assign each subset to a large ob-

ject. This assignment must satisfy geometric condi-

tions, ensuring that all small items in a subset lie com-

pletely within the large object without overlap, while

optimising a given objective function (W

ascher et al.,

2007; Sheng et al., 2016).

The loading problem entails the efﬁcient place-

ment of a maximum quantity of identical rectangu-

lar boxes onto a single rectangular pallet. This chal-

lenge is also occasionally referred to as the manu-

https://orcid.org/0000-0003-2676-1628

https://orcid.org/0009-0002-9002-1229

facturer’s pallet loading problem (Silva et al., 2016).

The container loading problem (CLP), also known as

the packing problem, constitutes sub-problems of cut-

ting and packing problems (Hodgson, 1982). There

are two primary container loading problems (Dyck-

hoff, 1990). The ﬁrst problem involves loading ei-

ther the entire consignment or a portion thereof into

a single container. The aim is to optimise volume

utilisation by maximising the ﬁlled container vol-

ume or minimising any unused space within the con-

tainer. The second problem, known as the multiple

container loading problem, entails loading the entire

consignment into one or more identical containers.

The objective here is to minimise the total number of

containers required for the consignment (Lim et al.,

2012). When the primary aim is space utilisation, this

problem essentially reﬂects the pallet packing prob-

lem, which involves loading boxes onto pallets rather

than into containers. The primary distinction lies in

the fact that pallets do not offer lateral support for

the boxes. Given that the well-known knapsack prob-

lem is a component of the loading problem, the load-

ing problem belongs to the class of NP-hard prob-

lems (Terno et al., 2000).

This study focuses on the real-life problem of cor-

rectly loading ceramic tableware into a ceramic kiln

at speciﬁed temperatures within the ceramic industry.

The ceramic kiln loading problem is a variant of the

248

Delikta¸s, D. and Kaygısız, A.

Optimisation of Ceramic Kiln Loading Problem Using Multi-Objective Genetic Algorithm.

DOI: 10.5220/0012807100003753

Paper published under CC license (CC BY-NC-ND 4.0)

In Proceedings of the 19th International Conference on Software Technologies (ICSOFT 2024), pages 248-257

ISBN: 978-989-758-706-1; ISSN: 2184-2833

pallet loading problem. Due to the signiﬁcant impact

of loading on product quality, particularly in produc-

tions catering to the ceramic sector where the number

and variety of items are extensive, the company takes

the loading problem seriously. The optimal utilisation

of shelves of various heights and dimensions placed

on the pallet to be inserted into the kiln is crucial due

to the extended baking time of the products in the kiln.

Additionally, the complexity of the problem is exac-

erbated by the heterogeneous sizes of the products to

be placed on the shelves, which prevent each product

from being placed on every shelf due to these sizes.

Each ceramic tableware item has priorities regarding

the places they can be assigned to on the shelves. In

this study, these assignment priorities have been con-

sidered to reduce deformations caused by products

touching each other and being placed on the wrong

shelf. Furthermore, maximising the total proﬁts of the

products placed in the kiln is also aimed. For this pur-

pose, it is aimed to fully load the kiln tool (or pallet)

by calculating the maximum score of the products to

be assigned to the shelves on the kiln tool, considering

their priorities, and maximising the total proﬁt calcu-

lated by considering the unit proﬁts of the products

assigned to the kiln. A multi-objective genetic algo-

rithm is proposed to solve this problem. The genetic

algorithm (GA) is combined with the weighted-sum

(WSM) and the conic scalarisation method (CSM).

Since the parameters of the proposed algorithm will

affect the results, the irace method is used for param-

eter tuning. The results obtained from GA with WSM

are compared with the results obtained from GA with

CSM. A decision support system (DSS) has also been

designed.

The remainder of this paper is structured as fol-

lows: Section 2 offers an overview of the literature.

In Section 3, the problem is described. Section 4 de-

tails the proposed approaches. Section 5 presents the

ﬁndings, and ﬁnally, Section 6 provides a summary of

the paper.

2 LITERATURE SURVEY

The CLP involves determining the optimal arrange-

ment and placement of cargo items within rectangu-

lar containers to maximise speciﬁc objectives. Con-

straints primarily revolve around container capacity in

terms of volume, weight or dimensions, weight distri-

bution, cargo orientation, loading order, and stability.

The problem may pertain to a single container or mul-

tiple containers, which can be homogeneous or het-

erogeneous (V

elez-Gallego et al., 2020). Objectives

include:

- maximising capacity utilisation (Dereli and

Sena Das, 2010; Ramos et al., 2018),

- minimising cost (Tian et al., 2016),

- minimising container count (Toffolo et al., 2017),

- maximising proﬁt (Liu et al., 2016),

- optimising space utilisation or replenishment

time (Y

uceer and

Ozakc¸a, 2010).

Given its NP-hard complexity (Young-Gun and

Kang, 2001; Zhou and Liu, 2017), exact methods face

limitations in effectively addressing the CLP (Chen

et al., 1995; Wu et al., 2010; Junqueira et al.,

2012; Paquay et al., 2016). Consequently, there

has been a proliferation of alternative methodolo-

gies such as heuristics (Toffolo et al., 2017), meta-

heuristics (Zhang et al., 2009; Zheng et al., 2015;

Ozdemir et al., 2022), and hybrid approaches (Dereli

and Sena Das, 2010; Rom

ao et al., 2012) in solving

the CLP.

In this study, we consider all practical constraints

mandated by ceramic industry and aim to furnish so-

lutions within a computational time frame of average

one minute. Experimental results show that the pro-

posed multi-objective algorithm, which includes max-

imising both total priority score and total proﬁt objec-

tives, can efﬁciently solve the practical ceramic kiln

loading problem.

3 PROBLEM DEFINITION

In a study conducted in a ceramic industry, an optimi-

sation study was carried out by placing the products

on shelves on pallets in the ceramic baking oven in the

glazing unit, in order to respond to the demands in the

most appropriate way. In this study, we can deﬁne the

assignment of i ceramic products to the racks on the

pallet to be loaded into the kiln as P = p

, ..., p

and

the j racks to be assigned as R = r

, ..., r

. The racks to

which each product can be assigned and their assign-

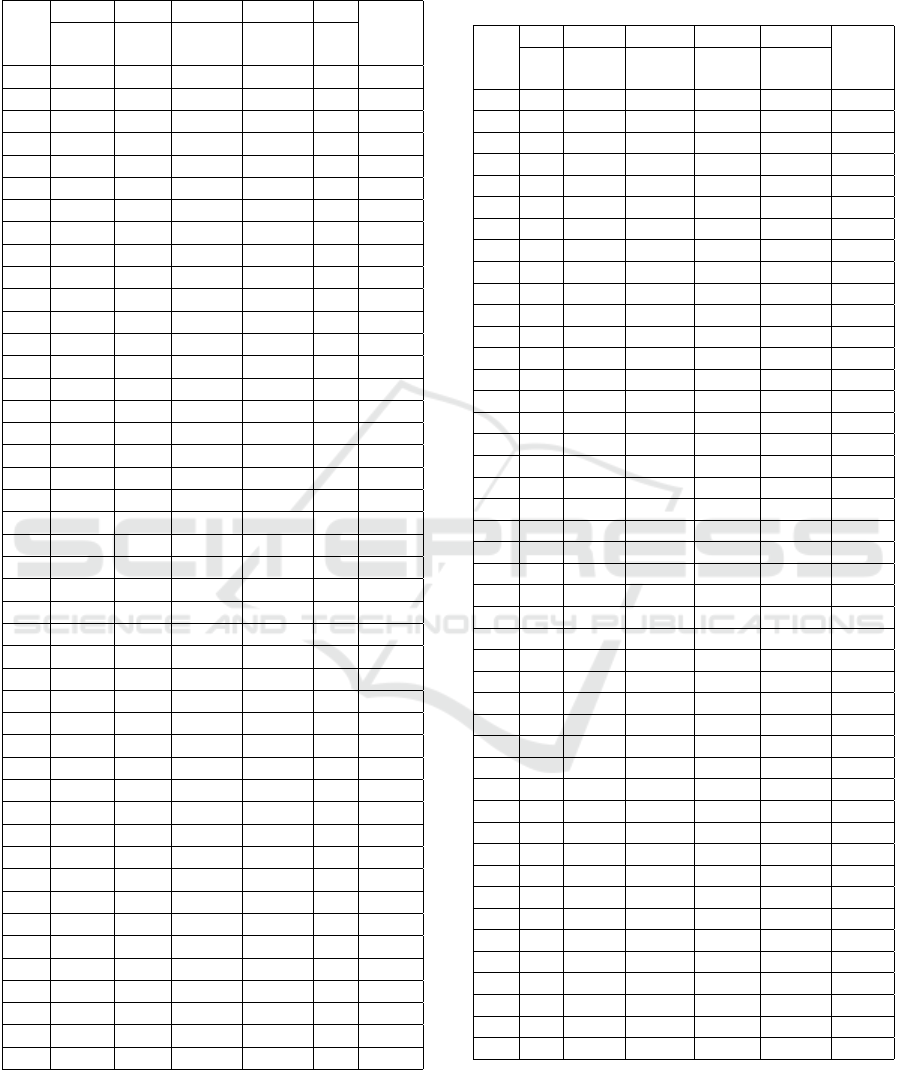
ment priorities are given in Table 3. According to this

dataset, there are a total of twelve racks (or kiln trays)

of three different types on the pallet to be loaded into

a ceramic kiln, namely, 2 square racks (Figure 1(a)), 4

six-layer rectangular shelves (Figure 1(b)), and 6 ﬁve-

layer rectangular racks (Figure 1(c)). In addition to

these racks, the products can also be assigned on the

ﬂoor (Figure 1(d)). The part shown with a red square

in Figure 1(a) represents the top-side of the square

rack. Some products can only be assigned to this part.

A visual of the pallet to be loaded into the kiln

is provided in Figure 2. Pallet and racks on it used

for the ceramic kiln loading problem was generated

Optimisation of Ceramic Kiln Loading Problem Using Multi-Objective Genetic Algorithm

249

by using the ZW3D package (ZWCAD Software Co.,

Ltd., Guangzhou, China). Each product must be as-

signed to the speciﬁed racks in Table 3. The ex-

pression ‘I’ in the table implies assigning ﬁrst prior-

ity to the corresponding rack; ‘II’ implies assigning

second priority to the corresponding rack, and ‘III’

implies assigning third priority to the corresponding

rack. While assigning products to speciﬁed racks is

a hard constraint, assigning according to priority of

racks is a soft constraint.

a) SR b) 5LRR

c) 6LRR d) FR

Figure 1: Racks on pallet loaded into kiln.

Primarily, it is preferred to assign the product to

the ﬁrst priority given in Table 3. However, if the rack

belonging to the ﬁrst priority is full, assignments are

made according to other priorities in the table. In this

study, 1000 points are assigned to the ﬁrst priority,

300 points to the second priority, and 100 points to the

third priority. This scoring was determined through

brainstorming with company employees. In addition

to priorities in Table 3, the values in parentheses in-

dicate the maximum number of products that can be

assigned from the relevant product to the appropriate

rack.

For example, P

can be assigned to only two types

of racks, namely six-layer rectangular (6LRR) and

ﬁve-layer rectangular (5LRR) racks. It cannot be as-

signed to other rack types. A maximum of two P

can be assigned to these racks. Here, P

is expected

to be assigned to 6LRR as the ﬁrst priority and to

5LRR as the second priority. Since the dimensions

of each product and rack are different, assignments

were made considering the ﬂoor area of each prod-

uct and the rack space. In addition, the unit proﬁts

of each product are also given in this table. In this

Figure 2: A visual of the pallet to be loaded into the kiln.

study, it is assumed that 10 units of each product are

ordered. Additionally, in this study, it was planned to

assign the 169 products most ordered for the last six

months to the kiln vehicle. These products are baked

in the kiln at the same temperature. Finally, this study

considers only one kiln pallet.

4 MULTI-OBJECTIVE GENETIC

ALGORITHM

GA is an evolutionary algorithm developed by Hol-

land that seeks solutions to problems through a

stochastic approach (Holland, 1973). GA can con-

currently explore solutions or sets of solutions across

the solution space. As GA is an intuitive approach, it

may not ﬁnd exact solutions to the problem; however,

it can yield solutions near the optimum. Therefore, it

can provide acceptable solutions for loading and opti-

misation problems falling under the NP-hard class in

a reasonable time frame (Panchal and Panchal, 2015).

The objective functions of the examined problem

are: maximising the total score of the products as-

signed to the racks on the pallet ( f

(x)) and max-

imising the total of the unit proﬁts of these prod-

ucts ( f

(x)). Solving multi-objective problems ne-

cessitates the utilisation of multi-objective optimisa-

tion techniques. Among these methods, scalarisa-

tion methods are prominent. Scalarisation involves

converting a multi-objective problem into a single-

objective problem. In this study, we proposed multi-

objective genetic algorithm by integrating weighted-

sum and conic scalarisation methods to solve the ce-

ramic kiln loading problem.

Although the weighted-sum method (WSM) is

recognised as the most popular, it does not ensure

the acquisition of all Pareto-optimal solutions in non-

convex regions owing to the linear combination of

its objectives (Miettinen, 2012). The conic scalar-

isation method (CSM) outlined by Gasimov facili-

tates Pareto-front via cones instead of the hyperplanes

ICSOFT 2024 - 19th International Conference on Software Technologies

250

employed in WSM, without necessitating convex-

ity assumptions (Gasimov, 2001; Kasimbeyli, 2013).

The proposed multi-objective model, scalarised using

WSM (Eq. (1)) and CSM (Eq. (2)) respectively, uses

the following calculation:

max WSM(x) = w

(x) + w

(x) (1)

max CSM(x) = w

( f

(x) − R f

) + w

( f

(x) − R f

α [ | f

(x) − R f

| + | f

(x) − R f

|| ]

(2)

where w

, w

> 0 are the importance degrees of the

total score ( f

(x)) and the total unit proﬁt ( f

(x)), re-

spectively. α is utilised to form a cone by adjusting

the angle of a cone surface, 0 ⩽ α < min

{

, w

}

R f

and R f

denote reference points that can be de-

termined by a decision maker for the objective values

in this study.

The objective function values are normalised by

dividing them by the maximum value of the corre-

sponding objective function because different objec-

tive functions may have varying magnitudes (Marler

and Arora, 2005). The normalised objective function

values are utilised as the ﬁtness value in the proposed

multi-objective genetic algorithm with the scalarisa-

tion methods. In these equations, the ideal value of

each objective represents the maximum value among

all solutions examined so far. The maximum value of

the corresponding objective is established as the ideal

point based on the 31 runs by setting w

= 1 (w

= 0)

and w

= 1 (w

= 0), respectively. The nadir value

is determined as the maximum value of each objec-

tive among the obtained results for the same objective

weights. I

denotes the ideal value of the kth objective

(k = 1, 2). The ﬁtness values scalarised by WSM and

CSM are normalised as depicted in Eqs. (3)-(4):

max WSM

Norm

(x) =



(x)

+ w

(x)



(3)

max CSM

Norm

(x) =





( f

(x) − R f





( f

(x) − R f





|( f

(x) − R f

|( f

(x) − R f



(4)

Algorithm 1 illustrates the solution of the multi-

objective GA approach for the ceramic kiln loading

problem.

Data: Table 3

Result: The best solution

Randomly generate an initial population;

Compute the ﬁtness of each individual (see

Eqs. (3-4));

while termination criteria not satisﬁed do

Choose parents from the population ←

(PopSize, TourSize);

Perform crossover to produce offspring

← (OOX, parents);

Perform mutation operator ← (SPM,

offspring);

Compute the ﬁtness of each individual

(see Eqs. (3-4));

Apply elitism operator;

end

Algorithm 1: Pseudo-code of the proposed multi-objective

algorithm.

4.1 Chromosome Representation and

Fitness Function Evaluation

A chromosome fundamentally represents a sequence

of genes, serving as a candidate solution for a given

problem. Depending on the problem, it can possess a

specialized structure such as one-dimensional, multi-

dimensional, or a tree data structure.

Figure 3: An illustration of the chromosome representation.

In this study, the chromosome structure utilised is

implemented as permutation encoding as illustrated

in Figure 3. The length of the chromosome corre-

sponds to the total number of products. As the con-

straint of the problem arises from the total area of the

racks on the pallet, the algorithm is designed to calcu-

late the number of products that will ﬁll the rack with

the highest priority to optimise the objective function.

Once the chromosome structure is established, allo-

cation begins by considering the ﬁrst priorities of the

racks from which each product can be assigned un-

der the constraint of the rack area and product area.

If the rack with the ﬁrst priority for a product has

already been occupied due to a previously assigned

product, a similar process is applied to other priori-

ties. This process is repeated for each order quantity

of each product. If all the racks to which a product

can be assigned, according to the dataset in Table 3,

are occupied, then the remaining order of that product

may not be assigned or only partially assigned. Sub-

sequently, a similar process begins for the following

gene representing a product. Thus, this process con-

Optimisation of Ceramic Kiln Loading Problem Using Multi-Objective Genetic Algorithm

251

tinues until the total rack area is ﬁlled. If the total area

is ﬁlled, other products in the chromosome structure

are not considered. Up to that point, the unit proﬁts of

the listed products are calculated, resulting in the to-

tal unit proﬁt calculation. The total score is computed

considering the priority scores of the racks assigned

to the products in the same list. This process is com-

pleted the calculation of each objective.

4.2 Genetic Operators

Genetic operators play a crucial role in promoting di-

versity within the population and are fundamental in

addressing the research problem. Speciﬁcally, mu-

tation, elitism, selection, and crossover operators are

tailored to provide viable solutions.

Crossover operator: the crossover operator facil-

itates the generation of improved chromosomes by

enabling gene exchange. This study applied order-

based one-point crossover (OOX) operator to the pro-

posed algorithm. Firstly, a random crossover point is

selected on the chromosome. Subsequently, all ge-

netic material in the parents up to the crossover point

is duplicated into two offspring. The products pre-

viously allocated are removed from the other parent.

Then, the remaining genetic material from each par-

ent is copied into the offspring without altering their

sequence (Ruiz et al., 2006; Deliktas¸ et al., 2021).

Mutation operator: the primary objective of the

mutation operator is to maintain population diversity.

The proposed algorithm incorporates a Swap (SPM)

operator (Deliktas¸, 2022; Deliktas¸ and Ustun, 2023).

SPM involves exchanging two operations and their

machine assignments randomly selected while pre-

serving the routing of operations across machines.

Selection operator: the selection operator plays

a crucial role in ensuring the survival probability of

the best individuals. Various standard selection oper-

ators, including roulette wheel selection, rank-based

selection, tournament selection, and seed selection,

are documented in the literature. At this stage, tourna-

ment selection is preferred due to its superior conver-

gence properties and manageable computational com-

plexity.

Elitism operator: it guarantees the preservation of

the ﬁttest chromosomes from one generation to the

next, thereby safeguarding their advantageous charac-

teristics following crossover and mutation operations.

5 COMPUTATIONAL RESULTS

This section presents the experimental results ob-

tained from the proposed multi-objective algorithm to

illustrate its robustness and effectiveness. We con-

duct 31 independent runs to compute the statistical

outcomes of the algorithms. All methodologies are

implemented using C# 2022, and the experiments are

conducted on a laptop equipped with a 2.1 GHz Core

i7 CPU and 16.00 GB RAM. The algorithm termi-

nates once the maximum number of ﬁtness evalua-

tions, which is a factor of the population size, is ex-

ceeded.

5.1 Parameter Tuning

The proposed approach employs the Iterated F-Race

algorithm (irace), an automated parameter conﬁgura-

tion tool (L

opez-Ib

nez et al., 2016). This algorithm

is an iterated version of the F-Race parameter setting

approach (Birattari et al., 2010), which operates on

a competition-based principle. This tool reﬁnes pa-

rameter values by conducting successive races, where

each race evaluates various candidate conﬁgurations

across different problem instances. Through the ap-

plication of the Friedman test, conﬁgurations demon-

strating signiﬁcantly poorer performance are identi-

ﬁed and eliminated iteratively. Winners from each

race contribute to generating improved candidate con-

ﬁgurations for subsequent iterations. The outcome

of this iterative process yields the optimal parameter

conﬁguration for the algorithm under examination.

Table 1: Ranges of the parameters for the proposed multi-

objective algorithm and the best parameter conﬁguration

obtained with irace.

Parameter Type Range Selected value

PopSize Integer [20, 100] 79

TourSize Integer [2, 10] 9

CrossProb Real [0.7, 1] 0.74

MutaProb Real [0.01, 0.5] 0.01

In order to facilitate parameter adjustment utilis-

ing irace, it is imperative to delineate the parameters

slated for tuning, establish their permissible ranges,

and delineate the set of instances earmarked for the

tuning process. Table 1 outlines the selected pa-

rameters earmarked for tuning and their respective

ranges tailored for the proposed algorithm. The pro-

posed algorithm based on scalarisation methods con-

tinues running until the termination criterion is satis-

ﬁed. This study deﬁnes the termination criterion as

the maximum ﬁtness evaluation number. This value

is derived by multiplying the total number of parts by

a constant. As shown in Figure 4, it is sufﬁcient to set

this value to 1000.

ICSOFT 2024 - 19th International Conference on Software Technologies

252

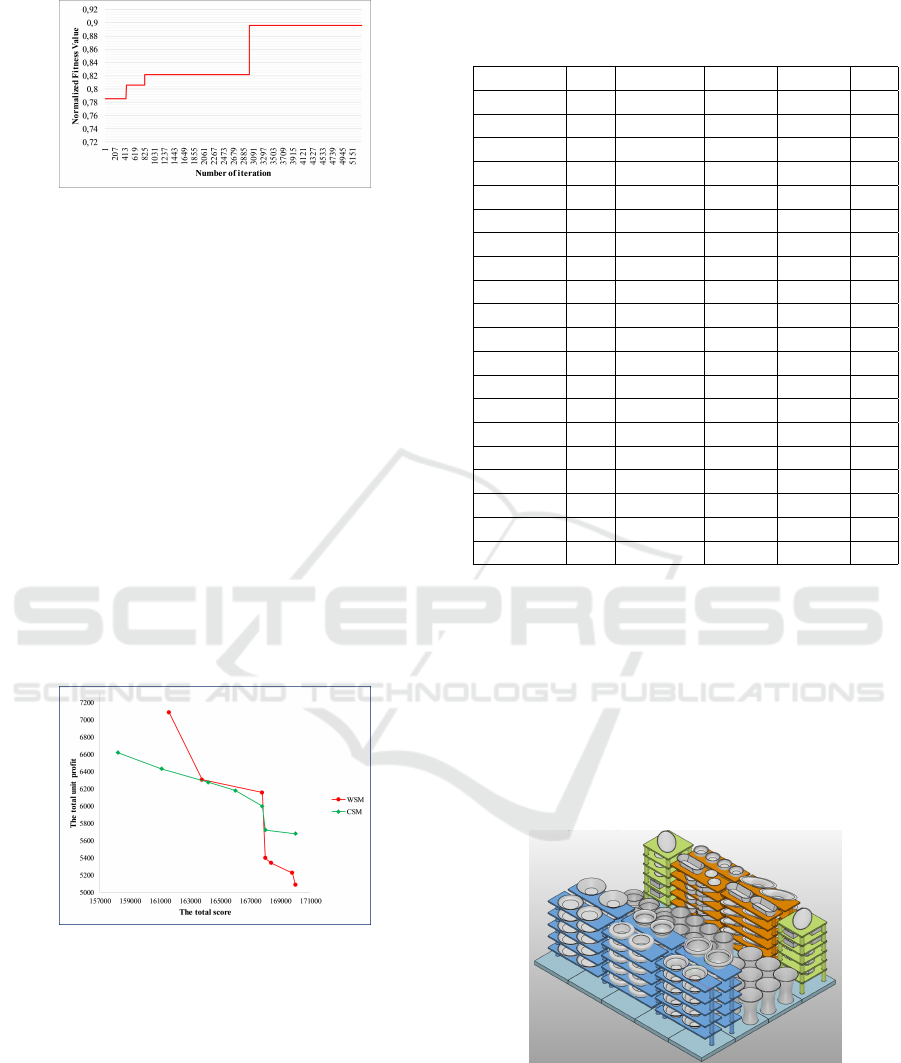
Figure 4: The convergence graph.

5.2 Results and Discussion

Utilising the aforementioned parameters, the perfor-

mance of the proposed multi-objective algorithm is

analysed and tested. The reference points employed

in CSM integrated with the GA are computed us-

ing the ideal points and nadir points of each objec-

tive. For the computation of the reference points,

a convex combination technique (Kim and Kim,

2006) is utilised to ensure well-distributed reference

points (Deliktas¸ and Ustun, 2023). Hence, differ-

ent reference point combinations are employed in this

study to compare the performance of each scalarisa-

tion method. Additionally, this study considers nine

different combinations of objective weights: [w

0.1;w

= 0.9], [w

= 0.2;w

= 0.8], [w

= 0.3;w

0.7], [w

= 0.4; w

= 0.6], [w

= 0.5; w

= 0.5], [w

0.6;w

= 0.4], [w

= 0.7;w

= 0.3], [w

= 0.8;w

0.2], and [w

= 0.9; w

= 0.1], respectively.

Figure 5: Pareto plot of the results obtained from WSM and

CSM.

We generated plots illustrating the Pareto fronts,

representing the set of non-dominated trade-off so-

lutions attained by each method. As shown in Fig-

ure 5, the GA with WSM mostly produces better re-

sults when compared to the GA with CSM.

The best solution obtained from the proposed

multi-objective algorithm is presented in Table 2.

Based on this table, a total of 185 tableware items

from 20 different products have been assigned to the

racks on the pallet. The occupancy rate of the kiln

pallet is 100%. The total objective value for the as-

Table 2: An illustration of the products assigned to the racks

on the pallet and the amount of assigned products according

to the result obtained from the best solution.

Products SR SR (TS) 6LRR 5LRR FR

— — 10 — —

— — 3 — —

— — — 10 —

— 2 — — —

— — — — 10

112

10 — — — —

125

10 — — — —

126

10 — — — —

158

— — 10 — —

159

— — 10 — —

164

— — 10 — —

SR: Square rack,

SR(TS): Square rack (top-side),

6LRR: Six-layer rectangular rack,

5LRR: Five-layer rectangular rack,

FR: Floor rack.

signed items on this pallet is 164,000, while the total

unit proﬁt objective value is 5205.5. Table 2 provides

information about each product assigned to each rack

and the order quantity of each product. According to

the results in Table 2, a sample visual of the fully oc-

cupied kiln pallet in Figure 6 was illustrated.

Figure 6: An illustration of the ceramic kiln loading ac-

cording to the best solution based on the proposed multi-

objective algorithm.

The conceptual design and enhancement of a De-

cision Support System (DSS) for strategic planning

are crucial for overseeing operational activities within

a ceramic industry system. Figure 7 illustrates the ap-

Optimisation of Ceramic Kiln Loading Problem Using Multi-Objective Genetic Algorithm

253

plication of the proposed DSS through a real-world

problem within the ceramic industry.

Figure 7: Screenshots of the decision support system.

6 CONCLUSIONS

This study investigated the Ceramic Kiln Loading

Problem. Given the NP-hardness of the proposed

model, we proposed a multi-objective genetic algo-

rithm approach to load the pallet, aiming to max-

imise both total score and total unit proﬁt simulta-

neously. We developed simple DSS software based

on the multi-objective GA approach to obtain prompt

and high-quality solutions to problems. Furthermore,

we utilised an irace method to ﬁne-tune the parame-

ters of the GA.

There are numerous avenues for future research.

Various heuristic or metaheuristic approaches, includ-

ing ant colony optimisation, genetic algorithms, simu-

lated annealing, and tabu search, can be applied to this

model. Enhancements could be made by incorporat-

ing multi-objective considerations into the research.

Additionally, future studies may explore the inclusion

of multiple-kiln pallets.

ACKNOWLEDGEMENTS

This work was supported by the Scientiﬁc and Tech-

nological Research Council of Turkey (T

ITAK)

under the BIDEB 2209-B Industry Oriented Research

Project Support Programme for Undergraduate Stu-

dents with grant number 1139B412102110.

7 CRediT AUTHORSHIP

CONTRIBUTION STATEMENT

Derya Deliktas¸: Conceptualization, Methodology,

Software, Validation, Formal analysis, Investigation,

Writing - Original draft, Reviewing and Editing,

Project administration, Visualization. Ays¸e Kaygısız:

Data acquisition, Validation, Visualization.

REFERENCES

Birattari, M., Yuan, Z., Balaprakash, P., and St

utzle, T.

(2010). F-Race and iterated F-Race: An overview.

Experimental methods for the analysis of optimization

algorithms, pages 311–336.

Chen, C.-S., Lee, S.-M., and Shen, Q. (1995). An analytical

model for the container loading problem. European

Journal of operational research, 80(1):68–76.

Deliktas¸, D. (2022). Self-adaptive memetic algorithms

for multi-objective single machine learning-effect

scheduling problems with release times. Flexible Ser-

vices and Manufacturing Journal, 34(3):748–784.

Deliktas¸, D.,

Ozcan, E., Ustun, O., and Torkul, O. (2021).

Evolutionary algorithms for multi-objective ﬂexible

job shop cell scheduling. Applied Soft Computing,

113:107890.

Deliktas¸, D. and Ustun, O. (2023). Multi-objective genetic

algorithm based on the fuzzy multimoora method for

solving the cardinality constrained portfolio optimiza-

tion. Applied Intelligence, 53(12):14717–14743.

Dereli, T. and Sena Das, G. (2010). A hybrid simu-

lated annealing algorithm for solving multi-objective

container-loading problems. Applied Artiﬁcial Intelli-

gence, 24(5):463–486.

Dyckhoff, H. (1990). A typology of cutting and packing

problems. European journal of operational research,

44(2):145–159.

Gasimov, R. N. (2001). Characterization of the benson

proper efﬁciency and scalarization in nonconvex vec-

tor optimization. In Multiple criteria decision making

in the new millennium, pages 189–198. Springer.

Hodgson, T. J. (1982). A combined approach to the pallet

loading problem. IIE Transactions, 14(3):175–182.

Holland, J. H. (1973). Genetic algorithms and the opti-

mal allocation of trials. SIAM journal on computing,

2(2):88–105.

Junqueira, L., Morabito, R., and Yamashita, D. S. (2012).

Three-dimensional container loading models with

cargo stability and load bearing constraints. Comput-

ers & Operations Research, 39(1):74–85.

Kasimbeyli, R. (2013). A conic scalarization method in

multi-objective optimization. Journal of Global Op-

timization, 56(2):279–297.

Kim, J. and Kim, S.-K. (2006). A chim-based interac-

tive tchebycheff procedure for multiple objective de-

cision making. Computers & Operations Research,

33(6):1557–1574.

Lim, A., Ma, H., Xu, J., and Zhang, X. (2012). An iterated

construction approach with dynamic prioritization for

solving the container loading problems. Expert Sys-

tems with Applications, 39(4):4292–4305.

Liu, J., Smith, A. E., and Qian, D. (2016). The vehicle

loading problem with a heterogeneous transport ﬂeet.

Computers & Industrial Engineering, 97:137–145.

opez-Ib

nez, M., Dubois-Lacoste, J., C

aceres, L. P., Bi-

rattari, M., and St

utzle, T. (2016). The irace package:

Iterated racing for automatic algorithm conﬁguration.

Operations Research Perspectives, 3:43–58.

ICSOFT 2024 - 19th International Conference on Software Technologies

254

Marler, R. T. and Arora, J. S. (2005). Function-

transformation methods for multi-objective optimiza-

tion. Engineering Optimization, 37(6):551–570.

Miettinen, K. (2012). Nonlinear multiobjective optimiza-

tion, volume 12. Springer Science & Business Media.

Ozdemir, D., Deliktas¸, D., and Ermutaf, E. G. (2022).

A decision support system for multi-objective porce-

lain container loading problem based on genetic algo-

rithm. Academic Platform Journal of Engineering and

Smart Systems, 10(2):78–85.

Panchal, G. and Panchal, D. (2015). Solving np hard prob-

lems using genetic algorithm. Transportation, 106:6–

Paquay, C., Schyns, M., and Limbourg, S. (2016). A

mixed integer programming formulation for the three-

dimensional bin packing problem deriving from an air

cargo application. International Transactions in Op-

erational Research, 23(1-2):187–213.

Ramos, A. G., Silva, E., and Oliveira, J. F. (2018). A new

load balance methodology for container loading prob-

lem in road transportation. European Journal of Op-

erational Research, 266(3):1140–1152.

Rom

ao, O. C., dos Santos, A. G., and Arroyo, J. E. C.

(2012). A two phase hybrid method to support 2d

and 3d vehicle loading. In 2012 12th International

Conference on Intelligent Systems Design and Appli-

cations (ISDA), pages 320–325. IEEE.

Ruiz, R., Maroto, C., and Alcaraz, J. (2006). Two new ro-

bust genetic algorithms for the ﬂowshop scheduling

problem. Omega, 34(5):461–476.

Sheng, L., Hongxia, Z., Xisong, D., and Changjian, C.

(2016). A heuristic algorithm for container loading

of pallets with inﬁll boxes. European Journal of Op-

erational Research, 252(3):728–736.

Silva, E., Oliveira, J. F., and W

ascher, G. (2016). The

pallet loading problem: a review of solution methods

and computational experiments. International Trans-

actions in Operational Research, 23(1-2):147–172.

Terno, J., Scheithauer, G., Sommerweiß, U., and Riehme,

J. (2000). An efﬁcient approach for the multi-pallet

loading problem. European Journal of Operational

Research, 123(2):372–381.

Tian, T., Zhu, W., Lim, A., and Wei, L. (2016). The multiple

container loading problem with preference. European

Journal of Operational Research, 248(1):84–94.

Toffolo, T. A., Esprit, E., Wauters, T., and Berghe, G. V.

(2017). A two-dimensional heuristic decomposition

approach to a three-dimensional multiple container

loading problem. European Journal of Operational

Research, 257(2):526–538.

elez-Gallego, M. C., Teran-Somohano, A., and Smith,

A. E. (2020). Minimizing late deliveries in a truck

loading problem. European Journal of Operational

Research, 286(3):919–928.

ascher, G., Haußner, H., and Schumann, H. (2007).

An improved typology of cutting and packing prob-

lems. European journal of operational research,

183(3):1109–1130.

Wu, Y., Li, W., Goh, M., and De Souza, R. (2010). Three-

dimensional bin packing problem with variable bin

height. European journal of operational research,

202(2):347–355.

Young-Gun, G. and Kang, M.-K. (2001). A fast algorithm

for two-dimensional pallet loading problems of large

size. European Journal of Operational Research,

134(1):193–202.

uceer,

U. and

Ozakc¸a, A. (2010). A truck load-

ing problem. Computers & Industrial Engineering,

58(4):766–773.

Zhang, D.-F., Peng, Y., Zhu, W.-X., and Chen, H.-W.

(2009). A hybrid simulated annealing algorithm

for the three-dimensional packing problem. Chinese

Journal of Computers, 32(11):2147–2156.

Zheng, J.-N., Chien, C.-F., and Gen, M. (2015). Multi-

objective multi-population biased random-key genetic

algorithm for the 3-d container loading problem.

Computers & Industrial Engineering, 89:80–87.

Zhou, Q. and Liu, X. (2017). A swarm optimization al-

gorithm for practical container loading problem. In

IECON 2017-43rd Annual Conference of the IEEE In-

dustrial Electronics Society, pages 5690–5695. IEEE.

Optimisation of Ceramic Kiln Loading Problem Using Multi-Objective Genetic Algorithm

255

APPENDIX

Table 3: Dataset of real-life ceramic kiln loading problem.

i\ j

Unit

proﬁt

(TS)

6LRR 5LRR FR

I ( 2 ) II ( 2 ) 86.97

I ( 2 ) II ( 2 ) 47.25

I ( 2 ) II ( 2 ) 24.45

I ( 2 ) II ( 2 ) 15.75

I ( 2 ) II ( 2 ) 37.1

I ( 2 ) II ( 2 ) 25.22

I ( 2 ) II ( 2 ) 42.89

I ( 2 ) II ( 2 ) 5.31

I ( 2 ) II ( 2 ) 5

I ( 2 ) II ( 2 ) 3.84

I ( 2 ) II ( 2 ) 6.62

I ( 2 ) II ( 2 ) 5.21

I ( 2 ) II ( 2 ) 6.62

I ( 2 ) II ( 2 ) 11.82

I ( 2 ) II ( 2 ) 7.41

I ( 2 ) II ( 2 ) 9.45

I ( 2 ) II ( 2 ) 15.75

I ( 2 ) II ( 2 ) 23.63

I ( 2 ) 3.84

I ( 2 ) 6.62

I ( 2 ) 5.21

I ( 2 ) 6.62

I ( 2 ) 23.63

I ( 2 ) 6.32

I ( 2 ) 5.85

I ( 2 ) 7.52

I ( 2 ) 17.18

I ( 2 ) 13.7

I ( 2 ) 9

I ( 2 ) 29.78

I ( 2 ) 22.94

I ( 2 ) 15.41

II ( 2 ) I ( 2 ) 24.95

II ( 2 ) I ( 2 ) 15.41

II ( 2 ) I ( 2 ) 23.9

I ( 1 ) 23.9

II ( 2 ) I ( 2 ) 37.86

II ( 2 ) I ( 2 ) 42.24

II ( 2 ) I ( 2 ) 6.93

II ( 2 ) I ( 2 ) 5.09

II ( 2 ) I ( 2 ) 3.66

II ( 2 ) I ( 2 ) 5.06

II ( 2 ) I ( 2 ) 6.27

II ( 2 ) I ( 2 ) 7.49

Table 3: Dataset of real-life ceramic kiln loading problem

(cont.).

i\ j

Unit

proﬁt

(TS)

6LRR 5LRR FR

II ( 2 ) I ( 2 ) 7.83

I ( 1 ) 8.85

I ( 1 ) 11.61

I ( 1 ) 20.76

I ( 1 ) 31.01

I ( 1 ) 7.07

I ( 30 ) 13.26

I ( 30 ) 20.91

I ( 30 ) 10.95

I ( 30 ) 23.06

I ( 30 ) 17.04

I ( 30 ) 16.25

I ( 30 ) 29.07

I ( 30 ) 39.95

I ( 30 ) 53.79

I ( 30 ) 58.32

I ( 30 ) 5.81

I ( 30 ) 4.43

I ( 30 ) 6.62

I ( 30 ) 25.19

I ( 30 ) 7.41

I ( 30 ) 30.02

I ( 30 ) 5.81

I ( 30 ) 4.02

I ( 30 ) 5.58

I ( 30 ) 4.02

I ( 30 ) 9.32

I ( 30 ) 5.55

I ( 30 ) 14.93

I ( 30 ) 7.85

I ( 30 ) 8.6

I ( 30 ) 10.35

I ( 30 ) 12.81

I ( 30 ) 22.86

I ( 30 ) 7.13

I ( 30 ) 7.83

I ( 30 ) 8.85

I ( 30 ) 10.11

I ( 30 ) 36.68

I ( 30 ) 7.5

I ( 30 ) 5.76

I ( 30 ) 2.6

ICSOFT 2024 - 19th International Conference on Software Technologies

256

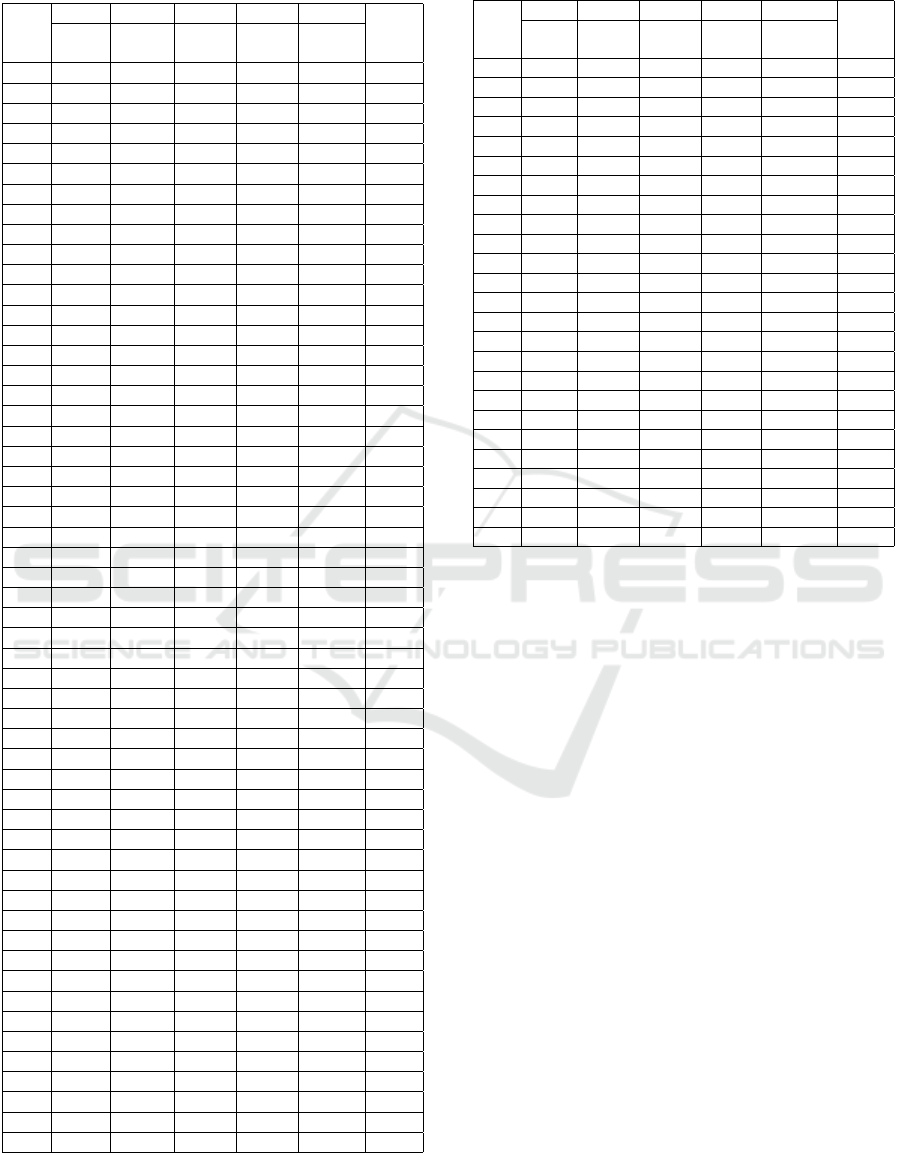
Table 3: Dataset of real-life ceramic kiln loading problem

(cont.).

i\ j

Unit

proﬁt

(TS)

6LRR 5LRR FR

I ( 30 ) 5.76

I ( 30 ) 3.84

I ( 30 ) 6.05

I ( 30 ) 9.42

I ( 30 ) 11.4

I ( 30 ) 10.83

I ( 30 ) 6.32

I ( 30 ) 11.93

100

I ( 30 ) 8.57

101

I ( 30 ) 9.42

102

I ( 30 ) 5.49

103

I ( 30 ) 5.49

104

I ( 30 ) 2.97

105

I ( 3 ) II ( 3 ) 10.37

106

I ( 3 ) II ( 3 ) 11.4

107

I ( 3 ) II ( 3 ) 10.37

108

I ( 3 ) II ( 3 ) 11.4

109

I ( 3 ) II ( 3 ) 6.65

110

I ( 3 ) II ( 3 ) 10.37

111

I ( 3 ) II ( 3 ) 8.46

112

I ( 3 ) II ( 3 ) 8.57

113

I ( 3 ) II ( 3 ) 5.49

114

I ( 3 ) II ( 3 ) 9.42

115

I ( 3 ) II ( 3 ) 6.05

116

I ( 3 ) II ( 3 ) 6.05

117

I ( 3 ) II ( 3 ) 11.4

118

I ( 3 ) II ( 3 ) 13.19

119

I ( 3 ) II ( 3 ) 7.7

120

I ( 3 ) II ( 3 ) 7.68

121

I ( 3 ) II ( 3 ) 11.15

122

I ( 3 ) II ( 3 ) 10.35

123

I ( 3 ) II ( 3 ) 11.04

124

I ( 3 ) II ( 3 ) 45.09

125

I ( 3 ) II ( 3 ) 51.8

126

I ( 3 ) II ( 3 ) 95.55

127

I ( 2 ) II ( 2 ) 19.62

128

I ( 2 ) II ( 2 ) 27.32

129

I ( 2 ) II ( 2 ) 24.23

130

I ( 2 ) II ( 2 ) 34.05

131

I ( 2 ) II ( 2 ) 14.39

132

I ( 2 ) II ( 2 ) 32.58

133

I ( 2 ) II ( 2 ) 90.21

134

I ( 2 ) II ( 2 ) 50.43

135

I ( 2 ) II ( 2 ) 22.92

136

I ( 2 ) II ( 2 ) 27.32

137

I ( 2 ) II ( 2 ) 42.06

138

I ( 2 ) II ( 2 ) 45.86

139

I ( 2 ) II ( 2 ) 17.42

140

I ( 1 ) II ( 1 ) 20.84

141

I ( 1 ) II ( 1 ) 41.67

142

I ( 1 ) II ( 1 ) 11.82

143

I ( 1 ) II ( 1 ) 23.63

144

I ( 1 ) II ( 1 ) 18.48

Table 3: Dataset of real-life ceramic kiln loading problem

(cont.).

i\ j

Unit

proﬁt

(TS)

6LRR 5LRR FR

145

I ( 1 ) II ( 1 ) 57.95

146

I ( 1 ) II ( 1 ) 15.63

147

I ( 1 ) II ( 1 ) 19.14

148

I ( 1 ) II ( 1 ) 50.97

149

I ( 1 ) II ( 1 ) 68.15

150

I ( 1 ) II ( 1 ) 7.22

151

I ( 1 ) II ( 1 ) 5.21

152

I ( 1 ) II ( 5 ) 5.21

153

I ( 1 ) II ( 5 ) 7.41

154

I ( 1 ) II ( 5 ) 9.53

155

I ( 1 ) II ( 5 ) 22.83

156

I ( 1 ) II ( 5 ) 15.03

157

I ( 1 ) II ( 5 ) 46.86

158

I ( 3 ) II ( 4 ) III ( 30 ) 20.58

159

I ( 3 ) II ( 4 ) III ( 30 ) 5.21

160

I ( 3 ) II ( 4 ) III ( 30 ) 15.75

161

I ( 3 ) II ( 4 ) III ( 30 ) 7.41

162

I ( 3 ) II ( 4 ) III ( 30 ) 5.21

163

I ( 3 ) II ( 4 ) III ( 30 ) 8.15

164

I ( 3 ) II ( 4 ) III ( 30 ) 6.48

165

I ( 3 ) II ( 4 ) III ( 30 ) 5.9

166

I ( 3 ) II ( 4 ) III ( 30 ) 7.41

167

I ( 3 ) II ( 4 ) III ( 30 ) 15.75

168

I ( 3 ) II ( 4 ) III ( 30 ) 7.41

169

I ( 3 ) II ( 4 ) III ( 30 ) 5.21

SR: Square rack,

SR(TS): Square rack (top-side),

6LRR: Six-layer rectangular rack,

5LRR: Five-layer rectangular rack,

FR: Floor rack.

Optimisation of Ceramic Kiln Loading Problem Using Multi-Objective Genetic Algorithm

257