Modeling and Simulation of Ethanol Steam Reforming for
Sustainable Hydrogen Production
Luca Cimmino
a
, Francesco Calise
b
, Francesco Liberato Cappiello
c
,
Massimo Dentice d’Accadia
d
and Maria Vicidomini
e
Department of Industrial Engineering, University of Naples Federico II, Piazzale V. Tecchio 80, 80125, Napoli, Italy
Keywords: Ethanol Reforming, Hydrogen Production, Sustainable Energy, Reactor Modeling, Energy Transition.
Abstract: The transition toward sustainable energy systems emphasizes hydrogen as a clean energy carrier, with ethanol
steam reforming emerging as a promising pathway for its renewable production. This study presents a one-
dimensional reactor model developed and simulated using MatLab, integrating thermodynamic, kinetic, and
heat transfer analyses to evaluate the performance of ethanol reforming. The model was validated against
existing literature and simulated under varying operational parameters. Key numerical results indicate that
the reactor achieves a hydrogen yield of 85% and an energy efficiency exceeding 75% at optimal conditions,
with inlet temperatures of 600°C and an ethanol-to-water molar ratio of 1:3. Sensitivity analysis revealed that
increasing the ethanol flow rate from 0.1 to 0.3 mol/s reduced the hydrogen yield by 12%, while adjusting the
reactor diameter from 0.05 m to 0.1 m improved the thermal efficiency by 10%. The system performance was
also significantly influenced by heat transfer coefficients, which ranged from 500 to 800 W/m²·K along the
reactor. The study also highlights the potential of integrating carbon capture technologies to mitigate CO
2
emissions generated as a byproduct. These findings provide valuable insights for optimizing ethanol
reforming reactors, paving the way for scalable and sustainable hydrogen production technologies in
renewable energy systems.
1 INTRODUCTION
The increasing urgency of addressing global climate
change has intensified the search for sustainable
energy solutions to reduce reliance on fossil fuels.
Hydrogen, as a clean energy carrier, has emerged as
a cornerstone of the global transition toward low-
carbon energy systems due to its versatility and
environmental benefits (Kovač, 2021). Among
various hydrogen production technologies, ethanol
steam reforming stands out as a renewable and
scalable method that leverages biomass-derived
ethanol, aligning with global sustainability goals (Ni,
2007).
Ethanol steam reforming involves complex
chemical and physical processes, including
endothermic reactions, heat transfer, and intricate
a
https://orcid.org/0000-0001-6382-3619
b
https://orcid.org/0000-0002-5313-7592
c
https://orcid.org/0000-0001-6292-686X
d
https://orcid.org/0000-0002-6766-5029
e
https://orcid.org/0000-0003-2827-5092
kinetic mechanisms, which present significant
modeling and optimization challenges (Palma,
2014).
Kinetic modeling plays a crucial role in
understanding the chemical reaction rates involved in
ethanol steam reforming (ESR). Various studies have
proposed kinetic models utilizing mechanisms such
as Eley-Rideal (ER) (Zhang, 2014) and Langmuir –
Hinshelwood – Hougen - Watson (LHHW)
(Olafadehan, 2015), which describe the interactions
between reactants, intermediates, and catalysts while
identifying the rate-determining steps (RDS)
governing the overall reaction. The ER mechanism
involves the reaction of a gas-phase molecule with an
adsorbed species on the catalyst surface. For ESR,
this is represented by a gas-phase species reacting
directly with an adsorbed molecule to form products.
232
Cimmino, L., Calise, F., Cappiello, F. L., Dentice d’Accadia, M. and Vicidomini, M.
Modeling and Simulation of Ethanol Steam Reforming for Sustainable Hydrogen Production.
DOI: 10.5220/0013465000003953
Paper published under CC license (CC BY-NC-ND 4.0)
In Proceedings of the 14th International Conference on Smart Cities and Green ICT Systems (SMARTGREENS 2025), pages 232-238
ISBN: 978-989-758-751-1; ISSN: 2184-4968
Proceedings Copyright © 2025 by SCITEPRESS – Science and Technology Publications, Lda.

Akande et al. developed a kinetic model for
hydrogen production over a Ni-Al₂O₃ catalyst based
on this mechanism. They identified the dissociation
of adsorbed ethanol as the RDS, with reaction rates
evaluated using Levenberg-Marquardt regression.
Their model demonstrated a mean absolute deviation
of 21% and provided critical kinetic parameters to
optimize reactor performance (Akande, 2006).
The LHHW mechanism, in contrast, involves
the adsorption of both reactants on the catalyst
surface, followed by surface reactions to form
products. This mechanism is mathematically
described by equations accounting for adsorption,
surface reactions, and desorption steps. Akpan et al.
extended the LHHW model for ESR using a Ni-based
catalyst, demonstrating the absence of methane and
carbon monoxide in the effluent at operational
temperatures between 673 and 863 K. Their work
highlighted the significance of dehydrogenation,
dehydration, and C-C bond cleavage reactions in the
reforming process (Akpan, 2007).
Mas et al. developed two LHHW-based models for
ESR using Ni-Al/OH catalysts, considering both
ethanol and methane reforming reactions. Their
Model A ignored CO and CO
2
adsorption, while
Model B included methane adsorption as a
competitive step. They identified the surface reaction
between ethanol and water as the RDS, with
activation energies ranging from 145 to 213 kJ/mol
(Mas, 2008).
Similarly, Sahoo et al. investigated ESR on
Co/Al₂O₃ catalysts, focusing on acetaldehyde
formation as the RDS. Their study demonstrated
nearly 100% ethanol conversion and hydrogen yields
of 5 mol/mol ethanol at 973 K (Sahoo, 2007).
Graschinsky et al. proposed a LHHW model for
ESR using a Rh/MgAl₂O₄-Al₂O₃ catalysts,
emphasizing the interplay between ethanol
dissociation, water-gas shift reactions, and methane
reforming. Their experiments achieved 83%
conversion at 873 K and revealed significant insights
into the role of surface reactions in hydrogen
production (Graschinsky, 2010).
Punase et al. advanced the field by applying a
multi-objective optimization approach to ESR
reactors, balancing hydrogen yield and thermal
efficiency. Using a model based on Mas et al.'s
framework, they identified optimal operating
conditions through advanced numerical algorithms.
Their findings underscored the importance of
operational parameters, such as temperature,
pressure, and the steam-to-ethanol ratio, in
maximizing reactor performance (Punase, 2019).
Despite these advances, challenges remain in
integrating kinetic models with practical reactor
simulations. Most studies assume idealized
conditions, neglecting heat and mass transfer
phenomena that significantly affect reactor
performance. Moreover, the competitive adsorption
of intermediates and byproducts introduces additional
complexities, necessitating further experimental
validation and model refinement to improve the
accuracy of simulations.
Accurate modeling of these processes is critical
to optimizing reactor performance and achieving the
efficiency required for commercial viability. While
existing studies have made considerable strides in
modeling ethanol reforming reactors (Punase, 2019),
most focus on either thermodynamic or kinetic
aspects, often neglecting their integration with heat
transfer and practical operational conditions (Mas,
2008). Furthermore, limited sensitivity analyses are
available to evaluate the impact of varying
operational parameters on hydrogen yield and
thermal efficiency (Olafadehan, 2015).
This study addresses these gaps by developing a
one-dimensional reactor model for ethanol steam
reforming that integrates thermodynamics, kinetics,
and heat transfer considerations. Implemented in
MatLab, the model advances existing research by
evaluating the reactor performance under realistic
operating conditions and conducting a
comprehensive sensitivity analysis. Key innovations
include the incorporation of heat transfer coefficients
along the reactor length and the identification of
optimal operating parameters, such as ethanol flow
rate, reactor dimensions, and inlet temperatures.
These improvements provide a more robust
framework for optimizing hydrogen production while
minimizing energy consumption and environmental
impact.
The outcomes of this work contribute
significantly to the ongoing development of
sustainable hydrogen production technologies. By
addressing the complexities of ethanol reforming and
bridging gaps in the literature, this study offers
actionable insights for scaling up ethanol-based
hydrogen systems as a viable alternative in the global
energy transition.
2 METHODOLOGY
The methodology adopted in this study follows a
structured and systematic approach. Initially, the
theoretical foundations of ethanol reforming
chemistry and the thermodynamics governing heat
Modeling and Simulation of Ethanol Steam Reforming for Sustainable Hydrogen Production
233

exchange phenomena were thoroughly reviewed,
with a focus on identifying the most robust and
widely recognized models in the scientific literature.
Subsequently, these models were individually
developed and implemented in MatLab environment
to verify the accurate behavior and performance of
the one-dimensional (1D) field. Following this, the
models were integrated to simulate the performance
of an ethanol reforming reactor under varying
operating conditions, providing insights for its
potential integration into a more comprehensive
dynamic model.
Finally, sensitivity analyses were conducted to
assess the system dependence on various variables
and operating parameters, highlighting key factors
that influence reactor performance.
3 MODEL
This section describes the model used to simulate
ethanol reforming within the reformer component.
The model integrates concepts of thermodynamics,
chemical kinetics, and reactor engineering. Various
approaches and methodologies were employed to
develop an accurate and efficient model for hydrogen
production via ethanol reforming.
3.1 Heat Transfer Model
The reactor was modeled as a concentric tube heat
exchanger in equi-current configuration.
The global heat transfer coefficient (U) is a key
parameter in determining the efficiency of the heat
exchanger. It is calculated considering both
convective and conductive thermal resistances:
11 1
wall
int wall ext
t
Uh k h
=+ +
(1)
where h
int
and h
ext
are the convective heat transfer
coefficients for the inner and annular fluids,
respectively, t
wall
is the wall thickness of the inner
tube, and k
wall
is the thermal conductivity of the tube
material.
Calculating U is crucial to assess the efficiency of
heat exchange between two slices in the reactor
model. To calculate U, it is necessary to determine the
properties of the two fluids: a hot fluid (a mixture of
nitrogen and oxygen resulting from post-combustion
in a Solid Oxide Fuel Cell, (SOFC) and a fuel mixture
(a combination of nitrogen, ethanol, and water vapor).
The calculated properties include dynamic viscosity
(interpolated from NIST tables, in case of pure
components, while for ethanol, values are obtained
from REFPROP), thermal conductivity, gas density,
fluid velocity, dimensionless convective flow
numbers (Re, Pr, Nu).
3.1.1 Thermal Energy Balance
The energy balance for a concentric tube heat
exchanger equates the heat released by the hot fluid
to the heat absorbed by the cold fluid:
()()
,, , ,, ,h p h h in h out c p c c out c in
QmC T T mC T T=−= −


(2)
Where Q is the heat transferred, ṁ
h
and ṁ
c
are the
mass flow rates of the hot and cold fluids,
respectively, c
p,h
and c
p,c
are the specific heat
capacities of the hot and cold fluids, respectively, T
h,in
and T
h,out
are the inlet and outlet temperatures of the
hot fluid, respectively, and T
c,in
and T
c,out
are the inlet
and outlet temperatures of the cold fluid.
The heat transfer balance is coupled with the Log
Mean Temperature Difference (LMTD), representing
the driving force for heat transfer in the heat
exchanger (Bergman, 2011).
The efficiency of a concentric tube heat exchanger
is given by:
max
Q
Q
η =


(3)
Where Q is the actual heat transferred and Q
max
is
the maximum theoretically transferable heat
(Bergman, 2011).
3.2 Ethanol Reforming Model
The ethanol steam reforming process was modeled as
a one-dimensional plug flow reactor (PFR), assuming
that axial variations dominate over radial variations.
This approach is suitable for systems where gradients
in temperature, concentration, and velocity in the
radial direction are negligible.
The reactor was discretized into differential
control volumes, allowing for numerical integration
of the governing equations.
The assumptions and simplifications of the model
are as follows:
1. The system operates under steady-state
conditions.
2. Ideal gas behavior is assumed for all species.
3. Radial gradients in temperature and
concentration are negligible.
SMEN 2025 - Special Session on Smart City and Smart Energy Networks
234

4. Heat losses to the surroundings are ignored,
and only heat transfer between the catalyst
bed and the gas phase is considered.
5. The reaction rates are governed by kinetic
models based on the LHHW mechanism.
In ethanol reforming, the LHHW mechanism is
frequently used to model catalytic reactions. The key
reactions involved include:
• Ethanol decomposition:
C
2
H
5
OH → CH
3
CHO + H
2
(4)
• Steam reforming:
C
2
H
5
OH + H
2
O → 2CO + 4H
2
(5)
• Water-gas shift reaction:
CO + H
2
O → CO
2
+ H
2
(6)
The kinetic equations for these reactions account for
the adsorption of reactants, surface reactions, and
desorption of products. For example, the reaction rate
(r) for ethanol steam reforming can be expressed as:
()
25 2
25 25 2 2
2
1...
reac C H OH H O
CHOH CHOH E HO HO
k
r
KPKKP
θθ
=
+++
(7)
where k
reac
is the reaction rate constant, θ represents
the fraction of adsorption sites occupied by each
species, and K denotes the adsorption constants for
the reactants. This formulation captures the
competitive adsorption of reactants and the role of
catalyst surface phenomena in determining reaction
rates.
3.2.1 Chemical Kinetic Model
The kinetic model employed in this study is the
Model B described by Mas et al. (Mas, 2008). This
model adopts the LHHW approach to describe the
catalytic surface reactions involved in ethanol steam
reforming. The key reactions considered in this model
include:
• Adsorption of ethanol on the catalyst
surface:
C
2
H
5
OH+(a)↔C
2
H
5
OH∗
(8)
• Adsorption of water on the catalyst surface:
H
2
O+(a)↔H
2
O∗
(9)
• Surface reaction causing ethanol
dissociation:
C
2
H
5
OH∗→CO+CH
4
∗+H
2
(10)
• Surface reaction between water and ethanol:
C
2
H
5
OH∗+H
2
O∗→CO
2
+CH
4
∗+2H
2
+(a)
(11)
• Desorption of methane:
CH
4
∗↔CH
4
+(a)
(12)
• Surface reaction of methane and molecular
rearrangement of water:
CH
4
∗+H
2
O∗→CO+3H
2
+2(a)
CH
4
∗+2H
2
O∗→CO
2
+4H
2
+3(a)
(13)
The four rate-determining steps (RDS) in this
model are ethanol decomposition, ethanol steam
reforming, methane steam reforming-I, and methane
steam reforming-II.
The reaction rates (r
1
, r
2
, r
3
, and r
4
) are functions
of temperature and the concentrations of the
reactants. These rates are used to solve the differential
mass balance equations between slices of the reactor.
The rate equations for each reaction, along with the
standard heats of formation (ΔH
0
), are presented in
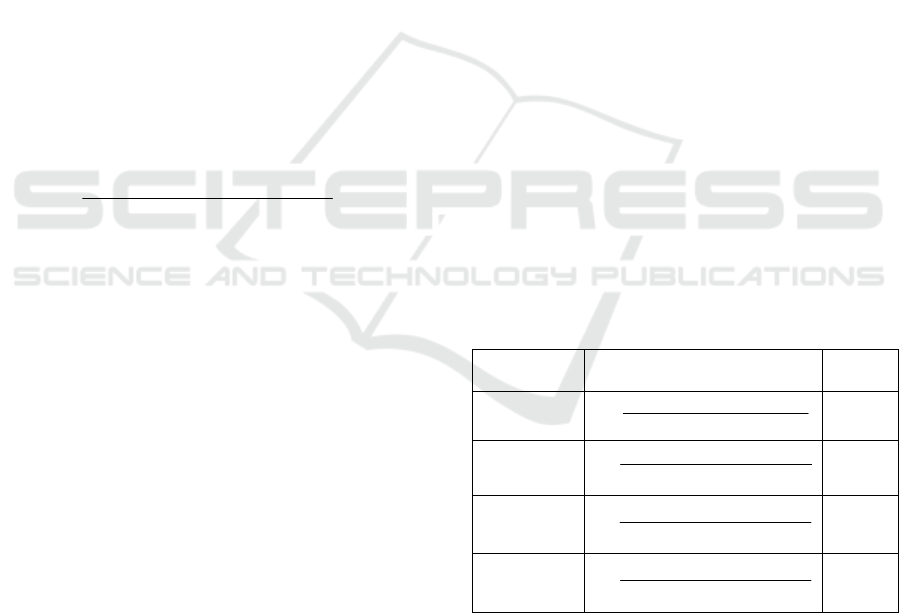
Table 1.
Table 1: Rate equations (Model B) and Standard Heats of
Formation.
Reaction Rate Equation
ΔH
0
(kJ/mol)
Ethanol
Decomposition
22
1
1
1
EE
E
EHOHOMM
kK P
r
PK P K P K
=
++ +
49.7
Ethanol Steam
Reforming
()
22
22
2
2
2
1
EHOEHO
EE HOHO MM
kK K PP
r
PK P K P K
=
++ +
205.0
Methane Steam
Reforming-I
()
22 2
22
4
33
3
2
1
MHOMHO COH
EE HOHO MM
kK K P P KP P
r
PK P K P K
−
=
++ +
206.1
Methane Steam
Reforming-II
()
22
22
2
4
4
3
1
MHOMHO
EE HOHO MM
kK K PP
r
PK P K P K
=
++ +
165.0
Where the subscript E refers to ethanol, k
i
is the
reaction rate constant and K
i
is the equilibrium
constant, for the i-th chemical species. Kinetic
parameters, such as rate constants and adsorption
coefficients, were taken from several studies. For the
implementation of this model, the parameters
proposed by K. D. Punase et al. (Punase, 2019) were
adopted, shown in Table 2.
Modeling and Simulation of Ethanol Steam Reforming for Sustainable Hydrogen Production
235

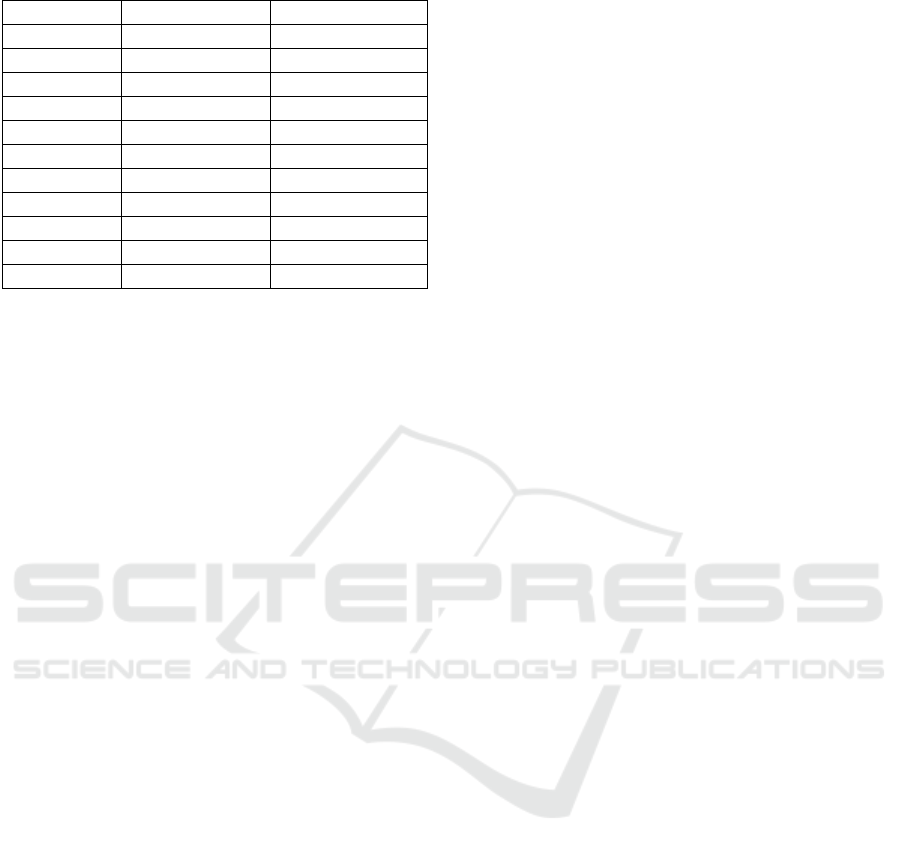
Table 2: Kinetic parameters adopted in this model.
Parameter Value Unit
k
1,0
3.27x10
11
mol/(min∙C
p
∙
g
ca
t
)
k
2,0
1.39x10
10
mol/(min∙C
p
∙
g
ca
t
)
k
3,0
2.21x10
3
mol/(min∙C
p
∙
g
ca
t
)
k
4,0
1.26x10
9
mol/(min∙C
p
∙
g
ca
t
)
E
a,1
271,902 J/mol
E
a,2
226,768 J/mol
E
a,3
123,279 J/mol
E
a,4
213,936 J/mol
ΔH
E
-197,964 J/mol
ΔH
H
2O
-91,708 J/mol
ΔH
M
-124,789 J/mol
3.2.2 Mass and Energy Balances
The mass balance in a plug flow reactor assumes one-
dimensional, pseudo-homogeneous, steady-state, and
isothermal conditions to model species changes via
differential equations accounting for reaction rates. For
non-ideal isothermal behavior, the reactor is divided
into slices where local isothermality is assumed.
Energy balances for each slice account for heat transfer
and reaction enthalpies, and an iterative solution
(implemented in MATLAB) across many slices
provides a detailed temperature and composition
profile for the ethanol reforming process.
3.3 Simulation Setup
The simulations were conducted by using reactor
parameters typical of lab-scale processes. Boundary
conditions were defined based on inlet flow rates and
temperatures, and the constants of the model are the
ones given from Punase et al. (Punase, 2019).
The reactor catalyst is a Ni/Al material with an
apparent density of 5.0 g/cm
3
and the reactor operates
at ambient pressure (1 atm). The reactor was
simulated under various operating conditions, with
key parameters summarized in (Punase, 2019).
The inlet temperature and pressure are 875 K for
the cold fluid and 1350 K for the hot fluid, and 1 atm
for both, respectively.
Ethanol, used as the carbon and hydrogen source,
is supplied at 15.00 kmol/h. A steam-to-ethanol (S/E)
ratio of 3.5 ensures an excess of steam, minimizing
solid carbon (coking) formation and enhancing
reaction efficiency (Mas, 2008).
Nitrogen at 30.0 kmol/h is included in the cold
fluid as an inert component, consistent with previous
studies. The cold fluid inlet temperature of 875 K falls
within the optimal range for reforming reactions
(Mas, Bergamini et al. 2008).
The hot fluid composition primarily consists of
nitrogen (210.1 kmol/h) and oxygen (20.9 kmol/h),
along with a negligible fraction of steam and carbon
dioxide from combustion. The inlet temperature of
1350 K reflects typical exhaust gas conditions from
SOFC systems, providing a nitrogen-rich stream
(from air used as an oxidant) with residual oxygen.
The model was validated against published
experimental data
from (Mas, 2008) to ensure accuracy.
4 RESULTS AND DISCUSSION
This section presents the results of the simulations
performed with the coupled chemical-heat transfer
model, discussing the key findings.
4.1 Results of the Simulation
Figure 1 shows the temperature trends of the hot and
cold fluids along the reactor, comparing scenarios of
pure heat exchange and heat exchange coupled with
chemical reactions. The comparison was conducted
using the same model, but in one case excluding
chemical reactions and the associated heat
consumption from the energy balances. This analysis
aimed to evaluate the impact of chemical reactions on
temperature profiles.
The graph shows that, as expected, the cold fluid
temperature reaches higher values when reactions are
not considered. Without the heat consumption
required for endothermic reactions, all heat
transferred from the hot fluid is utilized solely to
increase the cold fluid temperature.
Figure 2 shows the heat transfer coefficient along
the reactor. This coefficient reflects the efficiency of
heat transfer from the hot fluid to the cold fluid and
varies along the reactor, influenced by local
temperature conditions and fluid composition.
The graph displays the variation of the heat
transfer coefficient (U) along the reactor. Despite the
decreasing temperature difference between the two
fluids, the heat transfer coefficient increases. This
behavior can be explained by specific factors in the
one-dimensional discrete model:
• Improved Convection Conditions: Even as the
temperature difference decreases, the flow
conditions may enhance convection. This could
result from increased turbulence or improved
fluid velocity profiles, leading to more effective
heat transfer.
• Changes in Fluid Properties: The thermophysical
properties of the fluids, such as viscosity and
thermal conductivity, change with temperature.
SMEN 2025 - Special Session on Smart City and Smart Energy Networks
236

These variations may favour the heat transfer.
For example, reduced viscosity improves
convection, while increased thermal conductivity
enhances heat transfer capacity.
Figure 3 depicts the composition of the cold fluid
along the reactor, divided into reactants and products.
The concentration changes reflect the progression of
chemical reactions, showing a decrease in reactants
(ethanol and water) and an increase in products
(hydrogen, carbon monoxide, carbon dioxide,
methane) along the reactor length.
Figure 1: Temperatures of hot and cold fluids along the
reactor with and without reactions.
Figure 2: Heat transfer coefficient along the reactor.
As observed in the graph, ethanol conversion
results are not close to optimal values. This is
influenced by the initial parameter selection and heat
exchanger configuration, which affect:
• Cold Fluid Velocity: It must remain low to
ensure sufficient residence time for ethanol in the
reactor but cannot be too low, as this would
compromise heat transfer.
• Reactor Volume and Catalyst Quantity: These
determine the extent of reaction and conversion
rates.
• Initial Composition of the Cold Fluid: This
impacts reactant concentrations and,
consequently, reaction rates.
Figure 3: Composition of the cold fluid along the reactor.
5 CONCLUSIONS
This study confirms the potential of ethanol steam
reforming as a sustainable pathway for hydrogen
production, underlining its significance in advancing
clean energy technologies. The integrated reactor
model, encompassing thermodynamic, kinetic, and
heat transfer analyses, was validated against literature
and applied under various operational conditions,
yielding valuable insights into reactor performance
and optimization.
The simulations revealed a hydrogen yield of 85%
and an energy efficiency exceeding 75% at optimal
conditions, specifically at an inlet temperature of
600°C and an ethanol-to-water molar ratio of 1:3.
Sensitivity analysis further highlighted the impact of
critical parameters. For instance, increasing the
ethanol inlet flow rate from 0.1 mol/s to 0.3 mol/s
resulted in a 12% reduction in hydrogen yield,
attributed to reduced residence times. Adjusting the
reactor diameter from 0.05 m to 0.1 m led to a 10%
improvement in thermal efficiency. The heat transfer
coefficients, varying between 500 and 800 W/m²·K
along the reactor, were shown to significantly
influence reactor efficiency.
Moreover, the study emphasized the role of the
heat exchange area-to-geometric area ratio in
optimizing the process. Enhancements in this ratio,
achieved through technological adjustments like fins,
substantially improved hydrogen production and
ethanol conversion.
In conclusion, the developed model offers a robust
framework for designing and optimizing ethanol
reforming reactors. The findings not only deepen the
understanding of reaction mechanisms and heat
transfer dynamics but also provide actionable strate-
gies for scaling up hydrogen production technologies
while supporting the global energy transition.
Modeling and Simulation of Ethanol Steam Reforming for Sustainable Hydrogen Production
237

ACKNOWLEDGEMENTS
The authors gratefully acknowledge the partial
financial support of the project PRIN 2020:
OPTIMISM – Optimal refurbishment design and
management of small energy micro-grids, funded by
the Italian Ministry of University and Research
(MUR).
REFERENCES
Akande, P., Rao, Vijay (2006). "A review on mechanistic
kinetic models of ethanol steam reforming for hydrogen
production using a fixed bed reactor." Chemical Papers
73: 1027-1042.
Akpan, E., A. Akande, A. Aboudheir, H. Ibrahim and R.
Idem (2007). "Experimental, kinetic and 2-D reactor
modeling for simulation of the production of hydrogen
by the catalytic reforming of concentrated crude ethanol
(CRCCE) over a Ni-based commercial catalyst in a
packed-bed tubular reactor." Chemical Engineering
Science 62(12): 3112-3126.
Bergman, T. L., A. S. Lavine and F. P. Incropera (2011).
Fundamentals of Heat and Mass Transfer, 7th Edition,
John Wiley & Sons, Incorporated.
Graschinsky, C., M. Laborde, N. Amadeo, A. Le Valant, N.
Bion, F. Epron and D. Duprez (2010). "Ethanol Steam
Reforming over Rh(1%)MgAl2O4/Al2O3: A Kinetic
Study." Industrial & Engineering Chemistry Research
49(24): 12383-12389.
Kovač, A., M. Paranos and D. Marciuš (2021). "Hydrogen
in energy transition: A review." International Journal
of Hydrogen Energy 46(16): 10016-10035.
Mas, V., M. L. Bergamini, G. Baronetti, N. Amadeo and M.
Laborde (2008). "A Kinetic Study of Ethanol Steam
Reforming Using a Nickel Based Catalyst." Topics in
Catalysis 51(1): 39-48.
Ni, M., D. Y. C. Leung and M. K. H. Leung (2007). "A
review on reforming bio-ethanol for hydrogen
production." International Journal of Hydrogen Energy
32(15): 3238-3247.
Olafadehan, O. A., A. Ayoola, O. Akintunde and V.
Adeniyi (2015). "Mechanistic Kinetic Models For
Steam Reforming Of Concentrated Crude Ethanol On
Ni/Al
2
O
3
Catalyst."
Palma, V., F. Castaldo, P. Ciambelli and G. Iaquaniello
(2014). "CeO2-supported Pt/Ni catalyst for the
renewable and clean H2 production via ethanol steam
reforming." Applied Catalysis B: Environmental 145:
73-84.
Punase, K. D., N. Rao and P. Vijay (2019). "A review on
mechanistic kinetic models of ethanol steam reforming
for hydrogen production using a fixed bed reactor."
Chemical Papers 73(5): 1027-1042.
Sahoo, D. R., S. Vajpai, S. Patel and K. K. Pant (2007).
"Kinetic modeling of steam reforming of ethanol for the
production of hydrogen over Co/Al2O3 catalyst."
Chemical Engineering Journal 125(3): 139-147.
Zhang, C., S. Li, G. Wu, Z. Huang, Z. Han, T. Wang and J.
Gong (2014). "Steam reforming of ethanol over skeletal
Ni-based catalysts: A temperature programmed
desorption and kinetic study." AIChE Journal 60(2):
635-644.
SMEN 2025 - Special Session on Smart City and Smart Energy Networks
238