From Coefficients to 3D Planes: A VR Mathematics Module for Teaching
Systems of Linear Equations with 3D Interaction
Adam Nowak
2
, Anita Dabrowicz-Tlalka
1
, Magdalena M. Musielak
1
, Katrzyna Kujawska
1
,
Zuzanna Partyka
3
, Dorota Krawczyk-Stando
2
, Anna Laska-Lesniewicz
2
, Nina Szczygiel
4
and Jacek Sta
´
ndo
2
1
Gdansk University of Technology, Mathematics Center, Gdansk, Poland
2
Lodz University of Technology, Lodz, Poland
3
Medical University of Lodz, Lodz, Poland
4
University of Aveiro, Aveiro, Portugal
Keywords:
Extended Reality, Virtual Reality, Linear Equations, Immersive Learning.
Abstract:
Traditional approaches to teaching systems of linear equations frequently fail to connect abstract algebraic
principles with students’ spatial reasoning, resulting in recurring errors in solution determination. To ad-
dress this, we developed a virtual reality (VR) application for Meta Quest headsets that enables immersive
interaction with 3D graphical representations of systems of linear equations. The application allows users
to manipulate equations as interactive planes in virtual space, adjust coefficients and visualize intersections
in real time. In a study of 38 students, participants were tasked with identifying valid solutions (empty set,
point, line, plane) in a paper-based exercise. Results indicated that students frequently misidentified solutions,
selecting incorrect options such as two lines, three lines, several points, lines, and two triangles. Further-
more, correct solutions were not consistently identified (empty set: 60%, point: 44%, line: 51%, plane 68%).
After a VR session, all students correctly recognized proper solution types (empty set, point, line, plane) in
post-test scenarios, demonstrating improved conceptual mastery. Qualitative feedback highlighted heightened
engagement and reduced cognitive load during VR tasks. These findings suggest that VR presentation can
significantly improve algebraic problem-solving skills compared to static, paper-based methods. This study
underscores the potential of immersive technologies to transform mathematics education while emphasizing
the need for teacher training to integrate such tools effectively.
1 INTRODUCTION
The evolution of educational methodologies has his-
torically mirrored technological progress. For cen-
turies, teaching relied on oral traditions and static
texts, but the 20th century introduced audiovisual
tools, followed by digital platforms, each reshaping
pedagogical strategies. Today, the acceleration of
technologies such as artificial intelligence (AI), aug-
mented reality (AR), and virtual reality (VR) has ush-
ered in a paradigm shift, demanding yet another trans-
formation in how educators teach and students learn.
While these tools promise immersive, personalized
learning experiences, they also create significant chal-
lenges for teachers – many of whom were trained in
didactic approaches rooted in pre-digital eras (Ert-
mer and Ottenbreit-Leftwich, 2010), (Bieniecki et al.,
2010).
Modern students, as digital natives, increasingly
inhabit virtual worlds, rendering traditional methods
like textbooks and lectures less engaging. For mathe-
matics education, this disconnect is particularly acute.
Abstract concepts such as systems of linear equations,
often taught through paper, struggle to resonate with
learners who thrive on interactivity and instant feed-
back (Makransky and Petersen, 2021). Simultane-
ously, teachers face a dual burden: mastering emerg-
ing technologies while reconciling them with peda-
gogical frameworks from their own training, which
may lack emphasis on digital tools (Voogt et al.,
2013). This tension underscores a critical responsibil-
ity for researchers – to design evidence-based techno-
874
Nowak, A., Dabrowicz-Tlalka, A., Musielak, M. M., Kujawska, K., Partyka, Z., Krawczyk-Stando, D., Laska-Lesniewicz, A., Szczygiel, N. and Sta
´
ndo, J.
From Coefficients to 3D Planes: A VR Mathematics Module for Teaching Systems of Linear Equations with 3D Interaction.
DOI: 10.5220/0013500000003932
Paper published under CC license (CC BY-NC-ND 4.0)
In Proceedings of the 17th International Conference on Computer Supported Education (CSEDU 2025) - Volume 1, pages 874-881
ISBN: 978-989-758-746-7; ISSN: 2184-5026
Proceedings Copyright © 2025 by SCITEPRESS – Science and Technology Publications, Lda.

Figure 1: User wearing VR glasses and working on the
given system of linear equations in the application.
logical solutions that empower educators, rather than
replace them, by aligning innovation with curricular
goals and classroom realities.
Virtual reality (VR) exemplifies this potential. By
immersing learners in 3D environments (Dalgarno
and Lee, 2010) where they can spatially manipulate
equations, VR bridges the gap between abstract math-
ematics and tangible experience, aligning with em-
bodied cognition theory (Johnson-Glenberg, 2017).
However, its adoption hinges on addressing educa-
tors’ practical concerns, such as usability, curriculum
integration, and training demands (Nicholson et al.,
2022). Without tools that are both pedagogically
sound and teacher-friendly, even the most advanced
technologies risk exacerbating, rather than alleviat-
ing, educational inequities.
This study focuses on developing and evaluating
a VR application for teaching systems of linear equa-
tions – a topic foundational to algebra yet persistently
challenging due to its reliance on spatial reasoning
and multi-step problem-solving. By collaborating
with educators during the design process, we aim to
create a tool that not only enhances student engage-
ment and understanding but also supports teachers in
transitioning from traditional methods to technology-
aided instruction. Our work seeks to answer follow-
ing question:
• Will students, based on their knowledge of the ge-
ometric interpretation of systems of linear equa-
tions with two unknowns, be able to present and
geometrically interpret solutions to systems of
three linear equations with three unknowns?
The remainder of this article is organized as fol-
lows. First we synthesize existing research on VR
in mathematics education, focusing on theoretical
frameworks like embodied cognition and construc-
tivist learning. The next section details the tech-
nical implementation of our VR tool and System
of Linear Equations in VR introduces mathematical
background in terms of VR application, emphasiz-
ing its interactive 3D graphing system and gamified
problem-solving tasks. The study design then de-
scribes the methodology, including participant selec-
tion, experimental procedures, and assessment met-
rics. The Results section presents quantitative and
qualitative findings on learning gains and engage-
ment, followed by a Discussion interpreting these out-
comes in light of pedagogical challenges and oppor-
tunities. Finally, we summarize the implications for
future research and practical applications of VR in al-
gebra education.
2 LITERATURE REVIEW
Several recent studies have explored the integration of
Virtual Reality (VR) in mathematics education at uni-
versities, highlighting its potential to improve learn-
ing outcomes and student engagement. Mathemat-
ics often involves abstract concepts that can be chal-
lenging for students to grasp. VR allows learners to
visualize and manipulate these concepts in a three-
dimensional space, making it easier to understand
complex topics such as geometry, measurement, and
spatial relationships. This hands-on experience can
significantly enhance comprehension and retention.
The (L
´
opez and Garcia, 2023) study evaluates the
impact of VR on mathematics education in a rural sec-
ondary school in Antiguo Morelos, Mexico. It high-
lights how VR enhances student motivation and aca-
demic performance, particularly in understanding ge-
ometric concepts such as perimeters and areas. The
study employs a quasi-experimental design compar-
ing an experimental group using VR with a control
group receiving traditional instruction. The results in-
dicate a significant improvement in the experimental
group’s performance, suggesting that VR can effec-
tively bridge educational gaps, especially in resource-
limited settings. The implementation of VR tech-
nology necessitates training for educators, which can
lead to improved teaching practices overall. As teach-
ers become more proficient with technology integra-
tion, they can improve their instructional methods and
better support student learning outcomes.
In (Li, 2024) the author examines the potential of
XR technologies, including VR, to transform math-
From Coefficients to 3D Planes: A VR Mathematics Module for Teaching Systems of Linear Equations with 3D Interaction
875

ematics assessments. They discuss how immersive
environments can enhance the assessment process by
making mathematical concepts more accessible and
engaging. The paper outlines challenges to XR adop-
tion in educational settings and proposes a research
agenda for further exploration of its benefits in math-
ematics education.
The study of (Awang et al., 2016) reviews the in-
tegration of AI alongside AR and VR technologies in
mathematics education, emphasizing their role in en-
hancing student motivation and problem-solving abil-
ities. It presents thematic analyses from recent studies
that illustrate how these technologies can reduce math
anxiety and improve learning outcomes. The findings
indicate a growing interest in using immersive tech-
nologies to create more effective educational experi-
ences.
In (Qui
˜
nones et al., 2016) the authors evaluate stu-
dents’ experiences using a virtual reality (VR) tool
and their learning of three-dimensional vectors in
an introductory physics university course. The re-
searchers used an experimental research design, with
control and experimental groups, measuring student
performance using a pre-post 3D vectors question-
naire. They found that students in the experimen-
tal group outperformed the control group on items in
which visualization was important. The students per-
ceived the VR tool as having a positive impact on their
learning and as a valuable tool to enhance their learn-
ing experience.
In turn, in (Du and Li, 2022), the authors anal-
yse the application of VR technology in higher edu-
cation, selecting 80 empirical studies from the Web
of Science database. The review finds that VR ap-
plication research mainly focuses on undergraduates
in science, engineering, and medical-related majors.
Head-mounted devices are the primary VR equip-
ment. Most studies indicate that VR has positive
effects on higher education and teaching, primarily
by influencing student behaviour and, secondarily,
cognition and emotional attitudes. The authors sug-
gest that VR is mainly used in higher operability
courses and that researchers assess teaching effective-
ness mainly through objective tests, subjective ques-
tionnaires, and descriptive/variance analyses.
The work of (Qui
˜
nones et al., 2016) and (Du and
Li, 2022) contributes to the ongoing discourse on the
integration of VR in higher mathematics education,
showcasing its potential to transform teaching prac-
tices and enhance student learning outcomes.
The use of VR in mathematics education offers
numerous advantages that can enhance student en-
gagement, understanding, and overall academic per-
formance. As this technology continues to evolve, it
has the potential to transform educational practices in
underserved areas, making high-quality mathematics
education more accessible to all students.
3 VR APPLICATION DESIGN
The application, developed for Meta Quest 2/3 head-
sets (Android .apk), was built using Unity 2021.3.16
with the XR Interaction Toolkit to ensure cross-
platform functionality. A modular architecture was
adopted, comprising 13 distinct mathematical sub-
jects, though this study focuses exclusively on the sys-
tems of linear equations module.
3.1 Technical Implementation
Custom 3D models, including dynamic coordinate
grids and equation planes, were designed in Blender
and integrated into Unity. Real-time interaction was
enabled through a combination of Meta’s native hand
tracking API and controller-based input. Hand recog-
nition allows users to manipulate variables via intu-
itive gestures (e.g., pinching to ”grab” coefficients,
swiping to rotate planes), while controller support en-
sures accessibility for users less familiar with gesture
controls.
To mitigate motion sickness, comfort fea-
tures such as snap-turning (45°increments) and
teleportation-based locomotion were implemented.
Users can physically walk in room-scale environ-
ments or navigate virtually in seated mode. Per-
formance optimization was prioritized through baked
light maps, reducing computational load while main-
taining visual fidelity.
3.2 Module Overview: Systems of
Linear Equations
The module enables users to plot and manipulate sys-
tems of two or three linear equations in 3D space.
Equations are represented as interactive planes, with
sliders for adjusting coefficients and constants. Real-
time visual feedback, powered by custom shaders,
highlights intersections between planes using colour
gradients. Haptic vibrations confirm correct solu-
tions, while incorrect attempts prompt contextual
hints, such as textual cues (e.g. ”These planes are
parallel – no solution exists!”) or visual markers in-
dicating inconsistent coefficients.
A hybrid interface accommodates diverse interac-
tion preferences: hand tracking for direct manipula-
tion of equation components and controllers for pre-
cision tasks like numerical input. The UI includes an
ERSeGEL 2025 - Workshop on Extended Reality and Serious Games for Education and Learning
876

undo/redo system to encourage experimentation with-
out penalty.
4 SYSTEMS OF LINEAR
EQUATIONS IN VR
4.1 Justification for the Choice of Topic
In mathematics, a system of linear equations (or linear
system) refers to a set of one or more linear equations
that involve the same set of variables. This concept
forms the foundation of linear algebra, a discipline
that underpins much of modern mathematics and finds
applications in numerous fields. The development of
computational algorithms to solve these systems is
a cornerstone of numerical linear algebra, with crit-
ical implications for disciplines such as engineering,
physics, chemistry, computer science, and economics.
Furthermore, systems of non-linear equations can of-
ten be approximated by linear systems through a pro-
cess known as linearization. This technique is particu-
larly valuable when developing mathematical models
or computer simulations for analysing complex sys-
tems.
Given the prevalence of linear systems across vari-
ous scientific disciplines, many students encounter the
challenge of solving or interpreting such systems dur-
ing their education. Since linear equations possess a
straightforward geometrical interpretation, VR appli-
cations offer a promising avenue for enhancing the
learning experience. By leveraging VR technology,
students can engage with linear systems in an immer-
sive and interactive manner, facilitating a deeper un-
derstanding of the underlying concepts.
4.2 Learning Goals and Outcomes
When solving a system of equations, students must
address three fundamental questions:
1. Does the system have a solution ?
2. How many solutions exist ?
3. What are the solutions ?
To answer these, a student needs a solid understand-
ing of concepts like rank and reduced row echelon
form, as well as the ability to interpret calculations
and analyse the system’s geometric properties.
The VR application provides an interactive way
to explore these aspects. It enables students to visual-
ize the system graphically, adjust coefficients, and ob-
serve how changes affect its geometry. Through this,
students can explore the relationship between matrix
rank, the number of solutions, and the system’s geo-
metric interpretation. This hands-on approach helps
students build intuition for tackling larger systems
with more variables, where direct geometric visual-
ization is impossible.
4.2.1 Example 1
System of equations
(
−25x + 19y = −3
x − 2y = 0
(1)
is represented by two intersecting lines. The solution
of the system, which may be obtained from the aug-
mented matrix in its reduced row echelon form

−25 19 −3
1 −2 0

→

1 0
6
31
0 1
3
31

(2)
is the point of intersection (x, y) =

6
31
,
3
31

.
Figure 2: Example 1. Snapshot from VR application.
4.2.2 Example 2
System of equations





7x = −9
x − 3y = 7
−3x − 5y = 16
(3)
is represented by three lines that do not intersect at
one point. The system is inconsistent and has no solu-
tion, which can be concluded through the Kronecker-
Capelli theorem, since r(A) ̸= r([A|B]) in this case.
Figure 3: Example 2. Snapshots from VR application.
From Coefficients to 3D Planes: A VR Mathematics Module for Teaching Systems of Linear Equations with 3D Interaction
877

4.2.3 Example 3
System of equations





−6x − 19y + 4z = −13
−5x + 16z = 20
19x − 15y + 6z = 14
(4)
is represented by three planes that intersect at one
common point. The solution may be obtained from
the reduced row echelon form of the augmented ma-
trix.
Figure 4: Example 3. Snapshots from VR application.
4.3 Some Practical Applications
We must remember that an important element of the
process of acquiring mathematical knowledge during
engineering studies is learning the applications of the
concepts discussed. Students of various fields of sci-
ence will encounter system of equations in their stud-
ies.
In economy, problems connected to cost and profit
calculations appear throughout all levels of education.
Even primary school students may be faced with solv-
ing problem like this one:
”The cost of a ticket to the amusement park is 25C
for children and 50C for adults. On a certain day, at-
tendance at the park is 2 000 and the total gate revenue
is 70 000C. How many children and how many adults
bought tickets?”
Quick analysis of the problem leads us to a system
of two equations
(
x + y = 2000
25x + 50y = 70000
(5)
where x denotes the number of children and y denotes
the number of adults.
Students of chemistry very often have to work out
problems of balancing chemical equations, for exam-
ple
N
2
+ H
2
→ NH
3
(6)
A student must find the quantities of molecules of ni-
trogen and hydrogen on the left side of the reaction
that would produce a certain proportional amount of
the compound on the right side of the reaction. Simple
analysis of the problem leads to a system of equations
that is represented by the augmented matrix

2 0 −1 0
0 2 −3 0

(7)
The solution of the system yields a balanced equation
N
2
+ 3H
2
→ 2NH
3
(8)
In physics, a typical problem that leads to a system
of equation is determining unknown values in a given
circuit.
Figure 5: Example of electrical circuit that yields a system
of equations.
By applying Kirchhoff’s laws, one creates a sys-
tem of linear equations that allow to find the unknown
values, such as currents, voltages, or resistances. For
example, the circuit shown in Figure 5 yields the sys-
tem of equations





I
1
− I
2
+ I
3
= 0
3I
1
+ 2I
2
= 7
2I
2
+ 4I
3
= 8
(9)
where the currents I
1
, I
2
, I
3
are the unknown values.
5 STUDY DESIGN
5.1 Study Group
The study participants were first-year Electrical En-
gineering students at the Lodz University of Technol-
ogy. The research was conducted in January 2025,
with a total of 38 respondents.
5.2 Course of the Study
A month before the start of the study, first-year elec-
trical engineering students were taught the basics of
analytical geometry in three-dimensional space. As
part of these classes, they learned the equation of the
ERSeGEL 2025 - Workshop on Extended Reality and Serious Games for Education and Learning
878

plane, Ax + By +Cz = D, and its properties, as well
as vectors and operations on them. The aim of these
classes was to introduce students to 3D geometry and
prepare them for further mathematics classes.
On the day of the study, the teacher gave an hour-
long lecture on solving systems of linear equations
with two unknowns. The first part of the lecture re-
called the methods of solving systems of equations
(substitution, Gaussian elimination), and the second
part focused on the geometric interpretation of the so-
lution. The teacher explained that the solution of a
system of two linear equations with two unknowns
can be the intersection point of two lines in the plane,
the empty set – parallel lines that do not overlap, and
lines that overlap. Students were encouraged to ask
questions and actively participate in the discussion.
The following research question was posed: Will
students, based on their knowledge of the geometric
interpretation of systems of linear equations with two
unknowns, be able to present and geometrically in-
terpret solutions to systems of three linear equations
with three unknowns?
Research hypothesis: Students, based on their
knowledge of the geometric interpretation of systems
of linear equations with two unknowns, will have dif-
ficulties with the full and correct presentation and ge-
ometric interpretation of solutions to systems of three
linear equations with three unknowns.
In particular, they will not be able to correctly
present three planes and their relative position in 3D
space. They will not be able to recognize different
types of solutions in terms of geometric interpreta-
tion.
5.3 Results
In order to investigate whether students are able to
geometrically interpret solutions to a system of three
linear equations with three unknowns based on their
knowledge of the interpretation of systems of equa-
tions with two unknowns, a survey was conducted.
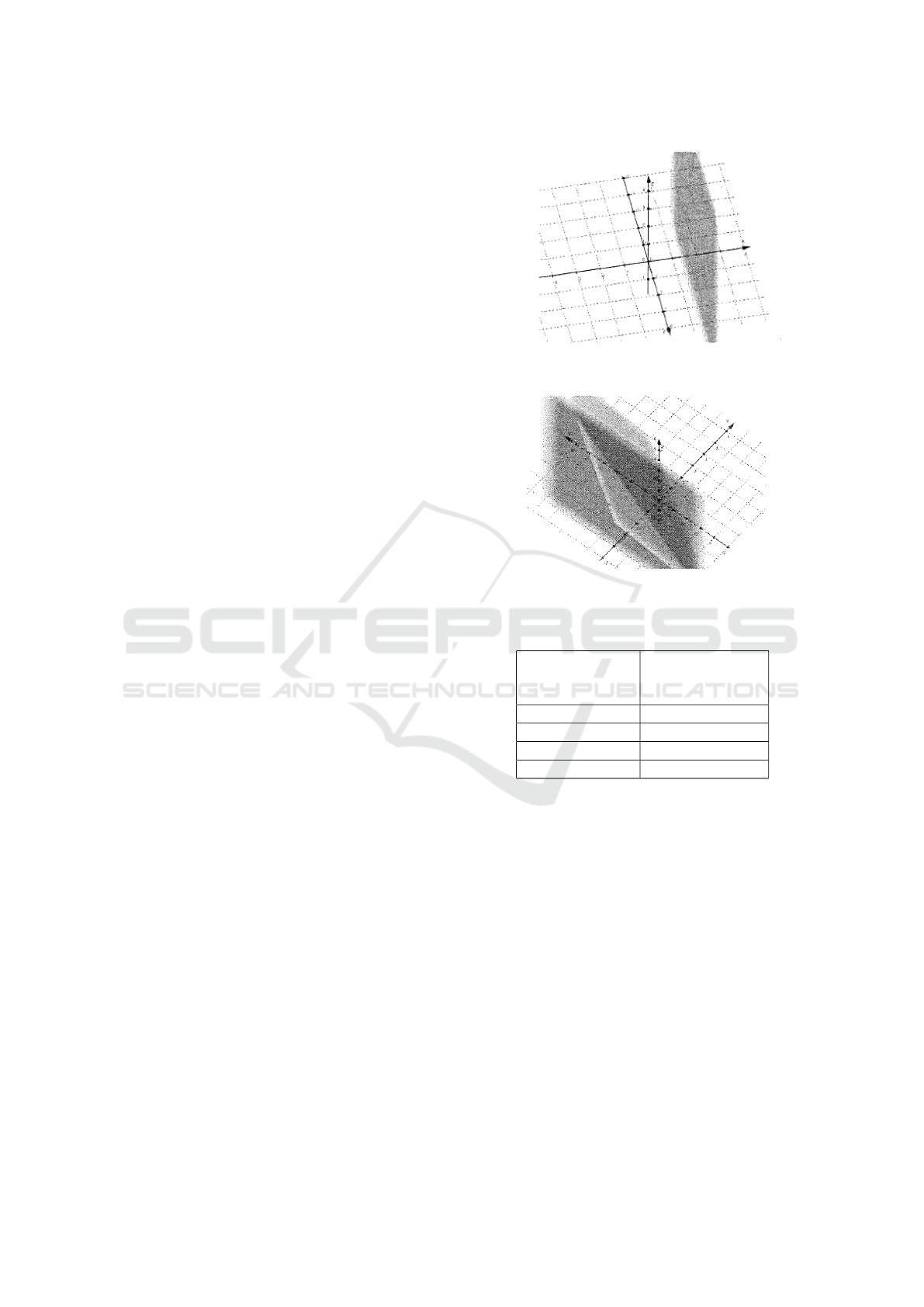
Students were presented with two drawings:
1. A drawing of a single plane in a three-dimensional
coordinate system (see Figure 6).
2. A drawing of two planes in a three-dimensional
coordinate system, with a marked line of their in-
tersection (see Figure 7).
Then the students were asked the following ques-
tion: List all possible geometric solutions to a system
of three planes in three-dimensional space. Describe
what geometric figures could be solutions to such a
system. In addition to correct answers, summarized
in Table 1, students were also giving incorrect ones:
Figure 6: The first drawing shown to students during study.
Figure 7: The second drawing shown to students during
study.
Table 1: Results of the study.
Possible solution Percentage
of students who
gave the answer
Empty set 60%
Point 44%
Line 51%
Plane 68%
two lines, three lines,several points, lines, two trian-
gles.
After engaging with the VR simulation of solving
systems of linear equations with three unknowns, stu-
dents correctly identified all possible solutions.
5.4 Discussion
As many as 60% of students indicated that the solu-
tion to a system of three planes can be the empty set.
This suggests that students have a good understanding
that three planes can have no common point, which
corresponds to the lack of solutions to a system of
equations.
Only 44% of students correctly identified a point
as a possible solution. This suggests that although
students may understand the concept of no solutions,
they may have difficulty visualizing a situation in
From Coefficients to 3D Planes: A VR Mathematics Module for Teaching Systems of Linear Equations with 3D Interaction
879

which three planes intersect at a single point, which
is the solution to the system.
51% of students indicated a line as a possible so-
lution. This suggests that students have a good under-
standing that three planes can intersect along a line,
which corresponds to an infinite number of solutions
to a system of equations.
As many as 68% of students identified a plane as
a possible solution. This suggests that students may
understand situation with plane as a solution, which
has infinite number of solutions.
These results suggest that students have difficulty
understanding the geometric interpretation of systems
of linear equations in three unknowns. They are good
at understanding the concept of no solutions and have
a reasonable understanding that a solution can be a
line. However, they have considerable difficulty vi-
sualizing a point as a solution and distinguishing be-
tween a plane as a member of the system and a plane
as a solution.
Thus, developing and utilizing a VR applica-
tion for solving systems of linear equations in three-
dimensional space is a logical and effective approach.
6 CONCLUSIONS
This study highlights the potential of VR applications
in enhancing the understanding of systems of linear
equations, particularly in three-dimensional space.
Our findings suggest that while students can grasp
certain geometric interpretations, such as the absence
of solutions or solutions as plane, they struggle with
visualizing points and lines as solutions.
The VR application proved effective in addressing
these challenges, allowing students to interact with
linear systems dynamically and gain a deeper concep-
tual understanding. By engaging with geometric rep-
resentations in an immersive environment, students
were able to correctly identify all possible solutions
after using the application.
These results suggest that VR can be a valuable
tool for algebra education, particularly in topics re-
quiring spatial reasoning. Future research should ex-
plore its effectiveness across different student popu-
lations and mathematical concepts, as well as inves-
tigate how VR-based learning compares to traditional
instructional methods in the long term.
6.1 Limitations
While the application currently lacks multiplayer
functionality, its single-user focus aligns with the
study’s goal of individualized skill assessment. Fu-
ture iterations could incorporate collaborative fea-
tures for group problem-solving scenarios.
We recognize the limitations of our data and study
design. Firstly, the sample was limited to a single field
of study and one university. Conducting several ex-
periments at different universities and taking into ac-
count students from different majors is considered to
be done in the future. Secondly, we believe that the
participants’ motivation to fully engage in the study
was moderate.
REFERENCES
Awang, L., Yusop, F., and Danaee, M. (2016). Current prac-
tices and future direction of artificial intelligence in
mathematics education: A systematic review. Journal
of Mathematics Education, 20(2).
Bieniecki, W., Stolinski, S., and Stando, J. (2010). Au-
tomatic evaluation of examination tasks in the form
of function plot. In 2010 Proceedings of VIth Inter-
national Conference on Perspective Technologies and
Methods in MEMS Design, pages 140–143.
Dalgarno, B. and Lee, M. J. W. (2010). What are the learn-
ing affordances of 3-d virtual environments? British
Journal of Educational Technology, 41(1):10–32.
Du, X. and Li, Z. (2022). A review of the application of
virtual reality technology in higher education based on
web of science literature data as an example. Frontiers
in Education, 7.
Ertmer, P. and Ottenbreit-Leftwich, A. (2010). Teacher
technology change: How knowledge, beliefs, and cul-
ture intersect. Journal of Research on Technology in
Education, 42:255–284.
Johnson-Glenberg, M. (2017). Embodied Education in
Mixed and Mediated Realties, pages 193–217.
Li, X. (2024). Extended reality (xr) in mathematics assess-
ment: A pedagogical vision. Research Matters: A
Cambridge University Press & Assessment publica-
tion, 37:6–23.
L
´
opez, J. and Garcia, M. (2023). Assessing the impact of
virtual reality on mathematics teaching in rural sec-
ondary schools. Journal of Educational Technology,
15(2):123–135.
Makransky, G. and Petersen, G. B. (2021). The cognitive
affective model of immersive learning (camil): A the-
oretical research-based model of learning in immer-
sive virtual reality. Educational Psychology Review,
33(3):937–958.
Nicholson, R., Bartindale, T., Kharrufa, A., Kirk, D.,
and Walker, C. (2022). Participatory design goes to
school: Co-teaching as a form of co-design for educa-
tional technology. pages 1–17.
Qui
˜
nones, D. I. P., Peniche, J., and Rosas, J. (2016). Impact
of virtual reality use on the teaching and learning of
vectors. Frontiers in Education, 7.
ERSeGEL 2025 - Workshop on Extended Reality and Serious Games for Education and Learning
880

Voogt, J., Fisser, P., Pareja Roblin, N., Tondeur, J., and van
Braak, J. (2013). Technological pedagogical content
knowledge – a review of the literature. Journal of
Computer Assisted Learning, 29(2):109–121.
From Coefficients to 3D Planes: A VR Mathematics Module for Teaching Systems of Linear Equations with 3D Interaction
881